Examples Using Formula For Period
Example 1: Using the formula for period, find the period of the function f = 2 sin + 5.
Solution:
We know that the period of the parent function, which is sin, is 2.
The coefficient of x in the given function is 3.
Using the formula for period,
Period, T = / |Coefficient of x|
Period, T = 2 / |3| = 2 / 3
Therefore, The period of f = 2 / 3.
Example 2: Find the period of the function \ = 3 \tan \left \right) – 7\).
Solution:
We know that the period of the parent function, which is tan, is .
The given function can be written as:
\ = 3 \tan \left – 7\)
The coefficient of x in the given function is /2.
Using the formula for period,
Period, T = / |Coefficient of x|
Therefore, the period of f = 2.
Example 3: Using the formula for period, find the period of the function f = 2 sin + 10.
Solution:
We know that the period of the parent function, which is sin, is 2.
The coefficient of x in the given function is 4.
Using the formula for period,
Period, T = / |Coefficient of x|
Period, T = 2 / |4| = 2 / 4 = / 2
Therefore, The period of f = / 2
What Is A Period In Math
The time period for any function f is the instant where the function f repeats its value. In other words, if the function f is plotted, then the time interval between the two points sharing the same occurrence is known as the period for the function.
The mathematical representation of the time period for any function f is given below:
f = f
This representation states that after a specific time interval, the function f will repeat its value.
The concept of time periods is more prominent in trigonometric functions. This is because the graphical representation of these trigonometric functions dominantly represents the areas in the graph sharing the same occurrence.
Period Calculator Math + Online Solver With Free Steps
The Period Calculator Math is an online tool that is used to calculate the period of any function by demonstrating the solution graphically. The Period Calculator Math takes the input from the user, which can be any type of function, and presents the solution.
The Period Calculator Math is a free online tool that provides accurate and quick solutions. This calculator provides the solution in both mathematical as well as in graphical form so the user can understand the solution with ease.
Also Check: What Is Goal Setting In Sport Psychology
Example Question #: Find The Period Of A Sine Or Cosine Function
Please choose the best answer from the following choices.
Find the period of the following function.
Possible Answers:Correct answer:
The period is defined as the length of one wave of the function. In this case, one full wave is 180 degrees or radians. You can figure this out without looking at a graph by dividing with the frequency, which in this case, is 2.
What Is The Period Of Sine Function
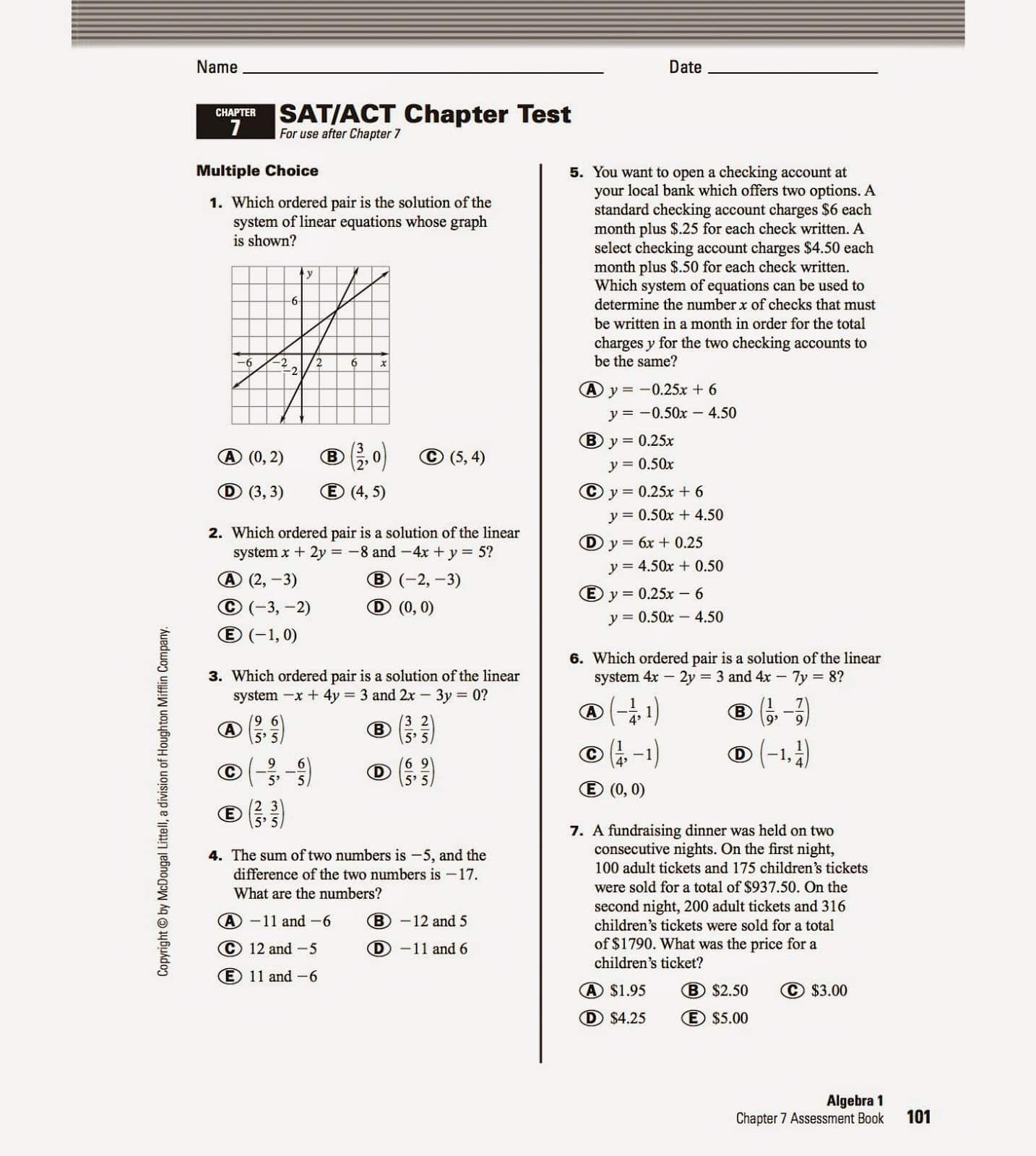
The period of the sine function is 2, which means that the value of the function is the same every 2 units.
The sine function, like cosine, tangent, cotangent, and many other trigonometric function, is a periodic function, which means it repeats its values on regular intervals, or “periods.” In the case of the sine function, that interval is 2.
TL DR
TL DR
The period of the sine function is 2.
For instance, sin = 0. If you add 2 to the x-value, you get sin, which is sin. Just like sin, sin = 0. Every time you add or subtract 2 from our x-value, the solution will be the same.
You can easily see the period on a graph, as the distance between “matching” points. Since the graph of y = sin looks like a single pattern repeated over and over again, you can also think of it as the distance along the x-axis before the graph starts to repeat itself.
On the unit circle, 2 is a trip all the way around the circle. Any amount greater than 2 radians means that you keep looping around the circle that’s the repeating nature of the sine function, and another way to illustrate that every 2 units, the function’s value will be the same.
Don’t Miss: What Is Nu In Chemistry
What Is Meant By Formula For Period
The formula for period is used to calculate the time interval taken by a wave to complete one cycle of vibration at a given point . A periodic function is defined as a function that repeats its values at regular intervals or periods. The period of a function f is p, if f = f, for every x. A function is said to be periodic if its value repeats after regular periods . The formula is Period, P = Period of parent function/ |Coefficient of x|
Rational Function And Birational Equivalence
In contrast to the preceding sections, this section concerns only varieties and not algebraic sets. On the other hand, the definitions extend naturally to projective varieties , as an affine variety and its projective completion have the same field of functions.
If V is an affine variety, its coordinate ring is an integral domain and has thus a field of fractions which is denoted k and called the field of the rational functions on V or, shortly, the function field of V. Its elements are the restrictions to V of the rational functions over the affine space containing V. The domain of a rational function f is notV but the complement of the subvariety where the denominator of f vanishes.
As with regular maps, one may define a rational map from a variety V to a variety V‘. As with the regular maps, the rational maps from V to V‘ may be identified to the field homomorphisms from k to k.
Two affine varieties are birationally equivalent if there are two rational functions between them which are inverse one to the other in the regions where both are defined. Equivalently, they are birationally equivalent if their function fields are isomorphic.
An affine variety is a rational variety if it is birationally equivalent to an affine space. This means that the variety admits a rational parameterization, that is a parametrization with rational functions. For example, the circle of equation x
- }}}\,,}
which may also be viewed as a rational map from the line to the circle.
Read Also: What Does Substrate Mean In Biology
Th And Early 20th Century
It took the simultaneous 19th century developments of non-Euclidean geometry and Abelian integrals in order to bring the old algebraic ideas back into the geometrical fold. The first of these new developments was seized up by Edmond Laguerre and Arthur Cayley, who attempted to ascertain the generalized metric properties of projective space. Cayley introduced the idea of homogeneous polynomial forms, and more specifically quadratic forms, on projective space. Subsequently, Felix Klein studied projective geometry from the viewpoint that the geometry on a space is encoded in a certain class of transformations on the space. By the end of the 19th century, projective geometers were studying more general kinds of transformations on figures in projective space. Rather than the projective linear transformations which were normally regarded as giving the fundamental Kleinian geometry on projective space, they concerned themselves also with the higher degree birational transformations. This weaker notion of congruence would later lead members of the 20th century Italian school of algebraic geometry to classify algebraic surfaces up to birational isomorphism.
The second early 19th century development, that of Abelian integrals, would lead Bernhard Riemann to the development of Riemann surfaces.
How To Use The Period Calculator Math
The Period Calculator Math can be used by entering the function and calculating the period of the function. It is one of the most convenient period calculating tools available online.
The Period Calculator Math has a very simple interface which makes it very easy for the user to obtain the desired solution.
The Period Calculator Math consists of a very user-friendly interface. Its interface consists of a single box entitled f. This input box prompts the user to insert the function for which they wish to calculate the time period.
Once the input function has been inserted, the user simply needs to click on the button that says Calculate for the Period Calculator Math to do its magic.
For a more detailed understanding of using the Period Calculator Math, consider the step-by-step guide given below:
Also Check: Which Of The Following Best Represents Psychology’s Basic Goals
The Important Punctuation Marks The End Of A Sentence
- Ph.D., Rhetoric and English, University of Georgia
- M.A., Modern English and American Literature, University of Leicester
- B.A., English, State University of New York
A period is a punctuation mark indicating a full stop, placed at the end of as well as after many abbreviations. The period is actually called a full stop in British English, according to R.D. Burchfield in “The New Fowler’s Modern English Usage,” and is also known as a full point. Rene J. Cappon, author of “The Associated Press Guide to Punctuation,” explains that the period may appear small but it has an important function in punctuation:
“The period is a mere dot in the panorama of punctuation, but it packs an impressive punch. Unlike, say, the colon or semicolon, it can bring a sentence to a complete halt.”
As Merriam-Webster succinctly defines it: “A period is a point used to mark the end of a declarative sentence or an abbreviation.”
Period Of Trigonometric Functions
When you plot the trigonometric functions on a graph, regularly-repeating wave shapes are produced by the trigonometric functions. Like in any wave, the shapes have recognizable features such as peaks and troughs . The word period tells you the angular distance of one full cycle of the wave that is usually measured between two adjacent peaks or the troughs. For this reason, in Mathematics, you have to measure a functions period in angle units. This is known as the period of trigonometric function
Heres for example, is suppose we start at an angle of zero, the trigonometric function produces a smooth curve that rises to a maximum of a value of 1 at / 2 radians , crosses zero at radians , decreases to a minimum of 1 at 3 / 2 radians and reaches zero again at 2 radians . And after this point, the cycle repeats indefinitely, producing the same features and the same values as the angle increases in the positive x direction.
Recommended Reading: What Makes Something Living Biology
What Is Formula For Period
According to the definition of a period of a function, a function f will be periodic with period p, so if we have f = f , for every p > 0. The period of each of sin x, cos x, csc x, and sec x = 2. The period of each of tan x and cot x = . The period of the wave decreases as its frequency increases. Here is the formula for period of a trigonometric function:
Period, T = Period of parent function/ |Coefficient of x|
Frequency, F = 1/ Period
Example: The period of tan 3x using the period formula is / 3. You can observe this from the following graph also.
Before The 16th Century

Some of the roots of algebraic geometry date back to the work of the Hellenistic Greeks from the 5th century BC. The Delian problem, for instance, was to construct a length x so that the cube of side x contained the same volume as the rectangular box a2b for given sides a and b. Menaechmus considered the problem geometrically by intersecting the pair of plane conics ay = x2 and xy = ab. In the 3rd century BC, Archimedes and Apollonius systematically studied additional problems on conic sections using coordinates.Medieval Muslim mathematicians, including Ibn al-Haytham in the 10th century AD, solved certain cubic equations by purely algebraic means and then interpreted the results geometrically. The Persian mathematician Omar Khayyám discovered a method for solving cubic equations by intersecting a parabola with a circle and seems to have been the first to conceive a general theory of cubic equations. A few years after Omar Khayyám, Sharaf al-Din al-Tusi‘s Treatise on equations has been described by Roshdi Rashed as “inaugurating the beginning of algebraic geometry”. This was criticized by Jeffrey Oaks, who claims that the study of curves by means of equations originated with Descartes in the seventeenth century.
Read Also: What Is Density In Chemistry
The Role Of Complex Numbers
From an algebraic perspective, complex numbers enter quite naturally into the study of algebraic functions. First of all, by the fundamental theorem of algebra, the complex numbers are an algebraically closed field. Hence any polynomial relation p = 0 is guaranteed to have at least one solution for y at each point x, provided we allow y to assume complex as well as real values. Thus, problems to do with the domain of an algebraic function can safely be minimized.
yyxyx
Furthermore, even if one is ultimately interested in real algebraic functions, there may be no means to express the function in terms of addition, multiplication, division and taking nth roots without resorting to complex numbers . For example, consider the algebraic function determined by the equation
- y
, }},} the square root is not real, and one has to choose, for the square root, either non-real square root. Thus the cubic root has to be chosen among three non-real numbers. If the same choices are done in the two terms of the formula, the three choices for the cubic root provide the three branches shown, in the accompanying image.
It may be proven that there is no way to express this function in terms of nth roots using real numbers only, even though the resulting function is real-valued on the domain of the graph shown.
- 1
- d y =}\oint _}y}}\,dy}
which is an analytic function.
Find The Period Of A Sine Function
Say you want to calculate the period of a modified sine function like
The coefficient of x is the key let’s call that coefficient B.
So if you have an equation in the form y = sin, then:
The bars | | mean “absolute value,” so if B is a negative number, you would just use the positive version. If B was 3, for instance, you would just go with 3.
This formula works even if you have a complicated-looking variation of the sine function, like
The coefficient of x is all that matters for calculating the period, so you would still do:
Recommended Reading: What Does Anxiety Mean Psychology
Find The Period Of Any Trig Function
To find the period of cosine, tangent and other trig functions, you use a very similar process. Just use the standard period for the specific function you’re working with when you calculate.
Since the period of cosine is 2, the same as sine, the formula for the period of a cosine function will be the same as it is for sine. But for other trig functions with a different period, like tangent or cotangent, we make a slight adjustment. For example, the period of cot is , so the formula for the period of y = cot is:
where we use instead of 2.
Related Articles
What Is A Period Math
Graph the sine function with changes in amplitude and period.
·Graph the cosine function with changes in amplitude and period.
·Match a sine or cosine function to its graph and vice versa.
Introduction
You know how to graph the functions and. Now youll learn how to graph a whole family of sine and cosine functions. These functions have the form or
Periodic Functions
We used the variable previously to show an angle in standard position, and we also referred to the sine and cosine functions as and. Often the sine and cosine functions are used in applications that have nothing to do with triangles or angles, and the letterxis used instead of for the input . So from this point forward, well refer to these same functions as and. This change does not affect the graphs they remain the same.
You know that the graphs of the sine and cosine functions have a pattern of hills and valleys that repeat. The length of this repeating pattern is. That is, the graph of (or looks like the graph on the interval or. This pattern continues in both directions forever.
The graph below shows four repetitions of a pattern of length. Each one contains exactly one complete copy of the hill and valley pattern.
full cycle on the interval , which is the intervalneeds to complete one full cycle.
You can think of the different values ofbas having an accordion effect on the graphs of sine and cosine. A large value ofbsqueezes them in and a small value ofb
Amplitude
|
Example |
|
Example |
Also Check: What Is Computational Biology And Bioinformatics
Correct And Incorrect Use
Since printers dropped the use of the high and mid-dot centuries ago, the period has actually been the easiest punctuation mark to understand. But it is far from the easiest to use. Punctuation experts note that writers have long struggled with the rules for correctly placing the period. Casagrande gives these tips on the rules and correct use of the period.
Quotation marks: A period always comes before a closing quotation mark. Right: He said, “Get out.” Wrong: He said, “Get out”. Note that this rule applies to American English. British English requires that you place the period after the quotation mark.
Single quotation marks: A period always comes before the closing single quotation mark: He said, “Don’t call me a ‘jerk.'”
Apostrophe: An apostrophe indicates the omission of one or more letters from a word. You do place the period after the apostrophe at the end of a sentence but before the final quote mark: He said, “I know you were just talkin’.”
Ellipses : The AP says you should treat ellipses as a three-letter word, constructed with three periods and bounded by two spaces, as shown here. If the ellipses come after a complete sentence, however, place a period before the ellipses, such as in ‘s famous words: “I have a dream….I have a dream today.”
Initialism: An initialism is an abbreviation that consists of the first letter or letters of words in a phrase, such as EU and NFL . Omit periods from initialisms.