First Known Use Of Plane
Noun
1604, in the meaning defined at sense 2a
Verb
14th century, in the meaning defined at transitive sense 1a
Noun
14th century, in the meaning defined above
Noun
14th century, in the meaning defined above
Verb
15th century, in the meaning defined at sense 1a
Adjective
14th century, in the meaning defined at sense 1
Plane In Geometry Basics
Plane means a tiny word in geometry which means nothing but a surface not having any width or thickness.
- Planes are always two-dimensional.
- The plane can be extended any infinitely far.
- The plane has points or lines.
- It is basically a position, without any thickness.
The plane generally can be represented by
- Plane P, or
- Plane ABCD, etc.
Remember, this A, B, C or D can be written as small letters in many cases.
Some Properties Of Planes
Most basic properties
For any three points not situated in the same straight line there exists one and only one plane that contains these three points.
If two points of a given straight line lie in a given plane, then all points of this line lie in that plane.
If two planes have a common point then they have a second point in common.
Every plane contains three points not lying in the same straight line.
The space contains four points not lying in the same plane.
Further properties
Two planes either do not intersect .html” rel=”nofollow”> parallel), or intersect in a line, or coincide.
A line either does not intersect a plane , or intersects it in a single point, or is contained in the plane.
Two lines perpendicular to the same plane are parallel to each other .
Two planes perpendicular to the same line are parallel to each other .
Recommended Reading: How Much Physics Is On The Mcat
Reflections Across The Line Y = X
A reflection across the line y = x switches the x and y-coordinates of all the points in a figure such that becomes .
Triangle ABC is reflected across the line y = x to form triangle DEF. Triangle ABC has vertices A , B and C . Triangle DEF has vertices D , E , and F . All of the points on triangle ABC undergo the same change to form DEF.
Point Line Plane And Solid
A Point has no dimensions, only positionA Line is one-dimensionalA Plane is two dimensional A Solid is three-dimensional
Plane vs Plain
In geometry a “plane” is a flat surface with no thickness.
But a “plain” is a treeless mostly flat expanse of land … it is also flat, but not in the pure sense we use in geometry.
Both words have other meanings too:
- Plane can also mean an airplane, a level, or a tool for cutting things flat
- Plain can also mean without special things, or well understood
Read Also: Test Form 2b Answers
Why Is Plane Important
The first records of the word plane in a mathematical sense come from the early 1600s. It comes from the Latin plnum, meaning flat surface, which is a noun formed from the Latin adjective plnus, meaning flat.
Planes and many other basics of geometry are often credited to the Greek mathematician Euclid, who lived around the year 300 B.C. Euclid developed the idea of a plane, along with other fundamental concepts of geometry, such as points, lines, and two-dimensional shapes. All two-dimensional geometry exists on a plane. Because of this, geometry that deals with two-dimensional shapes is called plane geometry.
The other major type of geometry is solid geometry, which involves three-dimensional space and shapes, such as cubes and cylinders.
Definition Of A Plane
In geometry, a plane is a flat surface that extends into infinity. It is also known as a two-dimensional surface. A plane has zero thickness, zero curvature, infinite width, and infinite length. It is actually difficult to imagine a plane in real life all the flat surfaces of a cube or cuboid, flat surface of paper are all real examples of a geometric plane. We can see an example of a plane in which the position of any given point on the plane is determined using an ordered pair of numbers or coordinates. The coordinates show the correct location of the points on the plane.
The figure shown above is a flat surface extending in all directions. So, it is a plane.
You May Like: Formula For Volume Physics
Properties Of A Plane
- If there are two different planes than they are either parallel to each other or intersecting in a line, in a three-dimensional space
- A line could be parallel to a plane, intersects the plane at a single point or is existing in the plane.
- If there are two different lines, which are perpendicular to the same plane then they must be parallel to each other.
- If there are two separate planes that are perpendicular to the same line then they must be parallel to each other.
Properties Of A Plane In Geometry
There are few properties of plane, few of them are stated below,
- If there are three non-collinear points, a plane will be formed.
- If two straight lines are parallel, both these lines can for a plane.
- If two lines intersect, then also a plane can be formed.
- If you draw a line, and simultaneously one point you draw which is not on that line, then this line and point can form a plane.
- Collinear points along with one single point which not in the same line as collinear points, will form a plane.
- If you draw a line, that will be parallel to a plane or it will intersect to the plane at a point.
- If you take two or three different lines, and all are perpendicular to a plane, then these lines should be parallel.
- If two different planes, perpendicular to a line, then both the planes should be parallel.
In the geometry, we need to use a segment of planes, so we use plane figure instead of plane and it can be various shapes, like
Don’t Miss: Geometry Mcdougal Littell Answers
Pointnormal Form And General Form Of The Equation Of A Plane
In a manner analogous to the way lines in a two-dimensional space are described using a point-slope form for their equations, planes in a three dimensional space have a natural description using a point in the plane and a vector orthogonal to it to indicate its “inclination”.
Specifically, let r0 be the position vector of some point P0 = , and let n = be a nonzero vector. The plane determined by the point P0 and the vector n consists of those points P, with position vector r, such that the vector drawn from P0 to P is perpendicular to n. Recalling that two vectors are perpendicular if and only if their dot product is zero, it follows that the desired plane can be described as the set of all points r such that
- n
- }=}_+s}+t},}
where s and t range over all real numbers, v and w are given linearly independentvectors defining the plane, and r0 is the vector representing the position of an arbitrary point on the plane. The vectors v and w can be visualized as vectors starting at r0 and pointing in different directions along the plane. The vectors v and w can be perpendicular, but cannot be parallel.
Choose The Right Synonym For Plane
Adjective
level, flat, plane, even, smooth mean having a surface without bends, curves, or irregularities. level applies to a horizontal surface that lies on a line parallel with the horizon. the vast prairies are nearly levelflat applies to a surface devoid of noticeable curvatures, prominences, or depressions. the work surface must be flatplane applies to any real or imaginary flat surface in which a straight line between any two points on it lies wholly within that surface. the plane sides of a crystal even applies to a surface that is noticeably flat or level or to a line that is observably straight. trim the hedge so it is evensmooth applies especially to a polished surface free of irregularities. a smooth skating rink
You May Like: Why There Are Different Branches Of Chemistry
Definition Of Plane In Geometry
A plane in geometry is defined as a two-dimensional flat surface that can be extended infinitely far.
- The plane is sometimes called a two-dimensional surface.
- A plane consists of zero thickness, zero curvature but infinite width and length.
A plane is the analogue of three main things,
- Point
- 3-dimensional space
Definition Of Plane In Algebra
In the definition of the plane in geometry, we have extended the plane infinitely but what about the plane in algebra? Is it the same thing or different?
Lets check!
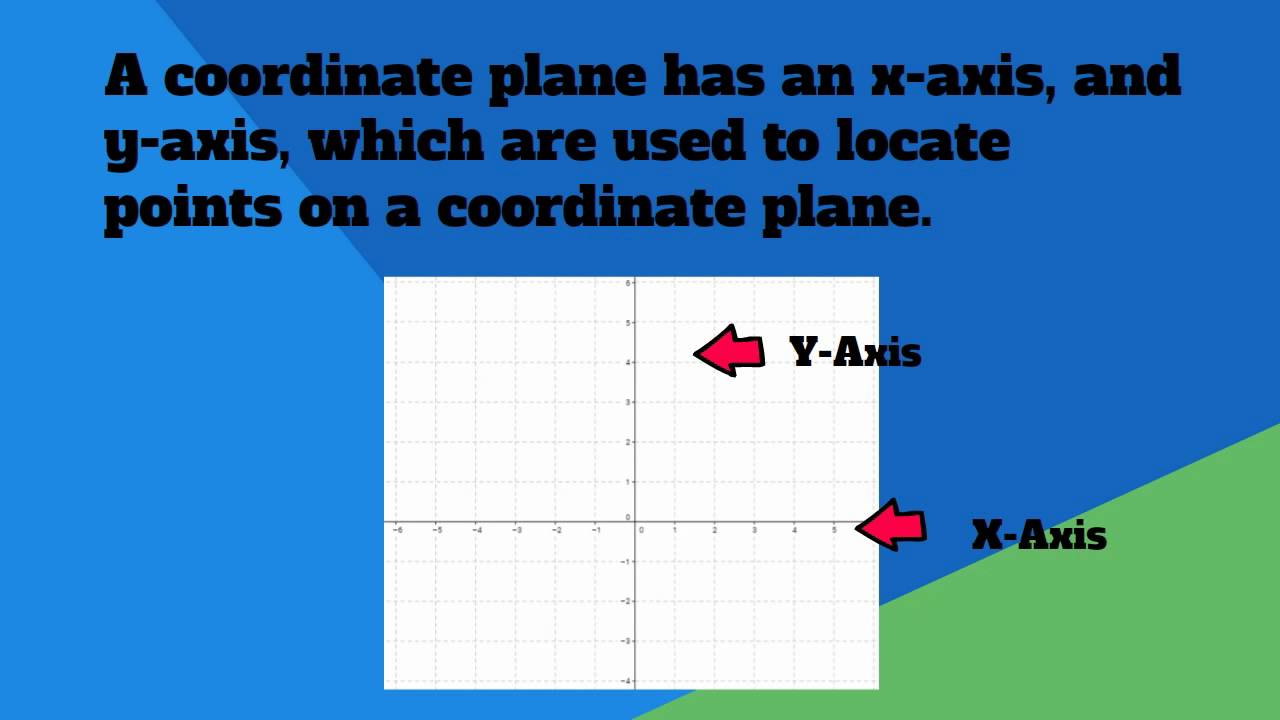
We need to do a lot of calculations, and we use the coordinate plane to plot the points, lines as well as planes.
- Points are marked in two-dimensional areas, like
- In this two-dimensional plot, there are two axes, one is the x-axis and another is the y-axis. Normally, the x-axis denotes the horizontal axis and the y-axis denotes the vertical axis.
- With the help of points, the plane can be drawn easily.
- Remember, any plane in algebra may contain several lines.
- All lines in the plane have arrow marks to indicated it as extended endlessly.
Check out a NICE VIDEO from Mathantics,
Don’t Miss: Beth Thomas Brother Now
Topological And Differential Geometric Notions
The one-point compactification of the plane is homeomorphic to a sphere the open disk is homeomorphic to a sphere with the “north pole” missing adding that point completes the sphere. The result of this compactification is a manifold referred to as the Riemann sphere or the complexprojective line. The projection from the Euclidean plane to a sphere without a point is a diffeomorphism and even a conformal map.
The plane itself is homeomorphic to an open disk. For the hyperbolic plane such diffeomorphism is conformal, but for the Euclidean plane it is not.
What Is R3 In Math
4.5/5R3R3
In respect to this, what is r3 in linear algebra?
If three mutually perpendicular copies of the real line intersect at their origins, any point in the resulting space is specified by an ordered triple of real numbers . The set of all ordered triples of real numbers is called 3-space, denoted R 3 .
Furthermore, what is r n Math? In mathematics, real coordinate space of n dimensions, written Rn is a coordinate space that allows several real variables to be treated as a single variable.
Also Know, what does R mean in matrices?
INTRODUCTION Linear algebra is the math of vectors and matrices. Let n be a positive integer and let R denote the set of real numbers, then Rn is the set of all n-tuples of real numbers. A vector v Rn is an n-tuple of real numbers.
What does R mean in vectors?
Vector is a basic data structure in R. It contains element of the same type. The data types can be logical, integer, double, character, complex or raw. A vector’s type can be checked with the typeof function. Another important property of a vector is its length.
Don’t Miss: Similar Math Definition
An Introduction To Geometry
A point in geometry is a location. It has no size i.e. no width, no length and no depth. A point is shown by a dot.
A line is defined as a line of points that extends infinitely in two directions. It has one dimension, length. Points that are on the same line are called collinear points.
A line is defined by two points and is written as shown below with an arrowhead.
$$\\ \overset \\$$
Two lines that meet in a point are called intersecting lines.
A part of a line that has defined endpoints is called a line segment. A line segment as the segment between A and B above is written as:
$$\overline$$
A plane extends infinitely in two dimensions. It has no thickness. An example of a plane is a coordinate plane. A plane is named by three points in the plane that are not on the same line. Here below we see the plane ABC.
A space extends infinitely in all directions and is a set of all points in three dimensions. You can think of a space as the inside of a box.
Planes In Various Areas Of Mathematics
In addition to its familiar geometric structure, with isomorphisms that are isometries with respect to the usual inner product, the plane may be viewed at various other levels of abstraction. Each level of abstraction corresponds to a specific category.
At one extreme, all geometrical and metric concepts may be dropped to leave the topological plane, which may be thought of as an idealized homotopically trivial infinite rubber sheet, which retains a notion of proximity, but has no distances. The topological plane has a concept of a linear path, but no concept of a straight line. The topological plane, or its equivalent the open disc, is the basic topological neighborhood used to construct surfaces classified in low-dimensional topology. Isomorphisms of the topological plane are all continuousbijections. The topological plane is the natural context for the branch of graph theory that deals with planar graphs, and results such as the four color theorem.
The plane may also be viewed as an affine space, whose isomorphisms are combinations of translations and non-singular linear maps. From this viewpoint there are no distances, but collinearity and ratios of distances on any line are preserved.
In the opposite direction of abstraction, we may apply a compatible field structure to the geometric plane, giving rise to the complex plane and the major area of complex analysis. The complex field has only two isomorphisms that leave the real line fixed, the identity and conjugation.
You May Like: Algebra 1 Warm Ups
Naming Of Planes In Geometry
Planes in geometry are usually referred to as a single capital letter in italics, for example, in the diagram below, the plane could be named UVW or plane P.
Important Notes
- Any three non-collinear points lie on one and only one plane.
- Two planes always intersect along a line, unless they are parallel.
- A plane is named by three points in that plane that are not on the same line.
History And Etymology For Plane
Noun
Latin planum, from neuter of planus level
Verb
Middle English, from Anglo-French planer, from Late Latin planare, from Latin planus level more at floor
Noun
Middle English, from Anglo-French, from Late Latin plana, from planare
Noun
Middle English, from Anglo-French, from Latin platanus, from Greek platanos probably akin to Greek platys broad more at place
Verb
Middle English, from Middle French planer, from plain level, plain
Adjective
Read Also: Algebra 2 Simplifying Radicals With Variables Worksheet Answers
What Is Boundary Line In Math
4.4/5BoundaryBoundary lines in math
Besides, what does boundary line mean?
Noun. 1. boundary line – a line that indicates a boundary. border, borderline, delimitation, mete. boundary, bounds, bound – the line or plane indicating the limit or extent of something.
Beside above, what are boundary points on number lines? The resulting values of x are called boundary points or critical points. Plot the boundary points on the number line, using closed circles if the original inequality contained a or sign, and open circles if the original inequality contained a < or > sign.
Also to know, what does it mean when the boundary line is solid?
This means that the solutions are NOT included on the boundary line. If the inequality symbol is greater than or equal to or less than or equal to, then you will use a solid line to indicate that the solutions are included on the boundary line.
What is an example of boundary?
The definition of a boundary is a line or something else that marks a limit or border. An example of boundary is a barbed wire fence.
What Is A Plane Figure
A plane figure is defined as a geometric figure that has no thickness. It lies entirely in one plane. It is possible to form a plane figure c with line segments, curves, or a combination of these two, i.e. line segments and curves. Lets have a look at some examples of plane figures in geometry such as circle, rectangle, triangle, square and so on. These are given in the below figure.
Put your understanding of this concept to test by answering a few MCQs. Click Start Quiz to begin!
Select the correct answer and click on the Finish buttonCheck your score and answers at the end of the quiz
Don’t Miss: Geometry Basics Segment Addition Postulate
Example Of Plane In Geometry
We can understand planes with few examples, like walls in our room or our books or tables.
- Take your physics book , and just keep it on the table.
- Now the book is closed and it is a plane.
- Take another book and hold above the first book
- The height is equal at all the corners.
Take your physics book, and just keep it on the table. Now the book is closed and you touch its surface. This is nothing but a part of the plane. If you imagine that this surface is not limited to the book itself, just increase the surface far, you will get a plane.
You can do the same with the wall. Just extend the wall and you will see the plane!
If you imagine that this surface is not limited to the book itself, just increase the surface far, you will get a plane.
You can do the same with the wall. Just extend the wall and you will see the plane!