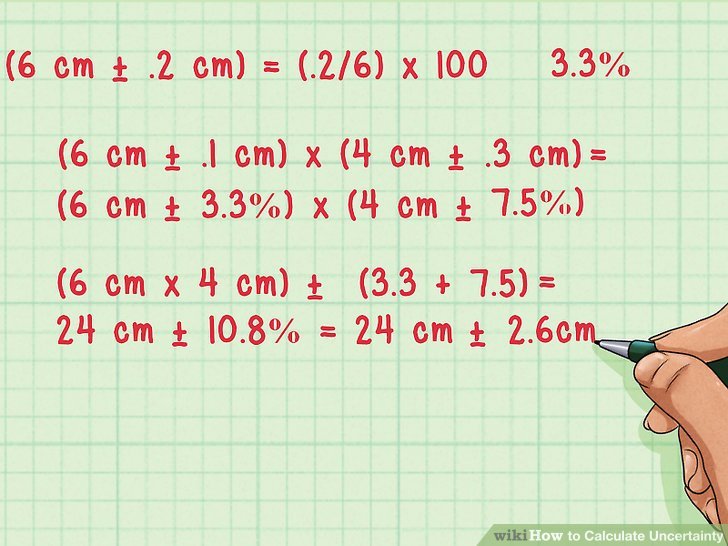
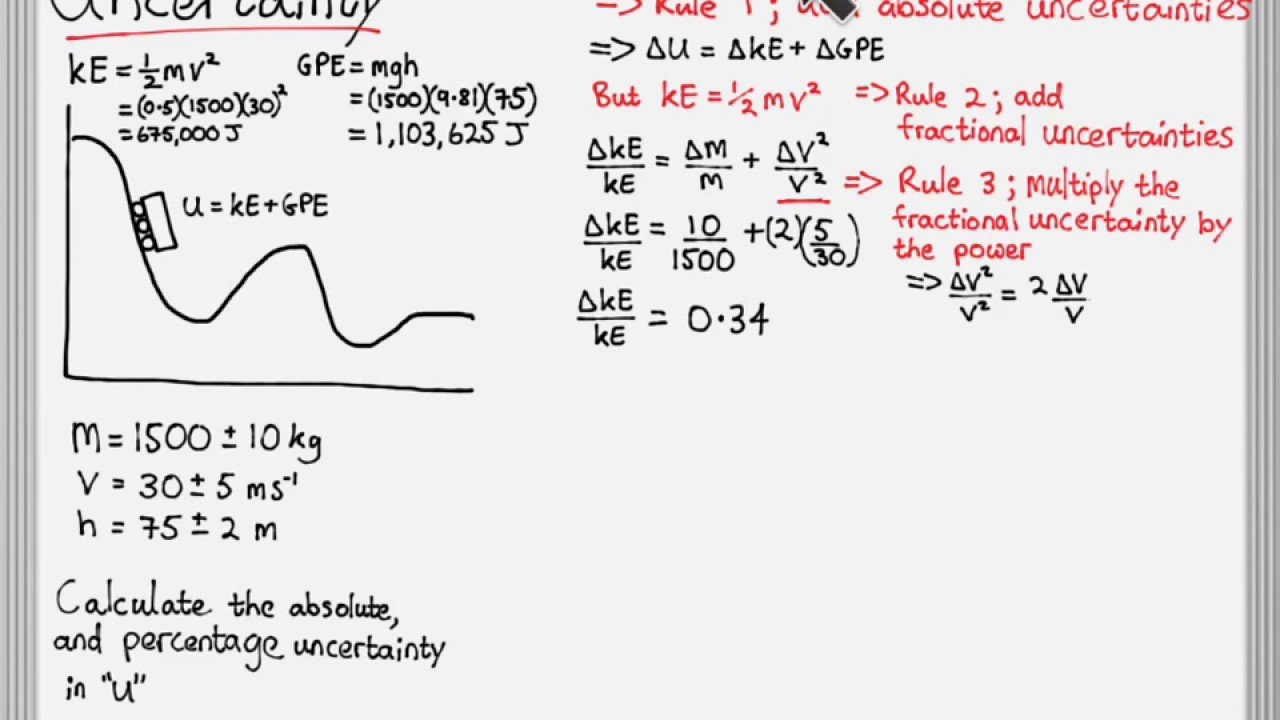
Perform Arithmetic Operations With Uncertain Measurements
Recommended Reading: Geometry Segment Addition Postulate Worksheet
Distinguish Between Fundamental And Derived Units And Give Examples Of Derived Units
In order to express certain quantities we combine the SI base units to form new ones. For example, if we wanted to express a quantity of speed which is distance/time we write m/s . For some quantities, we combine the same unit twice or more, for example, to measure area which is length x width we write m2.
Certain combinations or SI units can be rather long and hard to read, for this reason, some of these combinations have been given a new unit and symbol in order to simplify the reading of data.For example: power, which is the rate of using energy, is written as kg m2 s-3. This combination is used so often that a new unit has been derived from it called the watt .
Below is a table containing some of the SI derived units you will often encounter:
| N m-2 |
Calculate The Combined Uncertainty
After converting your uncertainty sources to standard deviation equivalents, it is time to calculate the combined uncertainty using the root sum of squares method recommended in the Guide to the Expression of Uncertainty in Measurement .
This will mathematically combine your uncertainty sources in quadrature. So, keep reading to learn how to combine uncertainty.
Dont Miss: What Is Figure Ground Perception Psychology
Read Also: Elasticity Formula Physics
Absolute Vs Relative Uncertainties
Quoting your uncertainty in the units of the original measurement for example, 1.2 ± 0.1 g or 3.4 ± 0.2 cm gives the absolute uncertainty. In other words, it explicitly tells you the amount by which the original measurement could be incorrect. The relative uncertainty gives the uncertainty as a percentage of the original value. Work this out with:
So in the example above:
The value can therefore be quoted as 3.4 cm ± 5.9%.
Selected Solutions To Problems & Exercises
1. 57.9 m/s 9.55 × 109 eV From Table 1 in Photon Energies and the Electromagnetic Spectrum, we see that typical molecular binding energies range from about 1eV to 10 eV, therefore the result in part is approximately 9 orders of magnitude smaller than typical molecular binding energies.
3. 29 nm 290 times greater
- College Physics. : OpenStax College. Located at: . License: CC BY: Attribution. License Terms: Located at License
Recommended Reading: Geometry Dash Demon Key Hack
Excel Function For Combining Uncertainty
If you use Microsoft Excel to estimate uncertainty, you can easily combine uncertainty using the formula below. It is a combination of the square root and sum of squares function.
=sqrt)
Take a look at the image below to see the function used in my Simple Uncertainty Calculator.
If you want to learn more about calculating the combined uncertainty, click the link to read the guide:
Note:
To meet ISO/IEC 17025:2017 requirements, you must expand uncertainty to approximately 95%. Most people use an expansion factor of 2 to achieve a confidence interval of 95.45%. However, you can also use an expansion factor of 1.96 for a confidence interval of exactly 95.00%.
Additionally, you can find your coverage factor using the Students T table. This is not common, but it is an option if you need it. Just calculate the effective degrees of freedom using the Welch Satterthwaite equation and use the table to find the right coverage factor to achieve a 95% confidence interval.
The choice is yours. Just make sure to select an expansion factor that you will consistently use in each of your uncertainty analyses. Furthermore, it helps to know why you chose your expansion factor so you can justify it to assessors .
TIP:
Heisenberg Uncertainty For Energy And Time
There is another form of Heisenbergs uncertainty principle for simultaneous measurements of energy and time. In equation form, \Delta\Delta\ge\frac\\, where E is the uncertainty in energy and t is the uncertainty in time. This means that within a time interval t, it is not possible to measure energy preciselythere will be an uncertainty E in the measurement. In order to measure energy more precisely , we must increase t. This time interval may be the amount of time we take to make the measurement, or it could be the amount of time a particular state exists, as in the next Example 2.
Recommended Reading: Draw The Lewis Structure For Ccl4
Also Check: Half Life Formula Chemistry
Finding Sources Of Uncertainty
Finding sources of uncertainty can be difficult. It requires a lot of time and effort to conduct research. It is the most time-consuming process when evaluating measurement uncertainty.
Based on my experience, finding factors that influence uncertainty typically requires 50% of the time you spend estimating uncertainty. Take a look at the graph below to see how you will typically spend your time estimating uncertainty.
However, if you spend time evaluating your process and conducting research, you should be able to identify several sources of uncertainty for your analysis. Afterward, make a list of these items. You will attempt to quantify them later.
Pro-Tip:
What About Indirect Measurements
If you are performing indirect measurements that require you to calculate your measurement results, then you should evaluate the equation used to determine your measurement result. Each variable in the equation will have its own uncertainty that will directly affect the uncertainty associated with the calculated measurement result.
To help you out, think of using dead weight testers or calibrating torque transducers and standard resistors. Each one of these measurement processes require you to use an equation to calculate a result for comparison purposes. To estimate uncertainty, you will want to break down the equation and evaluate the uncertainty of each variable in the equation.
If you want to learn more about specifying the measurement function and process for your uncertainty analysis, check out this guide:
Recommended Reading: What Is Elastic Force In Physics
Mean Standard Deviation And Standard Uncertainty
Brief summary: the lecture explains calculation of mean and standard deviation . Illustrates again the 68% probability of s. Explains how the standard uncertainty of repeatability u can be estimated as standard deviation of parallel measurement results. Stresses the importance of standard uncertainty as the key parameter in carrying out uncertainty calculations: uncertainties corresponding to different sources and to different distribution functions are converted to standard uncertainties when uncertainty calculations are performed.
Mean, standard deviation and standard uncertainty
One of the most common approaches for improving the reliability of measurements is making replicate measurements of the same quantity. In such a case very often the measurement result is presented as the mean value of the replicate measurements. In the case of pipetting n times with the same pipette volumes V1, V2, , Vn are obtained and the mean value Vm is calculated as follows:
As explained in section 3.1, the mean value calculated this way is an estimate of the true mean value .
The scatter of values obtained from repeated measurements is characterized by standard deviation of pipetted volumes, which for the same case of pipetting is calculated as follows:
Two important interpretations of the standard deviation:
Convert Between Different Units Of Quantities
Often, we need to convert between different units. For example, if we were trying to calculate the cost of heating a litre of water we would need to convert between joules and kilowatt hours , as the energy required to heat water is given in joules and the cost of the electricity used to heat the water is a certain price per kW h.
If we look at table 1.2.2, we can see that one watt is equal to a joule per second. This makes it easy to convert from joules to watt hours: there are 60 second in a minutes and 60 minutes in an hour, therefor, 1 W h = 60 x 60 J, and one kW h = 1 W h / 1000 .
Read Also: Geometry Segment Addition Postulate Worksheet
Estimating Uncertainty From A Single Measurement
In many circumstances, a single measurement of a quantity is often sufficient for the purposes of the measurement being taken. But if you only take one measurement, how can you estimate the uncertainty in that measurement? Estimating the uncertainty in a single measurement requires judgement on the part of the experimenter. The uncertainty of a single measurement is limited by the precision and accuracy of the measuring instrument, along with any other factors that might affect the ability of the experimenter to make the measurement and it is up to the experimenter to estimate the uncertainty .
State Random Uncertainty As An Uncertainty Range And Represent It Graphically As An Error Bar
In IB physics, error bars only need to be used when the uncertainty in one or both of the plotted quantities are significant. Error bars are not required for trigonometric and logarithmic functions.
To add error bars to a point on a graph, we simply take the uncertainty range and draw lines of a corresponding size above and below or on each side of the point depending on the axis the value corresponds to.
Example:
Plot the following data onto a graph taking into account the uncertainty.
Table 1.2.1 Distance vs Time data
Figure 1.2.2 Distance vs. time graph with error bars
In practice, plotting each point with its specific error bars can be time consuming as we would need to calculate the uncertainty range for each point. Therefor, we often skip certain points and only add error bars to specific ones. We can use the list of rules below to save time:
- Add error bars only to the first and last points
- Only add error bars to the point with the worst uncertainty
- Add error bars to all points but use the uncertainty of the worst point
- Only add error bars to the axis with the worst uncertainty
Recommended Reading: Write The Segment Addition Postulate For The Points Described
Measurement Functions Without Equations
Most of the measurement functions that you evaluate will not have equations. So, you will need to evaluate the measurement process to find the factors that influence measurement uncertainty.
Start by evaluating the core elements of the measurement process, including the:
Using the items in the list above, you should be able determine how much uncertainty is contributed from each source. If you need help, you can contact me for additional guidance or hire me to analyze the data for you.
Evaluate Information and Select the Right Data
Next, you need evaluate the information that you have and find data that you will use for estimating uncertainty. You need to find data related to your uncertainty analysis and eliminate everything else from consideration.
This should include information and data related to your:
Analyze the Data
Then, analyze the data that you have using appropriate methods of analysis to find the magnitude of each uncertainty component. You can analyze data in many ways, so choose methods that are appropriate for the data you are analyzing.
If you need help, get a quality statistics textbook or check the free NIST SEMATECH Engineering Statistics Handbook. You can also check out some of my guides on quantifying sources of uncertainty.
Quantify Uncertainty Components
You can add the uncertainty and unit of measurement directly into your uncertainty budget.
What Is Uncertainty Formula
In statistical parlance, the term uncertainty is associated with a measurement where it refers to the expected variation of the value, which is derived from an average of several readings, from the true mean of the data set or readings. In other words, the uncertainty can be considered as the standard deviation of the mean of the data set. The formula for uncertainty can be derived by summing up the squares of the deviation of each variable from the mean, then divide the result by the product of the number of readings and the number of readings minus one and then compute the square root of the result. Mathematically, Uncertainty Formula is represented as,
Uncertainty =
Where,
- xi= ith reading in the data set
- = Mean of the data set
- n = Number of readings in the data set
Don’t Miss: Algebra Age Problems
How Do You Calculate Uncertainty In Chemistry
The uncertainty of a measuring instrument is estimated as plus or minus half the smallest scale division. For a thermometer with a mark at every 1.0°C, the uncertainty is ± 0.5°C. This means that if a student reads a value from this thermometer as 24.0°C, they could give the result as 24.0°C ± 0.5°C.
Examples Of Uncertainty Formula
Lets take an example to understand the calculation of Uncertainty in a better manner.
Uncertainty Formula Example #1
Let us take the example of a 100m race in a school event. The race was timed using five different stopwatches and each stopwatch recorded slightly different timing. The readings are 15.33 seconds, 15.21 seconds, 15.31 seconds, 15.25 seconds and 15.35 seconds. Calculate the uncertainty of the timing based on the given information and present the timing with 68% confidence level.
Solution:
Now, we need to calculate the deviations of each reading
Similarly, calculate for all the readings
Calculate the square of the deviations of each reading
Uncertainty is calculated using the formula given below
Uncertainty =
- Uncertainty = 0.03seconds
68% of values fall within 1 standard deviation of the mean
So Timing at 68% confidence level = ± 1 * u
- Measurement at 68% confidence level = seconds
- Measurement at 68% confidence level = seconds
Therefore, the uncertainty of the data set is 0.03 seconds and the timing can be represented as seconds at 68% confidence level.
Uncertainty Formula Example #2
Solution:
Now, we need to calculate the deviations of each reading
Similarly, calculate for all the readings
Calculate the square of the deviations of each reading
Uncertainty is calculated using the formula given below
Uncertainty =
You May Like: Sacred Geometry Moon Phases Tattoo Spine
Root Sum Of Squares Method
If you are a more visual learner, like me, take a look at the process below to see if it makes more sense.
Below, you will see the equation for calculating the combined uncertainty.
This is the equation that I typically use since I usually include sensitivity coefficients earlier in the process before I convert uncertainty components to standard deviations.
If you evaluate measurement uncertainty the same way, you should be able to use the simplified equation. If you do not use sensitivity coefficients at all, you can use the simplified equation as well.
Both equations give you the same result. So, use the equation that works best for you. If you are using an excel spreadsheet calculator, you may find the function in the next section beneficial.
Systematic And Random Errors
accuracyprecisionrange of possible true values
Why should repeated measurements of a single quantity give different values? Mistakes on the part of the experimenter are possible, but we do not include these in our discussion. A careful researcher should not make mistakes!
We use the synonymous terms uncertainty, error, or deviation to represent the variation in measured data. Two types of errors are possible. Systematic error is the result of a mis-calibrated device, or a measuring technique which always makes the measured value larger than the “true” value. An example would be using a steel ruler at liquid nitrogen temperature to measure the length of a rod. The ruler will contract at low temperatures and therefore overestimate the true length. Careful design of an experiment will allow us to eliminate or to correct for systematic errors.
Even when systematic errors are eliminated there will remain a second type of variation in measured values of a single quantity. These remaining deviations will be classed as random errors, and can be dealt with in a statistical manner. This document does not teach statistics in any formal sense, but it should help you to develop a working methodology for treating errors.
You May Like: Introduction To Exponential Functions Algebra 1 Homework
What Divisor To Use To Convert Uncertainty
To convert uncertainty to standard deviations, it is best to understand more about probabilities distributions and their associated divisors.
Normal Distribution
If you select a Normal distribution, then you will divide your uncertainty by its associated coverage factor, k.Use the Table from the JCGM 100:2008, Appendix G.
Take a closer look at the sources of uncertainty that you are evaluating to determine what coverage factor you should use. Typically, your contributors will have a confidence level of 68%, 95%, or 99%. Respectively, this means that you will use a divisor of 1, 2, or 2.576.
Rectangular Distribution
If you select a Rectangular distribution, then you will divide your uncertainty component by the square root of 3 or 1.7321.
U-Shaped Distribution
If you select a U-shaped distribution, then you will divide your uncertainty component by the square root of 2 or 1.4142.
Triangular Distribution
If you select a Triangular distribution, then you will divide your uncertainty component by the square root of 6 or 2.4495.
Quadratic Distribution
If you select a Quadratic distribution, then you will divide your uncertainty component by the square root of 5 or 2.2361.
If you select a Rayleigh distribution, then you will divide your uncertainty component by 2.4477.