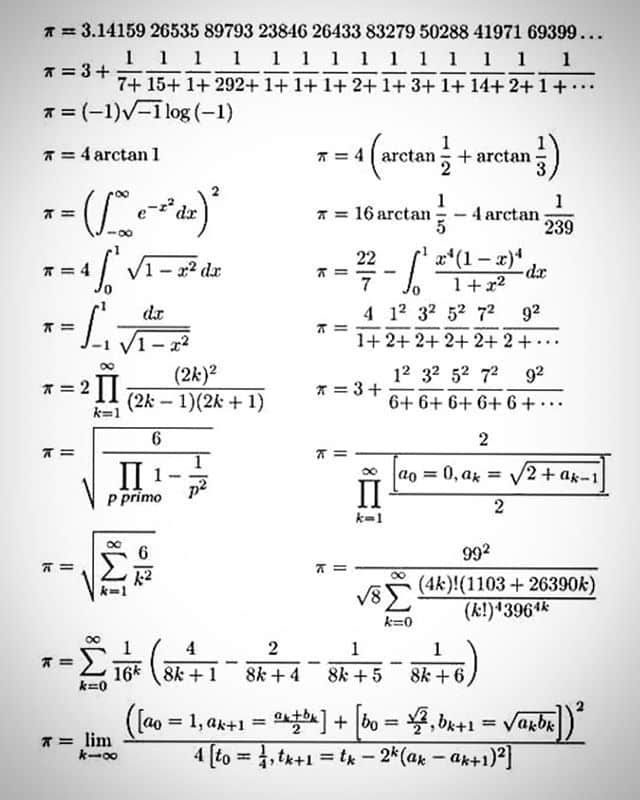
Why Is This Symbol Used To Represent Pi Where Did It Come From
The use of pi is dated back to the Babylonians about 4,000 years ago with calculations for the area of a circle. At that time, an approximation of 3 times the square of the radius of the circle was used, which returned a value of pi = 3. Egyptian mathematicians approximated pi with a bit more precision at 3.1605, as indicated in the Rhind Papyrus, which dates back to 1,650 B.C.
The Greek mathematician Archimedes used a visually creative approach to approximate pi by using the areas of two polygons. To envision this method, picture a polygon having so many sides that it resembles a circle in shape. With a lot of patience and grit, the area of such a polygon can be determined by constructing right triangles inside, using the Pythagorean Theorem to determine the side lengths, calculating the area of each triangle, and then adding them up.
Archimedes used this approach to estimate the area of the circle that could be drawn between two such polygons, with one being just smaller than the other. From there, he was able to approximate the value of pi to be between \ and \.
Before the pi symbol was used, pi was described as the quantity which, when the diameter is multiplied by it, yields the circumference and other long, roundabout descriptions.
Mapping Out A Better Society With Focus On Inclusion Environment
For centuries, pi the ratio of a circles circumference to its diameter has fascinated mathematicians and scientists. The number, which is infinite but never falls into a repeating pattern, is used in formulae throughout the sciences. For more perspective on the significance and fascination with the number, for Pi Day the Gazette spoke with Jacob Barandes, a lecturer and director of graduate studies for physics.
Happy Pi Day: 5 Ways Nasa Uses Pi
Got circles on the brain today? Its Pi Day and a celebration of math and science as well as the infinite and irrational! It is also Albert Einsteins birthday. Whats Pi? is the 16th letter in the Greek alphabet and is used to represent a mathematical constant, the ratio of a circles circumference to its diameter, approximately equal to 3.1415
In basic mathematics, Pi is used to find area and circumference of a circle. You might not use it yourself every day, but Pi is used in most calculations for building and construction, quantum physics, communications, music theory, medical procedures, air travel, and space flight, to name a few.You might imagine that NASA regularly uses to calculate trajectories of spacecraft. Above is a visible documentation of a technique called a pi transfer used by the Cassini spacecraft to complete a maneuver to fly by Saturns moon Titan flyby.
NASA explains:
A pi transfer uses the gravity of Saturns largest moon, Titan, to alter the orbit of the Cassini spacecraft so it can gain different perspectives on Saturn and achieve a wide variety of science objectives. During a pi transfer, Cassini flies by Titan at opposite sides of its orbit about Saturn and uses Titans gravity to change its orbital perspective on the ringed planet.
This image was taken on January 19, 2007, showing the perspective the spacecraft had of Saturn and its rings during the pi transfer.
Recommended Reading: Can You Use Tan Physics On Your Face
Is $\pi^2 \approx G$ A Coincidence
In spite of their different dimensions, the numerical values of $\pi^2$ and $g$ in SI units are surprisingly similar, $$\frac\approx 1.00642$$
After some searching, I thought that this fact isn’t a coincidence, but an inevitable result of the definition of a metre, which was possibly once based on a pendulum with a one-second period.
However, the definition of a metre has changed and is no longer related to a pendulum , but $\pi^2 \approx g$ still holds true after this vital change. This confused me: is $\pi^2 \approx g$ a coincidence?
My question isn’t about numerology, and I don’t think the similarity between the constant $\pi^2$ and $g$ of the planet we live on reflects divine power or anything alike – I consider it the outcome of the definitions of SI units. This question is, as @Jay and @NorbertSchuch pointed out in their comments below, mainly about units and somewhat related to the history of physics.
- 3 Carl WitthoftAug 31, 2016 at 18:51
- $\begingroup$I mean, really, why is it that “Earth weighs almost exactly milliJupiters” — what-if #146, note 7 . There are dozens of apparent coincidences involving fundamental constants and transcendental numbers none of them mean anything. Read James Randi’s work.$\endgroup$
The differential equation for a pendulum is
$$\ddot = -\frac\cdot\sin$$
If you solve this, you will get$$\omega = \sqrt}$$or$$T_=\pi\sqrt}$$$$g=\pi^2\frac^2}$$
I find two useful clues in their report. They point in opposite directions.
First, they say
Quantum Physicists Find Pi In A Very Strange Place
A pair of scientists from the University of Rochester has discovered something unexpected lurking far down in the mathematical depths of quantum mechanics: pi.
To be sure, pi, the endlessly repeating constant describing the ratio between a circle’s circumference and its diameter, underpins much of what we understand about the physical and mathematical worlds, including quantum mechanics. But it wasn’t expected to appear just out of the blue in calculations for the excited energy states of electrons in an atom, which is just what the Rochester physicists describe in a new paper published in the Journal of Mathematical Physics.
“The existence of such a derivation indicates that there are striking connections between well-established physics and pure mathematics that are remarkably beautiful yet still to be discovered,” the authors write.
Electron shells in various atoms. Image: Wikimedia Commons
They didn’t quite find pi in the form you or I normally consider it, which is just the number 3.14 with an endless string of junk after it. Instead, they found what’s known as the Wallis formula. Rather than looking at pi as decimal digits, the Wallis formula instead imagines it as the product of an endless string of ratios between two integers.
So, how do we get from quantum mechanics to that thing above?
Wallis formula calculations. Image: Google
Read Also: What Does Synthesis Mean In Biology
Fourier Transform And Heisenberg Uncertainty Principle
The constant also appears as a critical spectral parameter in the Fourier transform. This is the integral transform, that takes a complex-valued integrable function f on the real line to the function defined as:
Although there are several different conventions for the Fourier transform and its inverse, any such convention must involve somewhere. The above is the most canonical definition, however, giving the unique unitary operator on L2 that is also an algebra homomorphism of L1 to L.
The Heisenberg uncertainty principle also contains the number . The uncertainty principle gives a sharp lower bound on the extent to which it is possible to localize a function both in space and in frequency: with our conventions for the Fourier transform,
The physical consequence, about the uncertainty in simultaneous position and momentum observations of a quantum mechanical system, is discussed below. The appearance of in the formulae of Fourier analysis is ultimately a consequence of the Stonevon Neumann theorem, asserting the uniqueness of the Schrödinger representation of the Heisenberg group.
A Classic Formula For Pi Was Discovered Hidden In Hydrogen Atoms
Back in 2015, scientists found something amazing for the first time – a classic formula for pi hidden in the world of quantum physics.
Pi is the ratio between a circle’s circumference and its diameter, and is incredibly important in pure mathematics. But this was the first time scientists had found it “lurking” in the world of physics, when using quantum mechanics to compare the energy levels of a hydrogen atom.
Why was that exciting? Well, it revealed an incredibly special and previously unknown connection between quantum physics and maths.
“I find it fascinating that a purely mathematical formula from the 17th century characterises a physical system that was discovered 300 years later,” said one of the lead researchers at the time, Tamar Friedmann, a mathematician at the University of Rochester in the US.
The discovery was made when Carl Hagen, a particle physicist at the University of Rochester, was teaching a class on quantum mechanics and explaining to his students how to use a quantum mechanical technique known as the ‘variation principle’ to approximate the energy states of a hydrogen atom.
While comparing these values to conventional calculations, he noticed an unusual trend in the ratios.
He asked Friedmann to help him work out this trend, and they quickly realised that it was actually a manifestation of the Wallis formula for pi the first time it had even been derived from physics.
Digitised by Google
Also Check: Geometry Dash Mega Hack Tool
Modular Forms And Theta Functions
The constant is connected in a deep way with the theory of modular forms and theta functions. For example, the Chudnovsky algorithm involves in an essential way the j-invariant of an elliptic curve.
The constant is the unique normalizing factor such that H defines a linear complex structure on the Hilbert space of square-integrable real-valued functions on the real line. The Hilbert transform, like the Fourier transform, can be characterized purely in terms of its transformation properties on the Hilbert space L2: up to a normalization factor, it is the unique bounded linear operator that commutes with positive dilations and anti-commutes with all reflections of the real line. The constant is the unique normalizing factor that makes this transformation unitary.
How Do You Calculate Pi
Pi is an irrational number — a number with an unending string of non-repeating digits after the decimal point. While it has been calculated to more than 10 trillion places, most of the time just a few decimal places will do. We’ll look at two different ways to calculate pi: By measuring a circle and by solving a mathematical equation.
Read Also: What Is Sunshine In Geography
Why Do $\pi/2$ Pulses Make A $\pi$ Pulse
This is the topic Ramsey interferometry. I want to do this without referencing the Bloch sphere, just with the Hamiltonian and Time-Dependent Schrodinger Equation.
A pi/2 pulse in a two-level system is defined as a pulse that sends the ground state $$| g \rangle \rightarrow \frac}.$$ Two of them are supposed to just give the excited state $| e \rangle$, ie a pi pulse.
Using this rule, if you compose two pi/2 pulses you then get something like $$\frac} \rightarrow \frac} +| e \rangle).$$ I know time evolution of a Hamiltonian is needed, but I got that it only adds an overall phase and the same thing is found.
How the heck is this a pi pulse ?
A $\pi/2$-pulse can be represented by the unitary matrix
$$U_=\frac}\begin1 & 1\\-1 & 1\end$$and the ground and excited states by the vectors$$|g\rangle=\begin0\\1 \end \qquad\text, \qquad|e\rangle=\begin1\\0 \end.$$Which makes the application of the $\pi/2$-pulse on the ground state$$U_|g\rangle=\frac}\left.$$Applying a second $\pi/2$-pulse gives$$\beginU_U_|g\rangle=& \frac}U_\left\\=& \frac\left\right)\\=& |e\rangle\end.$$
In your last transformation, you have not considered the application of the $\pi/2$-pulse on the excited state.
What Is The Formula For Calculating Pi
The pi is a ratio and is obtained from a circle. If the diameter and the circumference of a circle are known, the value of pi will be as = Circumference/ Diameter.
Put your understanding of this concept to test by answering a few MCQs. Click Start Quiz to begin!
Select the correct answer and click on the Finish buttonCheck your score and answers at the end of the quiz
Also Check: What Does Cpctc Stand For In Math
Computer Era And Iterative Algorithms
. +b_)^}}}.}
The development of computers in the mid-20th century again revolutionized the hunt for digits of . Mathematicians John Wrench and Levi Smith reached 1,120 digits in 1949 using a desk calculator. Using an inverse tangent infinite series, a team led by George Reitwiesner and John von Neumann that same year achieved 2,037 digits with a calculation that took 70 hours of computer time on the ENIAC computer. The record, always relying on an arctan series, was broken repeatedly until 1 million digits were reached in 1973.
Two additional developments around 1980 once again accelerated the ability to compute . First, the discovery of new iterative algorithms for computing , which were much faster than the infinite series and second, the invention of fast multiplication algorithms that could multiply large numbers very rapidly. Such algorithms are particularly important in modern computations because most of the computer’s time is devoted to multiplication. They include the Karatsuba algorithm, ToomCook multiplication, and Fourier transform-based methods.
Role And Characterizations In Mathematics
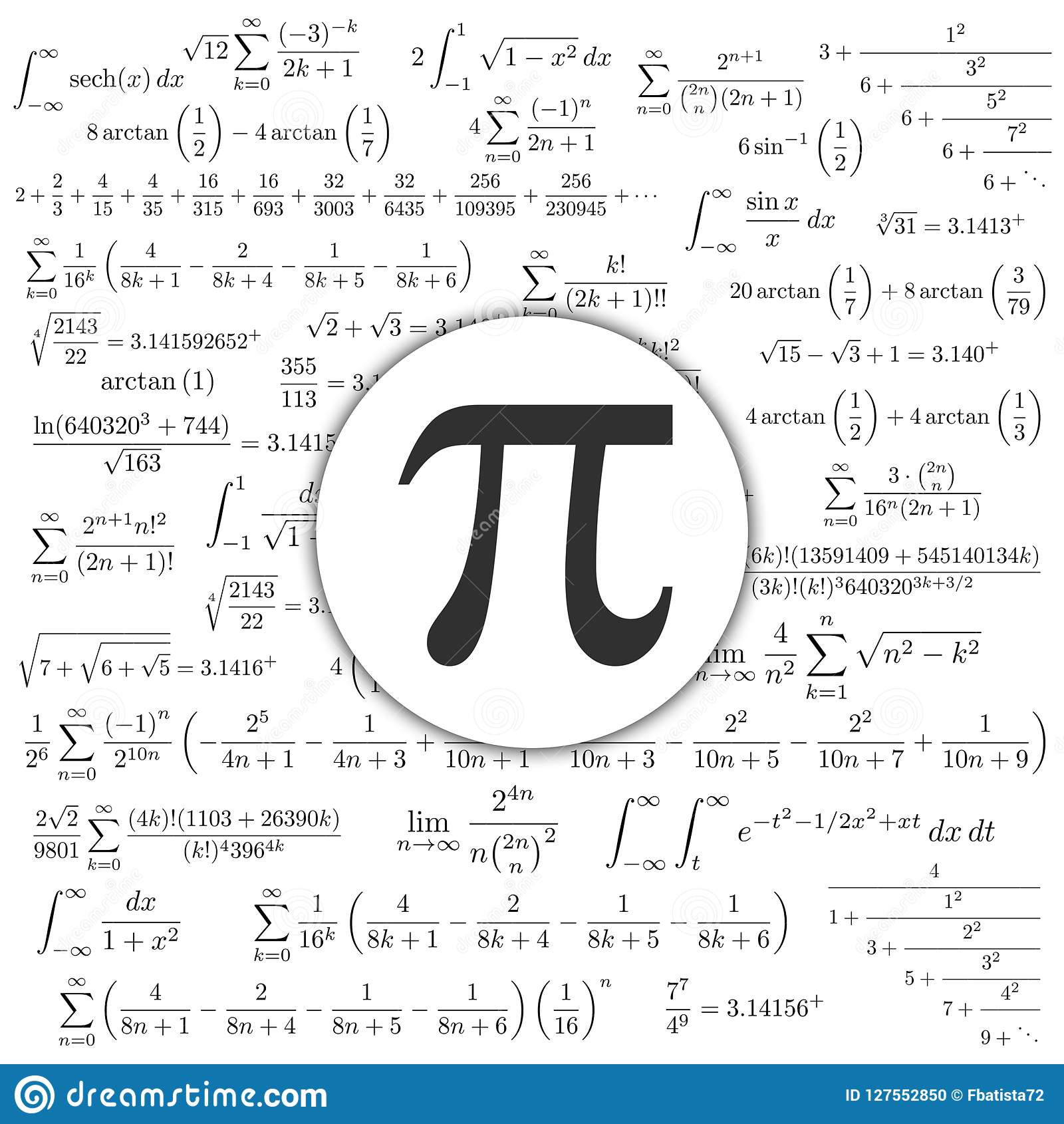
Because is closely related to the circle, it is found in many formulae from the fields of geometry and trigonometry, particularly those concerning circles, spheres, or ellipses. Other branches of science, such as statistics, physics, Fourier analysis, and number theory, also include in some of their important formulae.
You May Like: What Is Delta Y In Physics
Pi Is Not As Constant As You Think
3/12/2015
Yulia Maximenko
A lot of people are celebrating Pi Day on March 14, 2015 at 9:26 am. But let us not forget that is not actually constant in non-Euclidean geometry. And since we live on a two-dimensional spherical surface, this might actually make a difference for circles much smaller than we would intuitively might have guessed. But first, let’s do some simple geometry: Imagine a sphere of radius R. We define a circle on the surface of that sphere as we would define a circle anywhere: a geometrical shape consisting of points equally distant from a selected point. On a 2D spherical surface those circles look like this:
Fig. 1. 3D sphere with circles on its surface.
Note that radius r is measured along the curved line on the surface of the sphere from a point also on that surface. Now, if we actually calculate the circumference of one of the circles of radius r, it would be L=2R sin=2R sin, where is the flat angle from the center of the sphere to the circle on its surface . So, ‘, the varying ratio of the circumference to the diameter, would be’=L/D= sin /= sin /.
Fig. 2. Plot of vs. circle radius on Earth.
So, for changing from 0 to , ‘ would be changing from to 2 in the limiting case of the circle on the equator .
Happy Pi Day!
Adoption Of The Symbol
In the earliest usages, the Greek letter was used to denote the semiperimeter of a circle. and was combined in ratios with or to form circle constants. The first recorded use is Oughtred’s ” “, to express the ratio of periphery and diameter in the 1647 and later editions of Clavis Mathematicae.Barrow likewise used ” ” to represent 6.28… .
The earliest known use of the Greek letter alone to represent the ratio of a circle’s circumference to its diameter was by Welsh mathematician William Jones in his 1706 work Synopsis Palmariorum Matheseos or, a New Introduction to the Mathematics. The Greek letter first appears there in the phrase “1/2 Periphery ” in the discussion of a circle with radius one. However, he writes that his equations for are from the “ready pen of the truly ingenious Mr. John Machin“, leading to speculation that Machin may have employed the Greek letter before Jones. Jones’ notation was not immediately adopted by other mathematicians, with the fraction notation still being used as late as 1767.
Read Also: Geometry Chapter 10 Test Form 2b
What Is Pi Used For
In basic mathematics, pi is used to find the area and circumference of a circle. Pi can be used to find an area by multiplying the radius of the circle squared times pi. So, to find the area of a circle with a radius of 3 centimeters, the calculation would be 3^2 = 28.27 cm. Because circles appear commonly in nature and are often used in other mathematical equations, pi is all around us and is used constantly.
Pi has even trickled into the literary world. Pilish is a form of writing English in which the numbers of letters in successive words follow the digits of pi, according to author Mike Keith . Keith used Pilish in his book “Not A Wake.” Here is an example from the book: “Now I fall, a tired suburbian in liquid under the trees,Drifting alongside forests simmering red in the twilight over Europe.”
“Now” has 3 letters, “I” has 1 letter, “fall” has 4 letters, “a” has 1 letter, and so on.
This article was updated on Oct. 19, 2018, by Live Science senior writer Brandon Specktor. It was updated again on March 3, 2022, by Live Science contributor Adam Mann.
Where Does Pi Come From
By definition, pi is the ratio of the circumference of a circle to its diameter. In other words, pi equals the circumference divided by the diameter . Conversely, the circumference of a circle is equal to pi times the diameter . No matter how large or small a circle is, pi will always work out to be the same number. Pi is the 16th letter of the Greek alphabet and is used to represent the widely known mathematical constant.
Read Also: What Is Theoretical Orientation In Psychology
Significance Of $\pi$ In Physics
We all know this magical mathematical constant.
My question being , how and why pi just shows up in every other physics derivation or formula or even statistics for that matter .
- 2Jan 24, 2015 at 11:03
- 1$\begingroup$I think many $\pi$’s appear, because of the norm of the Fourier transform, which is used almost everywhere in physics.$\endgroup$Jan 24, 2015 at 11:41
- 1Jan 24, 2015 at 12:09
- 1$\begingroup$$\pi$ is a dangerous invasive item. All hail $\tau$ 🙂 You can always make $\pi$ go away by embedding in inside other constants, as suggested in Christoph’s answer. A more interesting question might be: why do we only use $\pi$ and $e$ , of all transcendental numbers, in physics?$\endgroup$ Carl WitthoftJan 24, 2015 at 13:42
- $\begingroup$Mystified as to how can a constant that describes a ratio in Euclidean Geometry be used in field equation that I though changed the geometry of space as the energy density increases. Could be wrong here.$\endgroup$ user86411
Because circles are everywhere, sometimes in the guise of periodicity and rotational symmetry.
In the particular case of gravity, the appearance of $\pi$ in Einstein’s field equations is due to an unfortunate choice of $G$ and it would go away if we switched to a rationalized unit system.
You wanted to know about Pi:
Pi also shows up in the Rocky Planet Density Equation
Density of Rocky Planets:
Den = x 10^-9 * R ^3 + x 10^-1 * R + 2900 kg/m^3.
Venus is 2658 , while Mars is 2954 .
28.9 % and 73.1 %