Sum Of N Terms Of Geometric Sequence Formula
Then sum of its first ‘n’ terms of the geometric sequence a, ar, ar2, ar3, …. is,
Sn = a + ar + ar2 + … + arn-1 …
Multiplying both sides by ‘r’,
rSn = ar + ar2 + … + arn …
Subtracting the equation from equation ,
Sn – r Sn = a – arn
Sn = a
Dividing both sides by ,
Sn = a /
Similarly we can derive the other formula / . Thus,
Sn = a / , when |r| < 1Sn = a / , when r > 1 when r < -1
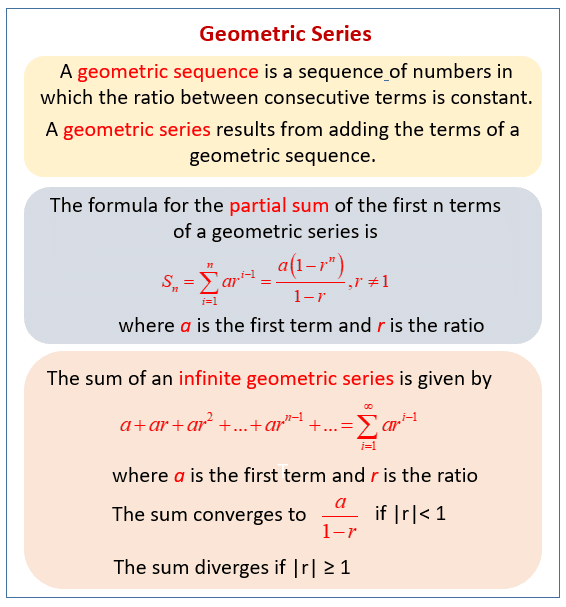
Sum Of Finite Geometric Sequence Formula
The sum of a finite geometric sequence formula is used to find the sum of the first n terms of a geometric sequence. Consider a geometric sequence with n terms whose first term is ‘a’ and common ratio is ‘r’. i.e., a, ar, ar2, ar3, … , arn-1. Then its sum is denoted by Sn and is given by the formula:
- Sn = a / when r 1 and Sn = na when r = 1.
Let us derive the formula now.
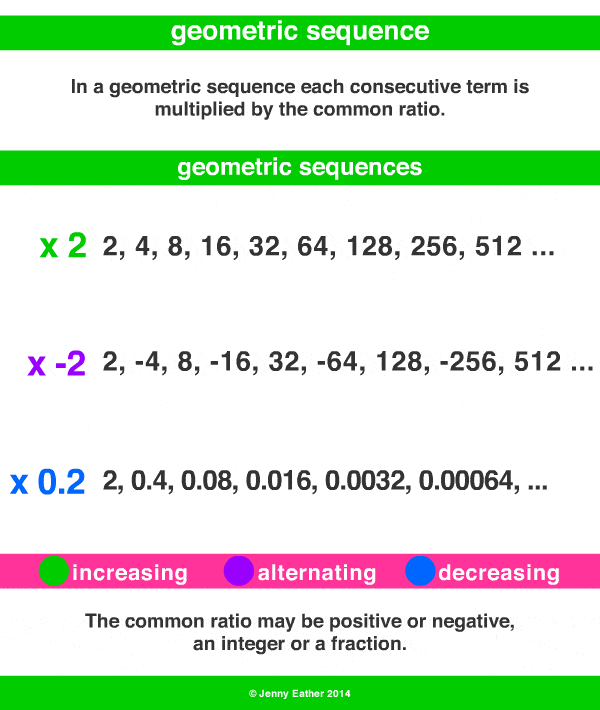
Geometric Progression: What Is A Geometric Progression
Now let’s see what is a geometric sequence in layperson terms. A geometric sequence is a collection of specific numbers that are related by the common ratio we have mentioned before. This common ratio is one of the defining features of a given sequence, together with the initial term of a sequence. We will see later how these two numbers are at the basis of the geometric sequence definition and depending on how they are used, one can obtain the explicit formula for a geometric sequence or the equivalent recursive formula for the geometric sequence.
Now, let’s construct a simple geometric sequence using concrete values for these two defining parameters. To make things simple, we will take the initial term to be 1 and the ratio will be set to 2. In this case, the first term will be a = 1 by definition, the second term would be a = a * 2 = 2, the third term would then be a = a * 2 = 4 etc. The n-th term of the progression would then be:
where n is the position of said term in the sequence.
As you can see, the ratio of any two consecutive terms of the sequence – defined just like in our ratio calculator – is constant and equal to the common ratio.
A common way to write a geometric progression is to explicitly write down the first terms. This allows you to calculate any other number in the sequence for our example, we would write the series as:
Also Check: Who Won The First Nobel Prize For India In Physics
How To Use The Geometric Sequence Calculator
Now that you know what a geometric sequence is and how to write one in both the recursive and explicit formula, it is time to apply your knowledge and calculate some stuff! With our geometric sequence calculator, you can calculate the most important values of a finite geometric sequence. These values include the common ratio, the initial term, the last term and the number of terms. Here’s a brief description of them:
- Initial term First term of the sequence.
- Common ration Ratio between the term a and the term a.
- Number of terms How many numbers does your geometric sequence contain?.
- n-th term Value of the last term.
- Sum of the first N terms Result of adding up all the terms in the finite series.
- Infinite sum Sum of all terms possible, from n=1 to n=.
These terms in the geometric sequence calculator are all known to us already, except the last 2, about which we will talk in the following sections. If you ignore the summation components of the geometric sequence calculator, you only need to introduce any 3 of the 4 values to obtain the 4th element. The sums are automatically calculated from these values but seriously, don’t worry about it too much, we will explain what they mean and how to use them in the next sections.
Sum Of Finite Geometric Sequence Proof
We have Sn = a + ar + ar2 + ar3 + … + arn-1…
Multiply both sides by r,
rSn = ar + ar2 + ar3 + … + arn…
Subtracting from ,
Sn = a
Sn = a /
Since is in its denominator, it is defined only when r 1. If r = 1, the sequence looks like a, a, a, … and the sum of the first n terms, in this case, = a + a + a + … = na.
Thus, we have derived the formula of the sum of a finite geometric sequence.
Recommended Reading: When Do You Use Prefixes In Chemistry
Advantages Of Using A Geometric Sequence
Summing A Geometric Series
We can use this handy formula:
a is the first term r is the “common ratio” between terms n is the number of terms
What is that funny symbol? It is called Sigma Notation
| means “sum up” |
And below and above it are shown the starting and ending values:
It says “Sum up n where n goes from 1 to 4. Answer=10
The formula is easy to use … just “plug in” the values of a, r and n
You May Like: What Is Turner Syndrome In Biology
Example: Sum The First 4 Terms Of
| 10, 30, 90, 270, 810, 2430, … |
This sequence has a factor of 3 between each number.
The values of a, r and n are:
You can check it yourself:
10 + 30 + 90 + 270 = 400
And, yes, it is easier to just add them in this example, as there are only 4 terms. But imagine adding 50 terms … then the formula is much easier.
Percentage Increase Or Decrease
As demonstrated in Example \ and Example \ , if the change to the initial quantity is given as a constant percentage , the sequence will be geometric. The sequence is also sometimes called a geometric progression.
The geometric progression in Example \ is a decreasing sequence. The \value is calculated by considering the offset from \. If the percentage, \, is a decrease, subtract the percentage from \: \\) then change the value to a decimal by moving the decimal two places left ). If the sequence decreases, \.The geometric progression in Example \ is an increasing sequence. The \value is calculated by considering the offset from \. If the percentage, \, is an increase, add the percentage to \: \\) then change the value to a decimal by moving the decimal two places left ). If the sequence increases, \.
Example 8.3.5
Alice puts \ grain of rice on the first square of an \ chess board. The White Rabbit tells her to put double the amount of rice in each successive square thereafter. The Cheshire Cat chuckles and tells Alice, you should join the Mad Hatters if you think you can accomplish this task. How many grains of rice would be on the \ square?
Solution
If one grain of rice occupies the first square, \ the second square, \ the third square, then the common ratio is \, doubling the grains of rice \ times. Remember, we dont start doubling until the \ square, so although there are \ squares, we double the quantity of rice \ times.
\ grains of rice on the \ square!
Read Also: How Have Hela Cells Contributed To Biology And Medicine
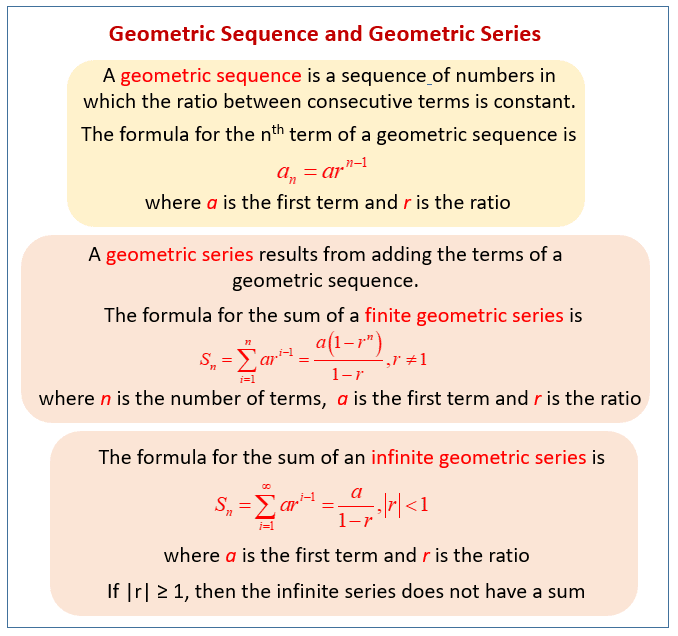
The General Term For A Geometric Sequence
From the flu example above we know that \ and \, and we have seen from the table that the \\ term is given by \.
The general geometric sequence can be expressed as:
Therefore the general formula for a geometric sequence is:
- \ is the first term in the sequence
- \ is the constant ratio.
Test for a geometric sequence
To test whether a sequence is a geometric sequence or not, check if the ratio between any two consecutive terms is constant:
If this condition does not hold, then the sequence is not a geometric sequence.
Recursive Formula Of Geometric Sequence
We know that in a geometric sequence, a term is obtained by multiplying its previous term by the common ratio . So by the recursive formula of a geometric sequence, the nth term of a geometric sequence is,
Example: Find a15 of a geometric sequence if a13 = -8 and r = 1/3.
Solution:
a14 = r a13 = = -8/3
Applying the same formula again,
a15 = r a14 = = -8/9.
Therefore, a15 = -8/9.
You May Like: What Psychology Says About Love In Hindi
What Is The Formula Of Sequence
The general term nth term defines a sequence. If a sequence belongs to specific types like arithmetic, geometric, etc, then we have formulas to find the general term of the respective sequence. Otherwise, one has to observe the numbers of the sequence to identify the pattern. For example, the sequence 1, 27, 125, …, does not represent any type of sequence but one can notice that it represents the cubes of odd natural numbers.
Recursive Vs Explicit Formula For Geometric Sequence
There exist two distinct ways in which you can mathematically represent a geometric sequence with just one formula: the explicit formula for a geometric sequence and the recursive formula for a geometric sequence. The first of these is the one we have already seen in our geometric series example. What we saw was the specific explicit formula for that example, but you can write a formula that is valid for any geometric progression you can substitute the values of a for the corresponding initial term and r for the ratio. The general formula for the n-th term is:
where n means that n = 1, 2, 3, …. The recursive formula for geometric sequences conveys the most important information about a geometric progression: the initial term a, how to obtain any term from the first one, and the fact that there is no term before the initial.
There is another way to show the same information using another type of formula: the recursive formula for a geometric sequence. It is made of two parts that convey different information from the geometric sequence definition. The first part explains how to get from any member of the sequence to any other member using the ratio. This meaning alone is not enough to construct a geometric sequence from scratch, since we do not know the starting point. This is the second part of the formula, the initial term . Let’s see how this recursive formula looks:
Recommended Reading: Pre Algebra 6th Edition Answers
How To Construct An Arithmetic Sequence
Follow the steps mentioned below to construct an arithmetic sequence.
- Take any number as the first term.
- Fix a number as a common difference.
- Add the common difference to the first term to obtain the second term.
- To obtain each successive term, keep adding the common difference to its preceding term.
Geometric Sequence Examples With Solutions
Identify the common ratio in the following geometric sequence: 11, 33, 99, 297…
Solution:
To find thecommon ratio divide the second term of the sequence by the first term of the sequence and so on:
Therefore, the term to term rule of this sequence is 3.
Find the next 3 terms of the geometric sequence 9, 18, 36, 72, 144â¦
Solution:
You May Like: Which One Does Not Belong Geography
Types Of Sequences In Math
There are a few special sequences like arithmetic sequence, geometric sequence, Fibonacci sequence, harmonic sequence, triangular number sequence, square number sequence, and cube number sequence. Apart from these, there can be sequences that follow some other pattern. For example, 2, 9, 28, 65, … is a sequence in which the numbers can be written as 13 + 1, 23 + 1, 33 + 1, 43 + 1,…. and this sequence does not belong to any of the following sequences.
We will discuss these sequences in detail.
How To Find The Common Ratio In A Geometric Sequence
When you are given a geometric sequence, you may not be given the common ratio. It can be helpful to be able to figure this out in case you need to find the next terms of the geometric sequence. To find the common ratio you shall divide one term by the term before it.
Find the common ratio for the geometric sequence 6, 18, 54, 162, 486…
Solution:
To find the common ratio of this geometric sequence, take the second term and divide it by the first term of the sequence. To check your result, divide the third term by the second the fourth by the third and so on.
Therefore the common ratio for this geometric sequence is 3.
Don’t Miss: Midpoint And Distance Formula Geometry
Why Does The Formula Work
Let’s see why the formula works, because we get to use an interesting “trick” which is worth knowing.
First“S”NextSr
Notice that S and S·r are similar?
Now subtract them!
Wow! All the terms in the middle neatly cancel out.
S S·r = a arn
Let’s rearrange it to find S:
Saa
Which is our formula :
So what happens when n goes to infinity?
We can use this formula:
But be careful:
r must be between 1 and 1
and r should not be 0 because the sequence is not geometric
So our infnite geometric series has a finite sum when the ratio is less than 1
Let’s bring back our previous example, and see what happens:
Geometric Sequence Vs Arithmetic Sequence
Here are a few differences between geometric sequence and arithmetic sequence shown in the table below:
| Geometric Sequence | |
|---|---|
| It is determined by the first term and the common ratio. | It is determined by the first term and the common difference. |
| In this, the ratio between every two successive terms is equal to the same number. | In this, the difference of every two successive terms is equal to the same number. |
| The terms form an exponential relation. | The terms form a linear relation. |
| The sum of an infinite geometric sequence may converge or diverge. | The sum of an infinite arithmetic sequence always diverges. |
Important Notes on Geometric Sequence:
- In a geometric sequence, every term is obtained by multiplying its previous term by a constant .
- If r > 1, then the terms are in ascending order.
- When r = 1, and the first term of a geometric sequence is ‘a’ then its sum is a · n.
- When r 1, the infinite geometric sequence diverges.
Related Topics:
Don’t Miss: What Is Homeostasis In Psychology
Disadvantages Of Using A Geometric Sequence
Nth Term Of Geometric Sequence
It is possible to use the term to term rule to find the nth terms of a geometric sequence. To do this multiply or divide the term you have by the common ratio to find the next term of the sequence.
Find the next three terms of the geometric sequence 8, 40, 200, 1000…
Solution:
Therefore the first three terms are 1000, 250, 62.5
Don’t Miss: How To Know If Someone Misses You Psychology
Example Of A Geometric Sequence
A simple example of a geometric sequence is the series 2, 6, 18, 54 where the common ratio is 3. Here, each number is multiplied by 3 to derive the next number in the sequence. Three times two yields 6, which is the second number. Six times three gives 18, which is consequently the following number.
Difference Between An Arithmetic Sequence And A Geometric Sequence
Arithmetic is a mathematical operation that deals with numerical systems and related operations. Its used to get a single, definite value. The word Arithmetic comes from the Greek word arithmos, which meaning numbers. It is a field of mathematics that focuses on the study of numbers and the properties of common operations such as addition, subtraction, multiplication, and division.
A sequence is a collection of items in a specific order . Arithmetic and geometric sequences are the two most popular types of mathematical sequences. Each consecutive pair of terms in an arithmetic sequence has a constant difference. A geometric sequence, on the other hand, has a fixed ratio between each pair of consecutive terms.
You May Like: What Are Dyes In Chemistry
What Is A Geometric Sequence
A geometric sequence is a special type of sequence where the ratio of every two successive terms is a constant. This ratio is known as a common ratio of the geometric sequence. In other words, in a geometric sequence, every term is multiplied by a constant which results in its next term. So a geometric sequence is in form a, ar, ar2… where ‘a’ is the first term and ‘r’ is the common ratio of the sequence. The common ratio can be either a positive or a negative number.