How To Calculate Uncertainty
Quantifying the level of uncertainty in your measurements is a crucial part of science. No measurement can be perfect, and understanding the limitations on the precision in your measurements helps to ensure that you dont draw unwarranted conclusions on the basis of them. The basics of determining uncertainty are quite simple, but combining two uncertain numbers gets more complicated. The good news is that there are many simple rules you can follow to adjust your uncertainties regardless of what calculations you do with the original numbers.
TL DR
If youre adding or subtracting quantities with uncertainties, you add the absolute uncertainties. If youre multiplying or dividing, you add the relative uncertainties. If youre multiplying by a constant factor, you multiply absolute uncertainties by the same factor, or do nothing to relative uncertainties. If youre taking the power of a number with an uncertainty, you multiply the relative uncertainty by the number in the power.
How Do You Calculate The Percent Error Formula For Chemistry Physics Etc
According to the How To Calculate Percent Error article in Thought Co. there are just a few simple steps involved in calculating this formula.
Here they are:
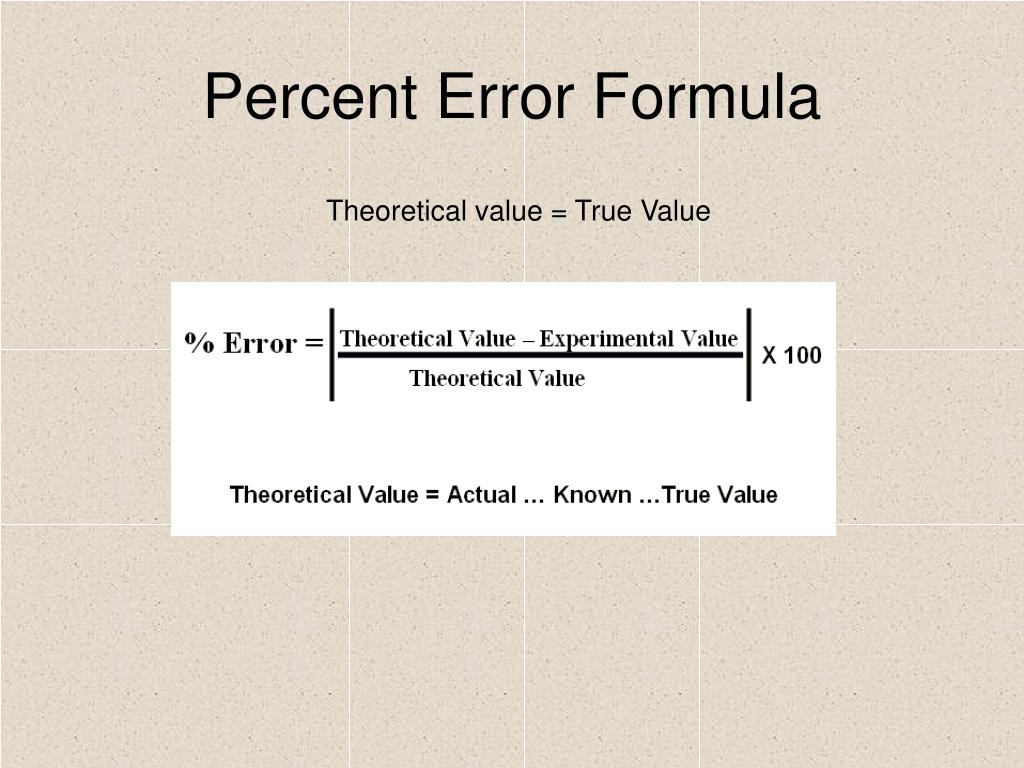
If you are more of a visual version, the Math Is Fun website shows that this is what the formula actually looks like:
Percent Error Of Mean
Percent error mean or Mean percentage error is the average of all percent errors of the given model. The formula for mean percentage error is given by:
Here,
Ti = True or actual value of ith quantity
Ei = Estimated value of ith quantity
n = number of quantities in the model
The main disadvantage of this measure is that it is undefined, whenever a single actual value is zero.
Read more:
The below examples help in better understanding of percent error.
Example 1:
A boy measured the area of a rectangle plot to be 468 cm2. But the actual area of the plot has been recorded as 470 cm2. Calculate the percent error of his measurement.
Solution:
Measured area value = 468 cm2
Actual area value = 470 cm2
Steps of calculation:
Step 1: Subtract one value from another 468 470 = -2
Step 2: Divide the error by actual value 2/470 = 0.0042531
Multiply this value by 100 0.0042531 × 100 = 0.42% Hence, 0.42% is the percent error.
Example 2:
A person started a new business on 1st January. Based on the demand in that particular area, he expected a certain number of customers who can visit his shop per month. The following table gives the information on the number of visitors for the shop during the first quarter.
|
Month |
Mean percent error = / 3
= 36.11%/3
=12.0367%
Note:
Put your understanding of this concept to test by answering a few MCQs. Click Start Quiz to begin!
Select the correct answer and click on the Finish buttonCheck your score and answers at the end of the quiz
You May Like: Demon Guardian Geometry Dash
Calculate The Percent Error Formula For Chemistry Physics Etc
One of the most useful formulas in chemistry, physics, and other sciences is the percentage error formula. If you would like to know how to calculate the percent error formula for chemistry, physics, etc., you have come to the right place.
Here at Error Codes Pro we normally focus on both common and rare errors that affect computers, smart devices or house appliances. But this is not all we do. We are here to help you solve any errors that you might come across.
In this article, I will be talking about the percent error formula (also sometimes known a the percentage error formula. There will all the background and general information about this formula you will ever need including, of course, how you would go about calculating this formula accurately with some practical examples. So, by the end of it, I hope that you will be able to both understand the percent error formula and use it.
What Is Percentage Error
Percentage error expresses as a percentage the difference between an approximate or measured value and an exact or known value. It is used in science to indicate the difference between a measured or experimental value and a true or accurate value. Heres how to calculate percentage error using a sample calculation.
Recommended Reading: Geometry Seeing Doing Understanding Answer Key Pdf
The Attempt At A Solution
smarties said:1. A person travels 800m +/-4m in a time of 3 minutes 12 seconds +/-2sec I have to work out the largest and smallest possible values for the speed along with the percentage error for the speed and distance travelled. 2. Homework Equations = Distance/Time = Speed3. The Attempt at a Solution This is were I get myself in a muddle I am not sure if I should add +/-4m to 800m to make it 804m and 796m or should it be 798m and 802m = +/-4m If someone could explain the correct steps in solving this problem that would be great.
+ / – means just that. 804 and 796 are the upper and lower bound on possible distances measured. What is the spread then in “speeds” using 190 and 194 seconds as the time traveled?
Largest average speed 804/190 = 4.23m/sSmallest average speed 796/194 = 4.10m/sNow what formula should I use to calculate the percentage errors?
- 152
- 0
- 7
- 0
- 152
- 0
Comparing Approximate To Exact
Subtract one value from the other. Ignore any minus sign.
Example: I estimated 260 people, but 325 came. 260 325 = 65, ignore the “” sign, so my error is 65
Then find the Percentage Error:Show the error as a percent of the exact value, so divide by the exact value and make it a percentage:
Example continued: 65/325 = 0.2 = 20%
Percentage Error is all about comparing a guess or estimate to an exact value. See percentage change, difference and error for other options.
Also Check: What Is The Molecular Geometry Of Ccl4
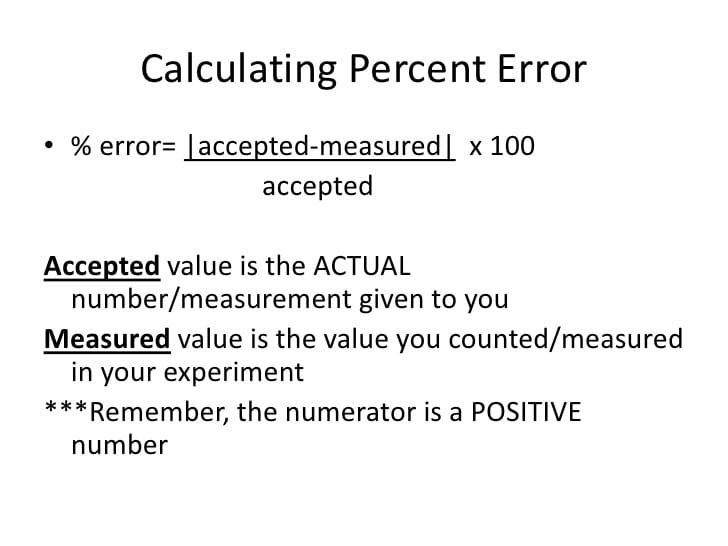
S On How To Calculate Error:
Note the values, and subtract one value from the other. Here, it does not matter in which order the values are being calculated.
Divide the error value with the theoretical value.
The value so obtained is in the form of decimals. Convert it into a percentage by multiplying it by 100, to obtain the percentage.
Tolerance/ Margin Of Error
Error in measurement may be represented by a tolerance . Machines used in manufacturing often set tolerance intervals or ranges in which product measurements will be tolerated or accepted before they are considered flawed.
Tolerance refers to the greatest range of variation that can be allowed or is acceptable and it is one half of the precision of an instrument. So to obtain the range, we add and subtract one half of the precision of measuring instruments.
For example, if a ruler has to measure an object of which has a length of 5.4 cm and it measures this with a precision of 0.1 cm. This gives a tolerance interval in this measurement is 5.4 ± 0.05 cm, or from 5.35 cm to 5.45 cm. Any measurements within this range are tolerated or perceived as correct.
Don’t Miss: Algebra 1 Warm Ups
How To Calculate Percentage Error: The Formula
The percent error is the difference between a measured or experimental value and an accepted or known value, divided by the known value, multiplied by 100%.
In many applications, the percentage error is always expressed as a positive value. The absolute value of the error is divided by an accepted value and given as a percentage. Also read how to find percentage increase with our step-by-step guide.
accepted value experimental value \ accepted value x 100%
It is common for chemistry and other sciences to keep a negative value in case one occurs. Whether the mistake is positive or negative is important. For example, you wouldnt expect a positive percentage error when comparing actual versus theoretical yield from a chemical reaction. If a positive value were calculated, this would indicate possible problems with the procedure or reactions that were not taken into account.
If the sign for an error is retained, the calculation is the experimental or measured value minus the known or theoretical value, divided by the theoretical value and multiplied by 100%.
percentage error = / theoretical value x 100%
How To Calculate Percentage Error In Excel
Calculate The Percentage Change / Difference Between Two Numbers Using The FormulaAs shown in the screenshot below, you need to calculate the percentage change between the new number 94 and the old number 80. The formula = / old_value can help you quickly calculate the percentage change between two numbers. Please follow these steps.
Now the percentage difference between two given numbers is calculated.
Don’t Miss: Ccl4 Geometric Shape
Can Percentage Error Be Negative
We generally take percentage error to be positive because it is the amount of error involved that is important not its sign. However some sciences like chemistry requires it to specify the sign of the error.In this case you do not take the absolute of the difference between two values. Here order of subtraction also matters where you subtract measured value from the true value.So, if your percentage error is negative , then measured value is higher than the true/accepted value.However until and unless it is desired you should always take the absolute value and report percentage error as a positive number.For example Suppose you estimated the length of the board and made a measure of 22 cm. A peer measures the length of board as well and obtains a measurement of 18 cm. You need to know the “true” or “accepted value” of the length of the board to determine the percentage error of each of the measurements. After checking the manufacturers documentation, you found the length of the board is 20 cm in length. This would be used as the accepted value of our estimate.The percentage error estimates for the two measures as seen below. Both measurements indicate an error of 1%. Your peer has made a mistake that is on the low side of the accepted value, so it is negative . You, on the other hand, have made an error on the high side of the accepted value and it is positive.$\text= \frac-\text|}|}\times 100$$\text= \frac\times 100 = 10%$$\text=+10%$
What Is Percent Error
Percent errors tells you how big your errors are when you measure something in an experiment. Smaller values mean that you are close to the accepted or real value. For example, a 1% error means that you got very close to the accepted value, while 45% means that you were quite a long way off from the true value. Measurement errors are mostly unavoidable: equipment can be imprecise, hands can shake, or your instruments just might not have the capability to measure accurately. Percent error will let you know how badly these unavoidable errors affected your results.
You May Like: What Is The Molecular Geometry Of Ccl4
How Does An Electrical Circuit Work
A complicated piece of electronics equipment may contain several resistors whose role is to control the voltage and current in the electrical circuit. Too much current and the apparatus malfunctions. Too little current and the system simply does not perform. The resistors values are always given with an error range. A resistor may have a stated value of 200 ohms, but a 10% error range, meaning the resistance could be anywhere between 195-205 ohms. By knowing these values, an electronics person can design and service the equipment to make sure it functions properly.
An individual measurement may be accurate or inaccurate, depending on how close it is to the true value. Suppose that you are doing an experiment to determine the density of a sample of aluminum metal. The accepted value of a measurement is the true or correct value based on general agreement with a reliable reference. For aluminum the accepted density is 2.70 g/cm 3. The experimental value of a measurement is the value that is measured during the experiment. Suppose that in your experiment you determine an experimental value for the aluminum density to be 2.42 g/cm 3. The error of an experiment is the difference between the experimental and accepted values.
Error = experimental value accepted value
\displaystyle\%\text=\frac-\text|}}\times100\%
\displaystyle\%\text=\frac^3-2.70\text^3|}^3}\times100\%=9.26\%
What Is The Percent Error
Sometimes, we try to estimate the value of a particular quantity by making repeated measurements. For example, we can try to find out what is the gravitational acceleration by measuring the time of free fall from a specific height.
The measured acceleration will probably deviate a bit from the actual acceleration of 9.80665 m/s². To check what is the relative – or percent – error, you need to know two values: the real value and observed one.
Recommended Reading: Geometry Segment Addition Postulate Worksheet
Percent Error Example Calculation
In a lab, you are given a block of aluminum. You measure the dimensions of the block and its displacement in a container of a known volume of water. You calculate the density of the block of aluminum to be 2.68 g/cm3. You look up the density of a block of aluminum at room temperature and find it to be 2.70 g/cm3. Calculate the percent error of your measurement.
Relevance And Uses Of Percent Error Formula
- The keys to reporting percent error correctly are to know whether or not to drop the sign on the calculation and to report the value using the correct number of significant figures.
- It is frequently used in the analysis of scientific research and is used by the physician and in the field of chemistry. Students also when use to do experiments in labs use the percent error formula in order to determine the variance. The acceptable range of error depends on the case to case basis and the quantum of percent error needs to be determined beforehand when experimenting a particular test in the lab always. The use of value with a high percent error in measurement is the judgment of the use.
You May Like: Segment Addition Postulate Find The Length Indicated
Examples Of Percent Error Formula
Lets take an example to understand the calculation of Percent Error in a better manner.
Example #1
The density of the aluminum block is 2.68 g/cm cube while the density of the same aluminum block at room temperature is to be 2.70 g/cm cube. Calculate the Percent Error of the measurement.
Solution:
Percent Error is calculated using the formula given below
Percent Error = || / |Theoretical Value| * 100
- Percent Error = ||/ |2.68| * 100
- Percent Error = 0.75%
Example #2
The user needs to find the volume and the mass of the cube of a paper in the experimental lab. When the user is calculating the value of the measurements it gets the value as 8.78 g/cm cube. Whereas, the accepted density of the cube is to be 8.96 g/cm cube. Calculate the percent error.
Solution:
Percent Error is calculated using the formula given below
Percent Error = || / |Theoretical Value| * 100
- Percent Error = || / |8.78| * 100
- Percent Error = 2.05%
Example #3
A scale measures wrongly a value of 10 cm due to some marginal error in the computation. The user needs to calculate the percent error of the measurement when the actual value of the scale is to be 12 cm.
Solution:
Percent Error is calculated using the formula given below
Percent Error = || / |Theoretical Value| * 100
- Percent Error = || / |10| * 100
- Percent Error = 20%
What Does A Percent Error Tell You
Percent error tells us how much extent few unavoidable errors affect our experimental results. It is measured by taking the difference between the actual value and the observed value. Small percent errors indicate that you are close to the accepted or real value.
Percent errors tell how big your errors are when you measure something in an experiment.
Don’t Miss: Theory Of Everything 2 Geometry Dash
Examples Of Calculations Of The Percent Error Formula
Now that I have gone through all the different variations for this formula both in for of steps and as an actual visual formula, it is time to see this calculation in practice.
The first example has been taking for the Thought Co article I referenced earlier one.
What Is the Context of the Experiment: This is a lab experiment that involves a block of aluminum.
The objective of the Experiment: Your goal in this experiment is twofold: on the one hand you are been asked to calculate the dimensions of the block of aluminum and, on the other hand, you will also need to calculate the displacement in a container with a volume of water that is also unknown.
In this example, you measure the density of the block of aluminum as 2.68 g/cm3
Then, as part of the experiment, you reckon that the density of the block of aluminum is 2.70 g/cm3 at room temperature.
With that information, you will now be in a position to calculate the percent error of your measurement.
So, heres how you will do this.
Firstly, subtract one value from the other, like this: 2.68 2.70 = 0.02
Then, you will have to decide whether you have to take the absolute value , or not. This will depend on your needs. In this case, we will remove the negative sign from 0.02 and get, instead 0.02. This value is the error.
Next, you will have to divide the error by the true value, like this: 0.02 / 2.70 = 0.0074074.
What you will need to do next is to multiply this value by 100, like this: 0.0074074 x 100 = 0.74.