What Does Notation Mean In Math

Naming A Function By A Letter

Math Antics – Scientific Notation

A function may be named by a letter of some alphabet, for temporary use in that particular section of text.

Examples:

A paragraph may begin, “Let $ f:\mathbb\to\mathbb $ be the squaring function”, then the name of the function in that paragraph is $f$. Thevalueof the function at $3$ is denoted by “$f$”, which in this example is $9$.
“Let $ \phi :\mathbb\to \mathbb $ be a continuous function with no derivative at 0.” In this case, the letter $\phi$ does not refer to a specific function such as the squaring function, but an unspecified function that is subject to theconstraint that it has no derivative at $0$.
“Let $ \psi =^}-1 $ “. $ \psi $ is the letter psi.This names the function and simultaneously gives a formula for calculating its value.

Symbolic expressions that are not functions may also be given names. The expression “$E$” mentioned under barred arrow notation is an example. Using letters for naming functions and expressions are examples of local identifiers.

History Of Mathematical Notation

The history of mathematical notation includes the commencement, progress, and cultural diffusion of mathematical symbols and the conflict of the methods of notation confronted in a notation’s move to popularity or inconspicuousness. Mathematical notation comprises the symbols used to write mathematical equations and formulas. Notation generally implies a set of well-defined representations of quantities and symbols operators. The history includes HinduArabic numerals, letters from the Roman, Greek, Hebrew, and German alphabets, and a host of symbols invented by mathematicians over the past several centuries.

The area of study known as the history of mathematics is primarily an investigation into the origin of discoveries in mathematics and, the focus here, the investigation into the mathematical methods and notation of the past.

What Does The Notation Mean In This Question

Taken from the Rhode Island Mathletes Documentation from 1986

if $$ is the largest integer not bigger than $x$, what is $ + $

I’m not sure what this question is asking at all, for a start, how can $$ be less than $x$? And what do the brackets mean at all?

1$\begingroup$The definition is given to you: “$$ is the largest integer not bigger than $x$.” You may know this as “the result after rounding down $x$ to the nearest integer.” We do have $=x$ if $x$ is an integer, but in general it might be that $ < x$.$\endgroup$ angryavianOct 26, 2017 at 2:28
$\begingroup$The meaning of the brackets is explained in the line you quoted: $[x[$ means the largest integer not bigger than $x.$$\endgroup$Oct 26, 2017 at 2:28
$\begingroup$This is a common notation. See en.wikipedia.org/wiki/Floor_and_ceiling_functions#Notation$\endgroup$Oct 26, 2017 at 2:29
$\begingroup$@bof So basically means to floor the value of $x$?$\endgroup$ TravisOct 26, 2017 at 2:31
$\begingroup$”How can $$ be less than $x$?” If $x$ is not an integer, then $$ has to be less than $x.$ It can’t be equal to $x$ because it’s an integer and $x$ is not an integer it can’t be bigger than $x$ because it’s “not bigger than $x $ the only possibility left is that it’s less than $x.$ For example, if $x=3.5$ then $=3\lt3.5=x.$$\endgroup$

Don’t Miss: What Is Mode In Algebra

How Do You Write Exponents In Math

Notation. The notation of an exponent in math is a pair of numbers, symbols or both. The number written normally is called the base number, while the number written in superscript is the exponent. The root form of most exponents is a number multiplied with itself by the exponents number of times.

What is an exponent and exponential notation?

Exponential notation is a way of shorthand for very large numbers and very small numbers . But first exponents. They are the numbers that you see at the top right of another number, called the base, as in #10^2#, where the 10 is the base and the 2 is the exponent.

What Does The Sideways U With A Line Under It Mean

AB. The line under the sideways means that A may also be equal to B . If we want to say that A is a proper subset of B then we can remove the line: AB.

Use the noun notation to describe a written note, like the comments an English teacher makes in the margins of a students essay. Another kind of notation is a series of symbols that represent words, numbers, or music, for example. Chemical formulas are one type of notation, and musical notes are another.

Read Also: Stitz Zeager College Algebra Book

Notation For Definition And Equivalence

I would like some clarification about the usage/meaning of $:=$ and $\equiv$.

I have been using $A := B$ to denote “Let $A$ be defined as $B$.” This is akin to assignment = in programming.

I also have been using $A \equiv B$ to denote “$A$ is equivalent to $B$,” but as a result of their definitions which do not directly depend on each other.

However, in books and papers, I rarely see “$:=$” used authors only seem to use “$=$”.

Could someone comment on the usage of these two symbols as well as what the convention is for notating the relations described above? Thanks!

My impression is that there are no situations where it is required to use $:=$ or $\equiv$ instead of $=$ . Rather, they are used to clarify what the author is trying to say.

Your usage of $:=$ I believe is the standard one, and it is used when there is a possibility of confusion between saying that $f = g$ “for the time being”, or based on the context, where we are actually trying to say that $f$ is defined to be $g$ and nothing else. Your usage for $\equiv$ is more often replaced in mathematics by $A \iff B$, where $A$ and $B$ are propositions.

The symbol $\equiv$ is used mostly in math to clarify the following ambiguity: if the author says $f = g$, it is perhaps unclear whether we are talking about a particular value of $x$, or whether the equality is between functions. $f \equiv g$ is used to emphasize that the functions are equal at all relevant points $x$.

I hope that explains something -)

How Do You Write Set Notation

The set notation is generally written using symbols between the sets for set operations, and certain symbols for representing some special kind of sets. The set notation for the union of sets is A U B, for the intersection of sets is A B. And the set notation for representing some important sets is the – universal set, Ø – null set.

Recommended Reading: How To Find Magnitude In Physics

What Does : = Mean

4$\begingroup$It’s one common notation for stating that the left-hand side is defined as the expression on the right-hand side.$\endgroup$Mar 5, 2011 at 22:33
7$\begingroup$For future reference, the table of mathematical symbols at wikipedia is fairly extensive and has a number of further references.$\endgroup$Mar 6, 2011 at 2:11
1$\begingroup$careful. the fist two comments seem to say that := says that the equality holds by definition. that would be wrong, the statement is not an equality at all, but it IS a definition.$\endgroup$

It is borrowed from computer programming: it means that the item on the left hand side is being defined to be what is on the right hand side. For example, $$y := 7x+2$$means that $y$ is defined to be $7x+2$.

This is different from, say, writing$$1 = \sin^2 + \cos^2$$where we are saying that the two sides are equal, but we are not defining “1” to be the expression “$\sin^2 + \cos^2$”.

Basically, some people think that there should be notational difference between saying “I define blah to be equal to blankety” and saying “blah is equal to blankety”. So they use := for the first and = for the latter. Usually, it is clear from context which of the two uses of the equal sign is intended

What Are The Types Of Set Notations For Representing Elements

What Does Scientific Notation Mean for a Scale? : Solutions to Your Math Problems

The two important types of set notations for representing the elements are the roster form and the set builder form. The set builder notation represents the elements of a set in the form of a sentence or mathematical expression, and the set builder form represents the elements by writing the elements distinctly. The set builder form of set notation is A = , and the roster of of the same set is A = }2, 4, 6, 8, 10}.

Don’t Miss: What Is N In Physics

How Do You Read Maths

The best way to understand what you are reading is to make the idea your own. This means following the idea back to its origin, and rediscovering it for yourself. Mathematicians often say that to understand something you must first read it, then write it down in your own words, then teach it to someone else.

What Is Function Notation Good For

There is nothing wrong with the notation y = 3x + 1. However, it has some limitations.

If you are dealing with more than 1 function, you still have to use y. However, with the function notation, you could use g or h to indicate other functions of x.

With the notation that uses y, you cannot see the inputs. However, with the function notation, you can see the inputs. For example, when you are looking for the output f, you can clearly see that the input is 6.

You can do composition of functions which you can study in another lesson.

Recommended Reading: Geometry Geometry Dash Sub Zero

Why Is The Input To A Function Called The Argument

The term “argument” has a long history. Originally, it was a logical term, referring to a statement that forwarded a proof or, in a less formal sense, a claim that was being used to try to convince somebody of something. Eventually, the term came to refer, in early scientific contexts, to any mathematical value that was needed as an input to other computations, or any value upon which later results depended.

In the twentieth century, when computer coding started becoming a thing, coders adopted the mathematical meaning to refer to inputs to their coding. In our mathematical context, the “argument” is the independent variable and the function’s output is the dependent variable .

Given h, what is the function name, and what is the argument?

I’ll do the second part first. The argument is whatever is inside the parentheses, so the argument here is s.

The function name is the variable that comes before the parentheses. In this case, then, the function name is h.

function name: h

argument: s

What is the argument of f ?

The argument is whatever is plugged in. In this particular case, the variable being plugged in is “y“. So:

the argument is y

Given g = t 2 + t, what is the function name? In g, what is the argument?

The function name is what comes before the parentheses, so the function name here is g.

function name: g

argument of g: 1

Content Continues Below

Where Is Exponential Notation Used

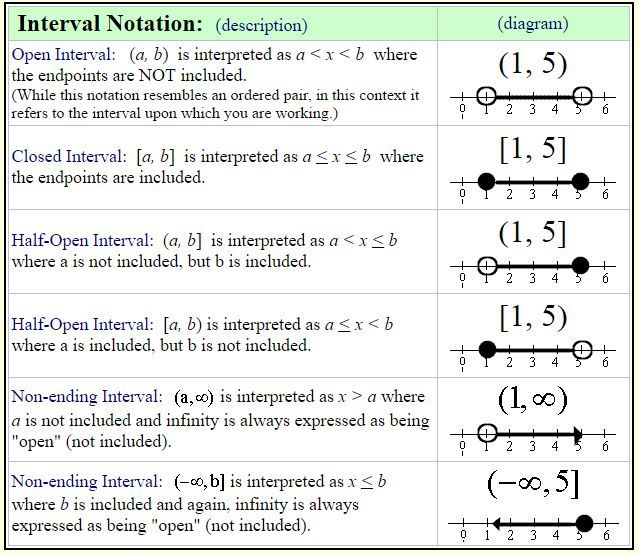
Exponential notation is used to write very large or very small numbers with a lot of zeros expressed in standard form. When converting from standard form to exponential notation, the goal is to end up with one non-zero digit on the left side of the decimal point, as in 0.00004, by multiplying or dividing.

What is the exponential notation of 81?

81 = 3 × 3 × 3 × 3 can be written as 81 = 34, here 3 is the base and 4 is the exponent. 103, which is 10 raised to the power 3, also read as 10 cubed.

What are some examples of exponential notation?

Exponential notation is an alternative method of expressing numbers. Exponential numbers take the form a n, where a is multiplied by itself n times. A simple example is 8=2 3 =2×2×2. In exponential notation, a is termed the base while n is termed the power or exponent or index.

Don’t Miss: What Is Causation In Psychology

Strategy Three: Writing Upside

Sometimes life can get boring, and we are forced to make our own fun. If so, maybe some of you consider writing upside down a break from the monotony of real life. Sure, it has little to do with math, but it can still be enjoyable!

Upside-down writing also has its advantages. You can use it when posting or sending messages on social media or when creating social media passwords.

Doing this is simple as well. Visit the site upsidedowntext.com, and write whatever you want to write in the Type text, words, letters, or symbols here: box.

The sentence will then show up reversed and flipped, where you can copy and paste it. You can get rid of the text effects by unclicking the boxes with the effects.

Image by Sona Digital Media

The text looks like this if you copy past it:

unb lsun o loqs ol s A s unq uop psdn u ldll ool loqs A l

Note: Bear in mind that the mathematical symbol is slightly different from the letter A turned by itself. If you look at the differences below, you can see that the math symbol is a little bit shorter.

Image by Sona Digital Media

If you are trying to write an , its a good idea to first try and make use of the previous methods rather than relying on this one, both for accuracy and your own convenience.

Indian & Arabic Numerals And Notation

Although the origin of our present system of numerical notation is ancient, there is no doubt that it was in use among the Hindus over two thousand years ago. The algebraic notation of the Indian mathematician, Brahmagupta, was syncopated. Addition was indicated by placing the numbers side by side, subtraction by placing a dot over the subtrahend , and division by placing the divisor below the dividend, similar to our notation but without the bar. Multiplication, evolution, and unknown quantities were represented by abbreviations of appropriate terms. The HinduArabic numeral system and the rules for the use of its operations, in use throughout the world today, likely evolved over the course of the first millennium AD in India and was transmitted to the west via Islamic mathematics.

Algebra

The modern Arabic numeral symbols used around the world first appeared in Islamic North Africa in the 10th century. A distinctive Western Arabic variant of the Eastern Arabic numerals began to emerge around the 10th century in the Maghreb and Al-Andalus , which are the direct ancestor of the modern Arabic numerals used throughout the world.

What Is The Math Symbol That Looks Like A Check Mark

is the symbol for square root. A square root is the number that, when multiplied by itself, gives the original number. For example, the square root of 4 is 2, because 2 x 2 = 4. The square root of 9 is 3, because 3 x 3 = 9.What is the mathematical equation for the electric force between two charges? electrostatic force formula example.

What Is Big O Function

Sigma notation for sums | Sequences, series and induction | Precalculus | Khan Academy

Big O notation is a mathematical notation that describes the limiting behavior of a function when the argument tends towards a particular value or infinity. In computer science, big O notation is used to classify algorithms according to how their run time or space requirements grow as the input size grows.

You May Like: What Is Psychological Safety At Work

What Does Sigma Notation Mean

4/5Sigma notationsigma notationsigma notationmore about it

Often mathematical formulae require the addition of many variables Summation or sigma notation is a convenient and simple form of shorthand used to give a concise expression for a sum of the values of a variable. Let x1, x2, x3, xn denote a set of n numbers. x1 is the first number in the set.

Also, what does Sigma mean in calculus? Calculus. Tables of Derivatives Summation notation. Summation notation allows an expression that contains a sum to be expressed in a simple, compact manner. The uppercase Greek letter sigma, , is used to denote the sum of a set of numbers.

Beside this, what does this symbol mean ?

The symbol means sum. is the Greek capital sigma symbol. Used commonly in algebraic functions, you may also notice it in Excel – the AutoSum button has a sigma as its icon.

What is the symbol for Sigma?

Sigma is the eighteenth letter of the Greek alphabet.

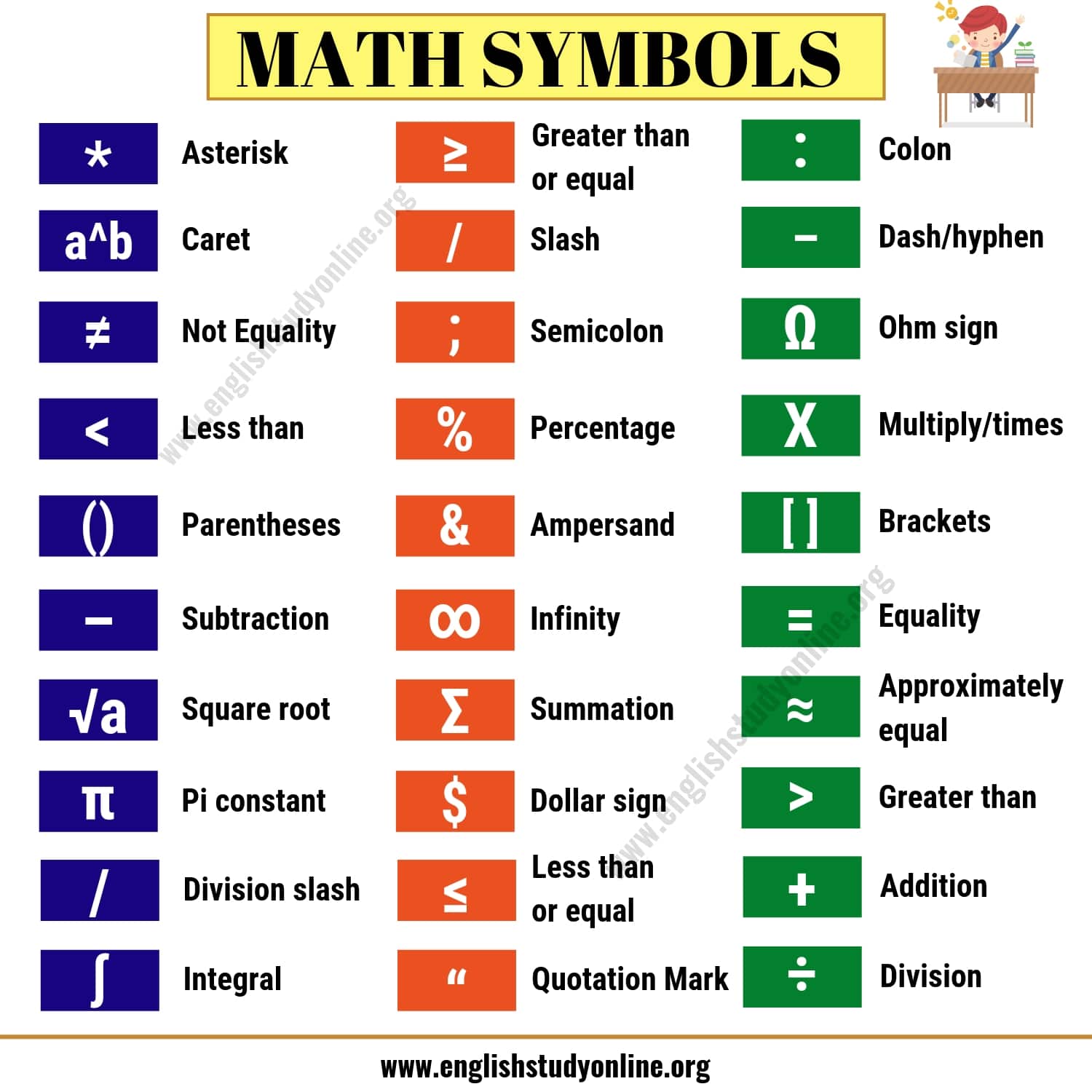
List Of Mathematical Symbols By Subject

The following list of mathematical symbols by subject features a selection of the most common symbols used in modern mathematical notation within formulas, grouped by mathematical topic. As it is impossible to know if a complete list existing today of all symbols used in history is a representation of all ever used in history, as this would necessitate knowing if extant records are of all usages, only those symbols which occur often in mathematics or mathematics education are included. Many of the characters are standardized, for example in DIN 1302 General mathematical symbols or DIN EN ISO 80000-2 Quantities and units Part 2: Mathematical signs for science and technology.

The following list is largely limited to non-alphanumeric characters. It is divided by areas of mathematics and grouped within sub-regions. Some symbols have a different meaning depending on the context and appear accordingly several times in the list. Further information on the symbols and their meaning can also be found in the respective linked articles.