How To Find Magnitude In Physics

Finding The Magnitude Of A Vector At The Origin

The Resultant Magnitude Of Two Or More Vectors

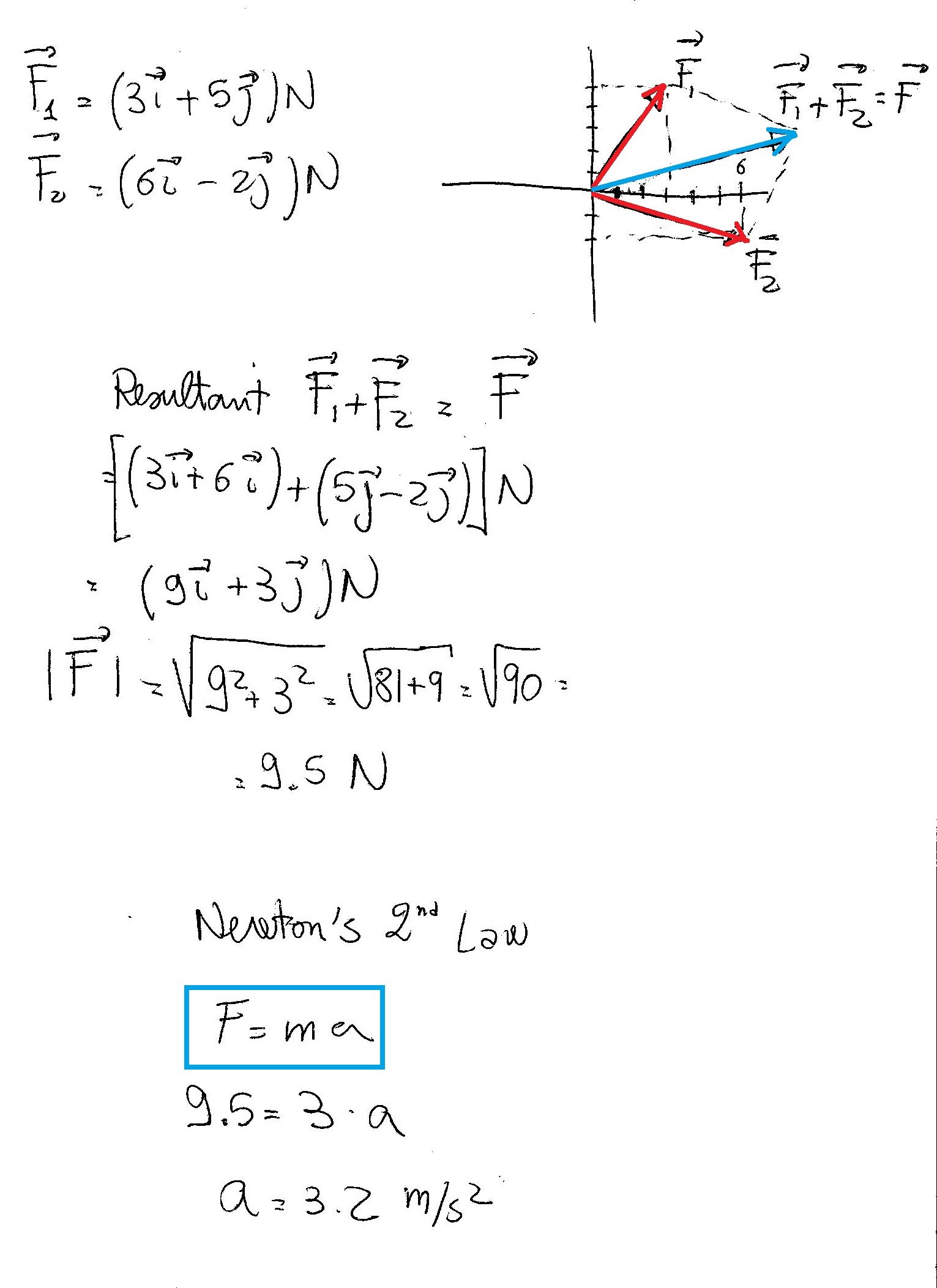
If you have several forces acting on an object, you need first to calculate the resultant vector. That is pretty straightforward. The secret is ensuring that you add the corresponding X and Y components. Here is a demonstration.

Think of a boat in the sea using the wind to sail. The water is impacting 4 N in an X-direction and 1 N in the Y-direction. Besides, wind adds another force of 5 N in the X-direction and 3 N in the Y-direction. Therefore, the resultant vector for the X-coordinate will be 4+5=9 N and for the Y-coordinate will be 3+1=4 N. This simply means that the 9 N will be acting in the X direction while 4 N will be exerted in the y-direction. Now, to calculate the magnitude of the resultant force, here is the equation to apply.

F=

Limitations Of The Coulomb Model

The Coulomb approximation follows from the assumptions that: surfaces are in atomically close contact only over a small fraction of their overall area that this contact area is proportional to the normal force and that the frictional force is proportional to the applied normal force, independently of the contact area. The Coulomb approximation is fundamentally an empirical construct. It is a rule-of-thumb describing the approximate outcome of an extremely complicated physical interaction. The strength of the approximation is its simplicity and versatility. Though the relationship between normal force and frictional force is not exactly linear , the Coulomb approximation is an adequate representation of friction for the analysis of many physical systems.

When the surfaces are conjoined, Coulomb friction becomes a very poor approximation . In this case, the frictional force may depend strongly on the area of contact. Some drag racing tires are adhesive for this reason. However, despite the complexity of the fundamental physics behind friction, the relationships are accurate enough to be useful in many applications.

Negative coefficient of friction

Dont Miss: What Is The Molecular Geometry Of Ccl4

You May Like: Simplifying Radicals With Imaginary Numbers Worksheet

How To Calculate Magnitude Of A Scalar

Here are some real life examples to help you understand. Scalar magnitude calculation is very simple which we do every day. We calculate scalar magnitude 90 percent of the time in real life. For example

1. Suppose you take a bus journey from New York to Boston. When you arrived in Boston, a friend of yours asked you, How much distance did you travel in total?

And when you tell your friend I have traveled 346 km. In this case, 346 km is the magnitude of the distance.

2. Suppose you mix 250 grams of water in one liter of pure milk. Then now your total amount of milk will be 1250 grams. In this case the calculation of magnitude has followed the rule of simple addition.

3. You bought a dozen bananas from a fruit shop for one dollar per banana. And in this case your total bill will be 12 dollars.

So, calculate the scalars magnitude in this simple way. That is, what we do all the time.

Determining Tension On A Single Strand

You May Like: How To Convert Units In Chemistry

Multiplying By A Scalar

While adding and subtracting vectors gives us a new vector with a different magnitude and direction, the process of multiplying a vector by a scalar, a constant, changes only the magnitude of the vector or the length of the line. Scalar multiplication has no effect on the direction unless the scalar is negative, in which case the direction of the resulting vector is opposite the direction of the original vector.

SCALAR MULTIPLICATION

Scalar multiplication involves the product of a vector and a scalar. Each component of the vector is multiplied by the scalar. Thus, to multiply \ by \, we have

Example \

Find a linear equation to solve for the following unknown quantities: One number exceeds another number by \ and their sum is \. Find the two numbers.

Solution

First, we must multiply each vector by the scalar.

\ & = 9,6 \\ 2v & = 21,4 \\ & = 2,8 \end\]

Then, add the two together.

\ & =9,6+2,8 \\ & = 92,6+8 \\ & = 7,2 \end\]

So, \.

Newtons Laws Of Motion

Newton came up with three laws of motion that explain the movement of all physical objects. They are the basics of all classical mechanics, which is also known as Newtonian mechanics.

An object will remain at rest or continue to move in uniform motion unless acted upon by an external force.

Force exerted by an object equals mass times acceleration of that object: F = m * a.

When one body exerts a force on a second body, the second body exerts a force equal in magnitude and opposite in direction on the first body .

You May Like: Ccl4 Molecular Geometry

Performing Operations On Vectors In Terms Of \ And \

When vectors are written in terms of \ and \, we can carry out addition, subtraction, and scalar multiplication by performing operations on corresponding components.

ADDING AND SUBTRACTING VECTORS IN RECTANGULAR COORDINATES

Given \ and \, then

\ vu & = i+j \end\]

Example \: Finding the Sum of the Vectors

Find the sum of \ and \.

Solution

\ & = 6i+2j \end\]

How To Calculate The Force Of Friction

Surfaces exert a frictional force that resists sliding motions, and you need to calculate the size of this force as part of many physics problems. The amount of friction mainly depends on the normal force, which surfaces exert on the objects sitting on them, as well as the characteristics of the specific surface youre considering. For most purposes, you can use the formula:

to calculate friction, with N standing for the normal force and incorporating the characteristics of the surface.

Don’t Miss: Cpm Algebra 2 Chapter 1 Answers

How To Calculate Magnitude Of A Vector

Vector quantities too have magnitude with direction. Some of the vector quantities are displacement, velocity, acceleration, force, etc. We call the magnitude of a vector as its absolute value.

We can determine that two vectors will only be equal if both have the same magnitude as well as direction. If we multiply a vector with a positive number, its magnitude will change but the direction remains the same. Similarly, if we multiply a vector with a negative number, its magnitude and direction both will change.

Magnitude Of Gravitational Force

To determine the magnitude of gravitational force, by definition we say that there is a force acting between the two objects with masses m1 and m2, which is mathematically represented as:

Where F is the magnitude of the gravitational force between the two objects.

Related articles:

Stay tuned with BYJUS to learn more about the concepts of Physics.

Don’t Miss: The Five Branches Of Chemistry

How To Find Magnitude Of Force Based On Graphs

I don’t get it. How do I get information from the graphs? How can it be solved?

• $\begingroup$Try finding eq of vx and vy in terms of t and differentiate them to get acceleration ax and ay.$\endgroup$Mar 1 ’17 at 5:43
• $\begingroup$What do you mean? How can I find those?$\endgroup$Mar 1 ’17 at 5:47
• $\begingroup$Do you know calculus.?$\endgroup$Mar 1 ’17 at 6:36
• $\begingroup$$a_x$ is the slope of first graph, so what’s that amount.?$\endgroup$

$a_x$ is the slope of first graph, so $a_x=3$.

$a_y$ is the slope of second graph, so $a_y=-3$.

Then $\vec=3\vec-3\vec$ and $\vec=m\vec=3.3 N$ from here $|\vec|=9.9\sqrt\,N$.

It’s direction $\tan\theta=\dfrac=-1$ then $\theta=-45^\circ$.

How To Find Force

Lets look at a few exercises so that nothing can surprise you in your physics class.

1. Find the accelerating and retarding force:

A cheetah has a mass of 50 kg. It accelerates from rest to 50 km/h in 3 seconds. Then it starts steadily slowing down and stops after 8 seconds.

• Accelerating force:

• a = / 8 s = -1.74 m/s2

  Fr = 50 kg * -1.74 m/s2 = -87 N

  Retarding force is negative, because it has an opposite direction to the accelerating force.

2. How much force do you need to accelerate an object by 8 m/s2? What about when the object is three times heavier? How does it affect the force?

• F1 = 2 kg * 8 m/s2 = 16 N

• F2 = 3 * 2 kg * 8 m/s2 = 48 N

If the mass is three times heavier, the force needs to be three times bigger.

You May Like: Fsa Answers 2021

Also Check: Hawkes Learning Systems Intermediate Algebra Answers

What Is Net Force

Newtons second law of motion relates the force applied to a body, F, and its resulting acceleration, a. It is expressed mathematically through the following equation, where the bodys mass, m, is the proportionality constant:

Lets do a thought experiment to try and understand what this equation means. Imagine you are at the grocery shop and want to move a cart around to put your groceries in. If the cart was initially at rest, meaning it was not moving, and you push it, it will start to roll at a certain speed, lets say 1 m/s. In this case, the cart went from zero velocity to 1 m/s in a very short period of time.

Since there was a change in the carts velocity, we can say it was accelerated. If that change in velocity occurred, for example, in a timeframe of one second, we can say the acceleration was exactly 1 m/s2. Go ahead and read the section called How to measure acceleration to understand how we got this result. Now, according to equation 1, if there was an acceleration, there had to be also a force causing it, which is the one you applied when pushing the cart. The mass in the equation is, in this case, that of the cart, which is constant. This thought experiment is summarized by this image:

For all our calculations, the center of mass will have the same effect as the entire cart, and it will be represented by a gray circle. We can now draw all forces acting on this point, a.k.a. our grocery cart:

Magnitude Of Electric Field Created By A Charge

Let us now first look at finding magnitude of electric field created by a charge.

To find electric field due to a single charge we make use of Coulombs Law.

If a point charge $q$ is at a distance $r$ from the charge $q$ then it will experience a force

$\vec=\frac\frac}$

Electric field at this point is given by relation

$\vec=\frac}=\frac\frac}$

This is electric field at a distance $r$ from a point charge $q$ and $\hat$ is the unit vector along the direction of electric field.

Above relation defining electric field at a distance $r$ tels about both magnitude and direction of the field.

Magnitude of electric field would be

$|\vec|=\frac\frac$

In above equation you could notice the missing $\hat$ part. This $\hat$ tells us about the direction of the electric field. Since electric force is a central force and we have defined electric field using Coulombs law we can conclude that electric field acts along the line joining the charge $q$ and field point at which it is being measured.

This vector This $\hat$ is known as unit vector of our displacement vector $\vec$ and is given by relation

$\hat=\frac$

Where $r=|\vec|$ which is the distance between our source point and the field point.

Don’t Miss: Edgenuity Health Unit 1 Test Answers

Is Force A Vector

Force is a vector. This means that it is determined by direction and size. Scalar quantities, such as length or mass, are determined only by size. Therefore, if you apply two forces to an object, you cannot add them as scalars. You must consider the direction and find the resultant force from the vector sum of the forces.

Why Is Net Force Important

Net force is a central idea of dynamics. Heres why:

Newtons Laws are the big deal in dynamics. Newtons First Law canbe stated:

If the on an object is zero, its acceleration will be zero.

You will soon find out that NewtonsSecond Law says:

where Fnet means net force.

In order to use Newtons Laws, the first thing you need to know isWhat is the net force.

You May Like: Edgenuity Algebra 1 Answer Key

Calculating The Component Form Of A Vector: Direction

We have seen how to draw vectors according to their initial and terminal points and how to find the position vector. We have also examined notation for vectors drawn specifically in the Cartesian coordinate plane using \ and \. For any of these vectors, we can calculate the magnitude. Now, we want to combine the key points, and look further at the ideas of magnitude and direction.

Calculating direction follows the same straightforward process we used for polar coordinates. We find the direction of the vector by finding the angle to the horizontal. We do this by using the basic trigonometric identities, but with \ replacing \.

VECTOR COMPONENTS IN TERMS OF MAGNITUDE AND DIRECTION

Given a position vector \ and a direction angle \,

\ x & = |v| \cos \theta \\ y & = |v| \sin \theta \end\]

Thus, \, and magnitude is expressed as \.

Example \: Writing a Vector in Terms of Magnitude and Direction

Write a vector with length \ at an angle of \ to the positive x-axis in terms of magnitude and direction.

Solution

Using the conversion formulas \ and \, we find that

\ & = \dfrac} \\ y & =7 \sinj \\ & = \dfrac} \end\]

This vector can be written as \i+7\sinj\) or simplified as

Exercise \

A vector travels from the origin to the point \\). Write the vector in terms of magnitude and direction.

Answer

\=59.04°\)

Newtons Second Law In Terms Of Momentum

When Newtonâs second law is expressed in terms of momentum, it can be used for solving problems where mass varies, since Î

Î p stays the same will decrease Fnet. This is another example of an inverse relationship. Similarly, a padded dashboard increases the time over which the force of impact acts, thereby reducing the force of impact.

Cars today have many plastic components. One advantage of plastics is their lighter weight, which results in better gas mileage. Another advantage is that a car will crumple in a collision, especially in the event of a head-on collision. A longer collision time means the force on the occupants of the car will be less. Deaths during car races decreased dramatically when the rigid frames of racing cars were replaced with parts that could crumple or collapse in the event of an accident.

Read Also: Psychopathic Child Beth