What Does Mean In Geometry

Look Up The Meaning Of Math Words

• Email

• Ph.D., Biomedical Sciences, University of Tennessee at Knoxville
• B.A., Physics and Mathematics, Hastings College

This is a glossary of common mathematical terms used in arithmetic, geometry, algebra, and statistics.

Abacus: An early counting tool used for basic arithmetic.

Absolute Value: Always a positive number, absolute value refers to the distance of a number from 0.

Acute Angle: An angle whose measure is between 0° and 90° or with less than 90° radians.

Addend: A number involved in an addition problem numbers being added are called addends.

Algebra: The branch of mathematics that substitutes letters for numbers to solve for unknown values.

Algorithm: A procedure or set of steps used to solve a mathematical computation.

Angle: Two rays sharing the same endpoint .

Angle Bisector: The line dividing an angle into two equal angles.

Area: The two-dimensional space taken up by an object or shape, given in square units.

Array: A set of numbers or objects that follow a specific pattern.

Attribute: A characteristic or feature of an objectsuch as size, shape, color, etc.that allows it to be grouped.

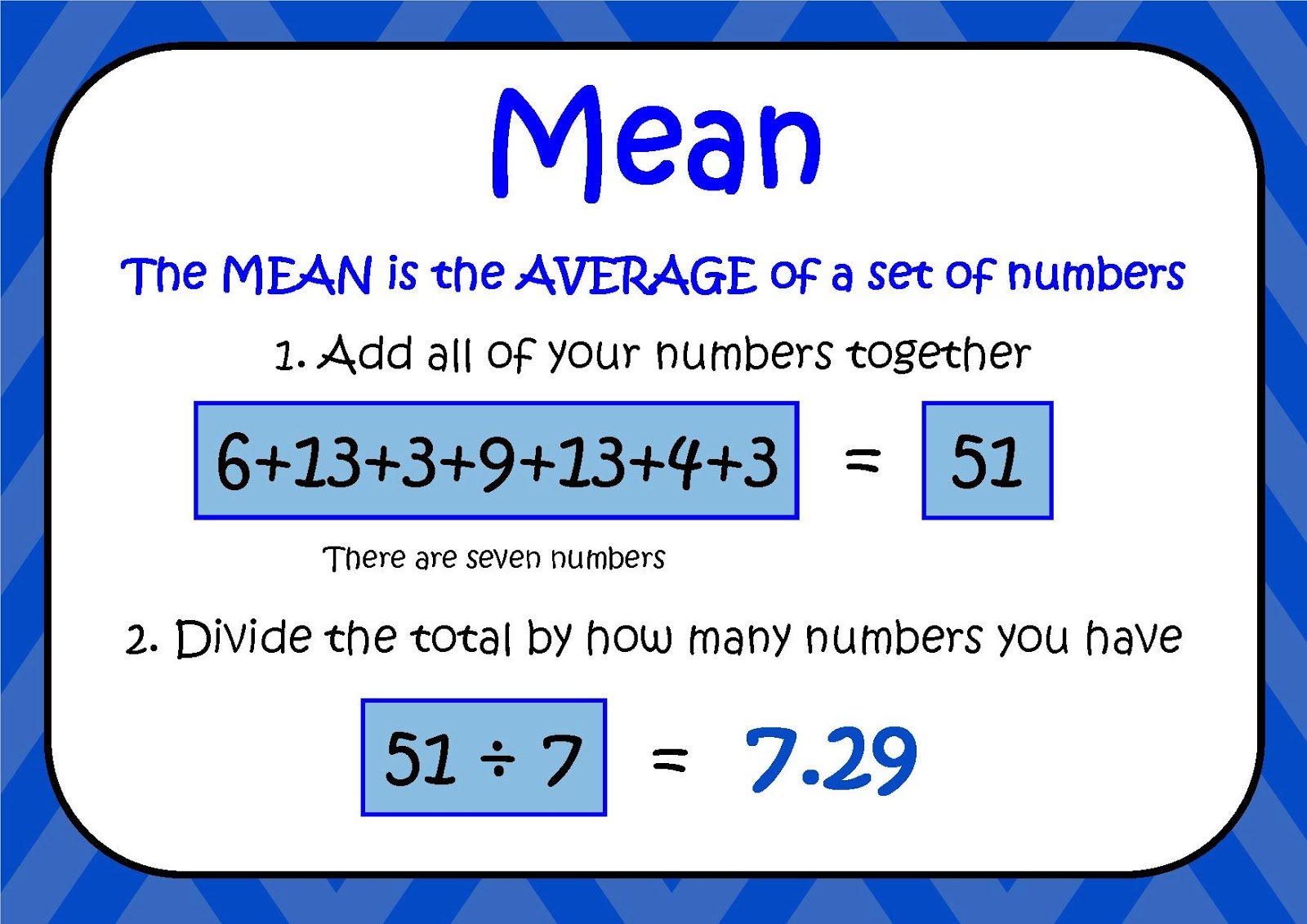
Average: The average is the same as the mean. Add up a series of numbers and divide the sum by the total number of values to find the average.

Base: The bottom of a shape or three-dimensional object, what an object rests on.

Base 10: Number system that assigns place value to numbers.

Capacity: The volume of substance that a container will hold.

Geometry In Early Schooling

When you take geometry in school, you are developing spatial reasoning and problem-solving skills. Geometry is linked to many other topics in math, specifically measurement.

In early schooling, the geometric focus tends to be on shapes and solids. From there, you move to learning the properties and relationships of shapes and solids. You will begin to use problem-solving skills, deductive reasoning, understand transformations, symmetry, and spatial reasoning.

Sigma As A Summation Operator

n=03n=0+1+2+3=6

This is commonly known as the sigma notation. In practice, the sigma operator is used in finance and accounting, managing balance sheets and in similar other instances where there is a need to add a series of numbers.

You will also see the sigma sign as a parameter in various well-known mathematical formulas. For example, it is used in the formula for calculating the Rayleigh distribution and Maxwell distribution. When used in conjunction with certain symbols, sigma can denote other functions such as the divisor function that consists of small sigma with a k in the subscript.

In physics, you will often come across terms like 2-sigma, 5-sigma, and so on. This is a further extension of the use of sigma for representing standard deviation. Sigma with a bigger number as a prefix signifies a relatively lesser deviation from a set value and vice versa. It is a technique used by scientists to measure the fluctuations in a set of data, and identify whether it was caused by a certain factor or was just a random error.

In biology, the lowercase sigma symbol was initially used to demonstrate the total life span of a multicellular unit in the bone marrow. However, its use in this respect is now obsolete.

Also Check: Mcdougal Littell Algebra 2 Chapter 2 Test Answers

How Do I Write A Trig Function That’s Raised To A Power

For trigonometric functions, powers are indicated directly on the function names. For instance, “the square of the sine of beta” is written as sin2, and this notation means 2.

Multipliers on the variable go inside the argument: sin does not mean the same thing as sin2.

Some texts omit the function-notation parentheses, writing sin2 and sin2, which can lead to confusion, especially when these expressions are hand-written. Try to remember to use the parentheses, so you can be clear in your own work.

Also, try not to get in the lazy habit of omitting the arguments of the functions, writing things like sin2 + cos2 = 1, as this will lead to severe problems when the argument is not something simple like just “x“.

The final “convention” I’ll mention is actually an assumption that you should remember not to make:

not

URL: https://www.purplemath.com/modules/conventn.htm

How To Find The Geometric Mean

Need help with a homework question? Check out our tutoring page!

Example 1: What is the geometric mean of 2, 3, and 6?First, multiply the numbers together and then take the cubed root = 1/3 = 3.30

Note: The power of is the same as the cubed root 3. To convert a nth root to this notation, just change the denominator in the fraction to whatever n you have. So:

• 5th root = to the power
• 12th root = to the power
• 99th root = to the power.

Example 2: What is the geometric mean of 4,8.3,9 and 17?First, multiply the numbers together and then take the 5th root = = 6.81

Example 3: What is the geometric mean of 1/2, 1/4, 1/5, 9/72 and 7/4?First, multiply the numbers together and then take the 5th root: = 0.35.

Example 4: The average persons monthly salary in a certain town jumped from $2,500 to $5,000 over the course of ten years. Using the geometric mean, what is the average yearly increase?

Solution:

Step 1: Find the geometric mean.^ = 3535.53390593.

Step 2: Divide by 10 .3535.53390593 / 10 = 353.53.

The average increase is 353.53.

Tip: The geometric mean of a set of data is always less than the arithmetic mean with one exception: if all members of the data set are the same (i.e. 2, 2, 2, 2, 2, 2, then the two means are equal.

Don’t Miss: When To Use Distribution In Math

What Does An Exclamation Mark Mean In Math

Our experts in all academic subjects are available 24/7. Don’t hesitate to ask questions and start discussions whenever you need professional advice.

A

The exclamation mark in math is used in permutation to indicate factorials. When the exclamation mark is used in front of a positive integer, it means that one should find the product of the integers from the positive integer to the number 1. For example, 2! equals 2 times 1.

Origin Of The Sigma Symbol

Some semioticians suggest that the sigma symbol is derived from shin.

Shin is the twenty-first character in the Phoenician alphabet, sometimes also referred to as the Proto-Canaanite alphabet. In case you are unaware, Phoenician is the oldest known system of alphabets that dates as far back as 1050 BC. It is believed that the ancient civilizations in what constitutes modern day Lebanon, Syria and northern Israel heavily relied on this system of alphabets for communication.

The Phoenician shin looks exactly like the capital letter W in the modern English alphabet. Rotate it 90 degrees clockwise and you get the capital version of the sigma symbol.

Don’t Miss: Algebra 2 Cp Geometric Series Worksheet Answers

Or * Or Multiplication

These symbols have the same meaning commonly × is used to mean multiplication when handwritten or used on a calculator 2 × 2, for example.

The symbol * is used in spreadsheets and other computer applications to indicate a multiplication, although * does have other more complex meanings in mathematics.

Less commonly, multiplication may also be symbolised by a dot . or indeed by no symbol at all. For example, if you see a number written outside brackets with no operator , then it should be multiplied by the contents of the brackets: 2 is the same as 2×.

See our page on Multiplication for more.

Dealing With Negative Numbers

Generally, you can only find the geometric mean of positive numbers. If you have negative numbers , its possible to find a geometric mean, but you have to do a little math beforehand .

Example: What is the geometric mean for an investment that shows a growth in year 1 of 10 percent and a decrease the next year of 15 percent?

Step 1: Figure out the total amount of growth for the investment for each year. At the end of the first year you have 110% of what you started with. The following year, you have 90% of what you started year 2 with.

Step 2: Calculate the geometric mean based on the figures in Step 1:GM = = 0.99.

The geometric mean for this scenario is 0.99. Your investment is slowly losing money at a rate of about 1% per year.

Don’t Miss: Introduction To Sequences Common Core Algebra 1 Homework Answers

United Nations Human Development Index

The Human Development Index is an index that takes into account factors other than economic development when reporting a countrys growth. It is a summary measure of average achievement in key dimensions of human development: a long and healthy life, being knowledgeable and have a decent standard of living. The HDI is the geometric mean of normalized indices for each of the three .UNDPThe normalized indexes refer to the fact that the geometric mean isnt affected by differences in scoring indices. For example, if standard of living is scored on a scale of 1 to 5 and longevity on a scale of 1 to 100, a country that scores better in longevity would score better overall if the arithmetic mean were used. The geometric mean isnt affected by those factors.

Webster Dictionaryrate This Definition:

Recommended Reading: How To Find The Product In Math

What Does E Mean In Math

The letter E can have two different meaning in math, depending on whether it’s a capital E or a lowercase e. You usually see the capital E on a calculator, where it means to raise the number that comes after it to a power of 10. For example, 1E6 would stand for 1 × 106, or 1 million. Normally, the use of E is reserved for numbers that would be too long to be displayed on the calculator screen if they were written out longhand.

Mathematicians use the lowercase e for a much more interesting purpose to denote Euler’s number. This number, like , is an irrational number, because it has a non-recurring decimal that stretches to infinity. Like an irrational person, an irrational number seems to make no sense, but the number that e denotes doesn’t have to make sense to be useful. In fact, it’s one of the most useful numbers in mathematics.

Difference Between Arithmetic Mean And Geometric Mean

Here is a table representing the difference between arithmetic and geometric mean.

Now, substitute and in , we get

HM = GM2 /AM

Or else,

GM =

Hence, the relation between AM, GM, and HM is GM2 = AM × HM. Therefore the square of the geometric mean is equal to the product of the arithmetic mean and the harmonic mean.

Let us also see why the G.M for the given data set is always less than the arithmetic mean for the data set. Let A and G be A.M. and G.M.

A = /2 and G=ab

Now lets subtract the two equations

AG = /2 ab = /2 = 2/2 0

AG 0

This indicates that A G

Also Check: What Are Physical Features In Geography

Length Area And Volume

Length, area, and volume describe the size or extent of an object in one dimension, two dimension, and three dimensions respectively.

In Euclidean geometry and analytic geometry, the length of a line segment can often be calculated by the Pythagorean theorem.

Area and volume can be defined as fundamental quantities separate from length, or they can be described and calculated in terms of lengths in a plane or 3-dimensional space. Mathematicians have found many explicit formulas for area and formulas for volume of various geometric objects. In calculus, area and volume can be defined in terms of integrals, such as the Riemann integral or the Lebesgue integral.

Metrics and measures

The concept of length or distance can be generalized, leading to the idea of metrics. For instance, the Euclidean metric measures the distance between points in the Euclidean plane, while the hyperbolic metric measures the distance in the hyperbolic plane. Other important examples of metrics include the Lorentz metric of special relativity and the semi-Riemannian metrics of general relativity.

In a different direction, the concepts of length, area and volume are extended by measure theory, which studies methods of assigning a size or measure to sets, where the measures follow rules similar to those of classical area and volume.

What Were The Typical Crafts For Maya

Mayans are well-known as great pottery makers. They did not use the pottery wheel. However,the tecnique they used was coiling consecutive rings of clay on top of each other and smoothing the surface by hand. Also, they were good when it came to working with stone stone craving is another important craft of Maya. Many

Also Check: How Many Biological Children Does Steve Harvey Have

Euler’s Number In Nature

Exponents with e as a base are known as natural exponents, and here’s the reason. If you plot a graph of

you’ll get a curve that increases exponentially, just as you would if you plotted the curve with base 10 or any other number. However, the curve y = ex has two special properties. For any value of x, the value of y equals the value of the slope of the graph at that point, and it also equals the area under the curve up to that point. This makes e an especially important number in calculus and in all the areas of science that use calculus.

The logarithmic spiral, which is represented by the equation

is found throughout nature, in seashells, fossils and and flowers. Moreover, e turns up in numerous scientific contexts, including the studies of electric circuits, the laws of heating and cooling, and spring damping. Even though it was discovered 350 years ago, scientists continue to find new examples of Euler’s number in nature.

Related Articles

Logarithmic Values And The Geometric Mean

One way to think of the geometric mean is that its the average of logarithmic values converted back to base 10. If youre familiar with logarithms, this can be a very intuitive way to look at it. For example, lets say you wanted to calculate the geometric mean of 2 and 32.

Step 1: Convert the numbers to base 2 logs :

• 2 = 21
• 32 = 25

Step 2:Find the average of the exponents in Step 1. The average of 1 and 5 is 3. Were still working in base 2 here, so our average gives us 23, which gives us the geometric mean of 2 * 2 * 2 = 8.

Don’t Miss: What Is Vapor Pressure In Chemistry

Line Segments And Rays

There are two kinds of lines: those that have a defined start- and endpoint and those that go on for ever.

Lines that move between two points are called line segments. They start at a specific point, and go to another, the endpoint. They are drawn as a line between two points, as you would probably expect.

The second type of line is called a ray, and these go on forever. They are often drawn as a line starting from a point with an arrow on the other end:

Where Does Euler’s Number E Come From

The number represented by e was discovered by mathematician Leonard Euler as a solution to a problem posed by another mathematician, Jacob Bernoulli, 50 years earlier. Bernoulli’s problem was a financial one.

Suppose you put $1,000 in a bank that pays 100% annual compound interest and leave it there for a year. You’ll have $2,000. Now suppose the interest rate is half that, but the bank pays it twice a year. At the end of a year, you’d have $2,250. Now suppose the bank paid only 8.33%, which is 1/12 of 100%, but paid it 12 times a year. At the end of the year, you’d have $2,613. The general equation for this progression is:

where r is 1 and n is the payment period.

It turns out that, as n approaches infinity, the result gets closer and closer to e, which is 2.7182818284 to 10 decimal places. This is how Euler discovered it. The maximum return you could get on an investment of $1,000 in one year would be $2,718.

Don’t Miss: Who Was The Founder Of Biology

How Is Congruency Denoted

Congruent angles are indicated by arcs in the congruent angles. Congruent lines are indicated with tick-marks.

Congruent angles are indicated with arcs .

If there is more than one pair of congruent angles, additional arcs will be used. So this picture shows that angle A is congruent to angle X and angle B is congruent to angle Y.

Congruent segments are indicated by tick-marks. If there is more than one pair of congruent segments, additional tick-marks will be used. So this picture shows that side AB is congruent to side CD and side DA is congruent to side BC.

Affiliate

Other notation, such as for rays, arcs, etc, is usually defined in the text. Unfortunately, as old as geometry is, the notation does not seem, even today, to be entirely standardized.

Therefore, it will be wise to pay particular attention to how your book does things, so you can follow along, but don’t be surprised if your instructor, especially in a later class with a different textbook, does something else.

Affiliate