What Is Dimensional Analysis In Chemistry

+ Beautiful Dimensional Analysis Worksheet Answers Chemistry

Awesome Dimensional analysis worksheet answers chemistry. Worksheets are significant figures name dimensional analysis factor label method chemistry if8766 answers dividing monomials substances. Dimensional analysis worksheet set up and solve the following using dimensional analysis. Chemistry answer key dimensional analysis practice worksheet.

It is a useful technique. 1000mm 1m 1m 1000 mm 150 mg 1 600 000 mm or 1 6 x 106 mm determine the number of m in 1600 mm. About this quiz worksheet.

3 days seconds 3. Dimensional analysis worksheet answer sheet is below worksheet 1. Some of the worksheets for this concept are dimensional analysis practice.

Convert 40 miles per hour to feet per second. The second dimensional analysis worksheet i assign as homework and then stamp and review at the next class session using the answer key. Use dimensional analysis and the group round robin to answer each question.

Metric Conversions Using Dimensional Analysis Dimensional Analysis Nursing Math Metric Conversions Convert 45 3 cm to its equivalent measurement in mm. Indicate all relationships needed before setting up and solving the problem. Below are some examples of basic dimensional analysis.

Limitations Of Dimensional Analysis

Some limitations of dimensional analysis are:

• It doesnt give information about the dimensional constant.
• The formula containing trigonometric function, exponential functions, logarithmic function, etc. cannot be derived.
• It gives no information about whether a physical quantity is a scalar or vector.

What Are The Steps For A Unit Analysis

The steps for performing a unit analysis are as follows: 1. Determine what conversion facts are needed 2. Write the starting fact with units included as a fraction 3. Write the ending fact with units included as a fraction 4. Between the starting and ending, build a product of fractions using the necessary conversion facts.

Recommended Reading: My Hrw Com Algebra 1

What Is Dimension Formula

Hint Dimension formula is the expression for the unit of a physical quantity in terms of the fundamental quantities. The fundamental quantities are mass , Length and time . A dimensional formula is expressed in terms of power of M, L and T. These will specify the nature of the unit and not its magnitude.

Access Is An Aact Member Benefit Activity: Measurement Tools Significant Figures And Conversions

In this activity, students will complete several hands-on measurements, using a variety of common measuring tools. They will carefully consider how to properly report each measurement based on the tool used. Students will then complete measurement conversions, and apply their knowledge of significant figures.

Introduction, Interdisciplinary, Dimensional Analysis, Experimental Design | High School

Recommended Reading: Cf4 Lewis Structure Molecular Geometry

What Is Dimensional Analysis

What is the definition of dimensional analysis? Dimensional analysis is an essential skill used widely in the field of chemistry. Using this technique can answer questions like: How much of this chemical do I need in my reaction? and What is the concentration of my solution? At its simplest form, dimensional analysis is the methodical canceling-out of units. Take the example below:

In more real-world applications, dimensional analysis is used to convert between different units of measurement, and find unknown characteristics from those that we do know.

Concrete Numbers And Base Units

Many parameters and measurements in the physical sciences and engineering are expressed as a concrete numberâa numerical quantity and a corresponding dimensional unit. Often a quantity is expressed in terms of several other quantities for example, speed is a combination of length and time, e.g. 60 kilometres per hour or 1.4 kilometres per second. Compound relations with “per” are expressed with division, e.g. 60 km/1 h. Other relations can involve multiplication , powers , or combinations thereof.

A set of base units for a system of measurement is a conventionally chosen set of units, none of which can be expressed as a combination of the others and in terms of which all the remaining units of the system can be expressed. For example, units for length and time are normally chosen as base units. Units for volume, however, can be factored into the base units of length , thus they are considered derived or compound units.

Sometimes the names of units obscure the fact that they are derived units. For example, a newton is a unit of force, which has units of mass times units of acceleration . The newton is defined as 1 N = 1 kgâmâsâ2.

Recommended Reading: Ap Human Geography Self Study

Siano’s Extension: Orientational Analysis

Angles are, by convention, considered to be dimensionless quantities. As an example, consider again the projectile problem in which a point mass is launched from the origin = at a speed v and angle θ above the x-axis, with the force of gravity directed along the negative y-axis. It is desired to find the range R, at which point the mass returns to the x-axis. Conventional analysis will yield the dimensionless variable Ï = Rg/v2, but offers no insight into the relationship between R and θ.

Siano has suggested that the directed dimensions of Huntley be replaced by using orientational symbols1x 1y 1z to denote vector directions, and an orientationless symbol 10. Thus, Huntley’s Lx becomes L1x with L specifying the dimension of length, and 1x specifying the orientation. Siano further shows that the orientational symbols have an algebra of their own. Along with the requirement that 1iâ1 = 1i, the following multiplication table for the orientation symbols results:

1 1 0 & \mathbf } & \mathbf }} & \mathbf }} & \mathbf }} \\\hline \mathbf } & 1_& 1_}& 1_}& 1_}\\\mathbf }} & 1_}& 1_& 1_}& 1_}\\\mathbf }} & 1_}& 1_}& 1_& 1_}\\\mathbf }} & 1_}& 1_}& 1_}& 1_\end}}

z ) }+\,1_})=1_}\cos} . Siano distinguishes between geometric angles, which have an orientation in 3-dimensional space, and phase angles associated with time-based oscillations, which have no spatial orientation, i.e. the orientation of a phase angle is 1 } .

R

What Is The Dimensional Analysis Definition Chemistry

Dimensional Analysis is a way chemists and other scientists convert units of measurement . We can convert any unit to another unit of the same dimension . This means we can convert some number of seconds into another unit of time , such as minutes , because we know that there are always 60 seconds in one minute . or we can convert some amount of mass from grams to kilograms knowing that there are always 1000 grams in one kilogram . or we can convert lengths, say, from kilometers to miles , although metric units are most frequently used in chemistry . with a known conversion factor , it is sometimes , possible to convert to a new dimension , for instance , 1 kilogram of pure water is equal to 1 liter . using that knowledge , you could convert a volume of water to a mass of water , or vice versa .

Explanation:

The answer is explained below.

Explanation:

In a few and easy words, the term dimensional analysis can be explained as a way chemists and other scientists convert units of measurement. For example, it is possible to convert grams into another unit of weight, such as pounds or kilograms because we know how many of them are contained in each unit

Read Also: Segment Addition Postulate And Midpoint Worksheet Answer Key

Checking Equations That Involve Dimensions

The factor-label method can also be used on any mathematical equation to check whether or not the dimensional units on the left hand side of the equation are the same as the dimensional units on the right hand side of the equation. Having the same units on both sides of an equation does not ensure that the equation is correct, but having different units on the two sides of an equation implies that the equation is wrong.

For example, check the Universal Gas Law equation of PV = nRT, when:

• the pressure P is in pascals
• the volume V is in cubic meters
• the amount of substance n is in moles

K 1 }=}}}}\times }}}}}\ }}}}}\times }}}}}

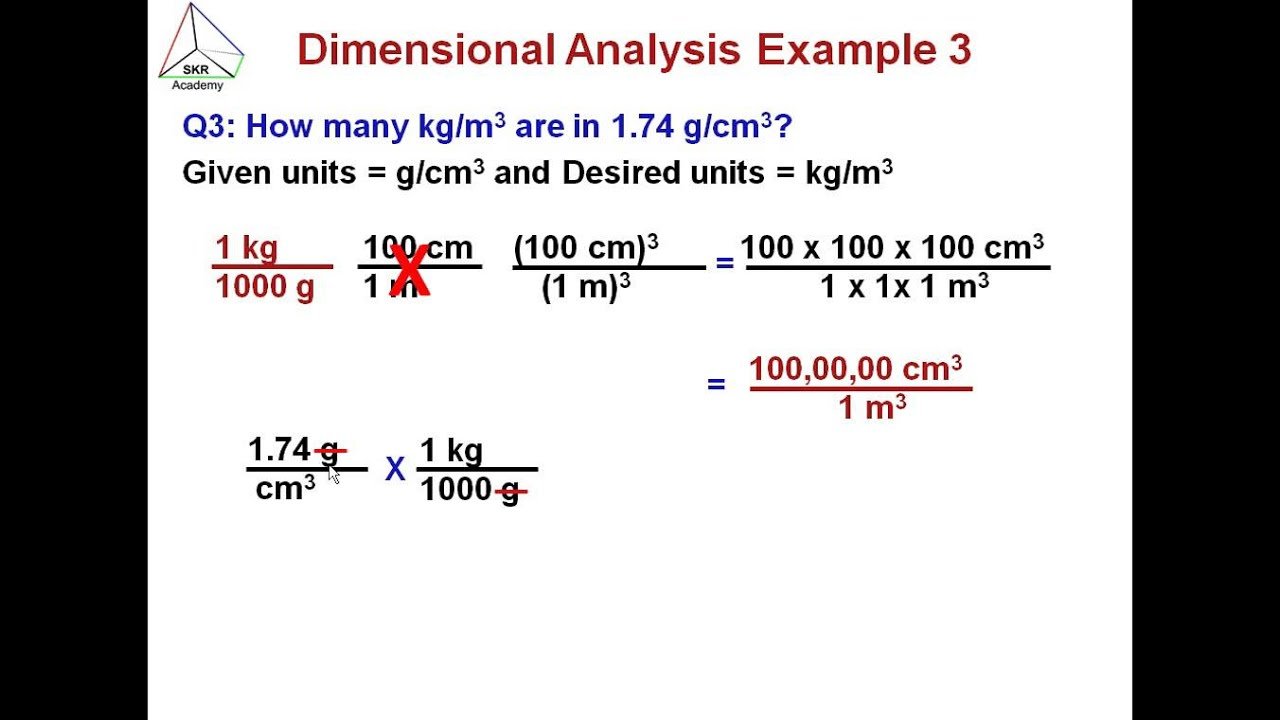
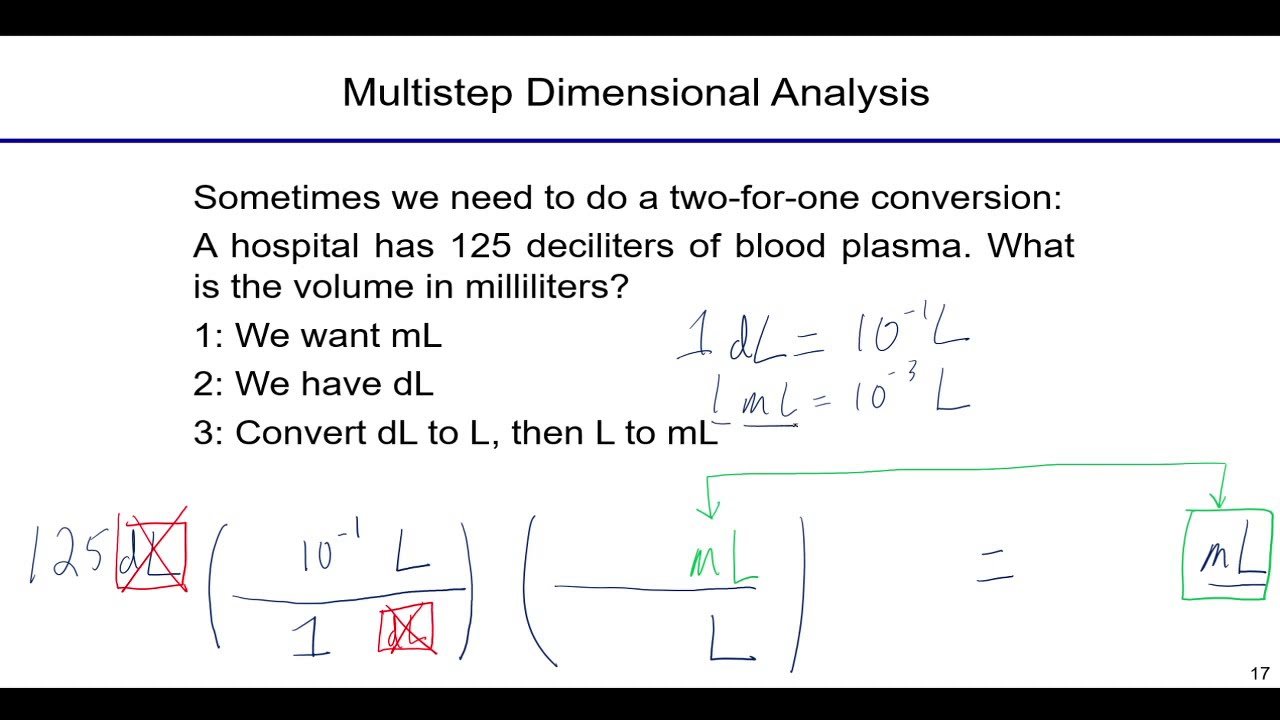
Unit Conversion And Dimensional Analysis

Dimensional analysis is also called Factor Label Method or Unit Factor Method because we use conversion factors to get the same units. To help you understand the stated better, lets say you want to know how many meters make 3 km?

We know that 1000 meters make 1 km,

Therefore,

3 km = 3 × 1000 meters = 3000 meters

Here, the conversion factor is 1000 meters.

Read Also: Eoc Fsa Practice Test Algebra 1 No Calculator Portion

Other Fields Of Physics And Chemistry

Depending on the field of physics, it may be advantageous to choose one or another extended set of dimensional symbols. In electromagnetism, for example, it may be useful to use dimensions of T, L, M and Q, where Q represents the dimension of electric charge. In thermodynamics, the base set of dimensions is often extended to include a dimension for temperature, Î. In chemistry, the amount of substance is also defined as a base dimension, N.In the interaction of relativistic plasma with strong laser pulses, a dimensionless relativistic similarity parameter, connected with the symmetry properties of the collisionless Vlasov equation, is constructed from the plasma-, electron- and critical-densities in addition to the electromagnetic vector potential. The choice of the dimensions or even the number of dimensions to be used in different fields of physics is to some extent arbitrary, but consistency in use and ease of communications are common and necessary features.

Dimensional Analysis Definition Chemistry

Answer:

Dimensional Analysis is a way chemists and other scientists convert units of measurement . We can convert any unit to another unit of the same dimension . This means we can convert some number of seconds into another unit of time , such as minutes , because we know that there are always 60 seconds in one minute . or we can convert some amount of mass from grams to kilograms knowing that there are always 1000 grams in one kilogram . or we can convert lengths, say, from kilometers to miles , although metric units are most frequently used in chemistry . with a known conversion factor , it is sometimes , possible to convert to a new dimension , for instance , 1 kilogram of pure water is equal to 1 liter . using that knowledge , you could convert a volume of water to a mass of water , or vice versa .

Explanation:

Also Check: The Founder Of Behaviorism Was:

Percentages Derivatives And Integrals

Percentages are dimensionless quantities, since they are ratios of two quantities with the same dimensions. In other words, the % sign can be read as “hundredths”, since 1% = 1/100.

Taking a derivative with respect to a quantity adds the dimension of the variable one is differentiating with respect to, in the denominator. Thus:

• position has the dimension L
• derivative of position with respect to time has dimension Tâ1Lâlength from position, time due to the gradient
• the second derivative / dt, acceleration) has dimension Tâ2L.

Likewise, taking an integral adds the dimension of the variable one is integrating with respect to, but in the numerator.

• force has the dimension Tâ2LM
• the integral of force with respect to the distance the object has travelled #Mathematical_calculation” rel=”nofollow”> work) has dimension Tâ2L2M.

In economics, one distinguishes between stocks and flows: a stock has units of “units” , while a flow is a derivative of a stock, and has units of “units/time” .

The most basic rule of dimensional analysis is that of dimensional homogeneity.

Only commensurable quantities may be compared, equated, added, or subtracted.

However, the dimensions form an abelian group under multiplication, so:

One may take ratios of incommensurable quantities , and multiply or divide them.

f + ⯠^a_x^=a_+a_x+a_x^+a_x^+\cdots }

How To Use The Dimensional Analysis Calculator

The procedure to use the Dimensional Analysis calculator is as follows:Step 1: Enter two physical quantities in the respective input fieldStep 2: Now click the button Submit to get the analysisStep 3: Finally, the dimensional analysis will be displayed in the new window

Here, the SI units are given along with their respective dimension symbol.

You May Like: What Is Figure Ground Perception Psychology

Polynomials And Transcendental Functions

Scalar arguments to transcendental functions such as exponential, trigonometric and logarithmic functions, or to inhomogeneous polynomials, must be dimensionless quantities.

While most mathematical identities about dimensionless numbers translate in a straightforward manner to dimensional quantities, care must be taken with logarithms of ratios: the identity log = loga â logb, where the logarithm is taken in any base, holds for dimensionless numbers a and b, but it does not hold if a and b are dimensional, because in this case the left-hand side is well-defined but the right-hand side is not.

Similarly, while one can evaluate monomials of dimensional quantities, one cannot evaluate polynomials of mixed degree with dimensionless coefficients on dimensional quantities: for x2, the expression 2 = 9 m2 makes sense , while for x2 + x, the expression 2 + 3 m = 9 m2 + 3 m does not make sense.

However, polynomials of mixed degree can make sense if the coefficients are suitably chosen physical quantities that are not dimensionless. For example,

1

ft }}}} is identical to the dimensionless 1, so multiplying by this conversion factor changes nothing. Then when adding two quantities of like dimension, but expressed in different units, the appropriate conversion factor, which is essentially the dimensionless 1, is used to convert the quantities to identical units so that their numerical values can be added or subtracted.

Lesson Plan: Chemical Measurement Unit Plan

The AACT High School Classroom Resource library has everything you need to put together a unit plan for your classroom: lessons, activities, labs, projects, videos, simulations, and animations. We constructed a unit plan for introducing concepts student need to collect and use chemical measurements: Percent Composition, Metric Units, Accuracy and Precision, Percent Error, Density, Scientific Notation, Significant Figures, and Unit Conversion. This unit is designed to be used at beginning of the school year and modified based on student math abilities.

Dimensional Analysis, SI Units | High School

Also Check: Endpoint Geometry Example

Access Is An Aact Member Benefit Activity: Cooking With Conversions

In this activity, students will be given a common homemade recipe for German chocolate cake with measurements in English units. They will be asked to convert the English ingredients list to metric units through scientific calculations. Students will also be asked to identify the ingredients as solid, liquid or gas. While reviewing the cooking procedures, students will classify certain steps as containing compounds or mixtures as well as identify whether chemical or physical changes are taking place. The culinary chemistry involved in this lesson should be introduced throughout the activity.

Dimensional Analysis, Measurements | High School

Access Is An Aact Member Benefit Project: Food Chemistry Infographic

In this project, students will research the chemical content and nutritional value of their favorite holiday foods, presenting their findings in an infographic. They will also include a recipe, which they will convert to metric units to practice dimensional analysis. As an extension to explore experimental design, they could alter one aspect of the recipe and observe how that change affects the final product.

Dimensional Analysis, Measurements | Middle School, High School

Don’t Miss: Definition Of Abiotic In Biology

Dimensional Analysis: Definition Examples And Practice

As a student of Biology or any of the sciences, you will have to use math of some kind, and theres a good chance that you will find dimensional analysis to be helpful. Math equations and other conversions can be overwhelming for some, but dimensional analysis doesnt have to be once you learn it, its relatively easy to use and understand.

Well give you the basics and give you some easy-to-understand examples that you might find on a dimensional analysis worksheet so that you can have a general understanding about what it is and how to use the technique in all types of applications as you continue to take science courses.

A Third Example: Demand Versus Capacity For A Rotating Disc

Consider the case of a thin, solid, parallel-sided rotating disc of axial thickness t and radius R . The disc has a density Ï , rotates at an angular velocity Ï and this leads to a stress S in the material. There is a theoretical linear elastic solution, given by Lame, to this problem when the disc is thin relative to its radius, the faces of the disc are free to move axially, and the plane stress constitutive relations can be assumed to be valid. As the disc becomes thicker relative to the radius then the plane stress solution breaks down. If the disc is restrained axially on its free faces then a state of plane strain will occur. However, if this is not the case then the state of stress may only be determined though consideration of three-dimensional elasticity and there is no known theoretical solution for this case. An engineer might, therefore, be interested in establishing a relationship between the five variables. Dimensional analysis for this case leads to the following non-dimensional groups:

demand/capacity = ÏR2Ï2/S

thickness/radius or aspect ratio = t/R

Through the use of numerical experiments using, for example, the finite element method, the nature of the relationship between the two non-dimensional groups can be obtained as shown in the figure. As this problem only involves two non-dimensional groups, the complete picture is provided in a single plot and this can be used as a design/assessment chart for rotating discs

Don’t Miss: Reversible Figure Ground Relationship

Finance Economics And Accounting

In finance, economics, and accounting, dimensional analysis is most commonly referred to in terms of the distinction between stocks and flows. More generally, dimensional analysis is used in interpreting various financial ratios, economics ratios, and accounting ratios.

• For example, the P/E ratio has dimensions of time , and can be interpreted as “years of earnings to earn the price paid”.
• In economics, debt-to-GDP ratio also has units of years .
• In financial analysis, some bond duration types also have dimension of time and can be interpreted as “years to balance point between interest payments and nominal repayment”.
• Velocity of money has units of 1/years : how often a unit of currency circulates per year.
• Interest rates are often expressed as a percentage, but more properly percent per annum, which has dimensions of 1/years.