Difference Between Algebraic Expression And Equation
| Expression | Equation |
| An expression is a number, a variable, or a combination of numbers and variables and operation symbols. | An equation is made up of two expressions connected by an equal sign. |
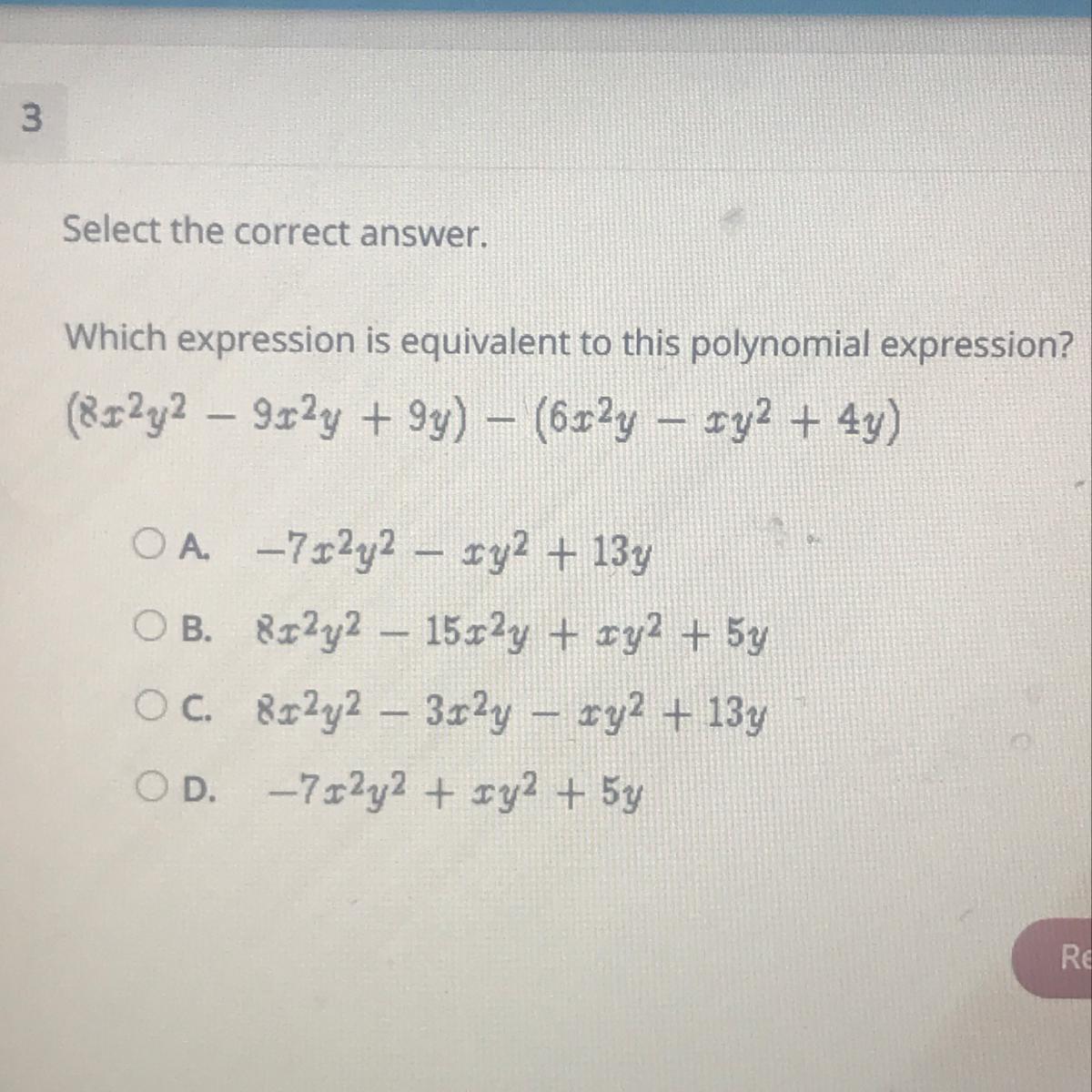
| Word example: The sum of 8 and 3 | Word example: The sum of 8 and 3 is equal to 11. |
| Expression: 8 + 3 | |
| Expression with exponent: x2 4 | Equation with exponent: x2 4 = 0 |
What Is Numerical Expression
The term numerical expression is made up of two words, numerical meaning numbers, and expression meaning phrase. Thus, it is a phrase involving numbers.
A numerical expression in mathematics can be a combination of numbers, and integers combined using mathematical operators such as addition, subtraction, multiplication, or division.
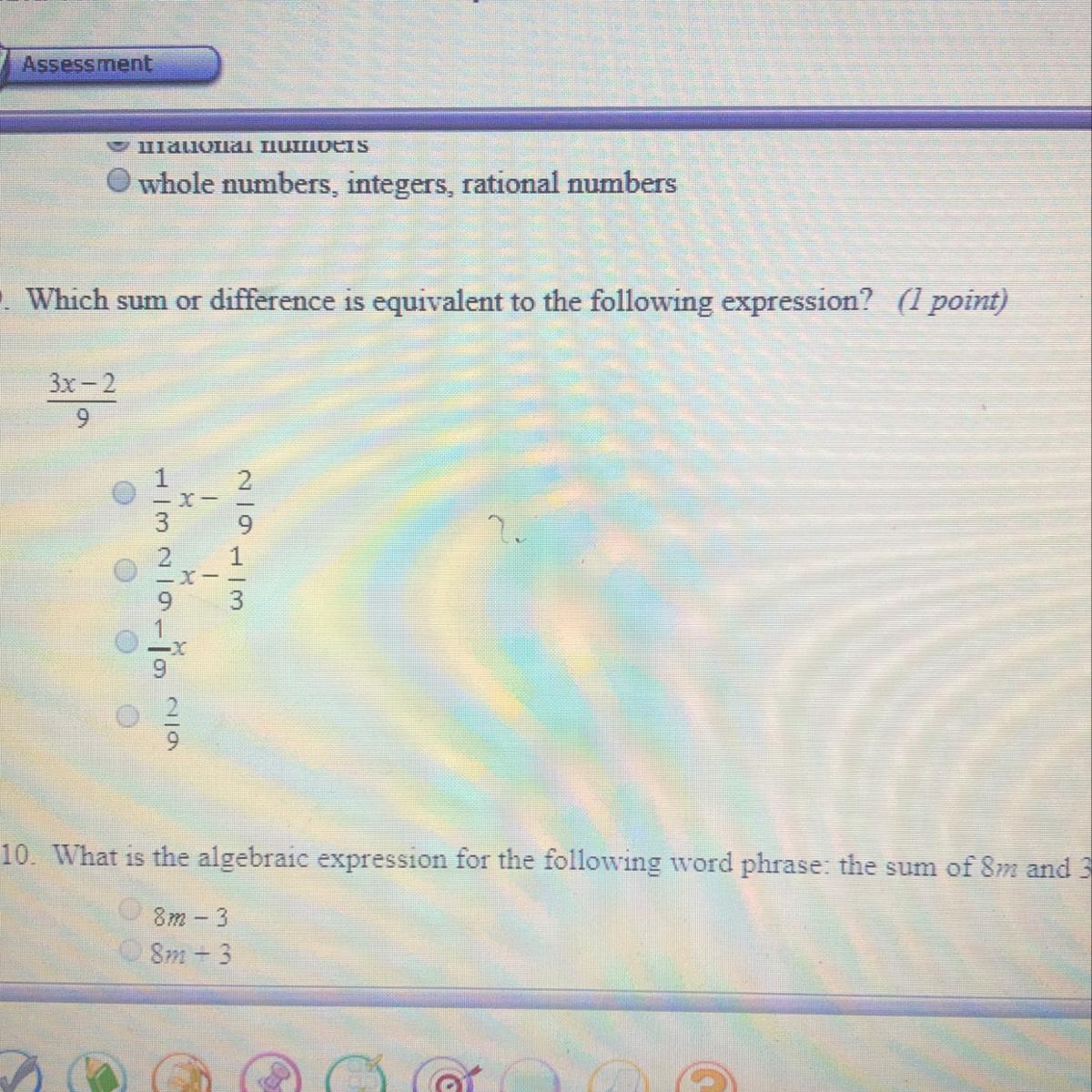
Testing Knowledge Of Mathematical Phrasing For Addition
Use the following questions and answers to help your student learn the correct way to formulate Algebraic expressions based on mathematical phrasing:
- Question: Write seven plus n as an Algebraic expression.
- Question: What expression is used to mean “a number increased by eight.”
- Answer: n + 8 or 8 + n
- Question: Write an expression for “the sum of a number and 22.”
- Answer: n + 22 or 22 + n
As you can tell, all of the questions above deal with Algebraic expressions that deal with the addition of numbers remember to think “addition” when you hear or read the words add, plus, increase or sum, as the resulting Algebraic expression will require the addition sign .
Also Check: Differential Equations And Linear Algebra 4th Edition
Different Terms In Algebra
There are two kinds of terms in algebra: Like Terms and Unlike Terms.
Like Terms: Like terms are terms whose variables and exponent power are the same. They can be simplified by combining them. The operations of addition and subtraction can be performed on them together.
For example, 5x + 8x is an algebraic expression with like terms.
Unlike Terms: Unlike terms are those terms whose variables and their exponents are different from each other. They cannot be simplified by combining them. The operations of addition and subtraction cannot be performed on them together.
For example, 5x + 8y is an algebraic expression with unlike terms.
Let Us Learn about Polynomials
Polynomial comprises two Greek words: the word poly means many and nominal means terms. So, we get the phrase many terms. Polynomials are classified into three different types based on the number of terms it consists of.
The three types of polynomials are:
Algebra: Expressions And Equations
Mathematics is known as the Queen of Science. Algebra is a special branch of mathematics that deals with numbers, shapes and letters. We use concepts of mathematics everywhere, every day, almost in every situation. So far, we have used lots of numbers, lots of shapes and figures. Here, we are going to use some letters in math. Yes, it is algebra lets learn more about it.
Don’t Miss: What Is Density In Physics
Algebraic Expression And Equation Problem
Question: Find the value of x in the given equation: 4x + 10 = 30
Solution:
Given Equation: 4x + 10 = 30
Keep the variable term on the left-hand side, and move the constant term on the right-hand side.
So, the given equation is written as:
4x = 30-10
4x = 20
x = 20/4
x = 5.
Therefore, the value of x is 5.
Alternative Method:
How To Simplify Expressions In Math
We can simplify expressions in math by reducing the given expression in the simplest form. If it is a numerical expression, then it can be simplified by finding the value of the expression. If it is an algebraic expression, then it can be simplified by reducing it to the simplest form such that it cannot further be reduced.
You May Like: How To Know If You Have Chemistry With Someone
What Is An Expression In Math
An expression in math is a sentence with a minimum of two numbers/variables and at least one math operation in it. Let us understand how to write expressions. A number is 6 more than half the other number, and the other number is x. This statement is written as x/2 + 6 in a mathematical expression. Mathematical expressions are used to solve complicated puzzles.
Simplifying Expression In Math
Expressions can be simplified to form an answer. For example, 3+6-2 is an expression that can be simplified to 7. There are two different ways to simplify arithmetic expressions and algebraic expressions. We use the BODMAS rule to simplify them. In case of algebraic expressions, like terms can be added or subtracted for simplification. Like terms are those that have the same variable raised to the same power. So, we can easily add or subtract two or more like terms by adding their coefficients. For example, 2x+5x results in 7x, whereas 7ab-b is an expression that has two unlike terms, which cannot be added.
In the case of expressions having multiple terms and operators, we apply the PEMDAS rule . For example, let us simplify 23 – 6 + 7 × 3. Here, as there are no brackets and exponents, we will first evaluate 7 × 3 which is 21. Now, the expression is 23-6+21. Now, there are two operators, addition and subtraction. Since both are same level operations and subtraction is first from the left side, we will subtract 6 from 23, i.e., 17. Now our expression has become 17+21, which results in 38 and 38 is the simplified value of the expression 23 – 6 + 7 × 3.
Important Notes on Expressions in Math:
- An expression has 3 parts: constant, variable, and term.
- There are 3 types of expressions: arithmetic/numerical, fractional, and algebraic.
- Polynomial is a type of variable expression.
Related Articles
You May Like: What Is The Definition Of Mantle In Geography
Formal Languages And Lambda Calculus
Formal languages allow formalizing the concept of well-formed expressions.
In the 1930s, a new type of expressions, called lambda expressions, were introduced by Alonzo Church and Stephen Kleene for formalizing functions and their evaluation. They form the basis for lambda calculus, a formal system used in mathematical logic and the theory of programming languages.
The equivalence of two lambda expressions is undecidable. This is also the case for the expressions representing real numbers, which are built from the integers by using the arithmetical operations, the logarithm and the exponential .
S Of An Expression In Math
An expression in Math is made up of the following:
a) Constant: itis a fixed numerical value.
Example: $7, 45, 4\frac, 18, \sqrt, 7 + \sqrt$
b) Variables: they do not take any fixed values. Values are assigned according to the requirement.
Example: a, p, z
c) Terms: can be constants, variables or constants multiplied by variable/. Each term in an expression is separated by + sign or sign
Example: In $5a + 2b -7$ the terms are: $5a, 2b, and 7$.
d) Operators: The four operations of addition , subtraction ,multiplication , division are used to combine the terms of an expression and are called operators.
Read Also: What Is Grid In Geography
How To Simplify Math Expressions
wikiHow is a wiki, similar to Wikipedia, which means that many of our articles are co-written by multiple authors. To create this article, 19 people, some anonymous, worked to edit and improve it over time. This article has been viewed 304,150 times.Learn more…
Math students are often asked to give their answers in “the simplest terms”in other words, to write answers as small as possible. Though a long, ungainly expression and a short, elegant one may technically equal the same thing, often, a math problem isn’t considered “done” until the answer has been reduced to the simplest terms. In addition, answers in the simplest terms are almost always the easiest expressions to work with. For these reasons, learning how to simplify expressions is a crucial skill for aspiring mathematicians.
Basic Mathematical Symbols With Name Meaning And Examples
The basic mathematical symbols used in Maths help us to work with mathematical concepts in a theoretical manner. In simple words, without symbols, we cannot do maths. The mathematical signs and symbols are considered as representative of the value. The basic symbols in maths are used to express mathematical thoughts. The relationship between the sign and the value refers to the fundamental need of mathematics. With the help of symbols, certain concepts and ideas are clearly explained. Here is a list of commonly used mathematical symbols with names and meanings. Also, an example is provided to understand the usage of mathematical symbols.
| Symbol |
|---|
Recommended Reading: Is Psychology Part Of Stem
Translating Words Into Mathematical Expressions
In this section we turn our attention to translating word phrases into mathematical expressions. We begin with phrases that translate into sums. There is a wide variety of word phrases that translate into sums. Some common examples are given in Table \, though the list is far from complete. In like manner, a number of phrases that translate into differences are shown in Table \.
| Phrase | |||
|---|---|---|---|
| sum of x and 12 | x + 12 | difference of x and 12 | x 12 |
| a) Phrases that are sums | b) Phrases that are differences |
Lets look at some examples, some of which translate into expressions involving sums, and some of which translate into expressions involving differences.
Example 1
Translate the following phrases into mathematical expressions:
Exercise
Translate the following phrases into mathematical expressions:
-
y 12
Example 2
Let W represent the width of the rectangle. The length of a rectangle is 4 feet longer than its width. Express the length of the rectangle in terms of its width W.
Solution
We know that the width of the rectangle is W. Because the length of the rectangle is 4 feet longer that the width, we must add 4 to the width to find the length.
Thus, the length of the rectangle, in terms of its width W, is 4 + W.
Exercise
- Answer
-
L 5
Example 3
What Does # Mean In A Mathematical Expression
What does # mean in this formula? )/)]1
I’ve tried to google it in various terms but haven’t found any examples of other formulas with it, and it’s also not explained in the appendices of the book I read.
- Dietrich BurdeSep 2 at 10:32
- $\begingroup$But some people use this also in a different context, i.e., for matrices, $A^$, or $A^$.$\endgroup$ Dietrich BurdeSep 2 at 10:39
- $\begingroup$Thank you both for your comments. @lulu, so in my first equation, hn = #/), the # refers to the number of x such that its index is between 1 and n and the x itself is between aj-1 and aj, right?$\endgroup$ Nin KhodorivskoSep 2 at 13:13
- $\begingroup$Well, that’s hard to parse. I have no idea what $x_i$ means nor what the $a_j$ are .$\endgroup$
Recommended Reading: What Is The Most Dangerous Biological Weapon
Example: Factor 4×2 9
Hmmm… there don’t seem to be any common factors.
But knowing the Special Binomial Products gives us a clue called the “difference of squares”:
Because 4×2 is 2, and 9 is 2,
So we have:
And that can be produced by the difference of squares formula:
= a2 b2
Where a is 2x, and b is 3.
So let us try doing that:
= 2 2 = 4×2 9
Yes!
So the factors of 4×2 9 are and :
Answer: 4×2 9 =
How can you learn to do that? By getting lots of practice, and knowing “Identities”!
Here is a list of common “Identities” .
It is worth remembering these, as they can make factoring easier.
| a2 b2 |
There are many more like those, but those are the most useful ones.
What Does If And Only If Mean In Mathematics
To understand if and only if, we must first know what is meant by a conditional statement. A conditional statement is one that is formed from two other statements, which we will denote by P and Q. To form a conditional statement, we could say if P then Q.
The following are examples of this kind of statement:
- If it is raining outside, then I take my umbrella with me on my walk.
- If you study hard, then you will earn an A.
- If n is divisible by 4, then n is divisible by 2.
You May Like: How Is Sociology Different From Psychology
Other Forms Of Algebraic Expressions
Multiplication, division, exponentials, and parentheticals are all part of the ways in which Algebraic expressions function, all of which follow an order of operations when presented together. This order then defines the manner in which students solve the equation to get variables to one side of the equals sign and only real numbers on the other side.
Like with addition and subtraction, each of these other forms of value manipulation come with their own terms that help identify which type of operation their Algebraic expression is performing words like times and multiplied by trigger multiplication while words like over, divided by, and split into equal groups denote division expressions.
Once students learn these four basic forms of Algebraic expressions, they can then begin to form expressions that contain exponentials and parentheticals . An example of an exponential expression with parentheticals would be 2×2 + 2.
Are Algebraic Expressions Polynomials
No, not all algebraic expressions are polynomials. But all polynomials are algebraic expressions. The difference is polynomials include only variables and coefficients with mathematical operations but algebraic expressions include irrational numbers in the powers as well.
Also, polynomials are continuous function but algebraic expression may not be continuous sometimes .
Recommended Reading: Which Theorist Published Research Related To The Psychology Of Personality
Types Of Expressions In Math
There are three basic types of mathematical expressions. Based on the terms that they have, they can be classified as arithmetic/numerical expressions, fractional expressions, and algebraic expressions. Let us learn more about each of them with the help of the table given below:
| Types of Mathematical Expressions |
|---|
Look at a few more examples of expressions and equations through the figure given below:
Expression Definition In Math
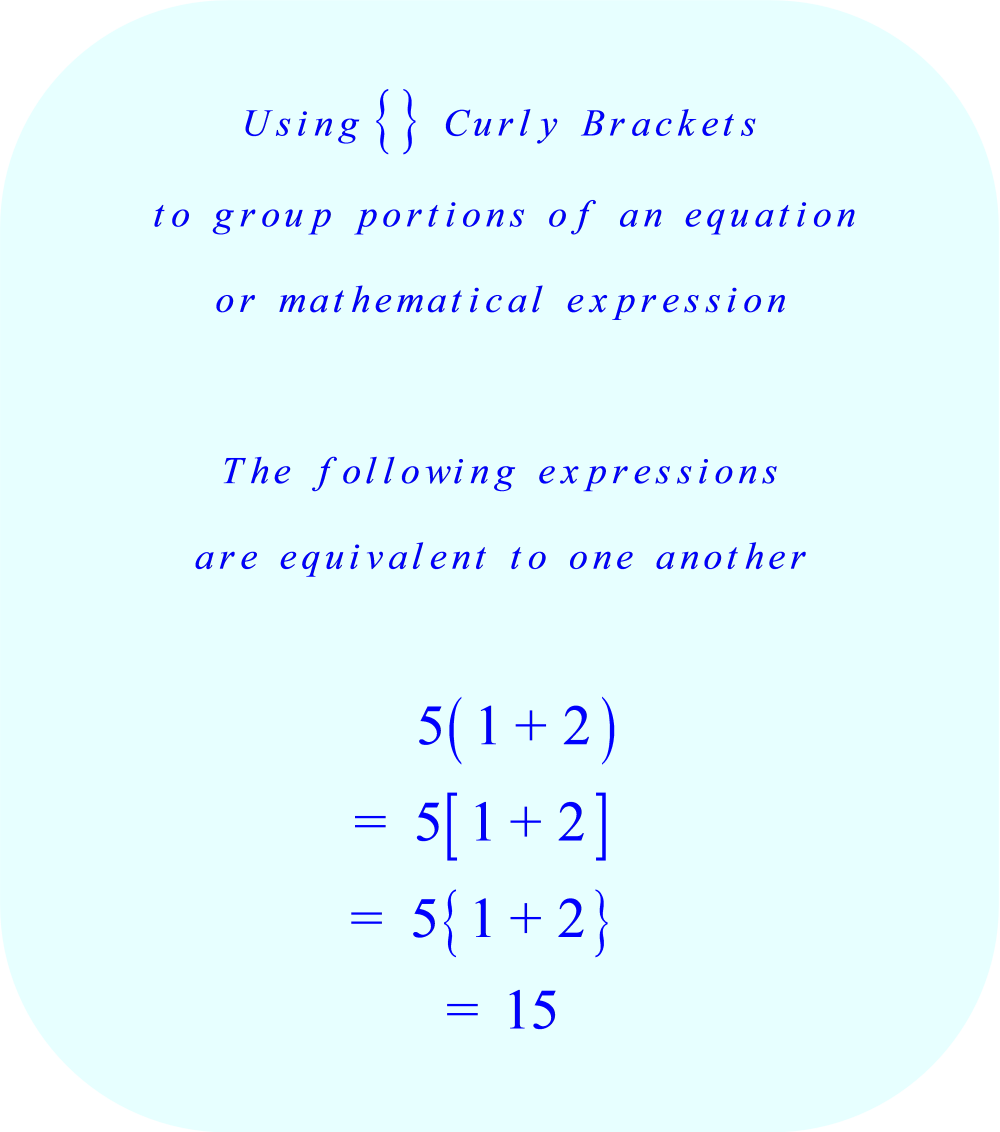
An expression is a combination of terms that are combined by using mathematical operations such as subtraction, addition, multiplication, and division. The terms involved in an expression in math are:
- Constant: A constant is a fixed numerical value.
- Variable: A variable is a symbol that doesn’t have a fixed value.
- Term: A term can be a single constant, a single variable, or a combination of a variable and a constant combined with multiplication or division.
- Coefficient: A coefficient is a number that is multiplied by a variable in an expression.
Also Check: What Is An Experimental Group In Psychology
Examples Of Numerical Expressions
We can form a numerical expression by combining numbers with various mathematical operators. There is no limit to the number of operators a numerical expression may contain. Some numerical expressions use only one operator between two numbers, and some may contain more.
Some examples of numerical expression are given below:
10 + 5
72 ÷ 8 × 5 4 + 1
82 + 4 10
How To Derive Algebraic Expressions
An algebraic expression is a combination of constants, variables and algebraic operations . We can derive the algebraic expression for a given situation or condition by using these combinations.
For example, Sima age is thrice more than Tina. And the total age of Sima and Tina is 40. Expressing the algebraic form of this condition
3x + x = 40 4x = 40 where x is the age of Tina.
Don’t Miss: Holt Algebra 2 Powerpoint Presentations
How To Solve Algebraic Equations
An algebraic equation contains two algebraic expressions separated by an equal sign in between. The primary purpose of solving algebraic equations is to find the unknown variable in the given expression. While solving the equation, separate the variable terms on one side and constant terms on another side. The variable term can be isolated using the various arithmetic operations such as addition, subtraction, multiplication, division, and other operations like finding square roots, etc.
Types Of Algebraic Expressions
Algebraic expressions are classified on the basis of the number of terms in the expression. The various types of algebraic expressions are:
Also Check: What Does Standard Deviation Mean In Math
Using The Order Of Operations