What Does Combining Like Algebraic Terms Mean
Combining like terms means, when an algebraic expression consists of like terms i.e. same variables, that expression can be further simplified to a point where no further calculation is required. After combining the like terms, an expression can be solved easily. For example: 5x – 10y + 34x + 2y – 3xy can be further simplified to 39x – 8y -3xy.
What Are Like Terms And How Do You Combine Them
What are like terms? They are terms whose variables and exponents have the same value. If two terms have different variables or different exponents, they are called unlike terms. Here are some examples of like and unlike terms:
Like terms:
Unlike terms:
Let’s break down the defining features of like terms and show you how to combine them.
Simplify By Combining Like Terms
Lets understand this with an example of 2xy + 5×2 + 6xy + 9×2 + 20y2
Notice the terms 2xy and 6xy, as well as 5×2 and 9×2 have common factors. Only the numerical coefficients are different. Apart from that, all the variable factors are the same, so we can add these terms.
On adding like terms,
2xy + 6xy = 8xy,
5×2 + 9×2 = 14×2
These terms have variable factors in common, and an arithmetic operation can be performed on them, as they are called like terms. After adding the like terms, above the expression can be written again.
2xy + 5×2 + 6xy + 9×2 + 20y2 = 8xy + 14×2 + 20y2
If we observe the expression now, none of the terms has any common factors and we cannot perform any further arithmetic operation on them. These terms are called, unlike terms.
You May Like: How Are Detergents Made Chemistry
Exponents And Square Roots
In this section, we expand our skills with applying the order of operation rules to expressions with exponents and square roots. If the expression has exponents or square roots, they are to be performed after parentheses and other grouping symbols have been simplified and before any multiplication, division, subtraction, and addition that are outside the parentheses or other grouping symbols.
Recall that an expression such as 7^ is exponential notation for 7\cdot7.
Exponents are a way to represent repeated multiplication the order of operations places it before any other multiplication, division, subtraction, and addition is performed.
Definition Of Unlike Terms
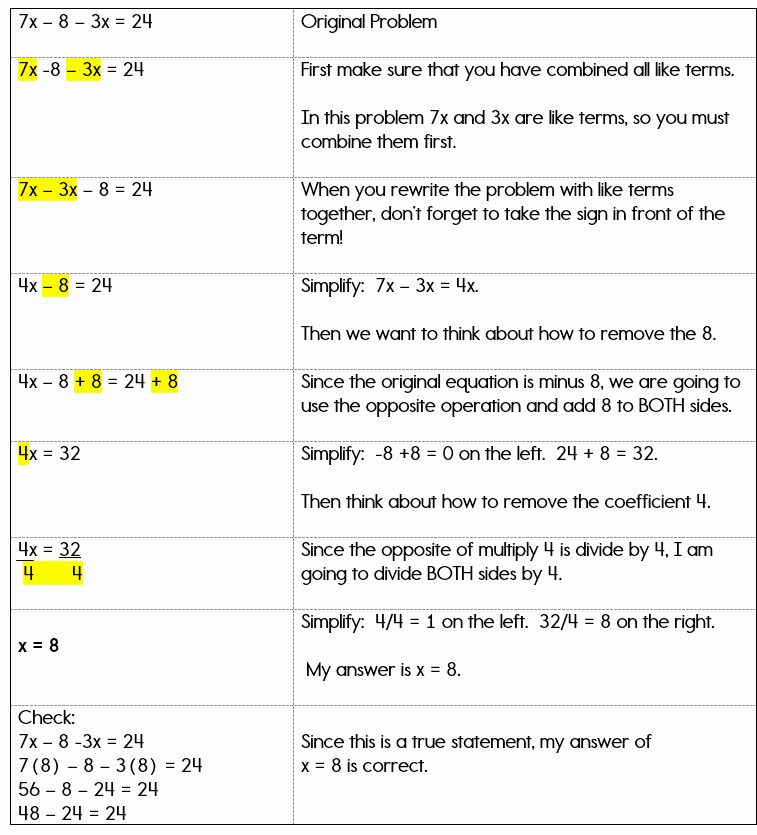
Unlike terms are those terms whose variables and their exponents are different from each other. In an expression, the coefficient is different, the variables are different i.e 2 variables, and the exponent powers are different, that expression is known to obtain, unlike terms. For example, the algebraic expression 3x + 9y where x and y are two different variables with different coefficients is known as, unlike algebraic terms.
Also Check: What Are The 4 Major Goals Of Psychology
Solved Examples On Like And Unlike Terms
1. Find out Like terms : 2x² + 6x + 4y + 9x
This expression may be rewritten as follows if it is rearranged:2x²+ 9x + 6x + 4yWhereas like terms are 9x and 6xSo, Like terms have identical variables raised to the same exponent.
2. Find out Unlike terms : 3xy + 2x² + 7xy +2y² +3x²
This expression may be rewritten as follows if it is rearranged:3x² +2x² + 2y²+7xy + 3xy Where unlike terms are 2y²So, Unlike terms that have different variables raised to the different exponent.
See More:
A Definition Of Like Terms In Algebra
- A term is a collection of numbers, letters and brackets all multiplied together. The letters may have exponents.2x2y is a term.
- A variable is a letter that stands for a number. Its value is not fixed, its value can change.The x and y in 2x2y are variables.
- An exponent tells you how many times a value is multiplied by itself.The 2 in 2x2y is an exponent.
- A coefficient is a constant placed in front of the variables in the term.The 2 in 2x2y is a coefficient.A constant has a fixed value which does not change. It is often a number .Constants can also be letters…
Recommended Reading: What Does Diminished Mean In Math
What Are Like Terms
In Algebra, the like terms are defined as the terms that contain the same variable which is raised to the same power. In algebraic like terms, only the numerical coefficients can vary. We can combine the like terms to simplify the algebraic expressions so that the result of the expression can be obtained very easily.
For example, 4x + 10x is an algebraic expression with like terms. In order to simplify this algebraic expression, we can add the like terms. Thus, the simplification of the given expression is 14x. In the same way, you can perform all the arithmetic operations on the like terms.
Addition And Subtraction Of Like Terms
Consider another expression 10×2 – 4×2, here we see that the variables have the same exponent but the coefficients are different. We can further simplify this expression by subtracting the same variables from each other. This ispossible since the variables and the exponents are the same irrespective of the coefficients being different. The coefficients can be considered as normal numbers along with variables and exponent values, which remain the same after subtraction. Hence, after simplifying the expression we get 10×2 – 4×2 = 6×2. The process of simplifying the expression is known as combining like terms. The addition of like terms is simple, for example add the expression 5z + 12z + 32z = z = 49z.
Read Also: What Is Psychological Safety At Work
What Are Like And Unlike Algebraic Terms
Like algebraic terms are those terms whose variables and their exponent power are the same. The coefficient of these variables can be different. Algebraic-like terms are terms that are similar to each other. These like terms in the algebraic expression can be combined to simplify the expression to derive the answer in a simple manner. For example: 12x – 6x, where x is the variable and 12 and 6 are the different coefficients.
Unlike algebraic terms are those terms whose variables and their exponents are different from each other. In an expression, the coefficient is different, the variables are different i.e 2 variables, and the exponent powers are different, that expression is known to obtain, unlike terms. For example, 3z – 8x, where z and x are 2 variables and 3 and 8 are the coefficients.
What Does Combine Like Terms Mean
Terms in an algebraic expression are normally separated by addition or subtraction.
For instance, a monomial expression has only one term. For example, 3x, 5y, 4x, etc. Similarly, a binomial expression contains two terms, for instance, 3x + y, 2x + 7, x + y etc. A trinomial contains three terms, whereas polynomials of higher degrees contain many terms.
Like terms in Algebra are terms that contain identical variables and exponents, regardless of their coefficients. Like terms are combined in algebraic expression so that the result of the expression can be calculated with ease.
For example, 7xy + 6y + 6xy is an algebraic equation whose terms are 7xy and 6xy. Therefore, this expression can be simplified by combining like terms as 7xy + 6xy + 6y = 13xy + y. You can note that, when combining like terms, we only add the coefficients of the terms.
On the other hand, unlike terms are terms that do not have identical variables and exponents.
For example, an expression 4x + 9y contains terms because variables x and y are different and are not raised to the same power.
Read Also: How To Find Total Energy Physics
Defining And Combining Like Terms It’s Easy
Algebraic expressions with a lot of terms, variables, and exponents can be intimidating. But they become much less scary when you know how to identify and combine like terms.
Like terms have variables and exponents that match. When you know how to group the like terms of an expression, you can simplify and shorten them, making it much easier to solve.
Addition And Subtraction Of Unlike Terms
The simplification of expressions or combining like terms cannot be done on unlike terms, as the variables and exponents are not similar. For example, 8xy + 6y – 9x – 10×2, as seen here, there are different variables, exponents, and coefficients. This expression cannot be simplified as all the terms are different from each other.
Don’t Miss: How Does Australia’s Geography Affect Its Economy
Combine Like Terms Methods & Examples
Before discussing like and unlike terms, lets take a quick review of an algebraic expression. In mathematics, an algebraic expression is a mathematical sentence made up of variables and constants, and operators such as addition and subtraction.
A variable in the expression is a term whose value is unknown, whereas a constant term has a definite value. The numerical number that accompanies a variable is called a coefficient. Examples of algebraic expressions are 3x + 4y -7, 4x 10, 2×2 3xy + 5 etc.
In this article, we will learn the meaning of like terms and how to combine them.
Can We Add Or Subtract Like And Unlike Algebraic Terms
We can add or subtract any number of like algebraic terms. Terms that do not have the same variables are called unlike algebraic terms. An expression with unlike terms cannot be added or subtracted. For example: 7a – 46z , 5×3 + 45xy , 32z + xy + 12, all these three expressions contain two unlike terms.
You May Like: What Is Implantation In Biology
Like And Unlike Algebraic Terms Examples
Algebraic terms are those individual elements in an equation or an expression separated by + or – signs.
Consider the expression: 9x + 6y
We cannot simplify this any further as x and y are unknown. Check out another example.
4x² + 3x + 4y + 8x + 10x²
If rearranged, this expression can be re-written as:
10x² + 4x²+ 8x + 3x + 4y
= 14x² + 11x + 4y
Thus, it is seen that algebraic terms with the same variables are added to each other. The addition of certain terms was possible only because the variables in both these cases are the same even if the numerical coefficients are different which can be added as normal numbers and the variable factor remains as it is. Now, these terms which have the same variables are called like terms.
Thus, terms having identical variables raised to the same exponent are like terms.
So, what is 14x², 11x and 4y called? These are called unlike terms since the variables or exponent raised to these variables are unlike or not same.
Further operations on unlike terms cannot be directly performed. Based on this, it is clear that an algebraic expression consists of terms which can be categorized into like terms and unlike terms.
Lets consider another example 2xy + 4x² + 5xy +5y² +16x²
If you notice properly, the terms 2xy and 5xy, as well as 4x²and 16 x² have common factors. Only the numerical coefficients are different. Apart from that, all the variable factors are the same, so these terms can be added.
2xy + 5xy = 7xy
4x² +16x² = 20 x²
What Are Unlike Terms
Unlike terms have variables and exponents that differ from one another. When the coefficient of an expression is different, the variables are different , and the exponent powers are dissimilar. For instance, unlike algebraic terms is the algebraic equation 5x + 8y, where x and y are two separate variables with different coefficients.
Do Check
Recommended Reading: Algebra Operations With Radical Expressions
Like And Unlike Terms Definitions Solved Examples
Algebraic expressions are those that contain both constants and variables, as well as arithmetic operations like addition, subtraction, multiplication, and division. As an example: 4x +6y = 30 is an algebraic expression since it has three terms: 4x, 6y, and 30. The first two terms are 4x and 6y, with x and y being variables and 30 being a constant. As a result, algebraic terms are discrete parts of an equation separated by plus or minus signs. There are two kinds of algebraic words: like terms and unlike terms.
In this article, 6th-grade math students can obtain complete information about similar and dissimilar terms in an algebraic expression.
Video On How To Combine Like Terms
- Exponents and Bases: You may have noticed that like terms always have the same base and exponent.
- Regarding Coefficients: Also, the coefficient in front of a variable does not change whether or not terms are alike. For instance 3x and 5x and 11x are all like terms. The coefficients do not have anything at all to do with whether or not the terms are like. All that matters is that each of ‘x’ factors or ‘bases’ have the same exponent.
Don’t Miss: What Is Parsimony In Biology
Difference Between Like And Unlike Terms
Listed below is the difference between the two terms, like terms vs unlike terms. Let’s take a look.
| Like Terms | |
|---|---|
| Terms with the same variables and exponents. | Terms with different variables and exponents. |
| Like terms can be simplified by combining them. | Unlike terms cannot be simplified by combining them. |
| Addition and Subtraction of like terms can be done together. | Addition and Subtraction of unlike terms cannot be done together. |
| 13×2 + 5×2 is an example of like terms. | 7z – 25r is an example of unlike terms. |
| Like terms are also called similar terms. | Unlike terms are also called dissimilar terms. |
How Do We Find Like And Unlike Algebraic Terms
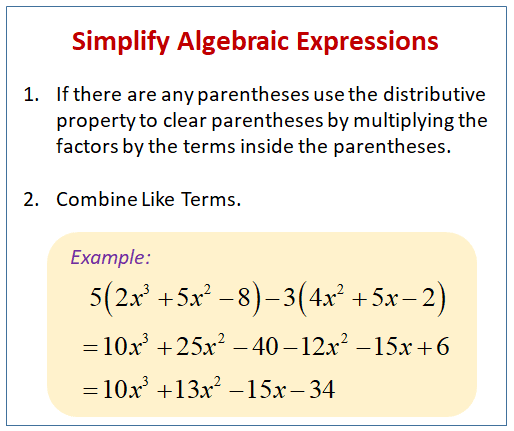
In a given algebraic expression, if the variables are the same irrespective of different coefficients along with the exponents being similar, those terms are called like terms. Whereas, if an expression consists of two different variables, different exponents, and different coefficients, that expression is known to have unlike algebraic terms. For example: Consider this expression 7xy + x – 9y + 13xy – 8x + 12z2 we can differentiate like and unlike algebraic terms. The like terms are 7xy + 13xy + x -8x which can be simplified further to 20xy – 7x. The, unlike terms, are 12z2 – 9y.
Also Check: What Math Classes Do You Need For Computer Science
Definition Of Like Terms
Like terms are those terms whose variables and their exponent power are the same. The coefficient of these variables can be different. Algebraic-like terms are terms that are similar to each other. These like terms in the algebraic expression can be combined to simplify the expression to derive the answer in a simple manner. For example, This is a like algebraic expression 8y + 2y where y is the same variable in the expression and the coefficients are different. To simplify it further, we can add the two like terms i.e. 8y + 2y = 10y. Hence, all arithmetic operations such as addition, subtraction, multiplication, and division can be performed only on like algebraic terms.
How To Combine Like Terms
Take a look at the following expression:
As you can see, this algebraic expression is made of like and unlike terms. To simplify it, well combine the like terms.
Let’s walk through the process of simplifying expressions by identifying the like terms in the expression. To do this, well look at the variables and exponents. Well keep the positive and negative signs of each value:
We’ve taken the original expression and separated it into like terms. Since the value of 14 has no variables, it’s considered a constant term.
Let’s combine the rest of the like terms and simplify the expression:
Now that we’ve combined these like terms, let’s write out our simplified expression:
Also Check: What Does Atp Mean In Biology
Like Terms Definition Simplification Examples
Like terms are those that include the same variables raised to the same power. The general and only difference is numerical coefficients. Only we can combine like terms in a phrase. To make algebraic formulas easier to deal with, we combine like terms to shorten and simplify them.
Like terms in mathematics refer to quantities that have the same variables and exponents. Lets learn the definitions of similar phrases and apply your maths abilities to appropriate sample problems. Students of 6th Grade Math can get ultimate grip on the concept by referring to this article.
Like And Unlike Algebraic Terms
Before getting into like terms and unlike terms, one should be able to distinguish what an algebraic term is? You can understand this by using an example. 9x + 4y² = 5 is an algebraic equation. It consists of 3 terms, i.e. 9x, 4y² and 5. The first two terms consist of variables and 5 is a constant. 9x + 4y² is an algebraic expression. It has two terms 9x and 4y². In this article, you are going to learn the definition of like and unlike algebraic terms in Maths with more examples.
Recommended Reading: How Can I Apply Psychology To My Future Life