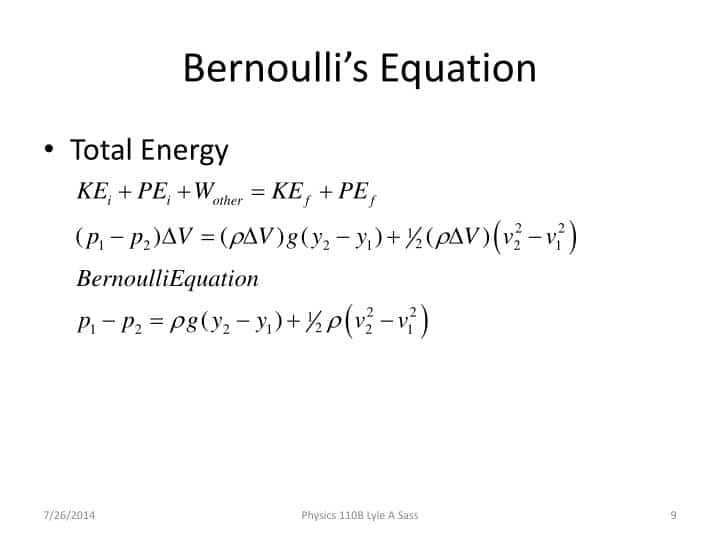
Using Kinetic Energy To Find Velocity Or Mass
How To Calculate The Total Energy From A Given Wavefunction
For a given wavefunction, $\Psi$ of a system, how one can calculate the total energy?
For example, the wavefunction for a particle of mass $m$ moving in a certain potential $U$ is given by:
$$\Psi=\left\ e^}\ e^}\ & ,& x> 0 \\ 0\ & ,& x\leq0 \end\right.$$
How one can express the potential energy $U$ and total energy $E$ in terms of $L$, $m$, $\hbar$?
- 1$\begingroup$Unless this is an energy eigenfunction, it does not have a well defined energy. If you want to calculate the expectation value of the energy, just apply the Hamiltonian operator $), multiply by the complex conjugate $\Psi^*$, and integrate.$\endgroup$ SandejoSep 5, 2021 at 3:23
- $\begingroup$You cannot express the potential in terms of the energy levels of the great multiplicity of wavefunctions. There is one potential function introduced in the differential equation and actually an infinity of possible wavefunction solutions of the equation. It is a one to many correlations.$\endgroup$
The wave function of the form$$\Psi = \psi_0 e^}$$corresponds to the stationary state of a system with energy $E$. This fact implies that $\psi_0$ is an eigenfunction of the Hamiltonian operator:$$-\frac\psi_0” + U\psi_0 = E\psi_0.$$Knowlege of $\psi_0$ and $E$ allows finding the potential $U$ from last equation:$$U = E + \frac\frac.$$For the $\psi_0$ from the example, it is easy to calculete $U$ for $x> 0$. $\psi_0 = 0$ for $x < 0$ implies that $U = +\infty$ in this area.
Gravitational Potential Energy And Total Energy
- Determine changes in gravitational potential energy over great distances
- Apply conservation of energy to determine escape velocity
- Determine whether astronomical bodies are gravitationally bound
We studied gravitational potential energy in Potential Energy and Conservation of Energy, where the value of \ remained constant. We now develop an expression that works over distances such that g is not constant. This is necessary to correctly calculate the energy needed to place satellites in orbit or to send them on missions in space.
Recommended Reading: What Is Psychology Class 11
Potential Energy Vs Kinetic Energy
These 2 energy states transfer energy back and forth to each other. Kinetic energy is the energy of motion. As an object moves, it is gaining kinetic energy. Potential energy is stored energy, which is the energy that can be used potentially for motion.
The gravitational potential energy can be calculated based on the equation Ug = m g h where Ug is gravitational potential energy, m = massMassThree-dimensional lesion that occupies a space within the breastImaging of the Breast of the object, g = acceleration due to gravity, and h = the height that the object has reached. If an apple is on the ground, it has no gravitational potential energy. As the apple is tossed upwards, it gains gravitational potential energy up to a peak value occurring at the maximum height of the apples travel.
An Example : Ionization Energy Of The Electron In A Hydrogen Atom
In the Bohr model of a hydrogen atom, the electron, if it is in the ground state, orbits the proton at a distance of r = 5.29 x 10-11 m. Note that the Bohr model, the idea of electrons as tiny balls orbiting the nucleus, is not a very good model of the atom. A better picture is one in which the electron is spread out around the nucleus in a cloud of varying density however, the Bohr model does give the right answer for the ionization energy, the energy required to remove the electron from the atom.
The total energy is the sum of the electron’s kinetic energy and the potential energy coming from the electron-proton interaction.
The kinetic energy is given by KE = 1/2 mv2.
This can be found by analyzing the force on the electron. This force is the Coulomb force because the electron travels in a circular orbit, the acceleration will be the centripetal acceleration:
Note that the negative sign coming from the charge on the electron has been incorporated into the direction of the force in the equation above.
This gives m v2 = k e2 / r, so the kinetic energy is KE = 1/2 k e2 / r.
The potential energy, on the other hand, is PE = – k e2 / r. Note that the potential energy is twice as big as the kinetic energy, but negative. This relationship between the kinetic and potential energies is valid not just for electrons orbiting protons, but also in gravitational situations, such as a satellite orbiting the Earth.
The total energy is:KE + PE = -1/2 ke2 / r = – 1/2 / 5.29 x 10-11
Don’t Miss: Why Do We Sleep Psychology
Kinetic Energy And Potential Energy
In this video were gonna talk about kinetic energy and potential energy but lets begin our discussion with kinetic energy. What is kinetic energy well think about the word kinetic what is what does that tell us kinetic kinetics has. To do with motion so kinetic energy really represents energy in motion anything that moves has kinetic energy so if. You have a ball moving at 5 meters per second it has kinetic energy if you have a block thats.
At rest its just sitting on the ground do nothing it has no kinetic energy so anything with mass and. Speed has kinetic energy the formula for it is ke is equal to 1/2 mv squared so kinetic energy depends. On the mass and the speed of the object the units for m is the kilogram the units for speed. Typically is in meters per second and if you use those units the kinetic energy will be in joules now. A typical question that you might see on a physics exam would be something like this if you double the.
Mass of an object thats moving what happens to the kinetic energy and what about if you double the speed. How do you answer those types of questions so lets talk about it first lets rewrite the formula ke is. Equal to 1/2 mv squared and notice that m is raised to the first power if you dont see a. Number there its a 1 now if we double the mass the kinetic energy will double what you can do. For a question like this is replace everything with from 1 except the stuff thats changing so well only change.
Calculating Heat In Joules
You May Like: Algebra 2 Cp Geometric Series Worksheet Answers
Oscillations About An Equilibrium Position
We have just considered the energy of SHM as a function of time. Another interesting view of the simple harmonic oscillator is to consider the energy as a function of position. Figure \ shows a graph of the energy versus position of a system undergoing SHM.
Consider the marble in the bowl example. If the bowl is right-side up, the marble, if disturbed slightly, will oscillate around the stable equilibrium point. If the bowl is turned upside down, the marble can be balanced on the top, at the equilibrium point where the net force is zero. However, if the marble is disturbed slightly, it will not return to the equilibrium point, but will instead roll off the bowl. The reason is that the force on either side of the equilibrium point is directed away from that point. This point is an unstable equilibrium point.
Figure \ shows three conditions. The first is a stable equilibrium point , the second is an unstable equilibrium point , and the last is also an unstable equilibrium point , because the force on only one side points toward the equilibrium point.
Consider one example of the interaction between two atoms known as the van Der Waals interaction. It is beyond the scope of this chapter to discuss in depth the interactions of the two atoms, but the oscillations of the atoms can be examined by considering one example of a model of the potential energy of the system. One suggestion to model the potential energy of this molecule is with the Lennard-Jones 6-12 potential:
\ \ldotp\]
Calculating Work In Joules
Also Check: What Is Conduction In Physics
Energy And Gravitationally Bound Objects
As stated previously, escape velocity can be defined as the initial velocity of an object that can escape the surface of a moon or planet. More generally, it is the speed at any position such that the total energy is zero. If the total energy is zero or greater, the object escapes. If the total energy is negative, the object cannot escape. Lets see why that is the case.
As noted earlier, we see that \ as \. If the total energy is zero, then as m reaches a value of r that approaches infinity, U becomes zero and so must the kinetic energy. Hence, m comes to rest infinitely far away from M. It has just escaped M. If the total energy is positive, then kinetic energy remains at \ and certainly m does not return. When the total energy is zero or greater, then we say that m is not gravitationally bound to M.
On the other hand, if the total energy is negative, then the kinetic energy must reach zero at some finite value of r, where U is negative and equal to the total energy. The object can never exceed this finite distance from M, since to do so would require the kinetic energy to become negative, which is not possible. We say m is gravitationally bound to M.
Example \: How Far Can an Object Escape?
Strategy
The object has initial kinetic and potential energies that we can calculate. When its speed reaches zero, it is at its maximum distance from the Sun. We use Equation 13.5, conservation of energy, to find the distance at which kinetic energy is zero.
Solution
Significance
Kinetic And Potential Energy
An object in motion possesses its energy of movement, which is equivalent to the work that would be required to bring it to rest. This is called its kinetic energy, and it is dependent on the square of the object’s velocity as well as one half of its mass . An object at rest in Earth’s gravitational field possesses potential energy by virtue of its altitude if it were to fall freely, it would gain kinetic energy equal to this potential energy. Potential energy is dependent on the object’s mass, its height and the acceleration due to gravity . Mathematically, this is:
You May Like: How To Calculate Average Uncertainty In Physics
Heres A Solved Question For You
Q: At the mean position, the total energy in simple harmonic motion is ________
a) purely kinetic b) purely potential c) zero d) None of the above
Answer: a) purely kinetic. At the mean position, the velocity of the particle in S.H.M. is maximum and displacement is minimum, that is, x=0. Therefore, P.E. =1/2 K x2 = 0 and K.E. = 1/2 k = 1/2 k = 1/2 ka2. Thus, the total energy in simple harmonic motion is purely kinetic.
Conservation Of Energy Physics Problems
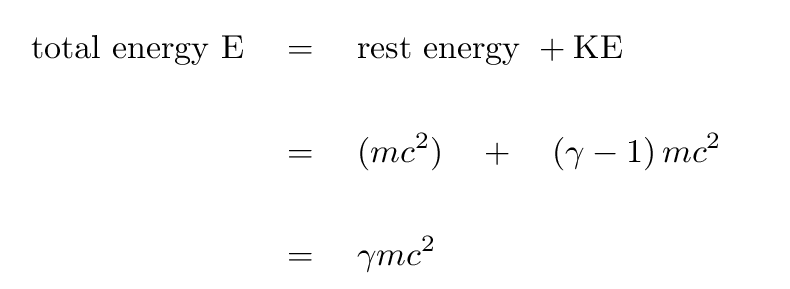
Lets work on this problem a block slides down a 150 meter inclined plane as shown in the picture below. Starting from rest what is the speed of the block when it reaches the bottom of the incline so were. Going to use conservation of energy to solve this problem so the initial mechanical energy has to equal the final. Mechanical energy the only force is acting on a block are conservative forces like gravity so mechanical energies can serve.
At point a the only form of energy that we have is potential energy and at point b choosing this. As the ground level theres no potential energy at point b however the block will have kinetic energy at point. B so we can set these two equal to each other as the block slides down potential energies being converted. To kinetic energy the potential energy of the block is mgh and the kinetic energy of the block is one. Half mv squared so we could cancel m therefore we dont need the mass of the block now im going.
To multiply both sides by two so on the left i have two g h is equal to two times. A half these will cancel so thats one and thats going to equal v squared taking the square root of. Both sides the final speed is going to be the square root of 2 g h so lets go ahead. And plug everything that we have so its 2 times the gravitational acceleration of 9.8 times the height of the. Block which is 150 meters high so by the way this is the height so thats 150 meters so if.
You May Like: Khan Academy Grade 5 Geometry
Calculating Electrical Energy In Joules