What Is Statistical Mechanics In Physics

What Is Statistical Mechanics About

• John and Marianne Schiffer Professor at Oberlin College

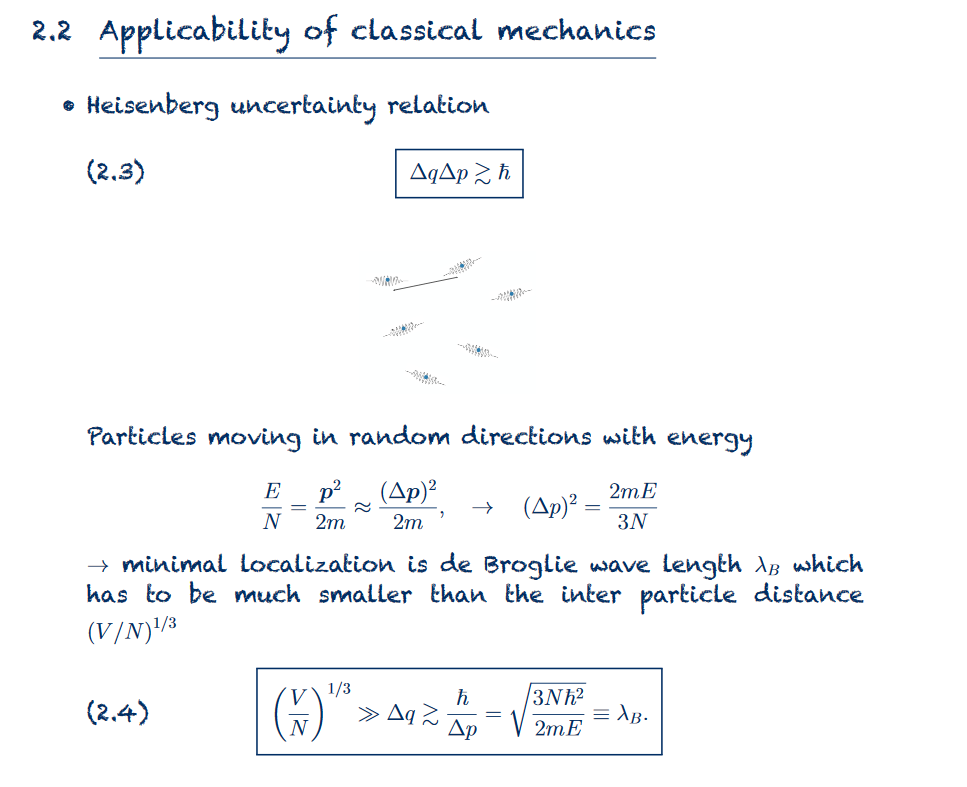
No headers

Statistical mechanics treats matter in bulk. While most branches of physics. . . classical mechanics, atomic physics, quantum mechanics, nuclear physics. . . deal with one or two or a few dozen particles, statistical mechanics deals with, typically, about a mole of particles at one time. A mole is \, considerably larger than a few dozen. Lets compare this to a number often considered large, namely the U.S. national debt. This debt is about 18 trillion dollars, so the national debt is about thirty trillionth of a mole of dollars.1 Even so, a mole of water molecules occupies only 18 ml or about half a fluid ounce. . . its just a sip.

1 In contrast, the Milky Way galaxy contains about 0.3 or 0.6 trillionth of a mole of stars. The entire universe probably contains fewer than a mole of stars.

Statistical Physics Of Fields

In abstract physics, statistical field theory, also known as SFT, is a theoretical outline that defines phase transitions. It does not mean a single theory but covers many models, such as magnetism, superfluidity, superconductivity, non-equilibrium phase transitions, wetting, and topological phase transition.

In statistical mechanics, SFT is denoted as an example where the degrees of freedom include fields or a field. Alternatively, the systems microstates are stated through field formations.

These theories are broadly familiar for describing the systems in biophysics or polymer physics, such as polyelectrolytes, polymer films, or nanostructured block copolymers.

Philosophy Of Statistical Mechanics

First published Thu Apr 12, 2001 substantive revision Fri Jul 24, 2015

Statistical mechanics was the first foundational physical theory inwhich probabilistic concepts and probabilistic explanation played afundamental role. For the philosopher it provides a crucial test casein which to compare the philosophers’ ideas about the meaning ofprobabilistic assertions and the role of probability in explanationwith what actually goes on when probability enters a foundationalphysical theory. The account offered by statistical mechanics of theasymmetry in time of physical processes also plays an important role inthe philosopher’s attempt to understand the alleged asymmetries ofcausation and of time itself.

Recommended Reading: How To Avoid Parallax Error In Physics

The Reduction Of Thermodynamics To Statistical Mechanics

It comes as no surprise that the relationship of the olderthermodynamic theory to the new statistical mechanics on which it isgrounded is one of some complexity.

The older theory had no probabilistic qualifications to its laws.But as Maxwell was clearly aware, it could not then be exactly trueif the new probabilistic theory correctly described the world. One caneither keep the thermodynamic theory in its traditional form andcarefully explicate the relationship its principles bear to the newerprobabilistic conclusions, or one can, as has been done in deeplyinteresting ways, generate a new statistical thermodynamics thatimports into the older theory probabilistic structure.

Conceptually the relationship of older to newer theory is quitecomplex. Concepts of the older theory must be related to the concepts of the newer theory .

Nothing in this complexity stands in the way of claiming thatstatistical mechanics describes the world in a way that explains whythermodynamics works and works as well as it does. But the complexityof the inter-relationship between the theories should make thephilosopher cautious in using this relationship as a well understoodand simple paradigm of inter-theoretic reduction.

Statistical Physics Of Particles

Statistical physics has its beginnings in efforts to define the thermal properties of matter with regard to its fundamental particles and has performed an essential part in the advancement of quantum mechanics.

The statistical physics of particles is a specific explanation of numerous particles in statistical mechanics.

A key requirement concept is that of a statistical ensemble that thoroughly highlights the properties of a large system at the cost of information about parameters of distinct particles.

When a group defines a system of particles with identical properties, their amount is named the particle number and is generally signified by N.

Also Check: Example Of Span Linear Algebra

What Is The Difference Between Thermodynamics And Statistical Mechanics

Statistical Mechanics is the theory of the physical behaviour of macroscopic systems starting from a knowledge of the microscopic forces between the constituent particles.

The theory of the relations between various macroscopic observables such as temperature, volume, pressure, magnetization and polarization of a system is called thermodynamics.

• 9$\begingroup$What he said. Statistical mechanics tries to justify the laws of thermodynamics by finding underlying microscopical models. Thermodynamics is just happy with finding the macroscopic laws. If the law was derived from stat mech, so be it, but it is not a prerequisite.$\endgroup$Jan 8 ’11 at 10:25
• 5$\begingroup$One could also add that since thermodynamics makes fewer assumptions about the microscopic details, it sometimes works even when you don’t understand why for example, the thermodynamics of black holes.$\endgroup$

Thermodynamics is an older theory that has studied work, energy, temperature, entropy and related concepts as continuous quantities describing continuous material objects. It was based on several principles – such as the conservation of energy and the growth of entropy – that prevent one from constructing perpetuum mobile gadgets.

The point of contact between the two disciplines is taken to be the function for Entropy S which is a function of the thermodynamic variables $S=S$ etc, with U being internal energy, V volume. Sometimes this is inverted as: $U=U$

The Direction Of Time

We have noted that it was Boltzmann who first suggested that ourvery concept of the future direction of time was fixed by the directionin time in which entropy was increasing in our part of the universe.Numerous authors have followed up this suggestion and the entropictheory of time asymmetry remains a much debated topic in the philosophyof time.

We must first ask what the theory is really claiming. In a sensibleversion of the theory there is no claim being made to the effect thatwe find out the time order of events by checking the entropy of systemsand taking the later event as the one in which some system has itshigher entropy. The claim is, rather, that it is the facts about theentropic asymmetry of systems in time that ground the phenomena thatwe usually think of as marking out the asymmetrical nature of timeitself.

The entropic theory in its most plausible formulation is a claim tothe effect that we can explain the origin of all of these intuitiveasymmetries by referring to fact about the entropic asymmetry of theworld.

Similarly the entropic theorist claims that it is the entropicfeatures that explain the intuitive asymmetries noted above, that inregions of the universe in which the entropic asymmetry wascounter-directed in time the past-future directions of time would beopposite, and that in a region of the universe without an entropicasymmetry neither direction of time would count as past or asfuture.

Read Also: Beth Thomas Parents

Entropy Of A Perfect Gas

Let us consider 1 g-mole of a perfect gas occupying a volume V at a pressure P and temperature T. Let a quantity of heat dQ be given to the gas, then by the first law of thermodynamics, we have

ddd

Now if Cv is the specific heat of the gas at constant volume, dT is the rise in temperature, dV is the change in volume then

dddddddentropy

What Is Statistical Mechanics

Statistical mechanics is the branch of the theory of physics, studying the use of probability theory and also the average or mean behavior of a mechanical system wherein the state of the system is said to be uncertain. The common use of this machine is in the thermodynamic accomplishment of large systems. The statistical thermodynamics or statistical mechanics are the branches of statistical mechanics.

The advantage of using these mechanics is to provide exact methods of connecting the thermodynamic quantities to the microscopic behavior. The statistical form of mechanics also makes possible in extending the laws of thermodynamics with few or some degrees of freedom.

Don’t Miss: Grade 6 Fsa Warm Ups Answer Key

What Is A Good Introductory Statistical And Quantum Mechanics Book Which Can Be Self Studied

• Quantum

149

23

Can you please suggest a good introductory statistical and quantum mechanics book which can be self studied.My math background :I’ve done multivariate calculus, vector calculus, linear algebra ,some complex analysis all at the usual undergraduate level.The books I’ve self studied thus far are:1) Mechanics : Kleppner and Kolenkow2) Electrodynamics : Griffiths4) Classical mechanics : Goldstein -) Quantum mechanics :Griffiths As you can see I’m lacking in quantum mechanics and statistical mechanics, so with my current background could you please suggest book on quantum mechanics and statistical mechanic which are easier to self study.Thank you.

Can you please suggest a good introductory statistical and quantum mechanics book which can be self studied.My math background :I’ve done multivariate calculus, vector calculus, linear algebra ,some complex analysis all at the usual undergraduate level.The books I’ve self studied thus far are:1) Mechanics : Kleppner and Kolenkow2) Electrodynamics : Griffiths4) Classical mechanics : Goldstein -) Quantum mechanics :Griffiths As you can see I’m lacking in quantum mechanics and statistical mechanics, so with my current background could you please suggest book on quantum mechanics and statistical mechanic which are easier to self study.Thank you.

Introduction To Statistical Physics

Statistical physics is the plot of Physics. It has educated us with countless modules in the universe and will demonstrate us further.

Statistical physics aims at learning the macroscopic factors of a system in equilibrium from the microscopic properties information through the law of mechanics. This method is not the same from thermodynamics that diagnoses the macroscopic system in equilibrium from the macroscopic position except seeing the microscopic parameters.

Statistical division is the division of statistical physics where a system is set up to determine free energy. In statistical physics, we practice the point that material contains atoms. On the basis of information of the microscopic laws that manage the atoms motion, and predominantly a surplus law of statistical physics.

Thus, it provides an overall expression for the free energy.

Statistical physics can help you to learn both thermal equilibrium states as well as non-equilibrium states.

The above figure shows a molecular solid in which the individual particles are confined at specific lattice places and have no center of mass in motion.

Read Also: Who Are Paris Jackson’s Biological Parents

Philosophers On Probability And Statistical Explanation

Philosophers concerned with the interpretation of probability areusually dealing with the following problem: Probability ischaracterized by a number of formal rules, the additivity ofprobabilities for disjoint sets of possibilities being the most centralof these. But what ought we to take the formal theory to be a theoryof? Some interpretations are objectivist, taking probabilities to be,possibly, frequencies of outcomes, or idealized limits of suchfrequencies or perhaps measures of dispositions or propensities ofoutcomes in specified test situations.

Other interpretations are subjectivist, taking probabilities to bemeasures of degrees of belief, perhaps evidenced in behavior insituations of risk by choices of available lotteries over outcomes.Still another interpretation reads probabilities as measures of a kindof partial logical entailment among propositions.

Although subjectivist interpretations ofprobability in statistical mechanics have been proffered , most interpreters of the theory opt for an objectivistinterpretation of probability. This still leaves open, however,important questions about just what objective feature the positedprobabilities of the theory are and how nature contrives to have suchprobabilities evinced in its behavior.

Application Of Statistical Physics

These are some statistical physics applications written below:

• The principal statistical physics application was focused on the dissemination of molecules in a gathering. It was functional in Maxwells distribution of molecular velocity.

• Gibb enlightened the thermodynamics in virtue of statistical physics.

Recommended Reading: Who Is Paris Jackson’s Biological Father

Statistical Mechanics Built On Sand

• School of Mathematical Sciences, Queen Mary University of London, London, United Kingdom

PhysicsFigure 1:

Beach sand, sugar, and coffee powder are just three examples of lifes ubiquitous granular materials, which are materials that are composed of small particles or grains. Despite this ubiquity, a fundamental understanding of the properties of these materials has, so far, remained elusive. Now, Yujie Wang at Shanghai Jiao Tong University, China, and collaborators take a step toward developing that understanding by performing experiments that validate a statistical mechanics framework for granular systems . Their results could help researchers in determining novel methods for predicting the properties of granular materials.

In equilibrium statistical mechanics, the central observable is the systems total energy. This energy relates to both the entropy of the system and the probability that the system sits in a configuration with a particular energy. For granular systems, however, the total energy is either unconserved or irrelevant , and thus energy is unsuitable as a state variable.

On A Statistical Mechanics Approach To Some Problems Of The Social Sciences

• 1Dipartimento di Matematica, Università di Bologna, Bologna, Italy
• 2Dipartimento di Scienze Fisiche, Informatiche e Matematiche, Università di Modena e Reggio E., Modena, Italy

This work is a survey of some results on a statistical mechanics approach to the social sciences emerged in the last two decades. The pioneering work of Daniel McFadden, known as discrete choice theory, is interpreted in terms of a non-interacting model and extended along the lines of the Brock and Durlauf interacting systems. The generalization to the multi-populated model is presented and two specific case studies are reviewed with their phenomenological and theoretical analysis.

Read Also: How To Avoid Parallax Error In Physics

Iiiaisomorphism Between Information Theory And Statistical Mechanics

Consider a system that can be described in terms of a set of events. We say that an event occurs when some variable used to characterize the event assumes some value. Generally, we lack sufficient information to specify the exact probability distribution of events. Rather, we possess only a limited amount of information, such as the average value of the variable characterizing the events. Nonetheless, it is desirable to make use of our limited information to make educated guesses about the probability of an event and to make estimates of the properties of our system. This is the basic problem confronting us in statistical mechanics.

The problem of determining the probability distribution of events requires us to find a least-bias distribution that agrees with the given information about a system. The resolution of this problem underwent a great advance with the advent of Shannon”s information theory. Shannon showed that there exists a unique measure of the statistical uncertainty in a discrete probability distribution , which is in agreement with the intuitive notion that a broad distribution represents more uncertainty than a narrow distribution. This measure is called the missing information, which is defined by

where is a normalized probability density. As for discrete distributions, the missing information is nonnegative and additive for independent sources of uncertainty.

Richard Smith, … Cor Peters, in, 2013

What Is Classical Mechanics In Physics

Classical mechanics is a theory of Physics that takes into aspect the motion of macroscopic objects , starting from projectiles to different parts of machinery, and astronomical objects, such as spacecraft, stars, planets, and galaxies.

For objects that are governed by classical mechanics, if the present state is known, we can predict how an object will move in the future and how it has moved in the past .

Don’t Miss: My Hrw Algebra 2

Where Does A Math Person Go To Learn Statistical Mechanics

The more math I read, the more I see concepts from statistical mechanics popping up — all over the place in combinatorics and dynamical systems, but also in geometric situations. So naturally I’ve been trying to get a grasp on statistical mechanics for a while, but I haven’t been very successful. I’ve skimmed through a couple of textbooks, but they tended to be heavy on the physical consequences and light on the mathematical underpinnings

I suspect that part of the problem is that, unlike the analogous situation with quantum mechanics, I’m not sure what mathematics I can fall back on if I don’t “get” some statistical model. So, is there a good resource for statistical mechanics for the mathematically-minded?

• 6Nov 5 ’09 at 0:49
• 4$\begingroup$I’ve had that problem with physics books as well. Idk why they’re so… unmathematical… and even not great at helping people get an intuition for the subject!!$\endgroup$ Michael HoffmanNov 5 ’09 at 1:24
• $\begingroup$There are good ones – even great ones – out there. You just have to look hard for them . One of the problems is that it is very difficult to strike the right balance between mathematical rigor and conceptual understanding, particularly when writing.$\endgroup$Feb 19 ’10 at 21:05
• 2$\begingroup$I would recommend ams.org/bookstore-getitem/item=ULECT-19 for a start.$\endgroup$ user39719

So, is there a good resource for statistical mechanics for the mathematically-minded?

Some examples:

What Is Mechanics

Mechanics is a science that deals with the motion of objects under the effect of force. It also deals with the special case when the body stays at rest.

Here, our foremost concern is with the two bodies that exert forces on each other.

For example, the effect of gravity on the planets revolving around the sun, magnetic forces by which iron filings get attracted to the magnet and the electric force under which the two charges get attracted towards each other, and so on.

Also Check: Eoc Fsa Warm Ups Algebra 1 Answers

What Is Entropy

Entropy of a system is a function of the thermodynamical coordinates defining the state of the systems viz, the pressure, temperature, volume or internal energy and its change between two states is equal to the integral of the quantity the dQ/T between the states along any reversible path joining them.

Physically the entropy of a system is a measure of disorder of its molecular motion. Greater is disorder of molecular motion of the system greater is the entropy. The change in entropy passing from one state A to another state B is given by

Where dQ is the quantity of heat absorbed or ejected at a temperature T in going from state A to state B. We also note that :