What Is The Definition Of Term In Math

What Is A Fraction

Fractions represent the parts of a whole or collection of objects. A fraction has two parts. The number on the top of the line is called the numerator. It tells how many equal parts of the whole or collection are taken. The number below the line is called the denominator. It shows the total number of equal parts the whole is divided into or the total number of the same objects in a collection.

Properties Of A Math Definition

The four rules below are absolute requirements that all mathematical definitions obey:

• LP: The definition consists of a list of some of the properties of the concept .
• EAP: Any example of the concept must have all the properties listed in the definition .
• OAP: Every math object that has all the properties listed in the definition is an example of the concept.
• COMP: Every correct statement about the concept follows logically from its definition.

Math definitions have other properties as well. The list below describes aspects of definitions that people new to abstract math don’t always understand:

• FP: The definition gives a fewproperties that are enough to determine the concept.
• ME: Much else may be known about the concept besides what is in the definition.
• NI: The info in the definitionmay not be the most important things to know about the concept.
• DL: The same concept can have verydifferent-looking definitions. In some cases, it is difficult to prove they give the same concept.
• SW: Mathtexts use special wording to give definitions. Newcomersmay not understand that the intent of an assertion isthat it is a definition.
• CW: The word or phrase being defined may involve a word that already exists, but the connotations of that word are worthless when you are proving something about the concept that the definition defines.

What Is An Algebraic Expression

An algebraic expression consists of unknown variables, numbers, and arithmetic operators.

x 5 is a simple algebraic expression. When we combine constants and variables connected by mathematical operations such as +, -, x, and ÷, we get an algebraic expression.

Let us look at a few more algebraic expressions.

A coefficient is a number multiplied by the variable.The coefficient of 5 x + 10 is 5 and

8 2 x is 2.

Don’t Miss: What Is Psychological Foundation Of Education

What Is An Expression In Math

An expression in math is a sentence with a minimum of two numbers/variables and at least one math operation in it. Let us understand how to write expressions. A number is 6 more than half the other number, and the other number is x. This statement is written as x/2 + 6 in a mathematical expression. Mathematical expressions are used to solve complicated puzzles.

Look Up The Meaning Of Math Words

• Ph.D., Biomedical Sciences, University of Tennessee at Knoxville
• B.A., Physics and Mathematics, Hastings College

This is a glossary of common mathematical terms used in arithmetic, geometry, algebra, and statistics.

Abacus: An early counting tool used for basic arithmetic.

Absolute Value: Always a positive number, absolute value refers to the distance of a number from 0.

Acute Angle: An angle whose measure is between 0° and 90° or with less than 90° radians.

Addend: A number involved in an addition problem numbers being added are called addends.

Algebra: The branch of mathematics that substitutes letters for numbers to solve for unknown values.

Algorithm: A procedure or set of steps used to solve a mathematical computation.

Angle: Two rays sharing the same endpoint .

Angle Bisector: The line dividing an angle into two equal angles.

Area: The two-dimensional space taken up by an object or shape, given in square units.

Array: A set of numbers or objects that follow a specific pattern.

Attribute: A characteristic or feature of an objectsuch as size, shape, color, etc.that allows it to be grouped.

Average: The average is the same as the mean. Add up a series of numbers and divide the sum by the total number of values to find the average.

Base: The bottom of a shape or three-dimensional object, what an object rests on.

Base 10: Number system that assigns place value to numbers.

Capacity: The volume of substance that a container will hold.

Recommended Reading: What Were The Psychological Effects Of Ww1

Metaphors: Typical Trivial And Monstrous

Typical

There is a sense in which a robin is a typical bird and a penguin is not a typical bird. The Wiktionary definition of “bird” says they are “characterized by being warm-blooded, having feathers and wings usually capable of flight, and laying eggs.” The word “usually” kills the possibility that that is a mathematical definition, but it allows us to say penguins are not typical birds. That is completely appropriate for definitions in biology, but does not work for math definitions.

A mathematical definition is simply a list of properties in the very strict sense of LP, EAP and OP. If an object has allthe properties, it is an example of the definition. If it doesnt, it is notan example. So in some basic sense no example is any more typical than anyother. This is in the sense of rigorous thinking described in the chapter on imagesand metaphors.

Now I contradict myself?

In spite of what I said in the previous paragraph, mathematicians talk about typical examples, trivial examples, monstrous examples and so on all the time!

The point is that these attitudes are based on intuition. Expressing an attitude about an example does not mean that it fits the definition “better” or “worse” than some other example.

Example: Continuous curves

Example: Finite groups

It may sound really peculiar to a non-mathematician when you say that the monster group is a simple group.

Intuition and rigor

Rigor and intuitionto understanding math.

Every Proof Originates Solely In The Definition

The special logical status of a definition is the reason that rewritingaccording to the definitions is a reasonable first step in comingup with a proof.

Here are some seemingly contradictory points about the purple prose above:

You generally have to use other theorems to prove a statement about the concept

The proof of any except the most elementary theorem about a concept will use other theorems about the concept. For example, perhaps the definition of the concept implies Theorem A and Theorem A implies Theorem B. Since implication is transitive, this means that the definition implies Theorem B.

The definition is not the only source of understanding the concept

Many facts about a concept can help in giving you an intuition about it, because the info that is inthe definition may not include the most important aspects of the concept. This is about understanding and is separate from the fact that the theorems can be used in the proof, as mentioned in the preceding point.

Example

Suppose you run across a line in a math text that says, “Because $\sqrt\lt1.5$…”. The definition of $\sqrt$ does not give its value, but the fact that it is approximately “$1.414$” tells you it is less that $1.5$. .

The definition must be taken literally.

The notation and terminology used may suggest propertiesthe definition does not actually require.

Example: Subset

Recommended Reading: What Is Stroma In Biology

What Is Term In Math And How Does It Works

Are you unable to solve algebraic problems? Or do you get stuck while solving the equation? Well, this might be because you are unable to identify like and unlike terms.

Excuse me!!! I do not have any idea regarding what is term in math?

Do not worry! I have explained all the details that will help you clear your doubts regarding terms in math and allow you to know how to analyze terms in algebraic equations.

But before that, let me explain to you what are the key components of an algebraic equation.

Complete Mathematical Terms Dictionary

Understanding math concepts is critical in our world today. Math is used daily by nearly everyone, from lay persons to highly degreed professionals. Situations in which math is used vary from simply balancing a checkbook or calculating the amount of change due from a store transaction all the way to making blueprints for an office building or house and the construction of those buildings. Understanding how to solve math problems becomes easier as one learns math terminology. Below is a list of many common math terms and their definitions.

Acute angle An angle which measures below 90°.

Acute triangle A triangle containing only acute angles.

Additive inverse The opposite of a number or its negative. A number plus its additive inverse equals 0.

Adjacent angles Angles with a common side and vertex.

Angle Created by two rays and containing an endpoint in common.

Arc A set of points that lie on a circle and that are positioned within a central angle.

Area The space contained within a shape.

Average The numerical result of dividing the sum of two or more quantities by the number of quantities.

Binomial An expression in algebra that consists of two terms.

Bisect To divide into two equal sections.

Canceling In multiplication of fractions, when one number is divided into both a numerator and a denominator.

Cartesian coordinates Ordered number pairs that are assigned to points on a plane.

Chord A line segment that connects two points on a circle.

Don’t Miss: What Is Input And Output In Math

What Is Meant By Constant

A constant is a numerical value that should not change its value. For example, in the expression 2x+6, 6 is a constant.

Put your understanding of this concept to test by answering a few MCQs. Click Start Quiz to begin!

Select the correct answer and click on the Finish buttonCheck your score and answers at the end of the quiz

What Is Division In Math

Division is the opposite of multiplication. If 3 groups of 4 make 12 in multiplication, 12 divided into 3 equal groups give 4 in each group in division.

The main goal of dividing is to see how many equal groups are formed or how many are in each group when sharing fairly.

In the above example, to divide 12 donuts into 3 similar groups, you will have to put 4 donuts in each group. So, 12 divided by 3 will give the result 4.

Read Also: What’s The 5 Themes Of Geography

What Are The Factors Of A Term

• The numbers or variables that are multiplied to form a term are called its factors. For example, 5xy is a term with factors 5, x and y.
• The factors cannot be further factorized. For example, 5xy cannot be written as the product of factors 5 and xy. This is because xy can be factorized to x and y.
• The factors of the term 3a4 are 3, a, a, a and a.
• 1 is not taken as a separate factor.

Term Structure Vs Representation

Originally, logicians defined a term to be a character string adhering to certain building rules. However, since the concept of tree became popular in computer science, it turned out to be more convenient to think of a term as a tree. For example, several distinct character strings, like “)/2”, “))/2″, and ” n 2 }} “, denote the same term and correspond to the same tree, viz. the left tree in the above picture.Separating the tree structure of a term from its graphical representation on paper, it is also easy to account for parentheses and invisible multiplication operators .

Recommended Reading: What Is Conceptual Understanding In Math

What Is A Coefficient In An Expression

A coefficient is an integer that is written along with a variable or it is multiplied by the variable. In other words, a coefficient is the numerical factor of a term containing constant and variables. For example, in the term 2x, 2 is the coefficient.

The variables which do not carry any number along with them, have a coefficient of 1. For example, the term y has a coefficient of 1. For example,in the expression 5ab, 5 is the coefficient.

More examples on Coefficients:

• -5 is the coefficient of the term 5ab2.
• When there is no numerical factor in a term, its coefficient is taken as +1. For example, in the term x2y3, the coefficient is +1.
• In the term x, the coefficient is -1.

However, all these parts of an algebraic expression are connected with each other by arithmetic operations such as addition, subtraction, or multiplication in general. Thus, these operators play a significant role in forming expressions in algebra. Even the single term can be expressed as a sum of two terms. For example, 5x can also be written as 5x + 0 or 5x 0. But this way of representation is not actually required except to define the expression as it is a combination of all those elements .

Expression In Math Example

There are infinite examples of an expression. For example, 2y-9, 3a×2, -7+6÷3, etc. Let us also look at a real-life example. Sarah told her younger brother Daniel that her age is 3 more than twice his age. She asked him to calculate her age if his age is x years. Let’s help him write an expression. Twice the age of Daniel can be written as 2x. Now Sarah’s age is 3 more than 2x. Therefore, Sarah’s age will be written as 2x+3.

Also Check: What Does Infrastructure Mean In Geography

Building Formulas From Terms

Given a set Rn of n-ary relation symbols for each natural number n 1, an atomic formula is obtained by applying an n-ary relation symbol to n terms. As for function symbols, a relation symbol set Rn is usually non-empty only for small n. In mathematical logic, more complex formulas are built from atomic formulas using logical connectives and quantifiers. For example, letting R denote the set of real numbers, x: x R } 0 is a mathematical formula evaluating to true in the algebra of complex numbers.An atomic formula is called ground if it is built entirely from ground terms all ground atomic formulas composable from a given set of function and predicate symbols make up the Herbrand base for these symbol sets.

Can We Solve Like And Unlike Terms If Yes Then How

Yes, you can solve like and unlike terms.

Moreover, it is quite easy to solve the like and unlike terms. But before solving them, you must remember what is term in math. This will help you to solve terms with ease.

So, lets check how to analyze terms in algebraic equations.

Suppose you and your other 10 friends went to a restaurant to eat some snacks. And all of you gave the order as :

• 1 ordered: Hamburger

You can see three similar or like snacks: Hamburger, French Fries, and Soft drinks. Now, you can separate them as like and unlike terms.

The unlike terms are Hamburger, French Fries, and Soft drinks.

The equation can be written as:

=> 2h+f+d+3h+2f+2d

Simplify it as:

This is what is term in math, and how does it get solved?

Don’t Miss: What Is Displacement In Physics Formula

Ground And Linear Terms

The set of variables of a term t is denoted by vars.A term that doesn’t contain any variables is called a ground term a term that doesn’t contain multiple occurrences of a variable is called a linear term.For example, 2+2 is a ground term and hence also a linear term, x is a linear term, n is a non-linear term. These properties are important in, for example, term rewriting.

Given a signature for the function symbols, the set of all terms forms the freeterm algebra. The set of all ground terms forms the initial term algebra.

Abbreviating the number of constants as f0, and the number of i-ary function symbols as fi, the number h of distinct ground terms of a height up to h can be computed by the following recursion formula:

• 0 = f0, since a ground term of height 0 can only be a constant,
• i =\sum _^f_\cdot \theta _^} , since a ground term of height up to h+1 can be obtained by composing any i ground terms of height up to h, using an i-ary root function symbol. The sum has a finite value if there is only a finite number of constants and function symbols, which is usually the case.

How To Simplify Expressions In Math

We can simplify expressions in math by reducing the given expression in the simplest form. If it is a numerical expression, then it can be simplified by finding the value of the expression. If it is an algebraic expression, then it can be simplified by reducing it to the simplest form such that it cannot further be reduced.

Recommended Reading: What Does The Difference In Math Mean

Different Terms In Algebra

There are two kinds of terms in algebra: Like Terms and Unlike Terms.

Like Terms: Like terms are terms whose variables and exponent power are the same. They can be simplified by combining them. The operations of addition and subtraction can be performed on them together.

For example, 5x + 8x is an algebraic expression with like terms.

Unlike Terms: Unlike terms are those terms whose variables and their exponents are different from each other. They cannot be simplified by combining them. The operations of addition and subtraction cannot be performed on them together.

For example, 5x + 8y is an algebraic expression with unlike terms.

Let Us Learn about Polynomials

Polynomial comprises two Greek words: the word poly means many and nominal means terms. So, we get the phrase many terms. Polynomials are classified into three different types based on the number of terms it consists of.

The three types of polynomials are:

Expression: Term Factor And Coefficient

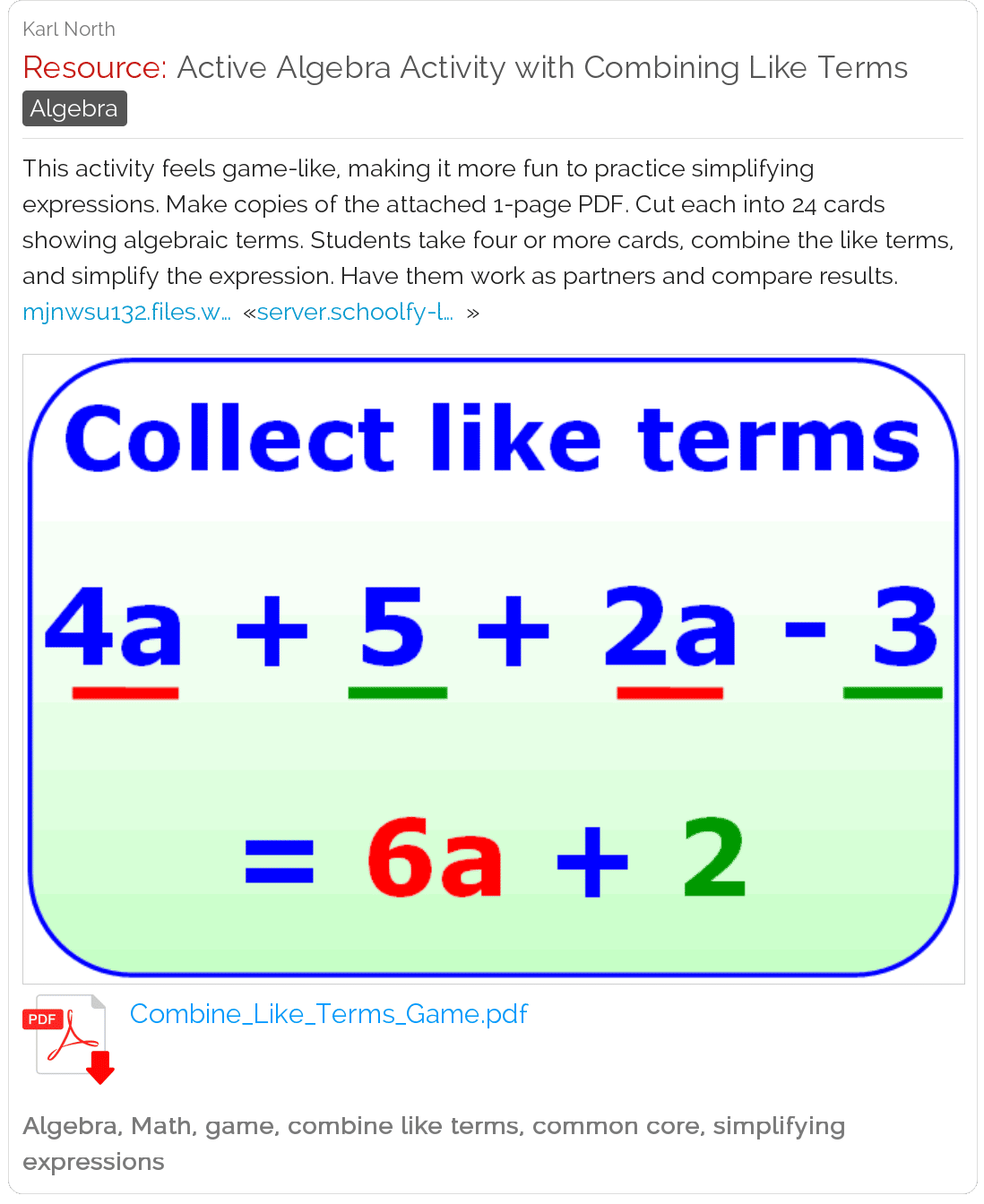
An algebraic expression is a mathematical phrase that contains integral or fractional constants , variables and algebraic operators operating on them. These expressions are expressed in the form of terms, factors and coefficients. a + 1, a + b, x2 + y, 5x/2, etc. are few examples of the algebraic expressions. The algebraic expressions are readily used as a number of mathematical formulas and find usage in generalizing them. In this article, we are going to discuss the definition of terms, factors, variables, what is a coefficient in Maths with many examples.

You May Like: What Does Ground State Mean In Chemistry