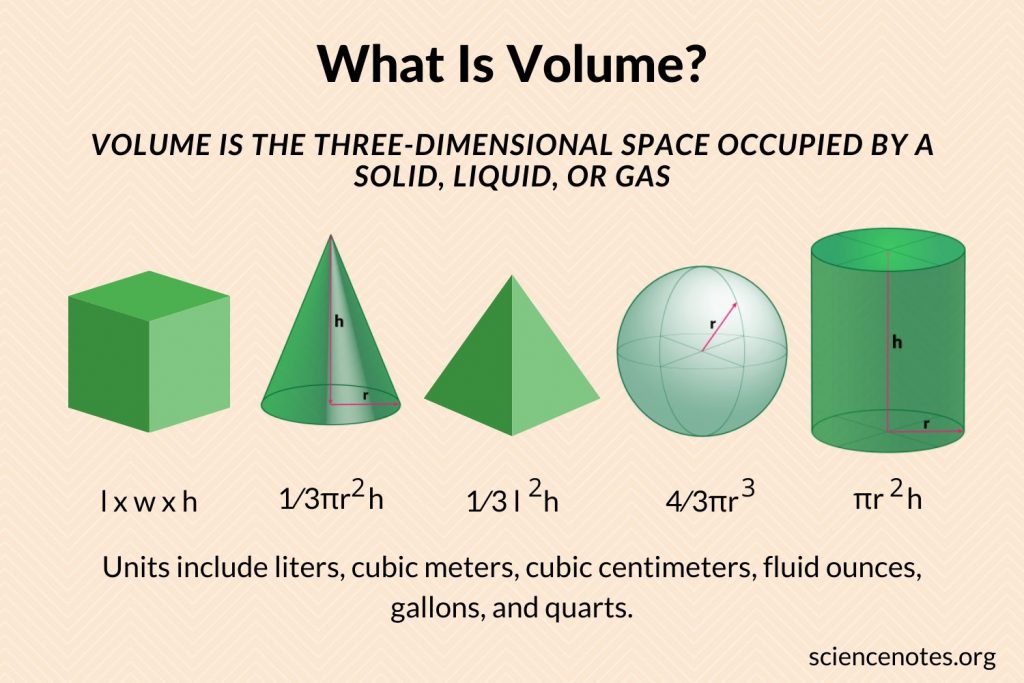
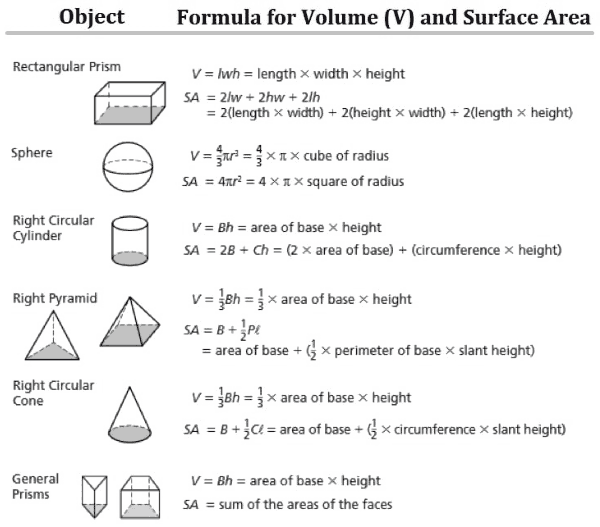
Choose The Right Synonym For Volume
Noun
bulk, mass, volume mean the aggregate that forms a body or unit. bulk implies an aggregate that is impressively large, heavy, or numerous. the darkened bulk of the skyscrapers mass suggests an aggregate made by piling together things of the same kind. a mass of boulders volume applies to an aggregate without shape or outline and capable of flowing or fluctuating. a tremendous volume of water
scaling Versus Dimensions Versus Units
Scaling arguments are more powerful that dimensional analysis.See reference& #XA0 9. See also section& #XA0 9.3and section& #XA0 1.7.2.
Dimensions are not the same as units. See reference& #XA0 10. There is a special class of problems where thetask is simply to convert the units, e.g. converting gallons to cubicinches. For these problems, there is a cut-and-dried algorithm & #X2014 thefactor label method & #X2014 that works fine.
| There is also a class of problems that involve a simpleproportionality with no fudge factors, for instance distance/time/rateproblems, Ohm& #X2019 s law problems, mass/volume/density problems, et cetera.For these problems, getting the units right pretty much guarantees acomplete solution. | This stands in contrast to other problems wherethere is a nontrivial factor out front, perhaps a factor of 4& #X3C0 or whatever. Dimensional analysis will never suss outthe correct factor. |
Densest Materials On The Earth
Since nucleons make up most of the mass of ordinary atoms, the density of normal matter tends to be limited by how closely we can pack these nucleons and depends on the internal atomic structure of a substance. The densest material found on earth is the metal osmium, but its density pales by comparison to the densities of exotic astronomical objects such as white dwarf stars and neutron stars.
List of densest materials:
It must be noted, plutonium is a man-made isotope and is created from uranium in nuclear reactors. But, In fact, scientists have found trace amounts of naturally-occurring plutonium.
If we include man made elements, the densest so far is Hassium. Hassium is a chemical element with symbol Hs and atomic number 108. It is a synthetic element and radioactive. The most stable known isotope, 269Hs, has a half-life of approximately 9.7 seconds. It has an estimated density of 40.7 x 103 kg/m3. The density of Hassium results from its high atomic weight and from the significant decrease in ionic radii of the elements in the lanthanide series, known as lanthanide and actinide contraction.
Also Check: Find The Message Pre Algebra With Pizzazz
University Physics Volume 3
Jeff Sanny, Loyola Marymount University
Samuel Ling, Truman State University
Copyright Year:2016
Reviewed by Thomas Burton, Assistant Professor, Massachusetts College of Liberal Arts on 5/24/21
The subjects and the depth to which they are covered is superb for most undergraduate classes and contexts. However, it may be insufficient for classes wholly dedicated to either optics or modern physics, or those wanting a deeply mathematical…read more
Reviewed by Thomas Burton, Assistant Professor, Massachusetts College of Liberal Arts on 5/24/21
Comprehensiveness rating:4 see less
The subjects and the depth to which they are covered is superb for most undergraduate classes and contexts. However, it may be insufficient for classes wholly dedicated to either optics or modern physics, or those wanting a deeply mathematical treatment of these subjects. The textbook’s glossary and index are thorough, greatly aid in navigating the material.
Content Accuracy rating:5
Throughout a semester of mostly teaching out of this textbook, my students and I were unable to find any significant errors or biased statements.
Relevance/Longevity rating:5
Myself and my students found the examples of many phenomena to be very relevant, as they were those we had seen or experienced in their day-to day lives. Often the textbook is updated with corrections or minor edits.
Clarity rating:5
Consistency rating:5
Modularity rating:4
Organization/Structure/Flow rating:5
Difference Between Mass And Volume
Mass and volumes are the two components used to measure the objects. Quantification is the procedure of unearthing the quantity of an entity based on its weight, mass, length, and volume. Units used to measure an object in kilograms , Meter , seconds, and Kelvin. However, a few of us tend to get confused between the differences between Mass and Volume. This article is a ready reckoner guide to explain the differences between Mass and Volume.
Read Also: Michael Jackson Kids Biological
Si Prefixes Applied To The Litre
The litre, though not an official SI unit, may be used with . The most commonly used derived unit is the millilitre, defined as one-thousandth of a litre, and also often referred to by the SI derived unit name “cubic centimetre”. It is a commonly used measure, especially in medicine, cooking and automotive engineering. Other units may be found in the table below, where the more often used terms are in bold. However, some authorities advise against some of them for example, in the United States, advocates using the millilitre or litre instead of the centilitre. There are two international standard symbols for the litre: L and l. In the United States the former is preferred because of the risk that the letter l and the 1 may be confused.
| Multiple |
|---|
Control Volume Control Volume Analysis
A control volume is a fixed region in space chosen for the thermodynamic study of mass and energy balances for flowing systems. The boundary of the control volume may be a real or imaginary envelope. The control surface is the boundary of the control volume.
A control volume analysis can be used for example to determine the rate of change of momentum for a fluid. In this analysis, we will consider a streamtube as we did for the Bernoulli equation. In this control volume any change in momentum of the fluid within a control volume is due to the action of external forces on the fluid within the volume.
See also: Momentum Formula
As can be seen from the picture the control volume method can be used to analyze the law of conservation of momentum in fluid. Control volume is an imaginary surface enclosing a volume of interest. The control volume can be fixed or moving, and it can be rigid or deformable. In order to determine all forces acting on the surfaces of the control volume we have to solve the conservation laws in this control volume.
You May Like: Mcdougal Geometry Workbook Answers
Density Of Various Materials Examples
1000 kg/m33.98oC . less dense as it freezes ice floats
= m/V = 1/
The specific volume of a substance is the total volume of that substance divided by the total mass of that substance . It has units of cubic meter per kilogram .
heavy water11% greater than water
This difference is caused by the fact, the deuterium nucleus is twice as heavy as hydrogen nucleus. Since about 89% of the molecular weight of water comes from the single oxygen atom rather than the two hydrogen atoms, the weight of a heavy water molecule, is not substantially different from that of a normal water molecule. The molar mass of water is M = 18.02 and the molar mass of heavy water is M = 20.03 , therefore heavy water has a density about 11% greater .
Pure heavy water has its highest density 1110 kg/m3 at temperature 3.98oC . Also heavy water differs from most liquids in that it becomes less dense as it freezes. It has a maximum of density at 3.98 °C , whereas the density of its solid form ice is 1017 kg/m3. It must be noted, the change in density is not linear with temperature, because the volumetric thermal expansion coefficient for water is not constant over the temperature range.
well knowndensity
The density of any substance is the reciprocal of its specific volume .
= m/V = 1/
The specific volume of a substance is the total volume of that substance divided by the total mass of that substance . It has units of cubic meter per kilogram .
K Times Greater Than
The phrase & #X201C … k times greater than …& #X201D is so problematic thatit deserves detailed discussion.
Consider the following scenario: VA denotes the volume of objectA, while VB denotes the volume of object B. We are given thatVA = 12 liters and VB = 36 liters.
The first three of the following statements are goodterminology, but then things go to pot:
- 1.& #XA0 & #XA0 & #XA0 VB is three times as great as VA.
- 2.& #XA0 & #XA0 & #XA0 B has 3 times the volume of A.
- 3.& #XA0 & #XA0 & #XA0 VB is 300% of VA.
- 4.& #XA0 & #XA0 & #XA0 VB is 200% greater than VA.
- 5.& #XA0 & #XA0 & #XA0 VB is greater than VA by a factor of3.
- 6.& #XA0 & #XA0 & #XA0 VB is three times greater than VA.
- 7.& #XA0 & #XA0 & #XA0 VB is two times greater than VA.
- 8.& #XA0 & #XA0 & #XA0 B is three times as big as A.
A different problem crops up in statement 8. Does thestatement mean that every dimension of B is bigger by a factor of 3, or does it only mean that the volume isbigger by a factor of 3? When you are scaling some property, you needto be specific about which property you are scaling.
Read Also: Paris Jackson Real Father
volume Area And Length In Three Dimensions
We can extend this idea into three dimensions, as shown infigure& #XA0 7. When it comes to length, every length in thelarge cube is two times as great as the corresponding length in thesmall cube. When it comes to area, the surface area of the large cubeis four times as great as the surface area of the small cube. When itcomes to volume, the volume of the large cube is eight times as greatas the volume of the small cube.
The volume goes up by a factor of eight because the cube is twice aswide and twice as deep and twice as tall. That& #X2019 s three factors oftwo.
Meanwhile the surface area of the cube went up by a factor of four,not a factor of eight. That& #X2019 s because on each face of the cube, thesurface goes like width times height the surface does not have anythickness. Each face of the cube is locally two dimensional, even though ifyou put all six faces together the total surface extends across threedimensions. Reconciling these facts requires a sophisticated notionof dimensionality. We say that the surface is intrinsicallytwo-dimensional but is embedded in three dimensions.
The scaling laws for volume, area, and length can be expressed interms of equations:
and if you want to get fancy, this can be expressed in one bigequation
| & #XA0 =& #XA0 =& #XA0 1& #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 |
In equation& #XA0 3, if a quantity has extent in N spatialdimensions, we take the Nth root.
The Density Of A Container
The amount of fluid, gas, or liquid that the container can hold is considered to be the capacity it can hold, rather than the amount of space the container itself displaces. The density of the substance is similar to the property of viscosity. The more the fluid’s density, the more it is like honey, oil, etc.
You May Like: Cci4 Lewis Structure
The Volume Of An Atom And Nucleus
The atom consists of a small but massive nucleus surrounded by a cloud of rapidly moving electrons. The nucleus is composed of protons and neutrons. Typical nuclear radii are of the order 1014 m. Nuclear radii can be calculated according to the following formula assuming spherical shape:
r = r0 . A1/3
where r0 = 1.2 x 10-15 m = 1.2 fm
If we use this approximation, we, therefore, expect the volume of the nucleus to be of the order of 4/3r3 or 7,23 ×1045 m3 for hydrogen nuclei or 1721×1045 m3 for 238U nuclei. These are nuclei volumes, and atomic nuclei contain about 99.95% of the atoms mass.
Is An Atom An Empty Space
The volume of an atom is about 15 orders of magnitudelarger than the volume of a nucleus. For uranium atom, the Van der Waals radius is about 186 pm = 1.86 ×1010 m. The Van der Waals radius, rw, of an atom is the radius of an imaginary hard sphere representing the distance of closest approach for another atom. Assuming spherical shape, the uranium atom have volume of about 26.9 ×1030 m3. But this huge space is occupied primarily by electrons, because the nucleus occupies only about 1721×1045 m3 of space. These electrons together weigh only a fraction of entire atom.
It may seem, that the space and in fact the matter is empty, but it is not. Due to the quantum nature of electrons, the electrons are not point particles, they are smeared out over the whole atom. The classical description cannot be used to describe things on the atomic scale. On the atomic scale, physicists have found that quantum mechanics describes things very well on that scale. Particle locations in quantum mechanics are not at an exact position, they are described by a probability density function. Therefore the space in an atom is not empty, but it is filled by a probability density function of electrons .
Also Check: Unit 1 Geometry Basics Answer Key
What Is Specific Volume
Specific volume is an intensive variable, whereas volume is an extensive variable. The standard unit for specific volume in the SI system is cubic meters per kilogram . The standard unit in the English system is cubic feet per pound mass .
The density of a substance is the reciprocal of its specific volume .
= m/V = 1/
Density is defined as the mass per unit volume. It is also an intensive property, which is mathematically defined as mass divided by volume:
= m/V
nucleonsdensest materialmetal osmium dwarf starsneutron stars
List of densest materials:
It must be noted, plutonium is a man-made isotope and is created from uranium in nuclear reactors. But, In fact, scientists have found trace amounts of naturally-occurring plutonium.
The density of Hassium is followed by Meitnerium , which has an estimated density of 37.4 x 103 kg/m3.
densityspecific volumechangedpressuretemperaturepressure always increasesdensityliquidssolidscompressibilityCompressibility
extensive Variables And Eulers Thermodynamic Equation
Here& #X2019 s a fancy example. Suppose we have a thermodynamic system with acertain set of extensive variables, such as volume , entropy, and the amount of each chemical component . Also suppose the energy can be expressed as a function ofthose variables.
It is more elegant to lump all the extensive variables into a vectorX with components Xi for all i.
We introduce the general notion of homogeneous function asfollows: If we have a function with the property:
| E |
| & #X2202 & #XA0 E |
| & #X2202 & #XA0 Xi& #XA0 |& #XA0 Xj& #X2260 i |
There are conventional names for the partial derivatives on the LHS:temperature, pressure, and chemical potentials. Note that these areintensive quantities . Using these names, weget:
| T& #XA0 S& #XA0 & #X2212 & #XA0 P& #XA0 V& #XA0 +& #XA0 |
| & #X2211 |
| & #X3BD |
which is called Euler& #X2019 s thermodynamic equation. It is a consequence ofthe fact that the extensive variables are extensive. It imposes aconstraint, which means that not all of the variables are independent.
Note: Nothing is ever perfectly extensive. There arealways boundary terms that don& #X2019 t scale the same way as thebulk terms. However, for big-enough systems, the boundaryterms can be neglected, and this scaling analysis is anexcellent approximation.
If we take the exterior derivative of equation& #XA0 14 weobtain:
| S& #XA0 dT& #XA0 & #X2212 & #XA0 V& #XA0 dP& #XA0 +& #XA0 |
| & #X2211 |
| & #X3BD |
The Gibbs-Duhem equation has many other practical applications.
Also Check: Geometry Workbook Answers
example: Equilibrium And Activity
9.3.1& #XA0 & #XA0 Introduction
Let& #X2019 s consider the ultra-simple chemical reaction
| F2& #XA0 & #X2194 & #XA0 2F& #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 & #XA0 |
and in particular let& #X2019 s consider the equilibrium state in a vesselwhere that reaction is occurring in the gas phase. Let X denote thereaction coordinate, i.e. the degree to which the reaction hasproceeded toward the right. Specifically, X=0 if we have 100%& #X201C reactants& #X201D , and X=1 if we have 100%& #X201C products& #X201D .
We choose conditions of temperature and molar volume such that X isinitially small but nonzero. We hold the temperature constant, andincrease the system volume V by moving a piston. We predict thatincreasing the volume by a factor of Q increases X by a factor of& #X221A Q.
Let& #X2019 s work out the numbers for a simple scenario. We adopt theconvention that in this context , squarebrackets denote number density, i.e. the reciprocal of themolar volume. This is the convention used in introductory-levelchemistry courses and in many advanced, practical applications.
| & #X2014 & #X2014 & #XA0 A& #XA0 & #X2014 & #X2014 |
| & #X2192 |
| 2 |
| 2 |
| 2 |
As the final step, we allow the chemical reaction to come toequilibrium under the new conditions. The number density of unboundF atoms will increase.
Another thing you can see is that the ratio in the bottom row isthe same in both equilibrium situations. This leads us to define
| Kd& #XA0 :=& #XA0 |
Here are the main points:
| & #X2212 E |
| kT |