How To Reduce A Shape By A Scale Factor
Suppose you are given a figure and told to reduce it by 25 . Think in steps:
Suppose we have a rectangle that is 16 wide and we need to reduce it by 25
That means it will be 75
Now, we simplify our answer:
The width of our smaller new shape must be 12 . We repeat these steps with the other dimension, 6
The height of our smaller rectangle must be 4.5 .
Working With Paper Sizes
When working with ISO paper layouts we know that the standard size of paper was developed on the basis of an area of 1m2, divided according to the ratio of the sides.
This basic format of 1m2;then forms the basis for all other smaller sizes. All A sized paper is either halving or doubling the basic format.
X x Y = 1
Below is a list of all the A paper sizes.
Where Do We Use A Scale Ratio
A scale ratio;is a number by which the size of any geometrical figure or shape can be changed with respect to its original size. When things are too large, we use scale factors to calculate smaller, proportional measurements. It is used to compare two similar geometric figures.;Scale drawings are useful;for construction engineers and designers because they can be used to visualize;landscape plans before constructing a building on;the ground.
Recommended Reading: What Does Amu Mean In Chemistry
Finding Scale Factor Of Similar Figures
Here are two similar triangles. What is the scale factor used to create the second, larger figure?
Since we are scaling up, we divide the larger number by the smaller number:
. To go from legs of 12 3 .
Now, let’s try to scale down. Here are two similar pentagons. What is the scale factor used to create the second, smaller figure?
Because we are scaling down, we divide corresponding side lengths :
. To get the second, smaller figure, we multiply 21 ; the figure on the right uses a scale factor of 1 h .
Let’s look at one more example and scale both up and down. Consider these two similar right triangles with labeled sides.
If we have the little right triangle above and want to scale it up to the larger triangle, we write this:
So every other linear measure is multiplied times 5 .
If we have the big right triangle and want to scale it down to make the smaller one, we write this:
So every other linear measure is multiplied times 1 5
Worked Example : Drawing Scaled Maps

Draw a scaled map of a room that has real dimensions \ \ by \ \. Use a number scale of \ : \.
The scale of \: \ means that \ unit on your drawing will represent \ units in real life so \ \ on your drawing will represent \ \ in real life.
-
The width of the room is \ \.
- Convert \ \ to cm:\ \ \ \ = \ \
- Use the scale to calculate the scaled width on the map:\ \ \ \ \ = \ \
The length of the room is \ \.
The scaled measurements are \ \ and \ \. We can now draw this on our plan. Don’t forget to include the scale on your map!
Don’t Miss: How To Get Answers On Delta Math
Paper Size Scales And Magnification
We can now look at amending paper size scales and magnification. There are times when you may have a drawing on an A4 piece of paper, that you need to scale up to an A3 piece of paper for example. Lets imagine you were needing to trace this drawing so would use a photocopier to scale the drawing up to the necessary size.;
Worked Example : Making A Scale Drawing
Draw a scale drawing of a room that has real dimensions of 300 cm by 450 cm. Use a scale of.
Step 1: Take note of the given scale and convert the width of the room.
\
Step 2: Take note of the given scale and convert the length of the room.
\
Step 3: Draw the scale drawing.
The scaled measurements are 6 cm by 9 cm. You can now draw the scale drawing.
It is possible that the solution shown above does not display correctly on your device. The accurate scale drawing should have the measurements as shown above.
Step 4: Remember to show the scale on the scale drawing.
The scale is
Using a scale of, calculate the scaled dimensions of the room and the furniture. Then draw a scale diagram of the room. Arrange the furniture in any sensible manner.
You May Like: How To Study For Ap Human Geography
How To Interpret Scale Drawing
A scale drawing can be interpreted very easily if the required information is given. Let us consider an example that shows how a scale is used to prepare the blueprint of a house and how the required dimensions are calculated and interpreted.
Example: Joe has used a scale of 1:100 for the blueprint of his new house. In the;floor plan, the dimensions of the master bedroom are represented as 5 units;by 3 units. What are the dimensions of the master bedroom in the real world?
Solution:
Step-1: The scale of 1:100 means that for every 100 units in a real-world, the house represents 1 unit on the blueprint drawing. In other words, 100;units;of the actual house = 1;unit on a blueprint drawing
Step 2: Using the scale of 1 :100, we will calculate the actual dimensions. The scale factor is 100, therefore, we will multiply the blueprint dimensions by 100.
Length of the master bedroom = 100 × 5 = 500;units; Width of the master bedroom = 100 × 3 = 300;units
Worked Example : Resizing And Accuracy
|
Diagram 1 |
|
|
Diagram 3 |
Diagram 4 |
Recommended Reading: What Is The Importance Of Biology In Our Daily Life
How To Find Scale Factor
To find the scale factor, you first decide which direction you are scaling:
| Scale Up | = |
| Scale Down | = |
The scale factor for scaling up is a ratio greater than 1 . The scale factor for scaling down is a ratio of less than 1 .
Once you know which way you are scaling, you compare corresponding sides using the correct basic equation. Compare the side length of the real object to the length of the corresponding side in the representation.
Preparing For Ways Of Thinking
Look for these types of responses to be shared during the class discussion:
- Students who use the scale more accurately, rather than rounding to multiples of the scale
- Students who use different methods to answer the question so that these can be compared during the class discussion
ELL: Define the term approximations in the context of the discussion. Allow ELLs to use the dictionary if they wish.
Don’t Miss: What Is An Experimental Study In Psychology
Commonly Used Scales For Blueprint Drawings
Blueprint drawings are typically drawn in;
- 1:20, 1:50 or 1:100
- 1/4″ or 1/8″ ;
scales.
- multiply the measurement on the drawing with the denominator
where the denominator is the number after the colon.
Example – Blueprint Drawing Scale 1:50
An actual length of 1 cm is measured on a 1:50 blueprint floor plan. The physical length can be calculated as
50 = 50 cm
Imperial Units – US
A 1/4″ scale means that each 1/4″ on the plan counts for 1′ of actual physical length.
To scale a blueprint in imperial units to actual feet
- multiply the measurement on the drawing with the denominator
where the denominator is the bottom number.
Example – Blueprint Drawing Scale 1/4″
An actual length is measured to 1-3/8″ on a 1/4″ blueprint floor plan. The physical length can be calculated as
4 = 4
= 5.5;feet
Floor Plans, General Arrangement
- 1:40
Block Plan, City Maps and larger
- 1:1000
- Basics – The SI-system, unit converters, physical constants, drawing scales and more
- Drawing Tools – 2D and 3D drawing tools
Introduction To Number And Bar Scales
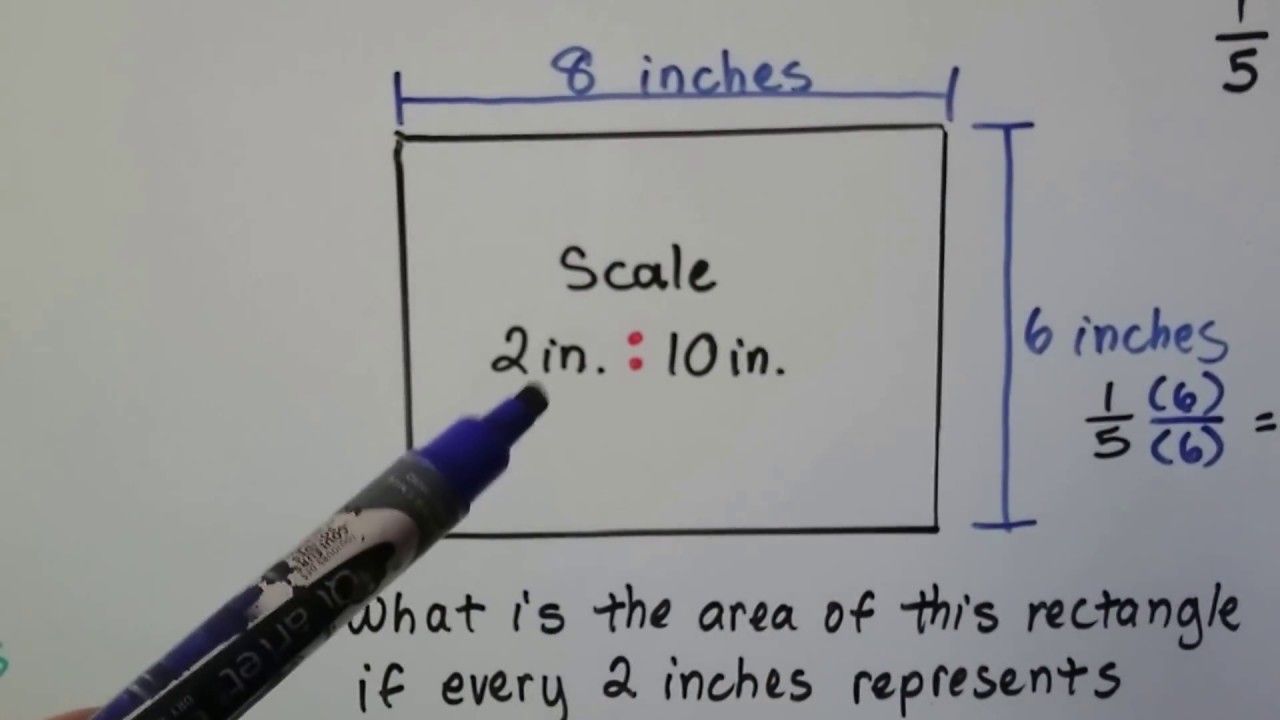
The two kinds of scale we will be working with in this chapter are the number scale and the bar scale. The number scale is expressed as a ratio like \ : \. This simply means that \ unit on the map represents \ units on the ground. So \ \ on the map will represent \ \ on the ground, or \ \ on the map will represent \ \ on the ground. To use the number scale, you need to measure a distance on a map using your ruler, and then multiply that measurement by the real part of the scale ratio ) given on the map, in order to get the real distance.
The bar scale is represented like this:
Each piece or segment of the bar represents a given distance, as labelled underneath. To use the bar scale, you need to measure how long one segment of the bar is on your ruler. You must then measure the distance on the map in centimetres; calculate how many segments of the bar graph it works out to be ; and then multiply it by the scale underneath. So, if \ \ on the bar represents \ \ on ground, and the distance you measure on the map is \ \ \ \ \ \ length of segment = \ segments) then the real distance on the ground is \ \ \ \ = \ \.
Read Also: What Does Fault Mean In Geography
Case Study _unit531 Example: In The Garden
Here is an example of typical scale drawing:
Whats the width and length of the patio?
Box _unit5.3.1
Hint: This scale drawing has been drawn on squared paper. This makes it easier to draw and understand. Each square is 1 cm wide and 1 cm long. So instead of using a ruler you can just count the squares and this will tell you the measurement in centimetres.
Worked Example : Drawing A Scaled Map
In this worked example we will add some furniture to the room in the previous example.
The room has the same dimensions \ \ \ \) and the scale to be used is still \ : \.
Draw the following items using the dimensions provided:
You may arrange the furniture in the room in any way which you think is sensible.
The scale of \: \ means that \ unit on your drawing will represent \ units in real life so \ \ on your drawing will represent \ \ in real life.
The scaled dimensions of the room are the same as in the previous worked example: \ \ \ \ \.
Read Also: What Is Activation Energy Biology
Activity _unit531 Activity : Getting Information From A Scale Drawing
What is the length of the longest side of the actual wild area in metres?
What will be the total actual width of the three disabled parking spaces in metres?
Worked Example : Converting A Scale Drawing To Real Measurements
The diagram below shows a scale drawing of a classroom. Study the scale drawing and answer the questions that follow.
The scale of this plan is given as. Use your ruler to measure the width and the length of the diagram of the classroom. Then calculate the real dimensions of the classroom.
It is possible that your device does not display the diagram correctly. The measurements given in the solution are the expected measurements.
Step 1: Measure the width of the classroom.
Use your ruler and measure the width, which is the shorter side of the diagram.
The classroom is 10.5 cm wide on the scale drawing.
Step 2: Use the given scale to calculate the width of the classroom in real life.
Step 3: Measure the length of the classroom.
Use your ruler and measure the length, which is the longer side, or the distance from the blackboard to the wall behind students,.
The classroom is 15 cm long on the scale drawing.
Step 4: Use the given scale to calculate the length of the classroom in real life.
So, in real life the classroom is 750 cm long and 525 cm wide.
Remember that there are 100 cm in a metre, so 100 cm = 1 m.
You May Like: What Does Coordinate Mean In Math
Working With Scales For Architectural Representation
In architecture, we use a collection of standard scales to represent our designs. For example, it is common practice to produce floor plans at a scale of 1:100 . Once you gain an understanding of scales, it is easy to understand which scale is most suited to which type of drawing.;
Scale bar blocks courtesy of cad-blocks.co.uk.
These scale bars show what one unit represents at different scales.
The general requirement of a scaled drawing is to convey the relevant information clearly with the required level of detail. If you are working in practice there will often be office standards. For example, they may only use layout sheets of either A3 or A1 depending on the scale of the project and information that is being represented. As a student, you need to make these decisions based on industry standard. It is always best to use a round scale, i.e., one of the scales mentioned below, and not make up your own.
What Is A Scale In Math
A scale in mathematics refers to the ratio of a drawing in comparison to the size of the real object. A ratio is a relative size that represents typically two values. For example, 1:3 pears and grapefruits represents that there is one pear for every three grapefruit.
In using scale, the ratio represents sizes of actual drawings or models. If the scale is 1:10, then the model or drawing is 10 times smaller than the actual object. If a die-cast car is listed as a 1:10 or 1/10 diecast, then the actual car is 10 times larger than the model car.
Scale is often used to represent items like diecast cars, maps and other items. A real horse may be 1,500 mm high, but the drawing of the horse may be 150 mm high. As with the diecast car, this scale is represented by writing the ratio 1:10.
Using scale drawings can also help with representing buildings. Architects often use scale when drawing a design, or to build models to show the design to others. A doll house is a good example of something that can be represented in scale. If a doll house modeled after a real house is 50 times smaller, then that scale can be represented by writing the ratio 1:50.
Recommended Reading: Geometry Dash Practice Song Hack