What Does Pictorial Mean In Math

How To Make A Pictograph

Let us take an example. We will represent the average hours spent by students on different activities.

The following table gives this data:

The below-given steps can be followed to represent the data given in the table in the form of a pictograph.

• Collect Data: Firstly, we need to collect the data of the different categories we want to represent. We then form a table or list. In the above example, the data is already provided in the form of a table.
• Review the data and Pick a symbol: Review the collected data and based on it pick a symbol or picture that accurately represents the data. For drawing a pictograph to represent the number of hours spent on a particular activity, we can use an hourglass as a symbol.
• Assign a Key: Based on the frequency of the data, decide the frequency for one symbol. This can be done by setting a numerical value that one symbol will represent. This key or numerical value must be written along with the pictograph. Example one icon of an hourglass represents. This is the key to the pictograph.
• Draw the pictograph: Finally, drawing the pictograph by initially drawing two columns that represent the category and the data. Then, draw the actual symbols representing the frequencies. These symbols can be drawn as fractions as well if the frequency is not a whole number or based on the key.
• Review Pictograph: Check the labeling and review your pictograph and make sure it correctly represents the data.

Use Pictorial Representations To Help Year 6 Students Prepare For Maths Sats

Give pupils visual tools to make clear mathematical links come test time, says Beth Smith

In the run-up to Y6 SATs, teachers are faced with not only recapping previous years learning but also teaching the new Y6 content of the curriculum.

In order to ensure SATs dont turn into a memory test, we need to make clear links between new learning and prior knowledge so children can build on their understanding, rather than starting afresh with every new concept.

One of the most important concepts that children need to understand is the use of equal groups throughout the curriculum.

In KS1, children are introduced to equal groups within multiplication and division this starts with a basic understanding that 2 × 3 is two equal groups of three before deepening their understanding by comparing equal groups.

Take this example from the 2016 KS1 SATs:

Complete the number sentence below.3 x 8 = 2 x __

Using a bar model, children can visualise the equal parts in order to help them solve the problem.

Drawing the bar model supports us to decide which calculation to use.

Here, we need to find out the total of the each bar. If we multiply 3 by 8, we find the total of the bar is 24.

Once we have found the total, we can see that as the bars are equal, the second bar will also be worth 24 but this time it is divided into two equal parts.

Therefore, to find the missing information, we need to divide 24 by 2 to find our missing box is 12.

Heres a question from the 2017 KS2 reasoning paper.

Examples Of Pictorial In A Sentence

pictorialpictorialpictorial CNNpictorial VoguepictorialLongreadspictorial WSJpictorial CNNpictorial Los Angeles Timespictorial Varietypictorial The New York Review of Bookspictorial The Arizona Republicpictorial The New Yorkerpictorial clevelandpictorials Timepictorials EW.compictorials Fox News

These example sentences are selected automatically from various online news sources to reflect current usage of the word ‘pictorial.’ Views expressed in the examples do not represent the opinion of Merriam-Webster or its editors. Send us feedback.

Recommended Reading: How To Calculate Net Force Physics

Using Concrete Pictorial And Abstract Math Progressions

Saint Theresa Catholic School, . Using the Singapore math method, Saint Teresa Catholic School employs a Concrete, Pictorial, and Abstract progression in mathematics instruction emphasizing number bonds, bar modeling, and mental math. This progression enables students to master concepts by stages. Beginning in PreK through grade three, the school teaches mathematics in two-block periods each day. Teachers introduce students to new concepts during the first period, while the second period is devoted to practice. Each day the school identifies struggling students through formative assessments in Math 1. The struggling students then receive daily remediation through direct instruction in Math 2.

What Is The Pictorial Stage Of The Cpa Approach

The pictorial step is the âseeingâ step. In this stage, problems are modelled using the visual representation of a physical object. This step of CPA method motivates children to make a direct connection between the concrete object they just dealt with and the visual representation, models or diagrams that represent the problem.

Once students understood a maths concept using real objects, students may proceed with drawing quick sketches or visual representations of the objects. While benefiting from pictorial representation, students would no longer manipulate the physical objects, but still take advantage of the pictorial support the resources may provide.

Some mathematics teachers tend to leave this step out, but visual recording is crucial to ensuring that students can make the connection between a physical resource and abstract notation. In absence of pictorial representation, children may find it difficult to visualise a problem.

Drawing or developing a model makes it easier for the students to understand complex mathematical and conceptual concepts . An example of the pictorial step is using the bar model where bars represent the unknown and known quantities in more complex multi-step problem-solving.

Recommended Reading: Punchline Bridge To Algebra 2nd Ed

Concrete Math For Young Learners

Children can, and should, learn math from a very early age. Developmental psychologist Herbert Ginsburg from the Teachers College at Columbia asserts that young students really enjoy learning math, especially when they are given the opportunity to manipulate objects, physically or digitally, in a fun and exciting way. Concrete math taps into that characteristic of the young learner to effectively lay the foundation for mathematical literacy.

Image by Design_Miss_C from Pixabay.

Results From Related Empirical Research

Since most pictorial charts rely on displaying quantities via representations of two- or three-dimensional objects, the readers ability and strategies to deal with measures might influence the interpretation of pictorial charts. However, the ability to calculate these measures exactly does not play a significant role when interpreting pictorial charts. Instead, the ability to estimate measures might be crucial.

Read Also: Simplifying Radicals With Imaginary Numbers Worksheet

Examples Of Concrete Math For Young Learners

Physical objects like toys and fruit can be used in a wide variety of applications. Children can compare groups of objects, such as a group of 2 bananas compared to a group of 3 oranges. At first, the learner could just choose which is bigger/smaller or more/less. As the child gains understanding and has ample practice, the greater than and less than vocabulary and symbol can be introduced.

Children can work on numbers by matching a group of items with the matching numeral, for example matching the number 5 with a group of 5 toy cars. Addition, subtraction, division, and even multiplication can all be represented with physical objects to lay a foundation of mathematical fluency and comprehension.

Digitally, children can practice problem solving skills as we see in this sample MathBRIX game that asks children to match the number with the correct brick. Virtually every early math skill can be practiced and explored with virtual concrete math games.

Approaching problem solving in a concrete way is a necessary first step in a learners journey to mathematical literacy. Utilizing real objects, math manipulatives, and digital versions of both help form a solid foundation from which a childs mathematical understanding can grow.

Cpa Maths Approach: Abstract

Abstract is the symbolic stage, where children are able to use abstract symbols to model and solve maths problems.

Once a child has demonstrated that they have a solid understanding of the concrete and pictorial representations of the problem, the teacher can introduce the more abstract concept, such as mathematical symbols.

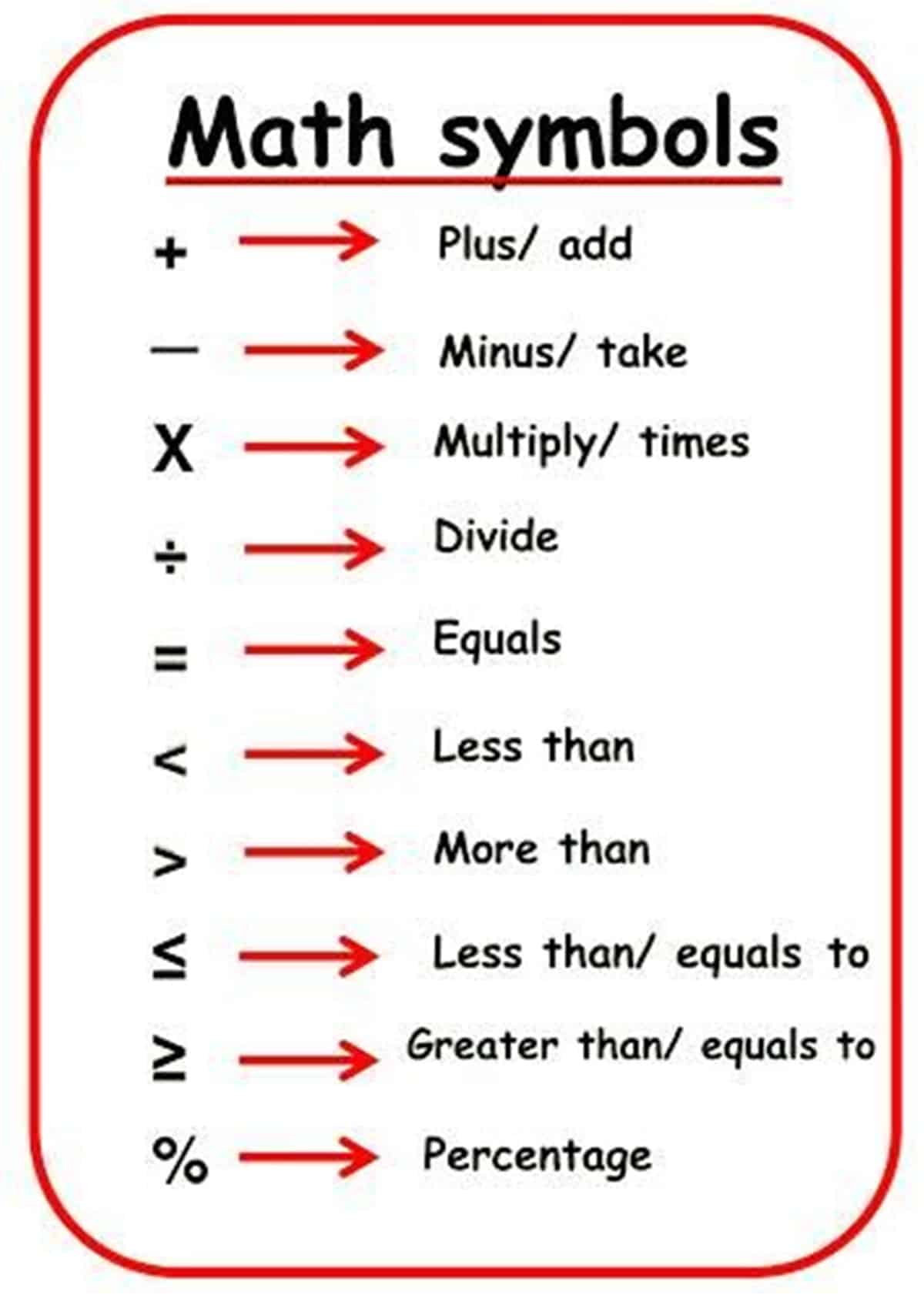
Children are introduced to the concept at a symbolic level, using only numbers, notation, and mathematical symbols, for example +, , x, / to indicate addition, multiplication, or division.

So, for the following problem:

Jim has 12 cookies. Julie has 8 cookies. How many do they have altogether?

Children at the abstract stage would be able to solve the problem by writing it out as 12 + 8 = 20.

You May Like: Is Physics Easier Than Chemistry

What Is Concrete > Pictorial > Abstract In Maths

The CPA method involves using actual objects for children to add, subtract, multiply or divide. They then progress to using pictorial representations of the object, and ultimately, abstract symbols.

Children often find maths difficult because it is abstract. The CPA approach helps children learn new ideas and build on their existing knowledge by introducing abstract concepts in a more familiar and tangible way.

Less Structured Recording Opportunities

Many recorded maths opportunities rely heavily on worksheets blank paper is available, but work done on this is rarely kept or valued. From questionnaires completed as part of the research carried out by Worthington and Carruthers , only 16% of teachers referred to children making their own mathematical marks and recordings in maths lessons and in child-initiated play, and in another article by Worthington and Carruthers , they say:

Without opportunities to explore their own mathematical thinking in their own ways and through their own choices of visual representation, children are prevented from developing their own understanding of the written symbols of mathematics.

So how do we ensure opportunities for pupils to create their own marks and jottings and incorporate the practical resources and ensure that pupils maths books are presentable and that they help show the childrens progression through the learning?

Lets consider the use of concrete resources to support maths learning.

I would never suggest not using a range of practical resources, but we have to ensure that pupils dont become over-reliant on them or use them purely procedurally and therefore dont make the link between the actions they carry out with the resources and the abstract calculations they are completing.

In the summary of Chapter 3 of the EEF guidance report: Improving mathematics in early years and key stage 1 about manipulatives and representations to develop understanding, it recommends:

Also Check: Ct Algebra 1 Curriculum Version 3.0

What Is The Best Way To Move Learners From A Concrete To Abstract Approach

Historically, the use of concrete objects in the classroom was limited to early childhood education. In current times, the maths masteryapproach expects children of both primary and secondary schools to progress using manipulatives. Every child grasps mathematical concepts at a different speed, so they may progress to the abstract stage in some areas but remain at the concrete stage in others. Following are three easy ways to move students from concrete to abstract representation:

What Is Concrete Math

Concrete math is a foundational practice that lays the groundwork for later abstract problem solving. Used extensively in preschool and early grades, it starts with what young learners already understand and builds upon it. It gives teachers and parents a way to introduce abstract ideas, such as adding or dividing, in a tangible way. This stage of learning is the doing phase, where young children sort, count, divide, add, or subtract using physical objects rather than symbolic representations.

Children may initially use objects that are real, such as pieces of fruit, before moving on to math manipulatives, such as counting cubes, that are meant to represent apples, oranges, and bananas. It is most useful for children to have access to a variety of objects so that they can choose their own method and approach to problem solving in a variety of ways.

One of the reasons concrete math and the use of physical objects is so effective is due to the mind-body connection. Using our bodies while learning helps us better remember what we learn and transfer that knowledge more readily. This phenomenon is called embodied cognition.

Read Also: Glencoe Geometry Worksheets

Concrete Math Is Conceptual Learning

The math curricula used today in early grade mathematics is conceptual in nature. Conceptual math focuses on giving kids a foundational understanding of problem solving rather than teaching students processes to approach a particular problem. Conceptual learning aims to increase literacy in mathematicsrather than teach steps to find solutions. This fosters deep understanding that students can transfer to higher level math work as well as other subject areas, such as science and engineering.

Part of the aim of conceptual learning is to aid students in moving along the continuum of concrete to abstract learning. CPA, or Concrete, Pictorial, Abstract, is an approach developed by American psychologist Jerome Bruner and is an integral component of the widely adopted curriculum Singapore Math. His research showed that children are most successful in math when they are instructed first with concrete materials, then pictorial representations, and finally with abstract problems that use symbols.

Many national groups have researched the efficacy and importance of using conceptual math in the classroom. The research concludes that the study of mathematics should emphasize higher order thinking skills, deep understanding of concepts, mathematical communication, making connections, and applying mathematics to other areas of learning. This level of understanding in young learners begins with concrete math.

Why Use The Concrete Pictorial Abstract Approach In Maths

Pupils achieve a much deeper understanding if they dont have to resort to rote learning and are able to solve problems without having to memorise.

When teaching reading to young children, we accept that children need to have seen what the word is to understand it. Putting together the letters c- a- t would be meaningless and abstract if children had no idea what a cat was or had never seen a picture.

People often dont think of this when it comes to maths, but to children many mathematical concepts can be equally meaningless without a concrete resource or picture to go with it. This applies equally to mathematics teaching at KS1 or at KS2.

You May Like: Who Is Paris Jackson’s Father

How To Teach Using The Concrete Pictorial Abstract Method At Primary School

A common misconception with this CPA model is that you teach the concrete, then the pictorial and finally the abstract. But all stages should be taught simultaneously whenever a new concept is introduced and when the teacher wants to build further on the concept.

When concrete resources, pictorial representations and abstract recordings are all used within the same activity, it ensures pupils are able to make strong links between each stage.

If youre concerned about differentiating effectively using the CPA approach, have a look at our differentiation strategies guide for ideas to get you started. Or if youre short on time, our White Rose Maths aligned lesson slides incorporate the CPA approach into them and some are free to download and use.

What Is Mean By Pictograph In Maths

Pictograph

. Thereof, what is pictograph in math with example?

A pictogram or pictograph represents the frequency of data as pictures or symbols. Each picture or symbol may represent one or more units of the data. Example : The following table shows the number of computers sold by a company for the months January to March.

Likewise, what is pictograph and its uses? A pictograph uses picture symbols to convey the meaning of statistical information. Pictographs should be used carefully because the graphs may, either accidentally or deliberately, misrepresent the data. One cookie symbol represents two students, and a half-cookie image is used to represent one student.

Just so, what is an example of a pictogram?

A pictogram is a symbol that conveys meaning through its resemblance to a physical object. Examples of pictograms include wayfinding signage, such as in airports and other environments where many people may not be familiar with the language of the place they are in. We can see pictograms and ideograms everywhere.

Why are pictograms used?

Pictograms are images that are used to represent data. It’s common for infographics to make use of a pictogram. They present data in a fun and new way, and can be very effective for communicating a message in an emotionally evocative way.

You May Like: Oxygen Difluoride Polar Or Nonpolar

Why Use The Concrete Pictorial Abstract Approach To Teach Maths

CPA is developed to help learners understand mathematics meaningfully. Under the CPA approach, the learners will be taught by providing:

• the essential learning experiences using the concrete materials
• the visual representations of the mathematical concepts or techniques
• And, the mathematical symbols at the abstract stage.

CPA is widely used to teach mathematics to primary school students in Singapore.

But, as a general teaching principle, it is also used to teach mathematics in secondary schools .

Many children find mathematics hard because it is abstract. The distinct stages of the CPA approach to teaching builds on childrenâs current understanding by instructing abstract concepts using tangible and concrete methods. It involves shifting from concrete materials, to pictorial representations, and then towards abstract concepts and symbols.

What Do We Mean By Pictorial In The Cpa Approach

Do we encourage young children to draw pictures when engaging with the CPA approach? Are these pictures important?

I am a great believer in the importance of using Bruners Concrete, Pictorial, Abstract approach to support the conceptual development of mathematics with pupils of all ages. See Rachel Rayners blog, The CPA approach, to find out more.

With our younger pupils, it is also important that the children get time to explore and play with a wide range of resources.

But do we give young children enough time, space and encouragement to develop the pictorial aspect of CPA and is this necessary?

My short answers are no and yes.

No, I dont feel we encourage or appreciate the importance of pictures in mathematics and yes, this is necessary.

Like all areas of development, childrens mark making is an essential skill that we must learn because mark making can be refined and developed into writing and drawing, both of which are a way of recording our explorations, thoughts, and interpretations about the world around us.

The new statutory Early Years framework for mathematics doesnt explicitly mention mark making or recording. It does however state that:

By providing frequent and varied opportunities to build and apply this understanding, children will develop a secure base of knowledge and vocabulary from which mastery of mathematics is built.

Lets look at some examples of childrens drawing of two:

For me, this example highlights several points:

Don’t Miss: What Does Open Sentence Mean In Math