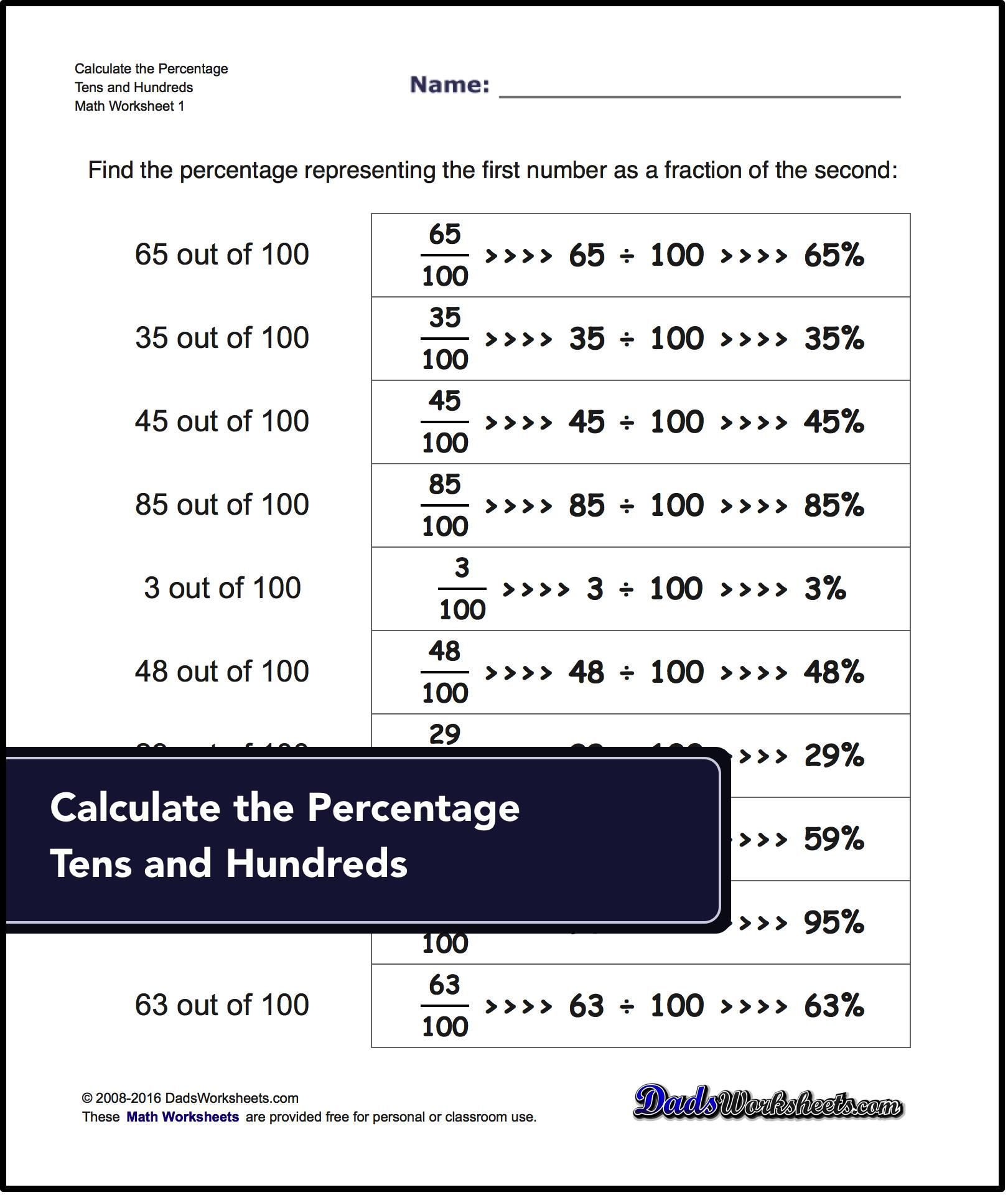
How To Calculate Percentages By Working Backward
Sometimes, you will be required to calculate percentages by working backward. This is also referred to as reverse percentages and is used when the percentage and the final number are given and the original number is to be calculated.
For example, if 40% of a number is 500, what is the number? The following are ways to calculate the percentage by working backward:
-
Find the percentage of the original or real number. In this case, it’s 500.
-
Multiply the final number by 100. 500 x 100 = 50,000.
-
Divide the result of the multiplication by the percentage. 50,000 divided by 40% = 1,250. Thus, 500 is 40% of 1,250. Therefore, the original number was 1,250.
Related:
Here are several examples of percentages and how to calculate them:
How Do You Find A If P% Of It Is B
For example: 12 is 20% of which number?
- Convert the problem to an equation using the percentage formula: B/P% = A
- B is 12, P% is 20, so the equation is 12/20% = A
- Convert the percentage into a decimal form by dividing it by 100.
- Substitute 0.20 for 20% in the equation: 12/0.20 = X
- So 12 is 20% of 60
Easy Ways To Calculate Percentages
How much is 15 percent of 210? How about 75 percent of 440 or 350 percent of 20? The standard way of calculating percentages can make these problems difficult to do without a calculator. Luckily, there are several tips and tricks you can use to solve percentage problems without a calculator. Once you understand these simple methods, these questions become easy to solve.
Don’t Miss: How To Find Net Force In Physics
How To Do Percentages In Your Head
So how much is 15 percent of 210? You can use the four key percentages you memorized to figure this out. Consider that 15 percent is 5 percent added to 10 percent. Since 10 percent of 210 is 21, and 5 percent is half that, or 10.5, then 15 percent is 21 added to 10.5, or 31.5.
How about 75 percent of 440? Here you can figure that 75 percent is 50 percent added to 25 percent. Since 50 percent of 440 is half that, or 220, and 25 percent of 440 is one fourth, or 110, then 75 percent is 220 added to 110, or 330.
In this way you can combine 5 percent, 10 percent, 25 percent, and 50 percent to calculate a wide variety of percentages in your head. If you need to calculate a percentage that is not a multiple of 5, you can use this technique to estimate the answer very closely.
Rating The Effectiveness Of A Treatment
Lets say we want to find out how much a treatment improves a metric we are tracking. The treatment could be a prescription medication or a diet plan, and the metric could be blood pressure, cholesterol, or other measures.
Example: Using Percentage To Rate Effectiveness Of A Treatment
Lets say we have two groups of people: one group goes on a specific diet, and the other group is a control group .
After a year on the diet, the first group has an average weight loss of 8%. The second group has an average weight loss of 0% .
It appears that the diet may have some effect on weight loss. However, we would need to do a hypothesis test to see if the diet really does have an effect.
We might have a null hypothesis that the diet has no effect, and our test would determine whether we reject that hypothesis or not. The outcome of the hypothesis test would depend on several things, including:
- the size of each group in the diet study
- the significance level
You May Like: Who Uses Math In Their Job
How To Calculate Percentage Using Formula
We can use the formula to calculate the percentage easily and quickly. The formula to calculate percentage is equal to the ratio of the actual value to the total value multiplied by 100. The percentage formula is:
| \ |
Let us understand this concept and formula with the help of an illustrative example given below:
Example: A circle below has ten divisions, and a few parts are shaded. Calculate the percentage of shaded parts in the circle.
Solution:
Total number of divisions in the circle = 10
Number of shaded parts = 2
We know that,
Percentage, % = × 100
Thus, the percentage of the shaded part of the circle = × 100
= × 100
How To Calculate Percentage Of Marks
Most of the students get a question on how to calculate the percentage of marks obtained in exams. Here is the answer to this question along with the example. Two simple steps give you the percentage of marks. They are:
Step 1: Divide the obtained marks by the maxim marks of the test.
Step 2: Multiply the result by 100.
Go through the example given below to understand the process of finding the percentage of marks.
Example 1: A student scored 1156 marks in the examination out of 1200 marks. Calculate the percentage of marks secured by the student.Go through the example given below to understand the process of finding the percentage of marks.
Solution:
Number of marks scored = 1156
Maximum marks = 1200
Percentage of marks = × 100
Percentage= 0.9633. × 100
Therefore, the percentage of marks obtained is 96.3%
Don’t Miss: What Is The Difference Between Geography And History
Estimating A Population Percentage From A Sample
Sometimes we want to find how many people in a population have a certain trait. However, it is difficult to poll an entire population if the group is large or hard to reach.
Instead, we can take a representative sample from the population, calculate a percentage for the sample, and then apply this to the population as a whole to get a reasonable estimate.
Note that we want a representative sample from the population. This means that if we are looking at all adults in the U.S., we dont want to take a sample from just one state.
Example: Using Percentages To Estimate Population Parameters From Sample Statistics
Lets say we have a population of 100,000 people in a city and we want to find out how many read at least one book per week.
Since there are so many people in the population, it will be difficult to poll the entire city. Instead, we will use a representative sample to make an estimate.
After randomly selecting people to poll, we get responses from a sample of 1,000 people in the city. The data shows that 85 of them read at least one book per week.
To find the percentage, we calculate the fraction 85/1000 , which gives us a decimal of 0.085. To get a percentage, we multiply by 100 and add a percent symbol to get 8.5%.
So, 8.5% of the sample reads at least one book per week. Assuming our sample is representative of the city population as a whole, we can apply this percentage to the entire group.
Nearest To The Milestone
50 and 100 are considered milestone numbers. This approach works best for calculating percentage near to these numbers, say upto +5 to -5 to the milestones.
Lets take the 51% of 140.
Here, the milestone will be 50. From the question, percentage value is 1 more than the milestone. So, we will rearrange it as follow:
51% of 140
= % of 140
Here, 51% of 140 will be equal to the addition to the 50% of 140 and 1% of 140.
As we know already that 50% is just half of the number and rearranging zeroes of 1% to 140 will give 1 x 1.4
The answer becomes = => 70 + 1.4 => 71.4
Lets take another example of 97% of 208.
Here, the milestone will be 100. From the question, percentage value is 3 less than the milestone. So, we will rearrange it as follow:
97% of 208
= % of 208
Here, 97% of 208 will be equal to the subtraction between the 100% of 208 and 3% of 208.
As we know already that 100% is complete whole of the number and rearranging zeroes of 3% to 208 will give 6.24 .
The answer becomes = => 208 6.24 => 201.76
Don’t Miss: What Does Aba Mean In Psychology
How To Obtain A Percentage
Lets find out how to calculate percentages using the two methods mentioned above.
If two or more numbers sum up to 100, the percentage of those individual values to the total value equals that number. For example, Sam purchased balls in three different hues. The following table lists the specifics of the purchase.
| Colour |
| 60% |
How To Calculate Percentages In 3 Easy Steps
The Indeed Editorial Team comprises a diverse and talented team of writers, researchers and subject matter experts equipped with Indeed’s data and insights to deliver useful tips to help guide your career journey.
Calculating percentages is an easy mathematical process to carry out. Sometimes, when there is the need to find the ratio or portion of a quantity as a part of another quantity, you will need to express it as a percentage.
In this article, we show you what percentages are and how to calculate them. We also provide examples of using percentages.
Also Check: What Is Mass Measured In Physics
How To Calculate Percentage Decrease
As a value is decreased over time, the percentage decline refers to the per cent change in the value. For example, a drop in rainfall over a region, a drop in the number of Covid patients, and so on. The following formula can be used to compute the percentage decrease:
Percentage Decrease or Percentage Decline= / The Original Value * 100
Free Video Lessons On Percents And Percentages
Find here free math videos on percent-related topics: what is percent, percentage of a number, discouns, sales tax, and percent of change. These videos are meant equally for students, teachers, and parents.
The videos match the lessons in my book Math Mammoth Percent , or the lessons in chapter 5 of Grade 6-A , or chapter 7 in Grade 7-B . In any of those books, you will get MANY more practice exercises, word problems, and puzzles than what are shown in the videos, and also some lessons that are not in covered in this set of videos.
You May Like: What Is The Peter Principle In Psychology
How To Calculate The Percentage Of A Number
For example: What is 15% of 200?
- Using the percentage formula, convert the problem to an equation, which is P% * A = B
- P is 15%, A is 200, so the equation is 15% * 200 = B
- Convert 15% to a decimal by removing the per cent sign and dividing by 100. Therefore, 15/100 = 0.15
- Substitute 0.15 for 15% in the equation: 15% * 200 = B, which becomes 0.15 * 200 = B
- So 15% of 200 is 30
Percentages In Everyday Life
In addition to being used throughout math and science, percentages are used in many aspects of everyday life, such as discounts when shopping, taxes, interest rates, statistics, food labels, and much more. As such, it is important to have a good understanding of percentages to enable you to perform the various percentage calculations you may need in your studies as well as in life. Below are a few such examples.
Recommended Reading: What Is C5 In Chemistry
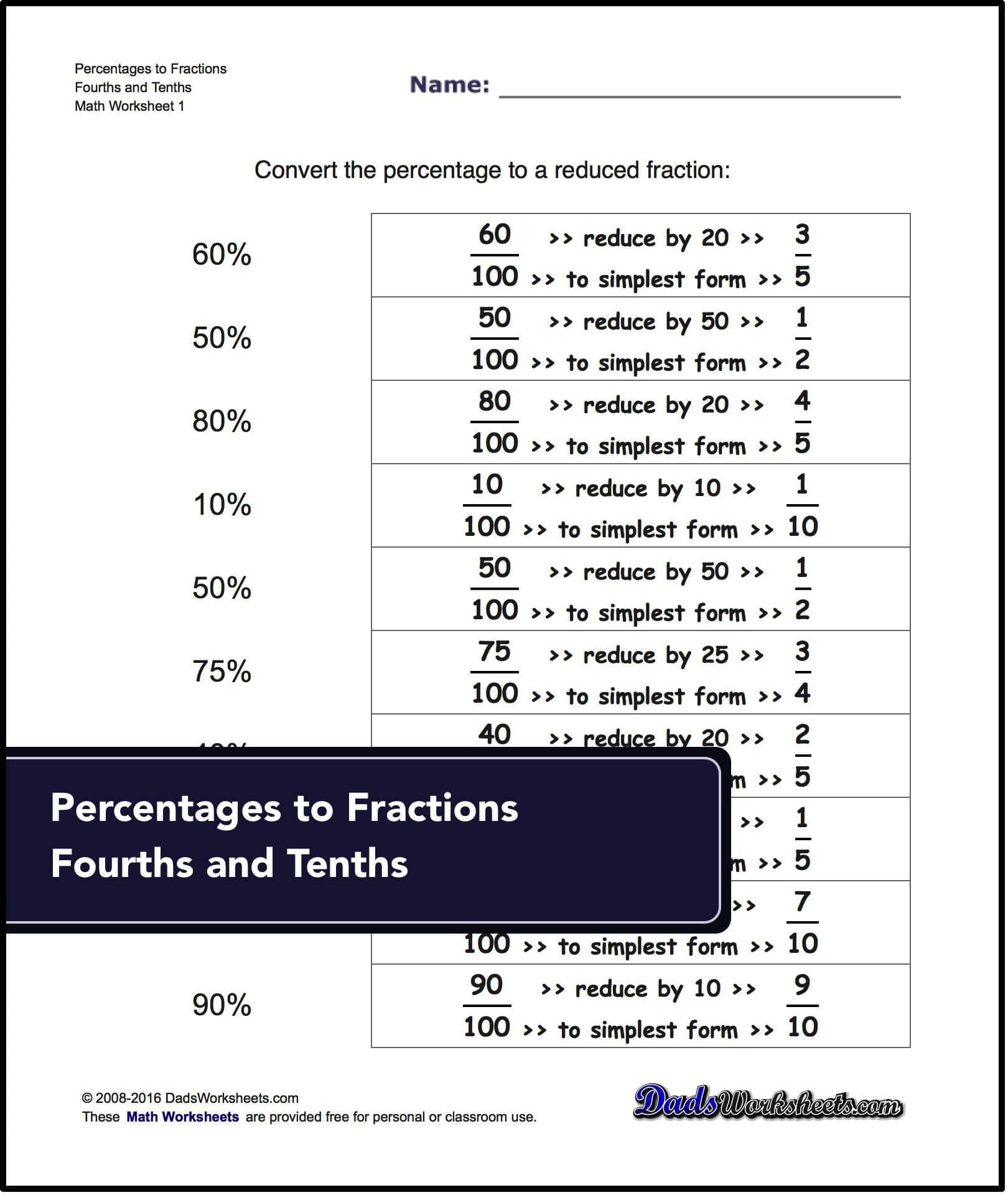
Converting Percent To Fraction
Sometimes, converting percent to fraction is an easier method to obtain the percentage. Fractions are preferred to be used than decimals if the decimal has many digits. This makes the multiplication more convenient since only factorization is used to simplify the percentage.
Here are the steps in converting percent to fraction:
1.Neglect the percent symbol .
2.Divide the percent by 100. If the numerator has digits to the right of the decimal point, move the decimal point until the numerator becomes a whole number. Move the decimal point of the denominator by the same number of decimal places that the decimal point of the numerator has moved.
3.Reduce to lowest terms.
Example 13:
Neglect the percent symbol and divide the percent by 100.
`804/100`
Reduce to lowest terms.
`804/100 = text/text = 201/text = 201/25 or 6 1/25`
Therefore, the fraction form for 804% is `8 1/25` or `201/25`.
Calculating The Percentage Of A Whole
To calculate a percentage, the whole amount must be known. This is in addition to the percentage or portion amount. You may be asked âwhat percentage of W is P,â where W is the whole amount and P is the portion amount. Or the question may be âhow much is X percent of W,â where X represents a percentage figure.
1. What is a percentage?
A percentage is a way to express a number as a part of a whole. To calculate a percentage, we look at the whole as equal to 100%. For example, say you have 10 apples . If you eat 2 apples, then you have eaten 2/10 Ã 100% = 20% of your apples and you are left with 80% of your original apples.
The term percent in English comes from the Italian per cento or the French pour cent, which literally mean per hundred.
2. What is the value of the whole?
For instance, lets say we have a jar containing 1199 red marbles and 485 blue marbles, making it 1684 marbles in total. In this case, 1684 makes up a whole jar of marbles and will be set equal to 100%.
3. Turn the value into percentage
Lets say we want to find out the percentage of the jar that is taken up by the 485 blue marbles.
4. Put the two values into a fraction
In our example, we need to find out what percent 485 is of 1684 . Therefore the fraction, in this case, is 485/1684.
5. Convert the fraction to a decimal.
To turn 485/1684 to a decimal, divide 485 by 1684. This comes to 0.288.
Formula: 485/1684 = 0.288
6. Convert the decimal into a percent
Formula: 0.288 x 100 = 28.8 or 28.8%
Also Check: Who Created Common Core Math
Rule Of Three For Calculating Percentages
In earlier posts, we have learned what a percent is and how to calculate it.
Today we are going to use the rule of three to solve different types of problems related to percentages.
Rule of three to calculate the percentage of a number
For example, we want to calculate 30% of 360.
30% means 30 for each 100. So the approach would be: if I have 30 from 100, I have X from 360:
100 30
X = / 100
X = 108
So, 30% of 360 is 108.
Rule of three to calculate a quantity knowing the percentage of it
For example, we know that 25% of a quantity is 49. What is the quantity?
If 25% is 49 then the 100%, which we do not know, will be X :
25 49
X = / 25
X = 196
The quantity we are looking for is 196.
Rule of three to calculate the percentage represented as a quantity of another
What percentage of 250 does 50 represent?
250 is the 100% and 50 is the percentage that we do not know, X :
250 100
X = / 250
X = 20
50 is 20% of 250.
Rule of three to calculate the percentage of an unknown quantity knowing another percentage of the quantity
We know that 40% of a quantity is 78, how much would 60% be of the same quantity?
The 40% is 78 and we want to calculate 60%, which will be X :
40 78
X = / 40
X = 117
So 60% of this quantity is 117.
Today we have learned to solve different problems related to percentages using the same tool: the rule of three. To learn more about this and other contents from primary mathematics, check out Smartick and try it for free.
Learn More:
How To Compute Percentage Greater Than 100 Percent
To calculate a percentage greater than 100 percent, simply move the decimal of the percent two spaces to the left and use the answer to multiply the number in question. You can do this to figure out what is 350 percent of 20. Moving the decimal of the percent two spaces to the left gives you 3.5. Multiplying 20 by 3.5 then gives you the answer, which is 70.
Related Articles
Recommended Reading: Thinkwell College Algebra Online Version
P Percent Of What Number Is Y