What Does Sequence Mean In Math

How To Find The Next Term In A Number Sequence

What Is the Meaning of Sequencing in Math?

A number sequence is a list of numbers arranged in a row. Let us lookat two examples below. 4, 6, 1, 10, 14, 5, 4, 7, 10, 13, .

Number sequence is a list of numbers without order or pattern. You cannot tell what number comesafter 5.

Number sequence has a pattern. Do you observe that each number is obtained by adding 3 to thepreceding number ?

In these lesson, we will only study number sequences with patterns.

Some other examples of number sequences are:

Number Sequence

divide by 3

What Is The Difference Between A Sequence And A Series

While the English words “sequence” and “series” have similar meanings, in mathematics they are completely different concepts. A sequence is a list of numbers placed in a defined order while a series is the sum of such a list of numbers. There are many kinds of sequences, including those based on infinite lists of numbers. Different sequences and the corresponding series have different properties and can give surprising results.

TL DR

Sequences are lists of numbers placed in a definite order according to given rules. The series corresponding to a sequence is the sum of the numbers in that sequence. Series can be arithmetic, meaning there is a fixed difference between the numbers of the series, or geometric, meaning there is a fixed factor. Infinite series have no final number but may still have a fixed sum under certain conditions.

Definition And Basic Examples Of Arithmetic Sequence

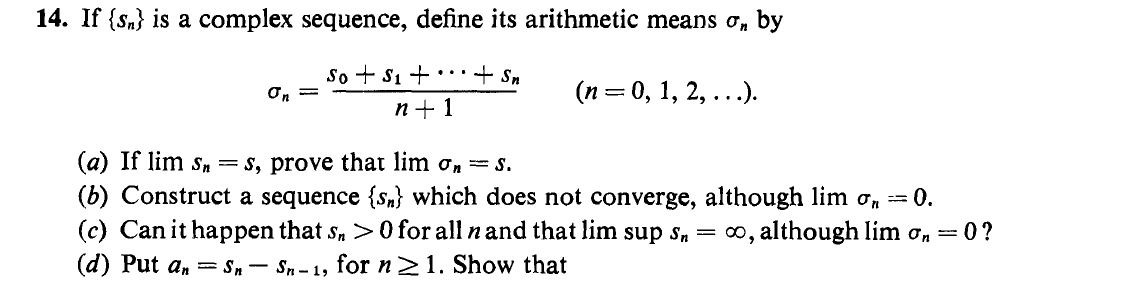
An arithmetic sequence is a list of numbers with a definite pattern. If you take any number in the sequence then subtract it by the previous one, and the result is always the same or constant then it is an arithmetic sequence.

The constant difference in all pairs of consecutive or successive numbers in a sequence is called the common difference, denoted by the letter d. We use the common difference to go from one term to another. How? Take the current term and add the common difference to get to the next term, and so on. That is how the terms in the sequence are generated.

If the common difference between consecutive terms is positive, we say that the sequence is increasing.
On the other hand, when the difference is negative we say that the sequence is decreasing.

Also Check: Kuta Angle Addition Postulate

What Are Arithmetic Sequences

Now lets talk about a specific type of mathematical sequence: the arithmetic sequence. I know it sounds complicated, but its really pretty simple. An arithmetic sequence is a sequence of numbers where the difference between any two successive elements is always the same constant value. For example, the sequence of years since the start of the new millennium is an arithmetic sequence:

2001, 2002, , 2009, 2010.

Why is this an arithmetic sequence? Because the difference between all successive elements is always the same2002 2001 = 1, 2010 2009 = 1the difference is always 1.

Notice Ive used ellipses here in the middle of the sequence. What does that mean? Well, ellipses are used like this to represent missing elementsin this case: 2003, 2004, and so on, up to 2008. I could have written them all out explicitly, but using ellipses saves some writing.

Arithmetic Sequences And Series

An arithmetic sequence is a sequence of numbers such that the difference of any two successive members of the sequence is a constant.

Example

2,4,6,8,10.is an arithmetic sequence with the common difference 2.

If the first term of an arithmetic sequence is a1 and the common difference is d, then the nth term of the sequence is given by:

$$a_=a_+d$$

An arithmetic series is the sum of an arithmetic sequence. We find the sum by adding the first, a1 and last term, an, divide by 2 in order to get the mean of the two values and then multiply by the number of values, n:

$$S_=\frac$$

Example

Find the sum of the following arithmetic series 1,2,3..99,100

We have a total of 100 values, hence n=100. Our first value is 1 and our last is 100. We plug these values into our formula and get:

$$S_=\frac=5050$$

What Are Even And Odd Numbers

The difference between successive elements in an arithmetic sequence doesnt have to be 1in fact, it can be anything. There are two famous arithmetic sequences youre already familiar with whose successive members have differences of 2: the even and odd positive integers. Positive even integers begin at 2 and increase in steps of 2:

2, 4, 6, 8, 10,

whereas positive odd integers begin at 1 and increase in steps of 2

1, 3, 5, 7, 9,

What Is A Sequence Equation

A geometric sequence is a sequence in which the ratio of any term to the previous term is constant. The explicit formula for a geometric sequence is of the form a n = a 1 r-1, where r is the common ratio. A geometric sequence can be defined recursively by the formulas a 1 = c, a n+1 = ra n, where c is a constant and r is the common ratio.

Don’t Miss: Lesson 4.5 Practice A

Whats The Difference Between A Mathematical Series And A Sequence

As you may recall, we talked about something called a mathematical sequence in earlier articles. To refresh your memory, a sequence in math is simply a list of numbers that are arranged in a particular order. The first five positive integers 1, 2, 3, 4, 5 and the Fibonacci numbers 1, 1, 2, 3, 5, 8, 13, which are related to the golden ratio are two examples of mathematical sequences. The first is whats called a finite sequence since its made up of a non-infinite number of things . Since the Fibonacci numbers go on and on forever, theyre whats called an infinite sequence.

But were not talking about sequences today, so why am I bringing them up? Well, as it turns out, theres something in math called a series thats very closely related to the idea of a sequence. And its precisely this idea of a series that we need to understand in order to answer our question about the length of an infinite number of measuring sticks. So, lets get to it.

Can An Infinite Number Of Things Have A Finite Sum

Geometric Mean | Geometric Sequence | Sequences & Series | Mathacademy

So, what about the question that kicked this whole thing off? Can an infinite number of measuring sticks stacked end-to-end have a finite length? The answer depends on whether were talking about real world measuring sticks or idealized mathematical ones. In the real world, there are limits on how small things can be. For example, itd be pretty tough to make a measuring stick thats smaller than a single atom. But in the mathematical world, you can imagine making measuring sticks that get smaller and smaller and infinitely smaller. Then, in exactly the same way that the total area of the infinite number of ever shrinking rectangles is finite, the total length of the stacked measuring sticks could be finite too.

What do you think? Pretty fascinating stuff, right? Indeed, mathematical series are very cool. And, as well see next week, theyre very useful in the real world too!

You May Like: What Is The Formula Of Volume In Physics

Other Types Of Sequences

Some other types of sequences that are easy to define include:

An integer sequence is a sequence whose terms are integers.
A polynomial sequence is a sequence whose terms are polynomials.
A positive integer sequence is sometimes called multiplicative, if anm = anam for all pairs n, m such that n and m are coprime. In other instances, sequences are often called multiplicative, if an = na1 for all n. Moreover, a multiplicative Fibonacci sequence satisfies the recursion relation an = anâ1anâ2.
A binary sequence is a sequence whose terms have one of two discrete values, e.g. base 2 values , a series of coin tosses H,T,H,H,T, …, the answers to a set of True or False questions , and so on.

How To Write Mathematical Sequences That Are Infinitely Long

If a sequence has infinitely many elements, we indicate that by writing ellipses at the end of the sequence if it extends out indefinitely in the positive direction, or at the beginning of the sequence if it extends out indefinitely in the negative direction. For example, the sequence of positive integers can be written

1, 2, 3, 4, 5,

The indicates the sequence continues forever in the positive direction. The sequence of negative integers can be written

, -5, -4, -3, -2, -1.

Here, the indicates the sequence continues forever in the negative direction. Putting these two together, the sequence of all integers can therefore be written

, -5, -4, -3, -2, -1, 0, 1, 2, 3, 4, 5, …

Don’t Miss: Eoc Fsa Warm Ups Algebra 1 Answers

Convergent And Divergent Series

Infinite series are divergent if the sum approaches infinity as the number of terms increases. An infinite series is convergent if its sum approaches a non-infinite value such as zero or another fixed number. Series are convergent if the terms of the underlying sequence rapidly approach zero.

The series adding the terms of the infinite sequence 1, 2, 4… is divergent because the terms of the sequence keep growing, allowing the sum to reach an infinite value as the number of terms increases. The series 1, 0.5, 0.25… is convergent because the terms rapidly become very small.

While sequences are ordered lists of numbers and series are sums, both can be important tools in evaluating sets of numbers, and the properties of convergence or divergence may have real life implications. A divergent series often represents an unstable condition while a convergent series often means that a process or structure will be stable.

Types Of Sequences And Series

Common sequences are arithmetic or geometric. In an arithmetic sequence, each number or term of the sequence differs from the previous term by the same amount. For example, if an arithmetic sequence difference is 2, a corresponding arithmetic sequence might be 1, 3, 5…. If the difference is -3, a sequence might be 4, 1, -2…. The arithmetic sequence is defined by the starting number and the difference.

For geometric sequences, the terms differ by a factor. For example, a sequence with a factor of 2 might be 2, 4, 8… and a sequence with a factor of 0.75 might be 32, 24, 18…. The geometric sequence is defined by the starting number and the factor.

The series types depend on the sequence that is being added. An arithmetic series adds the terms of an arithmetic sequence, and a geometric series adds a geometric sequence.

What Is Iteration Mean

1 : version, incarnation the latest iteration of the operating system. 2 : the action or a process of iterating or repeating: such as. a : a procedure in which repetition of a sequence of operations yields results successively closer to a desired result.

You May Like: Algebra 2 Chapter 3 Test Answer Key

What Does This Definition Of A Sequence Mean

I came across this problem in a book of mixed mathematical excersices:

A sequence $a_1,a_2,…$ we denote with $$. A sequence $$ of positive numbers $a_n$ is said to be a “zero-sequence” if there for every $ > 0$ exist an integer $n_ \geq 1$ such that $a_n \lt $ for every $n \geq n_$. Let $$ and $$ be sequences with positive integers and let $c_n = a_n + b_n$. Show that $$ is a “zero-sequence” if and only if both $$ and $$ are “zero-sequences“.

Can someone please explain with words what the definition of a “zero-sequence” means and why it is interesting to know wether a sequence is a “zero-sequence“, i.e. what unique properties do “zero-sequences” have? You may assume that I have no previous knowledge of what this type of sequences are about and that I am not familiar with sequences in general.

The question is a bit open. However, here is one possible explanation of convergences with words:

“A sequence converges to zero, if every $\varepsilon$-ball around $0$ contains all elements of the sequence with just finitely many exceptiones.”

$. In higher dimensions this would be replaces by the ball of all points which have distance less than $\varepsilon$ to $0$.)

How does this relate to your definition?

First we descide which $\varepsilon$ value we choose. However, it is important to make sure that everything we do holds for all possible choices we do here.

Notice that the value $n_$ depends on the choice of $\varepsilon$.

Why do we care for zero-sequences?

Finite And Infinite Sequences

HOW TO SOLVE ARITHMETIC SEQUENCE, MEAN AND SERIES (MATH TUTORIAL)

A more formal definition of a finite sequence with terms in a set S is a function from \left \ to S for some n > 0. An infinite sequence in S is a function from \left \ to S. For example, the sequence of prime numbers is the function

1\rightarrow 2, 2\rightarrow 3, 3\rightarrow 5, 4\rightarrow 7, 5\rightarrow 11, \cdots

A sequence of a finite length n is also called an n-tuple. Finite sequences include the empty sequence that has no elements.

You May Like: Cf4 Lewis Structure Molecular Geometry

How Can You Picture The Meaning Of A Series

To answer this question, allow me to paint a picture in your mind. Imagine drawing a perfect square on a perfect piece of paper. Lets say that the area of this square is 1. Now imagine drawing a line down the middle of this square to make two rectangles. Whats the area of each? Well, since theyre each half the size of the square, they must each have an area of 1/2. Next, divide one of these two new rectangles in half. Now do this again, and again, and again, and so onliterally forever!

What do you end up with? Well, if you think about it, youll see that the rectangles youve drawn inside your original square have areas that follow the sequence: 1/2, 1/4, 1/8, 1/16, 1/32, 1/64, and so on. Do you see the pattern? Each element of this sequence is just the fraction 1/2 raised to a higher and higher integer power. For example, 1/2 = ^1, 1/4 = ^2, 1/8 = ^3, and so on. When you add all of the elements of this sequence together, you get the series:

1/2 + 1/4 + 1/8 + 1/16 + 1/32 + 1/64 +

Though this series has an infinite number of terms, the fact that the original square we divided into smaller and smaller rectangles is not infinitely large means that the series must actually be finite. In fact, it must be equal to 1. Why? Because thats the area of the original square. Which means that some infinite series can indeed have finite values!

Recursive Formulas For Sequences

When discussing arithmetic sequences, you may have noticed that the difference between two consecutive terms in the sequence could be written in a general way:

a_n=a_+d

The above equation is an example of a recursive equation since the nth term can only be calculated by considering the previous term in the sequence. Compare this with the equation:

a_n=a_1+d.

In this equation, one can directly calculate the nth-term of the arithmetic sequence without knowing the previous terms. Depending on how the sequence is being used, either the recursive definition or the non-recursive one might be more useful.

A recursive geometric sequence follows the formula:

a_n=r\cdot a_

An applied example of a geometric sequence involves the spread of the flu virus. Suppose each infected person will infect two more people, such that the terms follow a geometric sequence.

The flu virus is a geometric sequence: Each person infects two more people with the flu virus, making the number of recently-infected people the nth term in a geometric sequence.

Using this equation, the recursive equation for this geometric sequence is:

a_n=2 \cdot a_

Recommended Reading: Does Kamala Harris Have Any Biological Children

Number Sequence Explanation & Examples

The number sequence is an essential mathematical tool for testing a persons intelligence. Number series problems are common in most management aptitude exams.

The problems are based on a numerical pattern that is governed by a logical rule. For example, you might be asked to predict the next number in a given series following the laid rule.

The three prevalent questions in this exam that can be asked are:

Identify a term that is wrongly placed in a given series.

Find the missing number in a certain series.

Complete a given series.

What Is A Mathematical Series

construct a sequence Sn that does not converge but its ...

Learn what mathematical series are and why theyre important.

Pop quiz time: Do you think its possible to add up an infinite number of anything and still get a finite answer? In other words, if I hand you an infinite number of measuring sticks, is it possible that laying them all out end-to-end could produce anything other than an infinitely long line?

You might be tempted to jump to the conclusion that the answer is No, an infinite number of anything must add up to an infinite number! And maybe youre right. But instead of guessing, today were going to take a look and see what math has to say. And to do that were going to use something called a mathematical series.

SPONSOR: . Use the code MATH to be entered to win $1000.

Also Check: Eoc Fsa Warm Ups Answers