What Materials Have R
All insulation materials have R-Value, but how much really depends on several factors.
Insulation R-Value basically means the thicker the insulation, the more efficiently it will keep your home comfortable, in the case of traditional insulation like fiberglass, cellulose, foam board, and a few other options. There are materials, like foam insulation, where R-Value isnt as much of a factor because it creates an air seal so there is no air movement through it.
Many homeowners use R-Value to compare insulation, but this can be problematic because of what R-Value doesn’t take into account.
But I digress, well have more on that in a moment.
Now do some math and figure out how R-Value is calculated.
Where Does Euler’s Number E Come From
The number represented by e was discovered by mathematician Leonard Euler as a solution to a problem posed by another mathematician, Jacob Bernoulli, 50 years earlier. Bernoulli’s problem was a financial one.
Suppose you put $1,000 in a bank that pays 100% annual compound interest and leave it there for a year. You’ll have $2,000. Now suppose the interest rate is half that, but the bank pays it twice a year. At the end of a year, you’d have $2,250. Now suppose the bank paid only 8.33%, which is 1/12 of 100%, but paid it 12 times a year. At the end of the year, you’d have $2,613. The general equation for this progression is:
where r is 1 and n is the payment period.
It turns out that, as n approaches infinity, the result gets closer and closer to e, which is 2.7182818284 to 10 decimal places. This is how Euler discovered it. The maximum return you could get on an investment of $1,000 in one year would be $2,718.
What Is The Meaning Of Algebra In The Modern World
Algebra is the study of properties of algebraic equations and their associated algebraic structures. Algebra is one of the few sciences that have a true global reach, as it is used in mathematics, physics, engineering, and other areas of study.
Algebra has been used to solve real-world problems for centuries, and has been the subject of many scientific and mathematical revolutions.
Recommended Reading: What Is Chromosome In Biology
Euler’s Number In Nature
Exponents with e as a base are known as natural exponents, and here’s the reason. If you plot a graph of
you’ll get a curve that increases exponentially, just as you would if you plotted the curve with base 10 or any other number. However, the curve y = ex has two special properties. For any value of x, the value of y equals the value of the slope of the graph at that point, and it also equals the area under the curve up to that point. This makes e an especially important number in calculus and in all the areas of science that use calculus.
The logarithmic spiral, which is represented by the equation
is found throughout nature, in seashells, fossils and and flowers. Moreover, e turns up in numerous scientific contexts, including the studies of electric circuits, the laws of heating and cooling, and spring damping. Even though it was discovered 350 years ago, scientists continue to find new examples of Euler’s number in nature.
Related Articles
The Complete Ordered Field

The real numbers are often described as “the complete ordered field”, a phrase that can be interpreted in several ways.
First, an order can be lattice-complete. It is easy to see that no ordered field can be lattice-complete, because it can have no largest element .
Additionally, an order can be Dedekind-complete, see § Axiomatic approach. The uniqueness result at the end of that section justifies using the word “the” in the phrase “complete ordered field” when this is the sense of “complete” that is meant. This sense of completeness is most closely related to the construction of the reals from Dedekind cuts, since that construction starts from an ordered field and then forms the Dedekind-completion of it in a standard way.
But the original use of the phrase “complete Archimedean field” was by David Hilbert, who meant still something else by it. He meant that the real numbers form the largest Archimedean field in the sense that every other Archimedean field is a subfield of R R } is “complete” in the sense that nothing further can be added to it without making it no longer an Archimedean field. This sense of completeness is most closely related to the construction of the reals from surreal numbers, since that construction starts with a proper class that contains every ordered field and then selects from it the largest Archimedean subfield.
Recommended Reading: How To Use Foil Math
A Glimpse Into The Larger World Of Correlations
There is more than one way to calculate a correlation. Here we have touched on the case where both variables change at the same way. There are other cases where one variable may change at a different rate, but still have a clear relationship. This gives rise to what’s called, non-linear relationships.
Note, correlation does not imply causation. If you need quick examples of why, look no further.
Below is a list of other articles I came across that helped me better understand the correlation coefficient.
- If you want to explore a great interactive visualization on correlation, take a look atthis simple and fantastic site.
- Using Python, there multiple ways to implement a correlation and there are multiple types of correlation. This excellent tutorial shows great examples of Python code to experiment with yourself.
- A blog post by Sabatian Sauer goes over correlations using “average deviation rectangles”, where each point creates a visual rectangle from each point using the mean, and illustrating it using the R programming language.
- And for the deeply curious people out there, take a look at this paper showing 13 ways to look at the correlation coefficient .
Follow me on and check out my personal blog where I share some other insights and helpful resources for programming, statistics, and machine learning.
Thanks for reading!
Definition Of Real Numbers
Real numbers include rational numbers like positive and negative integers, fractions, and irrational numbers. Now, which numbers are not real numbers? The numbers that are neither rational nor irrational are non-real numbers, like, -1, 2 + 3i, and -i. These numbers include the set of complex numbers, C.
Observe the following table to understand this better. The table shows the sets of numbers that come under real numbers.
| Number set |
|---|
Don’t Miss: Who Is Paris Jackson’s Biological Father
Notation For The Value Of A Function
In most math texts and on this website, the value of a function $ \phi $ at an input $x$ is written $ \phi $. For example, if $ \phi $ is the squaring function, $ \phi =9 $. . But there are several other common ways to write the value of a function:
- Factorial function: $x!$ .{/li>
- Absolute value: $ \left| x \right| $ .
- Addition: $x + y$ .
- Time derivative: $ \dot $ .
- Square root: $ \sqrt $ .
This section describes the major possibilities in some detail.
What Are The Most Important Things You Should Know About Algebra
The most important things you should know about algebra include the basic operations that are used in algebra, how to solve equations, how to graph linear equations, and how to solve systems of linear equations. Additionally, algebra is used to solve problems in physics, engineering, and other mathematics disciplines.
You May Like: How Hard Is Ap Biology
Is 0 Real Or Imaginary
It is a matter of philosophical and religious beliefs. However, many people think that 0 is real, as it is the smallest number that can be expressed in a natural language.
Additionally, many people believe that 0 is the basis for all other numbers, as it is the number that is the smallest of all numbers that can be expressed in a natural language.
What Does R Equal In Algebra
In algebra, the symbol for a number is called a Radical. In this context, it means that the number is not simple to represent using the number system we are familiar with.
That is, the number can be written in a more abstract way using a different type of number system. For example, if we are trying to represent a number that is 4 in our familiar number system, we could write it as This number is called the complex number because it can be written in the complex plane.
This is because the real and imaginary parts of a complex number can be written separately, and so the number is really a combination of these two types of numbers.
Radical numbers are really special because they can be used to represent numbers that are very complex. For example, the number 5 can be written as This number is called the real part of 5 and the imaginary part of 5. It is important to remember that these two parts of 5 are not the
Further reading: Is Z The Same As N?
Recommended Reading: What Grade Do You Learn Algebra
What Is Set Theory In Maths
As we have already discussed, in mathematics set theory, a set is a collection of different types of objects, and collectively itself is called an object. For example, number 8, 10, 15, 24 are the 4 distinct numbers, but when we put them together, they form a set of 4 elements, such that, .
In the same way, sets are defined in Maths for a different pattern of numbers or elements. Such as, sets could be a collection of odd numbers, even numbers, natural numbers, whole numbers, real or complex numbers and all the set of numbers which comes in the number line.
Set Theory Maths Example
Set theory Maths has numerous applications. Lets imagine youre in a class of 24 students . You want to know the number of males and females in your class. So you begin counting: Males: 1, 2, 3, 12 females: 1, 2, 3, 12 . Set theory can now be used to demonstrate this more clearly.
Here, the letter A represents your class.
As cardinality is 24.
I.e., |A|=24.
So there are 12 males and 12 females, right?
Lets show it off.
Now, F represents all females, while M represents all males.
|F|=12
|M|=12
As a result, the sets are really useful by means of classifying things.
How Do You Know If There Is No Solution
Know if there is no solution. This depends on a variety of factors, including the specific problem you are trying to solve, the available resources, and your own assumptions about the problem.
Some common methods to assess the feasibility of no solution include surveying the problem space, considering potential solutions, and interviewing participants.
Don’t Miss: What Does S Stand For In Chemistry
Is 2 A Counting Number
The answer can depend on a variety of factors. However, some experts feel that 2 A Counting Number is an accurate way to numbered items in a collection.
This number is typically used when counting objects that are of equal value, such as coins, toys, or dolls. Additionally, 2 A Counting Number can also be used when counting items in a pile, as it separates items by their number rather than their weight or size.
What Is The Difference Between Real Numbers Integers Rational Numbers And Irrational Numbers
The main difference between real numbers and the other given numbers is that real numbers include rational numbers, irrational numbers, and integers. For example, 2, -3/4, 0.5, 2 are real numbers.
- Integers include only positive numbers, negative numbers, and zero. For example, -7,-6, 0, 3, 1 are integers.
- Rational numbers are those numbers that can be written in the form of a fraction p/q, where ‘p’ and ‘q’ are integers and ‘q’ is not equal to zero. For example, -3, 0, -6, 5/6, 3.23 are rational numbers.
- Irrational numbers are those numbers that are square roots of positive rational numbers, cube roots of rational numbers, etc., such as 2, – 5, and so on.
Read Also: How To Calculate Dbe In Organic Chemistry
Correlations Can Have Different Levels Of Strength
We’ve covered some general correlations as either
- non-existent
Although those descriptions are okay, all positive and negative correlations are not all the same.
These descriptions can also be translated to numbers. A correlation value can take on any decimal value between negative one, \, and positive one, \.
A perfect zero correlation means there is no correlation.
For each type of correlation, there is a range of strong correlations and weak correlations. Correlation values closer to zero are weaker correlations, while values closer to positive or negative one are stronger correlation.
Strong correlations show more obvious trends in the data, while weak ones look messier. For example, the stronger high, positive correlation below looks more like a line compared to the weaker and lower, positive correlation.
Similarly, strongly negative correlations have a more obvious trend than the weaker and lower negative correlation.
Learn More About Foam Insulation And Air Seals
You now hopefully have more information about R-Value than you did when you started and knowledge is half the battle.
Your educational journey doesnt have to end here. Head over to our Learning Center where you can find even more information not just about foam insulation, but all things home insulation. Our YouTube channel also has some great information and of course, Foam University.
Don’t Miss: What Is Psychological Well Being
Layout Of This Article
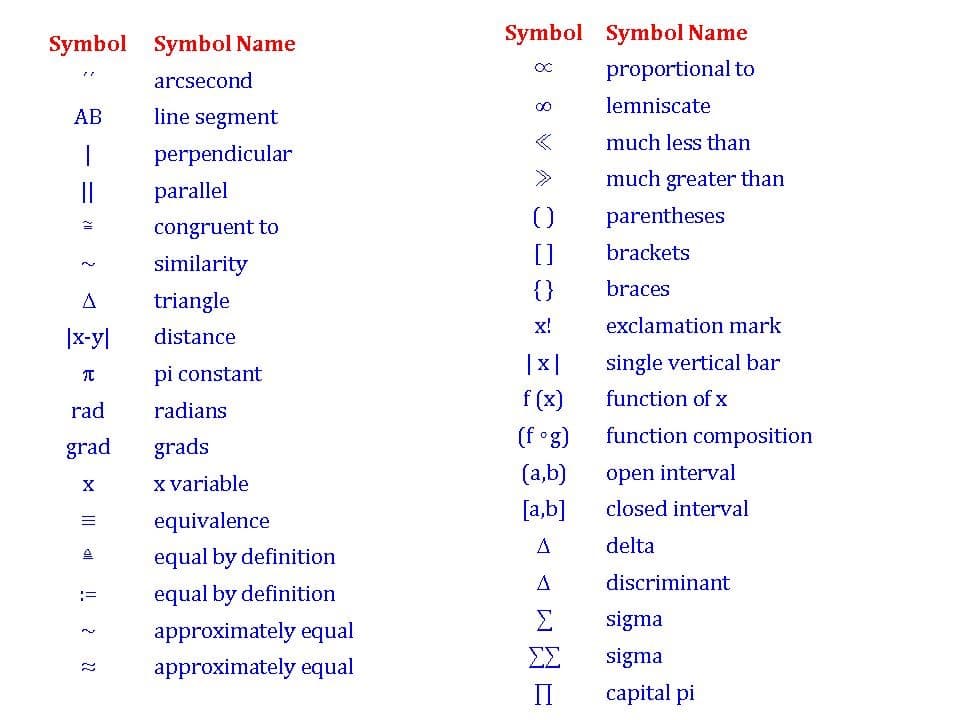
Normally, entries of a glossary are structured by topics and sorted alphabetically. This is not possible here, as there is no natural order on symbols, and many symbols are used in different parts of mathematics with different meanings, often completely unrelated. Therefore, some arbitrary choices had to be made, which are summarized below.
The article is split into sections that are sorted by an increasing level of technicality. That is, the first sections contain the symbols that are encountered in most mathematical texts, and that are supposed to be known even by beginners. On the other hand, the last sections contain symbols that are specific to some area of mathematics and are ignored outside these areas. However, the long section on brackets has been placed near to the end, although most of its entries are elementary: this makes it easier to search for a symbol entry by scrolling.
Most symbols have multiple meanings that are generally distinguished either by the area of mathematics where they are used or by their syntax, that is, by their position inside a formula and the nature of the other parts of the formula that are close to them.
As readers may not be aware of the area of mathematics to which is related the symbol that they are looking for, the different meanings of a symbol are grouped in the section corresponding to their most common meaning.
Finally, when there is an article on the symbol itself , it is linked to in the entry name.
- .
Types Of Real Numbers
We know that real numbers include rational numbers and irrational numbers. Thus, there does not exist any real number that is neither rational nor irrational. It simply means that if we pick up any number from R, it is either rational or irrational.
Rational Numbers
Any number which can be defined in the form of a fraction p/q is called a rational number. The numerator in the fraction is represented as ‘p’ and the denominator as ‘q’, where ‘q’ is not equal to zero. A rational number can be a natural number, a whole number, a decimal, or an integer. For example, 1/2, -2/3, 0.5, 0.333 are rational numbers.
Irrational Numbers
Irrational numbers are the set of real numbers that cannot be expressed in the form of a fraction p/q where ‘p’ and ‘q’ are integers and the denominator‘q’ is not equal to zero . For example, is an irrational number. = 3.14159265…In this case, the decimal value never ends at any point. Therefore, numbers like 2, -7, and so on are irrational numbers.
You May Like: What Is Induction In Physics
What Does E Mean In Math
The letter E can have two different meaning in math, depending on whether it’s a capital E or a lowercase e. You usually see the capital E on a calculator, where it means to raise the number that comes after it to a power of 10. For example, 1E6 would stand for 1 × 106, or 1 million. Normally, the use of E is reserved for numbers that would be too long to be displayed on the calculator screen if they were written out longhand.
Mathematicians use the lowercase e for a much more interesting purpose to denote Euler’s number. This number, like , is an irrational number, because it has a non-recurring decimal that stretches to infinity. Like an irrational person, an irrational number seems to make no sense, but the number that e denotes doesn’t have to make sense to be useful. In fact, it’s one of the most useful numbers in mathematics.
What Does $\mathbb^n \to \mathbb^m$ Mean And What Is $\mathbb^n$
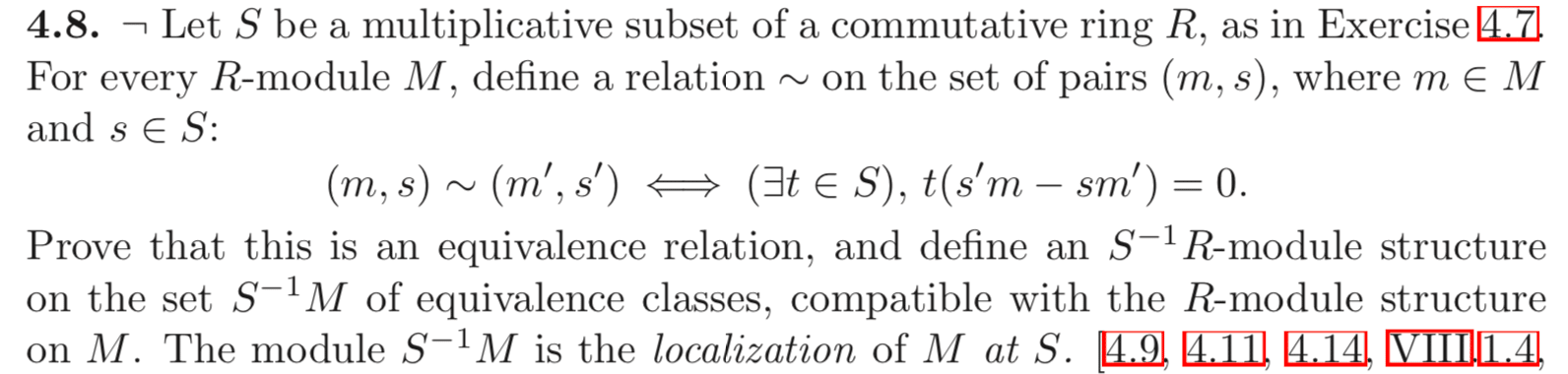
What the does $\mathbb$ mean? For example if something says that it is a transformation $T:\mathbb^2 \rightarrow \mathbb^3$. Does that mean that $\mathbb^2 = 2 \times 2$ matrix? and that $\mathbb^3 = 3 \times 3 $ matrix?
- 2$\begingroup$No. The domain of $T$ is a vector in $R^2$ and the range of $T$ is a vector in $R^3$. $T$ can be viewed as a matrix in $R^$.$\endgroup$ Xianjin YangJul 7, 2016 at 19:32
- $\begingroup$You say you don’t understand what $\mathbb^n$ means. Do you have difficulty with the real plane or real 3D space ?$\endgroup$ almagestJul 7, 2016 at 19:33
- $\begingroup$$\mathbb^n$ is the set of all points in $n$-dimensional space. $\mathbb^2$ is not a $2\times2$ matrix, but the transformation $T$ can be represented by a $3\times 2$ matrix.$\endgroup$Jul 7, 2016 at 19:33
- 1$\begingroup$@XianjinYang : “The domain of $T$ is” $\mathbb^2$ itself, not “a vector in $R^2$”. $\endgroup$ user57159Jul 7, 2016 at 19:36
- $\begingroup$@RickyDemer Thanks for the correction. I mean the elements in the domain.$\endgroup$
No, $\mathbb^2$ means the space of $2$ dimensional vectors. For example$$\pmatrix$$is an example of an element in $\mathbb^2$.
More generally $\mathbb^n$ means the space of all $n$-dimensional vectors. So, these are vectors have have $n$ coordinates.
The key thing is that $\mathbb^n$ is a vector space. All this means is that you have an addition of the vectors and you have a scalar multiplication.
for all $v,w\in \mathbb^n$ and $a$ a real number.
Don’t Miss: Electron Domain Geometry Vs Molecular Geometry