What Are Significant Digits In Chemistry

The Parable Of The Cement Block

People new to the field often question the importance of significant figures, but they have great practical importance, for they are a quick way to tell how precise a number is. Including too many can not only make your numbers harder to read, it can also have serious negative consequences.

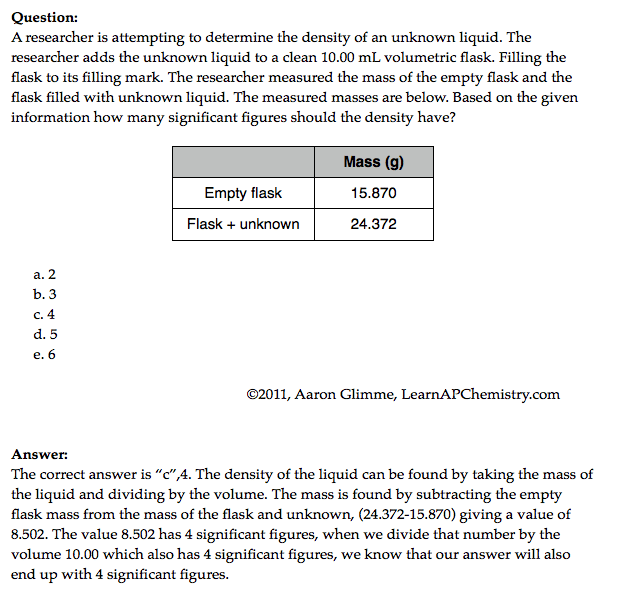
As an anecdote, consider two engineers who work for a construction company. They need to order cement bricks for a certain project. They have to build a wall that is 10 feet wide, and plan to lay the base with 30 bricks. The first engineer does not consider the importance of significant figures and calculates that the bricks need to be 0.3333 feet wide and the second does and reports the number as 0.33, figuring that a precision of ± would be precise enough for the work she was doing.

Now, when the cement company received the orders from the first engineer, they had a great deal of trouble. Their machines were precise but not so precise that they could consistently cut to within 0.0001 feet. However, after a good deal of trial and error and testing, and some waste from products that did not meet the specification, they finally machined all of the bricks that were needed. The other engineer’s orders were much easier, and generated minimal waste.

What Constitutes A Significant Figure

First, lets review these criteria that define sig figs. We can classify numbers as significant figures if they are:

• All digits comprising N are significant in accordance with the rules above
• Neither 10 nor x are significant

Specific amounts of precision, designated by significant figures, must appear in our mathematical calculations. These appropriate degrees of precision vary, corresponding to the type of calculation being completed.

To determine the number of sig figs required in the results of certain calculations, consult the following guidelines.

Significant Figures And Multiplication Or Division

In multiplication and division the number of significant figures is simply determined by the value of lowest digits. This means that if you multiplied or divided three numbers: 2.1, 4.005 and 4.5654, the value 2.1 which has the fewest number of digits would mandate that the answer be given only to two significant figures.

Also Check: What Kind Of Physics Is On The Mcat

Rules For Rounding Off

Before we move to addition, subtraction, multiplication, and division, it is necessary to understand the rounding off numbers. There are three rules which are explained below.

Rule 1

If the digit to be dropped is less than 5 , drop it and all the digits right to it. The preceding digit to dropped digit remains unchanged. The numbers which are dropped before the decimal point are replaced with zeros.

Round off number 864.73192 to 4 significant figures. To make the number to four significant number, we must drop number 3 and all the numbers right to it . Since number 3 is less than 5, the preceding number remains unchanged as per rule. Therefore, the round off figure is 864.7.

If the same number is round off to 2 significant figures, we must drop 4 and all the numbers following it . Since number 4 is less than 5, the preceding number remains unchanged. Also, 4 is replaced by 0, because it is before the decimal. The new round off number is 860.

Rule 2

If the digit to be dropped is more than 5 , drop it and all the digits right to it. And importantly, the preceding number is increased by one. The numbers which are dropped before the decimal point are replaced with zeros.

Consider rounding off the same number, 864.73192, to 6 significant figures. We have to drop number 9 and all the numbers following it. Since 9 is more than 5, preceding number 1 is up by one. Thus, the round off number is 864.732.

For 1 significant figure, the round off number will be 900.

Rule 3

What Are Significant Figures And Why Do They Matter

Significant figures tell us what amount of uncertainty we have in a reported value. The more digits you have, the more sure of yourself you are. That is why you should almost never report all the decimal places you see in your calculator.

The following is a reference for what counts as significant figures.

The following are rules for determining significant figures/digits:

NONZERO DIGITS

• All of them count, except if subscripted or past an underlined digit.

EX: has 2 significant nonzero digits.

• All digits here are significant. This is written so that the number to the left of #xx#

• Leading zeroes do NOT count.

EX: has two leading zeroes that do not matter. We could just say #7# and it numerically says the same thing.

EX: has 5 leading zeroes, none of which are significant.

• Trailing zeroes after a decimal point DO count.

EX: has 3 significant trailing zeroes .

• Trailing zeroes in a number larger than #1# that have a decimal point placed after them are still significant, but no decimal point would be ambiguous.

EX: has 3 significant zeroes, although it is better to write this as #2.color xx 10^3#

NOTE: If we write it as #1000# , we might report it as 1 significant digit, unless it is part of a unit conversion and thus exact. So, #”1000 g/kg”# does not affect significant figures in a calculation.

• Sandwiched zeroes DO count, except if no previous digits are nonzero.

EX: has two significant zeroes, but #0.01color3#

You May Like: What Is The Difference Between Alchemy And Chemistry

What Are Significant Figures

In chemistry, Significant figures are the digits of value which carry meaning towards the resolution of the measurement. They are also called significant figures in chemistry.

All the experimental measurements have some kind of uncertainty associated with them. In order to ensure precision and accuracy in measurements and get real data, a fixed method to compensate for these uncertainties was required and this led to the significant figures. Before moving on to significant figures, lets discuss the difference between precision and accuracy.

Significant Figures Chemistry

What Are The Significant Figures Rules

To determine what numbers are significant and which aren’t, use the following rules:

Don’t Miss: What Is Fe In Chemistry

Rules For Multiplication And Division

When multiplying or dividing numbers, the end result should have the same amount of significant digits as the number with the least amount of significant digits.

Example \

Y = 28 x 47.3 Find Y

Answer

Y = 1300

NOTE: 28 has 2 significant digits and 47.3 has 3 significant digits. The least amount of significant digits is 2. Thus, the answer must me rounded to 2 significant digits .

Rules For Multiplication And Division Calculations:

Also Check: What Is Adhesion In Biology

Conversion Of Significant Figures

The conversion of significant figures is easy with the use of a calculator. To do it manually, there are a few simple rules to follow.

• All non-zero digits are significant figures. The number 22.45 has four significant figures because all of its digits are non-zero.
• Zeros found between non-zero digits are significant figures. The number 2003 has 4 significant figures because the zeros are between the non-zero digits 2 and 3.
• Leading zeros are not significant figures. The number 0.14 has 2 significant figures, as does 0.014, 0.0014, and so on.
• Trailing zeros are significant figures, but only to the right of the decimal point. There are 4 significant figures in 4.000.
• Trailing zeros in a whole number are significant only if followed by a decimal point. The number 6000 only has one significant figure, but 6000. has 4.
• For a number in scientific notation N x 10x, only the digits comprising N are significant. 3.45 x 102 and 3.45 x 103 both have 3 significant figures.

: : : : : : : : : : : : : : : 24 : : : : : : : : : : : : : : :

Significant figures in derived quantities In all calculations, the answer must be governed by the least significant figure employed.

ADDITION AND SUBTRACTION: The answer should be rounded off so as to contain the same number ofdecimal places as the number with the least number of decimal places. In other words, an answer can beonly as accurate as the number with the least accuracy.Thus: 11 + 33 + 4 = 48 Rounded off to 48.

MULTIPLICATION AND DIVISION: The answer should be rounded off to contain the same number ofdigits as found in the LEAST accurate of the values.Thus: 5 x 3 = 18 Rounded off to 18.

Perform the following operations giving the proper number of significant figures in the answer:

• Access to all documents

Also Check: What Is Correlational Research In Psychology

Rules For Determining If A Number Is Significant Or Not

• All non-zero digits are considered significant. For example, 91 has two significant figures , while 123.45 has five significant figures .
• Zeros appearing between two non-zero digits are significant. Example: 101.12 has five significant figures: 1, 0, 1, 1, and 2.
• Leading zeros are not significant. For example, 0.00052 has two significant figures: 5 and 2.
• Trailing zeros in a number without a decimal are generally not significant . For example, 400 has only one significant figure . The trailing zeros do not count as significant.
• Trailing zeros in a number containing a decimal point are significant. For example, 12.2300 has six significant figures: 1, 2, 2, 3, 0, and 0. The number 0.000122300 still has only six significant figures . In addition, 120.00 has five significant figures since it has three trailing zeros. This convention clarifies the precision of such numbers. For example, if a measurement that is precise to four decimal places is given as 12.23, then the measurement might be understood as having only two decimal places of precision available. Stating the result as 12.2300 makes it clear that the measurement is precise to four decimal places .
• The number 0 has one significant figure. Therefore, any zeros after the decimal point are also significant. Example: 0.00 has three significant figures.
• Any numbers in scientific notation are considered significant. For example, 4.300 x 10-4 has 4 significant figures.

Rules For Numbers With A Decimal Point

Example \

The first two zeroes in 0.058000 are insignificant because they are before the first non-zero digit, and the last three zeroes are significant because they are after the first non-zero digit.

Example \

3 significant digits.

Read Also: Who Is Oersted In Physics

Tidiness At The End Of A Calculation

So you have carried out a calculation that requires a series of seven or eight mathematical operations and at the end, after punching everything into your calculator, you see the result “14.87569810512…”. The question you should ask yourself is how many digits to include when reporting your final answer.

It is at this point that you must refer back to the quality of the data you were given . We illustrate this here with one final example.

the samesample

Significant Figures And Units

Overview: In reporting numerical results, it is important to include the correct number of significant digits. While determining the correct number of digits to include is a straightforward process, beginning students often overlook this important detail. Here we outline the rules involved in determining the appropriate number of digits to include when reporting results of calculations and experimental measurements.

Don’t Miss: What Is Surface Tension In Chemistry

Relationship To Accuracy And Precision In Measurement

Traditionally, in various technical fields, “accuracy” refers to the closeness of a given measurement to its true value “precision” refers to the stability of that measurement when repeated many times. Hoping to reflect the way in which the term “accuracy” is actually used in the scientific community, there is a recent standard, ISO 5725, which keeps the same definition of precision but defines the term “trueness” as the closeness of a given measurement to its true value and uses the term “accuracy” as the combination of trueness and precision. In either case, the number of significant figures roughly corresponds to precision, not to accuracy or the newer concept of trueness.

Computer representations of floating-point numbers use a form of rounding to significant figures , in general with binary numbers. The number of correct significant figures is closely related to the notion of relative error .

Rules For Significant Figures

There are six rules for estimating the significant figures in which are states below.

Rule 1

All non-zero digits are significant whether the number is without the decimal point or not.

Rule 2

Zeros between two non-zero digits are significant. In other words, entrapped zeros are significant.

Rule 3

All zeroes preceding the first non-zero digit are insignificant.

Rule 4

If a number has the decimal point, all the zeros at the end are significant.

Rule 5

When a number is without the decimal point, all zeros at the end may or may not be significant. Consider a number 1400, It may have 2, 3, or 4 significant figures. It is not possible to estimate whether the number is certain up to ± 1, ± 10, or ± 100.

This ambiguity can be overcome by writing a number in scientific notations . All digits in scientific notations are significant . The above example of sodium chloride in scientific notations is as follows.

Don’t Miss: What Does Flaccid Mean In Biology

Rounding To Significant Figures

Rounding to significant figures is a more general-purpose technique than rounding to n digits, since it handles numbers of different scales in a uniform way. For example, the population of a city might only be known to the nearest thousand and be stated as 52,000, while the population of a country might only be known to the nearest million and be stated as 52,000,000. The former might be in error by hundreds, and the latter might be in error by hundreds of thousands, but both have two significant figures . This reflects the fact that the significance of the error is the same in both cases, relative to the size of the quantity being measured.

To round a number to n significant figures:

In UK personal tax returns, income is rounded down to the nearest pound, whilst tax paid is calculated to the nearest penny.

• x has only one or two significant figures as more precise uncertainty has no meaning.
• 1.79 ± 0.06 , 1.79 ± 0.96 , 1.79 ± 1.96 .

How To Count Significant Figures In Chemistry

You ask your friend how much cash he has on him. Is it different if he says around $80 versus around $76? He sounds surer of his estimate at $76, right?

A piece of mail weighs 14 g on your kitchen scale. You weigh the same piece of mail with a lab balance and get 14.46 g. Which scale gives the mass with greater certainty? The lab scale.

The higher the certainty of a measurement, the more accurate or precise it is. We usually use the words accuracy and precision interchangeably, but they have different meanings in the context of scientific measurements. Accuracy is how close a measurement is to the correct value while precision is how close the measurements are to each other.

The proper way of reporting a measured quantity is for the value to reflect the certainty of the measuring method or device. A lab scale which is capable of displaying two decimal places determines masses with greater certainty than a kitchen scale that does not show any decimal place. Another way of expressing the certainty of a value is the number is significant figures, or sig figs, for short. In the example above, $70 and $78 both have no decimal place, but $78 is more certain because it has more significant figures than $70.

Also Check: What Does The Term Geography Mean