How To Solve Inclined Plane Problems In Physics

Normal Force In Inclined Planes

The normal force in an inclined plane is not directed in the direction that we are accustomed to. Up until now, we have always seen normal force directed upwards in the direction opposite to the force of gravity. The truth about normal forces is that they are not always upwards, but rather that they are always directed perpendicular to the surface that the object is on.

Inclined Plane Formulas For A Cubic Block

While working out problems of these type, it is always worth finding forces which act on our body:

The resultant force F along the inclined plane can be worked out as a difference between Fi and Ff and thus rewritten asF = Fi – Ff = Fg *

One important note: the above expression of the net force is only valid if the angle of the inclined plane is not greater than the angle of frictionf, which can be estimated as tan = f. Otherwise, the friction force compensates Fi and the object stays at rest.

With the known expression of the resultant force, it is a piece of cake to find acceleration a, sliding time t, and final velocityV, using formulas from the acceleration calculator and the value of initial velocity V:

• a = F / m
• t = – V) / a
• V = V + a * t

If the object starts moving without initial velocity, the expression for sliding time simplifies to:

• t = .

Working With Inclined Planes

We discussed previously that when an object rests on a horizontal surface, there is a normal force supporting it equal in magnitude to its weight. Up until now, we dealt only with normal force in one dimension, with gravity and normal force acting perpendicular to the surface in opposing directions . Now that you have the skills to work with forces in two dimensions, we can explore what happens to weight and the normal force on a tilted surface such as an inclined plane. For inclined plane problems, it is easier breaking down the forces into their components if we rotate the coordinate system, as illustrated in Figure 5.34. The first step when setting up the problem is to break down the force of weight into components.

Don’t Miss: Does Kamala Harris Have Any Biological Children

Problems With Work On An Inclined Plane

A constant force $F_a$ of magnitude $82.0N$ is applied to a $3.00 kg$ shoe box at angle $53.0^$, causing the box to move up a frictionless ramp at constant speed. How much work is done on the box by $F$ when the box has moved a through vertical distance $h = 0.150 m$?

Without writing the full solution provided by my lecturer, why is the work only calculated by the product of $F_$ and the vertical distance of $0.150m$?

$i.e.$ $W = mgdcos\theta = – = -4.41J$

Why isn’t the vertical component of $F_a$ and the normal force, $F_N$ considered as well?

$i.e.$ W = $)dcos\theta$

• $\begingroup$Please see our guide on writing good titles.$\endgroup$ user10851

The solution to this problem needs to take into account the fact that the ramp is frictionless, and the mass is moving at constant speed. None of the solutions presented so far have taken this into account. If $\theta$ is the angle that the ramp makes with the horizontal, the component of F along the ramp is $F$. If the box is moving at constant speed, the net force on the box in the direction tangent to the ramp must be zero. So,$$F=mg\sin\theta$$From this equation it follows that $$\cot\theta=\frac\tag$$

The vertical component of the force F is $F\cos53$, and the vertical displacement is h.

The horizontal component of the force F is $F\sin53$, and the horizontal displacement is $h\cot\theta$

Solving An Inclined Plane Problem

In the presence of friction and other forces such as applied force and tensional force, it gets slightly complicated.

In the given diagram, the perpendicular component of the force balances the normal force. To find the net force, all the forces must be added. The perpendicular component and the normal force sum up to 0 N. The parallel component and the friction force add together to yield 5 N. The net force is 5 N, directed along the incline towards the floor.

Let us consider another example.

The free-body diagram shows the forces acting upon a 100-kg crate that is sliding down an inclined plane. The plane is inclined at an angle of 30 degrees. The coefficient of friction between the crate and the incline is 0.3. Determine the net force and acceleration of the crate.

Solution:

You May Like: What Is The Molecular Geometry Of Ccl4

Problem: Two Blocks In Contact Are Pushed Up An Inclined Plane With A Force 9mg Which Is Parallel To The Plane The Force Pushes On The Lower Block Which Has Mass 5m The Upper Block Has Mass 4m The Plane Is Inclined At An Angle With Respect To The Vertical What Is The Magnitude Of The Force On The Lower Block Exerted By The Upper Block1 9mg2 5mg 3 5mg 4 4mg5 5mg Cos 6 5mg7 4mg Cos 8 4mg 9 4mg

FREE Expert Solution

Frequently Asked Questions

What scientific concept do you need to know in order to solve this problem?

Our tutors have indicated that to solve this problem you will need to apply the Inclined Planes concept. You can view video lessons to learn Inclined Planes. Or if you need more Inclined Planes practice, you can also practice Inclined Planes practice problems.

How long does this problem take to solve?

Our expert Physics tutor, Jeffery took 7 minutes and 53 seconds to solve this problem. You can follow their steps in the video explanation above.

What professor is this problem relevant for?

Based on our data, we think this problem is relevant for Professor Criss’ class at USF.

• SUBJECTS

Physics Inclined Plane Question

An inclined plane is slowly lifted and has a mass of 10-kg resting on it. At exactly 34 degrees, the mass breaks loose and starts to slide down the plane. What is the coefficient of friction? Is this the static or kinetic coefficient?

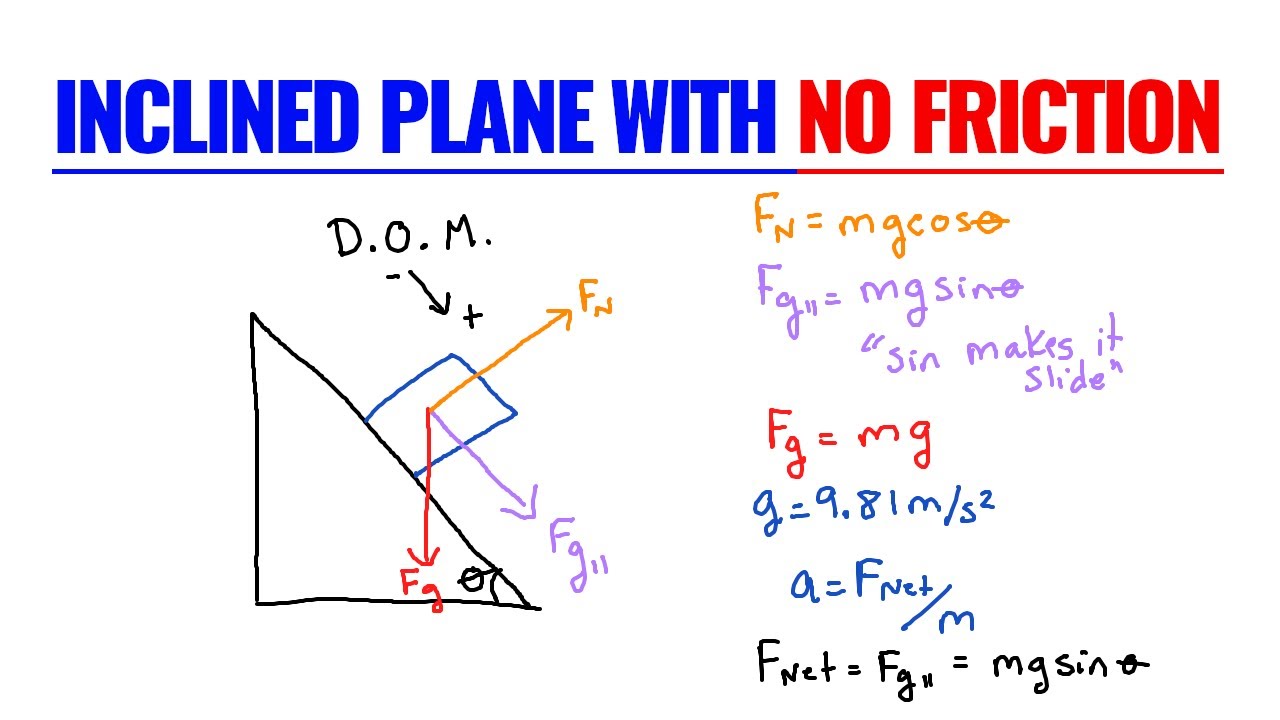
In all inclined plane problems, the Fg force due to gravity will be perpendicular to ground, while Fn Normal force will be perpendicular to the inclined plane.

In this case, since the tendency of the object to move down, the Ff Force due to Friction will be along the inclined plane upwards.

To solve for Normal, equate sum of all the forces in the direction perpendicular to plane to zero:

Fn – mg cos = 0 where x = angle of the inclined plane.

max Frictional force = µ * Fn = µ * m * g * cos

The component of the force of gravity along the plane = m * g * sin

When this is equal to max Frictional Force, the object is ready to move.

m * g * sin = µ * m * g * cos

sin/cos = µ

therefore µ = tan

This is static coefficient. The object has not started moving. When it starts moving the kinetic coefficient comes into play and the object will start moving down with increased acceleration.

If you have questions, contact me at 919 345 8889

You May Like: What Is Figure Ground Perception Psychology

Static Friction And Kinetic Friction

Recall from the previous chapter that friction is a force that opposes motion, and is around us all the time. Friction allows us to move, which you have discovered if you have ever tried to walk on ice.

There are different types of frictionâkinetic and static. Kinetic friction acts on an object in motion, while static friction acts on an object or system at rest. The maximum static friction is usually greater than the kinetic friction between the objects.

What Is An Inclined Plane

An inclined plane can be described as a flat surface which is lifted at one side so that it forms an angle with the ground. You can find the examples of inclined planes in everyday life, such as ramps or door wedges. A funicular is a type of transport vehicle which makes use of a concept of the inclined plane as well. The idea behind the simplicity and usefulness of the inclined plane is to reduce a force required to lift a body at some height.

You May Like: Hawkes Learning System Software

Basic Parameters Of The Inclined Plane

There are a few characteristics which can adequately describe a simple inclined plane. The primary one is a slope associated with already mentioned angle . The next ones are height which is the maximal level above the ground and length – the distance between the apex and the vertex at the angle . The side view of an inclined plane can be presented as a right triangle, so you can easily find a relationship between H, L, and if needed. Friction coefficient is another feature of the inclined plane, and it denotes the existence of a braking force which affects the body in motion or prevents the object from moving at all.

How Does Friction Affect Inclined Planes

Like an incline, a lever is also a simple machine that helps us with lifting heavy loads. But, how does it achieve this? What are the parts of a lever? How do they work? Watch the video to find answers to all your questions.

Stay tuned to BYJUS and FALL IN LOVE WITH LEARNING!

Put your understanding of this concept to test by answering a few MCQs. Click Start Quiz to begin!

Select the correct answer and click on the Finish buttonCheck your score and answers at the end of the quiz

You May Like: Lesson 4.5 Practice B Geometry Answers

Can Someone Please Explain To Me How To Do Question 2 Thanks

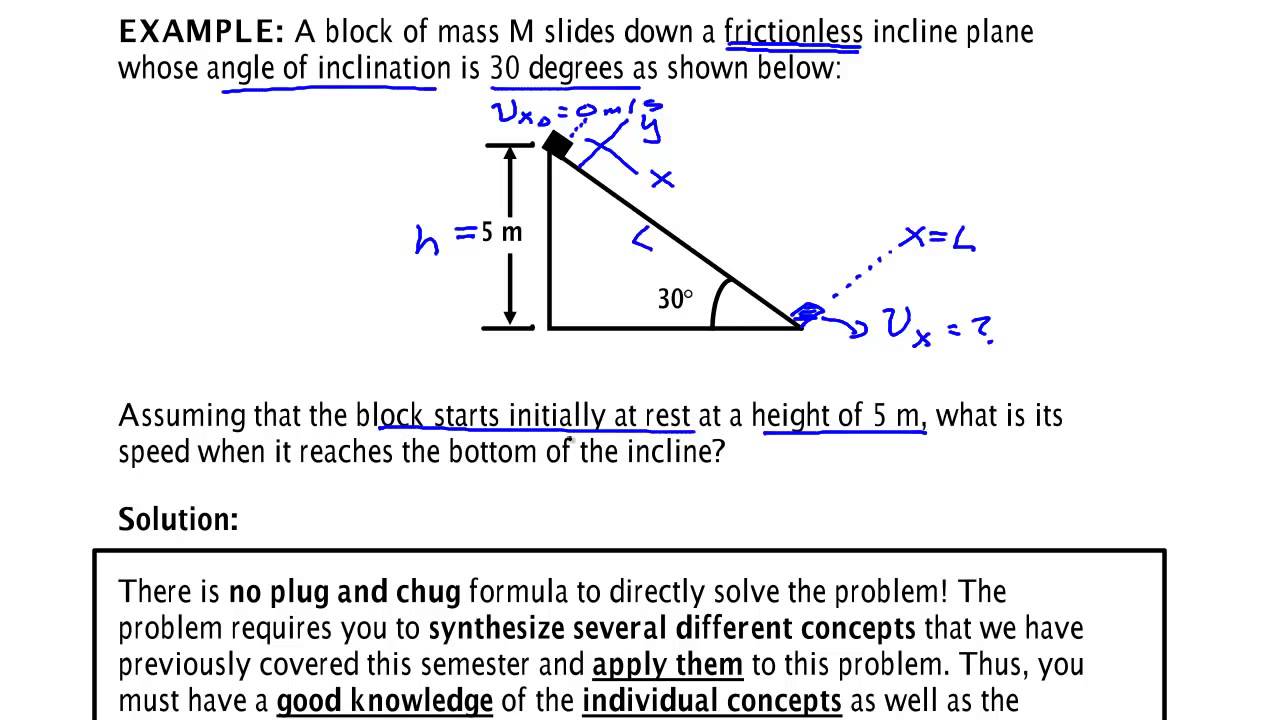
#v = 14 ” m/s”#

Explanation:

Funnily enough, this is not actually a force problem at all, as you might think. It’s a conservation of energy problem.

Let’s set up a diagram for this:

So, you can set up a conservation of energy equation:

#KE_i + PE_i = KE_f + PE_f#

Now, you plug in everything from he diagram. Notice that #KE_i = 0# , since you start at rest. Similarly, #PE_f = 0# since you end up at #H=0#

Notice that mass cancels out:

#=> cancelgH = 1/2cancelv^2#

Now, all you do is solve for #v#

#v = sqrt#

#v = sqrt) = color#

How would velocity vary if the mass were different? Well, look back, and recall that mass cancels out. Hence, ideally, the velocity would not change with mass! So whether you have an ant, a tennis ball, an elephant, or even a Soviet Tank, it would have a velocity of 14 m/s if it were sliding down a frictionless slope.

The reason this doesn’t happen in real life is because there is usually friction involved, and the strength of friction is proportional to normal force, which increases with mass.

Hope that helped 🙂

Rotary Solids On An Inclined Plane

It isn’t difficult to imagine some round object which would rather roll down instead of sliding, thus different approach has to be adopted for rotary bodies. This time friction prevents objects from slippage and simultaneously allows the rotation. We can repeat the calculating process from the previous section, taking into account both progressive and circular motions, which is quite tricky, but on the other hand, we can use the conservation of energy. It tells us that the sum of initial potential and kinetic energies equals the final kinetic energy. It’s essential to remember that rotational kinetic energy is fixed in total kinetic energy. The acceleration formula changes as follows:

• a = Fi / ,

where I is the object’s moment of inertia and r is the radius between the axis of rotation and the surface of the inclined plane, which is usually equivalent to the body’s radius . The remaining expressions for rolling time t and final velocity V are exactly the same as previously.

Don’t Miss: Segment Addition Postulate Practice Answer Key