Difference Between Variable And Parameter
May 31, 2012
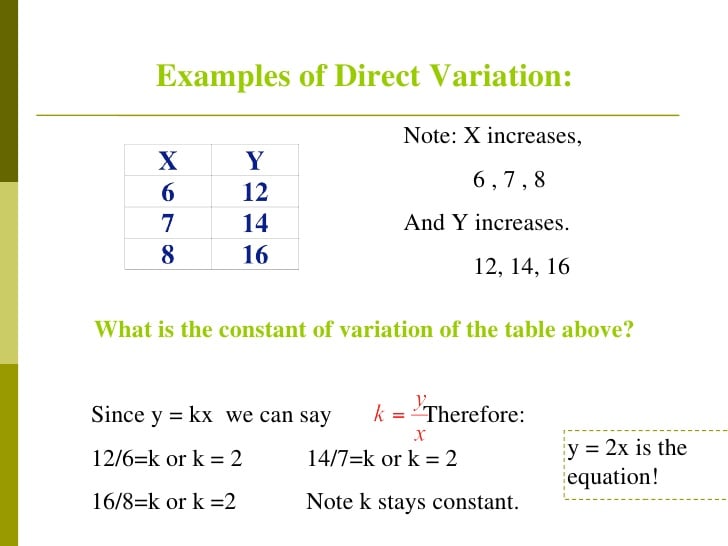
Variable vs Parameter ;
Variable and parameter are two terms widely used in mathematics and physics. These two are commonly misunderstood as the same entity. A variable is an entity that changes with respect to another entity. A parameter is an entity which is used to connect variables. The concepts of variable and parameter are very important in fields such as mathematics, physics, statistics, analysis and any other field that has usages of mathematics. In this article, we are going to discuss what variable and parameter are, their definitions, the similarities between variable and parameter, the applications of variable and parameter, some common usages of variable and parameter, and finally the difference between variable and parameter.
Variable
A variable is an entity which changes in a given system. Consider a simple example of a moving particle through space. In such a case, entities such as time, distance travelled by the particle, the direction of travelling are called variables.
Variables can also be categorized as discrete variables and continuous variables. This classification is mostly used in mathematics and statistics. Problems can be categorized depending on the number of variables. The number of variables is very important in fields such as differential equations and optimization.
Parameter
Variable vs Parameter
- A variable is a real world value with a measureable quantity whereas a parameter is an entity that we may or may not be able to measure.
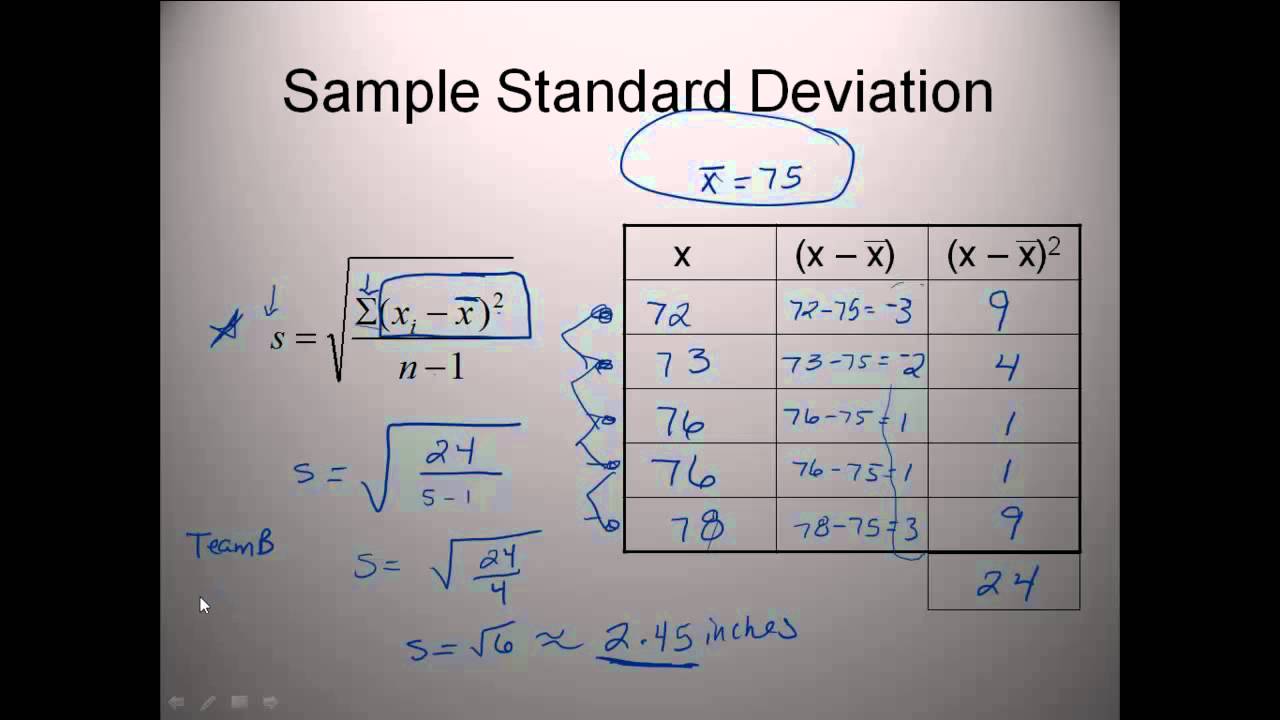
Why Use N 1 For Sample Standard Deviation
Samples are used to make statistical inferences about the population that they came from.
When you have population data, you can get an exact value for population standard deviation. Since you collect data from every population member, the standard deviation reflects the precise amount of variability in your distribution, the population.
But when you use sample data, your sample standard deviation is always used as an estimate of the population standard deviation. Using n in this formula tends to give you a biased estimate that consistently underestimates variability.
Reducing the sample n to n 1 makes the standard deviation artificially large, giving you a conservative estimate of variability.
While this is not an unbiased estimate, it is a less biased estimate of standard deviation: it is better to overestimate rather than underestimate variability in samples.
The difference between biased and conservative estimates of standard deviation gets much smaller when you have a large sample size.
What Is The Cause Of Variation
Definition of Common Cause Variation: Common cause variation is fluctuation caused by unknown factors resulting in a steady but random distribution of output around the average of the data. It is a measure of the process potential, or how well the process can perform when special cause variation removed.
Read Also: Geometry Segment Addition Postulate Worksheet
Biased Versus Unbiased Estimates Of Variance
An unbiased estimate in statistics is one that doesnt consistently give you either high values or low values it has no systematic bias.
Just like for standard deviation, there are different formulas for population and sample variance. But while there is no unbiased estimate for standard deviation, there is one for sample variance.
If the sample variance formula used the sample n, the sample variance would be biased towards lower numbers than expected. Reducing the sample n to n 1 makes the variance artificially larger.
In this case, bias is not only lowered but totally removed. The sample variance formula gives completely unbiased estimates of variance.
So why isnt the sample standard deviation also an unbiased estimate?
Thats because sample standard deviation comes from finding the square root of sample variance. Since a square root isnt a linear operation, like addition or subtraction, the unbiasedness of the sample variance formula isnt carried over the sample standard deviation formula.
The Interquartile Range And Other Percentiles
The interquartile range is the middle half of the data. To visualize it, think about the median value that splits the dataset in half. Similarly, you can divide the data into quarters. Statisticians refer to these quarters as quartiles and denote them from low to high as Q1, Q2, and Q3. The lowest quartile contains the quarter of the dataset with the smallest values. The upper quartile contains the quarter of the dataset with the highest values. The interquartile range is the middle half of the data that is in between the upper and lower quartiles. In other words, the interquartile range includes the 50% of data points that fall between Q1 and Q3. The IQR is the red area in the graph below.
The interquartile range is a robust measure of variability in a similar manner that the median is a robust measure of central tendency. Neither measure is influenced dramatically by outliers because they dont depend on every value. Additionally, the interquartile range is excellent for skewed distributions, just like the median. As youll learn, when you have a normal distribution, the standard deviation tells you the percentage of observations that fall specific distances from the mean. However, this doesnt work for skewed distributions, and the IQR is a great alternative.
Ive divided the dataset below into quartiles. The interquartile range extends from the low end of Q2 to the upper limit of Q3. For this dataset, the range is 21 39.
You May Like: Ccl4 Electron Geometry
Which Is Bestthe Range Interquartile Range Or Standard Deviation
First off, you probably notice that I didnt include the variance as one of the options in the heading above. Thats because the variance is in squared units and doesnt provide an intuitive interpretation. So, Ive crossed that off the list. Lets go over the other three measures of variability.
When you are comparing samples that are the same size, consider using the range as the measure of variability. Its a reasonably intuitive statistic. Just be aware that a single outlier can throw the range off. The range is particularly suitable for small samples when you dont have enough data to calculate the other measures reliably, and the likelihood of obtaining an outlier is also lower.
When you have a skewed distribution, the median is a better measure of central tendency, and it makes sense to pair it with either the interquartile range or other percentile-based ranges because all of these statistics divide the dataset into groups with specific proportions.
For normally distributed data, or even data that arent terribly skewed, using the tried and true combination reporting the mean and the standard deviation is the way to go. This combination is by far the most common. You can still supplement this approach with percentile-base ranges as you need.
Analysts frequently use measures of variability to describe their datasets. Learn how to Analyze Descriptive Statistics in Excel.
Strengthen Understanding Of Mean Absolute Deviation
To help them, I developed a Variability in Basketball Activity and used it with my students. This activity helped to start conversations about a real life situation they could relate to. We were able to talk about the variability in the basketball scores quite naturally. That gave more meaning to the mean absolute deviation that they were calculating.
I was very surprised to discover that developing an understanding of the concept of statistical variability is now part of the Grade 6 Common Core State Standards in math. At best, this was previously a topic in high school statistics courses and not part of middle school mathematics.
The purpose of this post is to assist you in helping your students overcome their confusion with mean absolute deviation and understand what variability is using my basketball scores concept.
I’ve created a;Variability in Basketball Activity you can download and use in the classroom. It would also be a great exercise to do during professional development days with some of your colleagues in order to strengthen your collective skills in this area.
Also please note that the names used for for this activity are gender neutral. Chris and Terry could be male or female. If you are interested in addressing students assumptions about gender, listen carefully for their use of pronouns as they discuss this activity.
Recommended Reading: Abiotic Meaning In Science
Limitations Of Descriptive Statistics
Descriptive statistics is a powerful form of research because it collects and summarizes vast amounts of data and information in a manageable and organized manner. Moreover, it establishes the standard deviation and can lay the groundwork for more complex statistical analysis.
However, what descriptive statistics lacks is the ability to:
To illustrate you can use descriptive statistics to calculate a raw GPA score, but a raw GPA does not reflect:
In other words, every time you try to describe a large set of observations with a single descriptive statistics indicator, you run the risk of distorting the original data or losing important detail.
What Is An Example Of Continuous Production
In a continuous process, as suggested by the name, the flow of material or product is continuous. Processing the materials in different equipment produces the products. Some examples of continuous processes are pasta production, tomato sauce and juice production, ice cream production, mayonnaise production, etc .
Read Also: The Segment Addition Postulate Answer Key With Work
Inspiration Pure And Applied Mathematics And Aesthetics
Mathematics arises from many different kinds of problems. At first these were found in commerce, land measurement, architecture and later astronomy; today, all sciences suggest problems studied by mathematicians, and many problems arise within mathematics itself. For example, the physicistRichard Feynman invented the path integral formulation of quantum mechanics using a combination of mathematical reasoning and physical insight, and today’s string theory, a still-developing scientific theory which attempts to unify the four fundamental forces of nature, continues to inspire new mathematics.
Some mathematics is relevant only in the area that inspired it, and is applied to solve further problems in that area. But often mathematics inspired by one area proves useful in many areas, and joins the general stock of mathematical concepts. A distinction is often made between pure mathematics and applied mathematics. However pure mathematics topics often turn out to have applications, e.g.number theory in cryptography.
As in most areas of study, the explosion of knowledge in the scientific age has led to specialization: there are now hundreds of specialized areas in mathematics and the latest Mathematics Subject Classification runs to 46;pages. Several areas of applied mathematics have merged with related traditions outside of mathematics and become disciplines in their own right, including statistics, operations research, and computer science.
What Does Variable Mean
In mathematics, a variable is a quantity that can change. Letters are used to represent these changing, unknown quantities.Einsteins famous equation E = MC2 uses the following variables:1. E for the amount of energy produced, 2. M for the amount of mass used, and 3. C2 to represent the speed of light squared. Variables, unknown quantities, are the opposite of constants, which are known, unchanging amounts.
Read Also: Geometry Segment Addition Postulate Worksheet
Genesis And Evolution Of The Concept
In the 7th century, Brahmagupta used different colours to represent the unknowns in algebraic equations in the Brhmasphuasiddhnta. One section of this book is called “Equations of Several Colours”.
At the end of the 16th century, François Viète introduced the idea of representing known and unknown numbers by letters, nowadays called variables, and the idea of computing with them as if they were numbersin order to obtain the result by a simple replacement. Viète’s convention was to use consonants for known values, and vowels for unknowns.
In 1637, René Descartes “invented the convention of representing unknowns in equations by x, y, and z, and knowns by a, b, and c“. Contrarily to Viète’s convention, Descartes’ is still commonly in use. The history of the letter x in math was discussed in a 1887 Scientific American article.
Starting in the 1660s, Isaac Newton and Gottfried Wilhelm Leibniz independently developed the infinitesimal calculus, which essentially consists of studying how an infinitesimal variation of a variable quantity induces a corresponding variation of another quantity which is a function of the first variable. Almost a century later, Leonhard Euler fixed the terminology of infinitesimal calculus, and introduced the notation y = f for a function f, its variablex and its value y. Until the end of the 19th century, the word variable referred almost exclusively to the arguments and the values of functions.
- (
What Is An Independent Variable In Math With Example
As the name itself suggests:;
independent => that doesnt seem dependent on anything.;
In most cases, it has been seen that the independent variable is called the manipulated variable. The independent variables changes are not affected by any other kinds of variables. That is why we can say that either the changes can occur in the experiment on its own or the scientist can change the independent variables.
You May Like: Math Caching
Lets Take The Most Common Example Of An Independent Variable:
It is easy to understand that time and age are the best examples of the independent variable. You can do nothing with time and age; neither you decrease or increase the value of time and age.
| Key points:;An independent variable is one of the variables that use to represent the quantity, which is manipulated within the experiment.X is the most used variable that represents the equations independent variable. |
Are Variables In Computer Programming The Same As That Of Mathematics
No!! Not at all.
The variables in computer programming are assigned to be a data type, such as alphabet, array, numeric, image, or video clips. Therefore, you must know what is variable in math and what is variable in computer programming.
The variables are used for assigning the memory location. The data at different locations can vary at the time of execution of the programs.;
But whenever the variable is encountered within the program, the computer can substitute the information at the right memory location for the specialized variable.
You May Like: What Is The Lewis Dot Structure For Ccl4
Solving Measures Of Variability Worksheets
This is a fantastic bundle which includes everything you need to know about Solving Measures of Variability across 15+ in-depth pages. These are ready-to-use Common core aligned Grade 6 Math worksheets.Each ready to use worksheet collection includes 10 activities and an answer guide. Not teaching common core standards? Dont worry! All our worksheets are completely editable so can be tailored for your curriculum and target audience.
Calculating Variability: The Basketball Example
About the line plot
Chris and Terry are basketball players. After the first eight games of the season, the coach analyzes their performance and creates a line plot of the number of baskets each player scored during these games.
After the first eight games of the seasons, they have made the following number of baskets:
Analyzing the line plot
If you calculate the measures of center using the mean and the median, you will find that Chris and Terry have mean of 9.5 baskets per game and a median of 9.5 baskets per game. By only looking at these measures of center without looking at the line plot, we might assume that these players perform equally well on the basketball court.
However, this is not the case. In fact, the players are quite different, and having students explain how they are different can help promote an understanding of how to describe variability. Terry is a very consistent player, scoring either nine or 10 baskets every game. Chriss results on the court have been much more variable. Sometimes Chris has a great game, and sometimes not. Chriss scores are spread out, while Terrys scores are all close to the mean and the median.
Also Check: Holt Geometry Lesson 4.5 Practice B Answers
How Do You Write The Independent And Dependent Variables In An Equation
You all might know that linear regressions for the two random variables depend on the linear equation. And this linear equation must consist of a single independent variable.
We write linear equation as:
y = a+b*x,;
=> In this equation, a and b are two constant numbers. Moreover, y is the dependent variable, and x is the independent variable.
If you draw the linear equation y = a+b*x, it will be a straight line.
NOTE: Keep in mind that you can select a value for substituting the independent variable. After this, it becomes quite easy to solve the dependent variable value.
Example Of Calculating The Sample Variance
Ill work through an example using the formula for a sample on a dataset with 17 observations in the table below. The numbers in parentheses represent the corresponding table column number. The procedure involves taking each observation , subtracting the sample mean to calculate the difference , and squaring that difference . Then, I sum the squared differences at the bottom of the table. Finally, I take the sum and divide by 16 because Im using the sample variance equation with 17 observations . The variance for this dataset is 201.
Because the calculations use the squared differences, the variance is in squared units rather the original units of the data. While higher values of the variance indicate greater variability, there is no intuitive interpretation for specific values. Despite this limitation, various statistical tests use the variance in their calculations. For an example, read my post about the F-test and ANOVA.
While it is difficult to interpret the variance itself, the standard deviation resolves this problem!
Read Also: Geometry Basics Segment Addition Postulate Answer Key