Factoring The Gcf From An Expression
And no, I don’t mean factoring the expression of your boss as you tell him you accidentally flooded the break room with coffee.Algebraic expressions consist of numbers, which are called coefficients, and variables, which can be raised to a power. In the expression x^2 + 6x + 8, 1 is the coefficient of x^2, the variable. Likewise, 6 is a coefficient of x^1. 8 is called a constant – it is not multiplied by a variable. .To factor an expression, you have to start by factoring out the GCF, or Greatest Common Factor. List the factors of each component of the expression. Here we are interested in finding the natural number factors.The expression x^2 + 6x + 8 would have factors that look like this:x^2: 1
What Is A Factor
A factor is a number that divides another number, leaving no remainder. In other words, if multiplying two whole numbers gives us a product, then the numbers we are multiplying are factors of the product because they are divisible by the product.
There are two methods of finding factors: multiplication and division. In addition, rules of divisibility may also be used.
Example: Let us consider the number 8. 8 can be a product of 1 and 8, and 2 and 4. As a result, the factors of 8 are 1, 2, 4, 8. Therefore, when finding or solving problems involving factors, only positive numbers, whole numbers, and non-fractional numbers are considered.
A general formula to remember is that a and b are factors of abs product.
2 3 = 6. Therefore, 2 and 3 are factors of 6. There is no remainder when 6 is divided by either 2 or 3. 9 3 = 27. Therefore, 9 and 3 are factors of 27. There is no remainder when 27 is divided by either 9 or 3. 7 5 = 35. Therefore, 5 and 7 are factors of 35. There is no remainder when 35 is divided by either 5 or 7.
Example: Find all the factors of the number 10.
Factor That Mamma Jamma
Factoring the rescue. My intuition: factoring lets us re-arrange a complex system as a bunch of linked, smaller systems.
Imagine taking a pile of sticks and standing them up so they support each other, like a teepee:
Remove any stick and the entire structure collapses. If we can rewrite our system:
as a series of multiplications:
we’ve put the sticks in a “teepee”. If Component A or Component B becomes 0, the structure collapses, and we get 0 as a result.
Neat! That is why factoring rocks: we re-arrange our error-system into a fragile teepee, so we can break it. We’ll find what obliterates our errors and puts our system in the ideal state.
Remember: We’re breaking the error in the system, not the system itself.
Read Also: What Is Content Validity In Psychology
How To Factor A Trinomial Example #2
Lets get more practice factoring trinomials when a is 1.
Factor: x^2 + 9x + 20
Step 1: Identify the values for b and c.
In this example, b=9 and c=20.
Step 2: Find two numbers that ADD to b and MULTIPLY to c.
Finding the right numbers wont always be as easy as it was in example 1.
To make factoring trinomials easier, write down all of the factors of c that you can think of.
In this case, c=20, so:
20 x 1 = 20
Remember that the two numbers have to multiply to c AND add to b.
The only factors of 20 that meet both of these requirements are 4 and 5.
Step 3: Use the numbers you picked to write out the factors and check
The last step is to write out the factors:
Reconfigure Your Expression To Multiply Your Greatest Common Factor Back In
Remember, when you are factoring a binomial you are looking for simplified expressions that combine to make that binomial expression. That means that all of the numbers that are removed from the expression eventually have to return, in a different order. If you divide 9x + 6 by 3 to get 3x + 2, you’ll need to reincorporate 3 using multiplication. In this example, it would go at the beginning, giving you a final factored result of 3.
Related:Algebraic Mathematical Equations: Definitions, Types and Examples
Read Also: What Is Physical Geography For Kids
What Is The Prime Factorization Of A Given Number
The prime factorization of a number is the product of all the prime-number factors of a given number, including the number of times each of the primes is a factor. The prime factorization does not include 1, but does include every copy of every prime factor. When factoring a number, you most often want to find the “prime factorization” of that number. For instance, the prime factorization of 8 is 2×2×2, not just “2”. Yes, 2 is the only factor, but you need three copies of it to multiply back to 8, so the prime factorization includes all three copies.
prime factors of 8: 2
prime factorization of 8: 2 × 2 × 2 = 23
Affiliate
On the other hand, the prime factorization includes ONLY the prime factors, not any products of those factors. For instance, even though 2 × 2 = 4, and even though 4 is a divisor of 8, 4 is NOT in the PRIME factorization of 8. That is because 8 does NOT equal 2 × 2 × 2 × 4.
This accidental over-duplication of factors is another reason why the prime factorization is often best: it avoids counting any factor too many times.
Suppose that you need to find the prime factorization of 24. Sometimes a student will just list all the divisors of 24: 1, 2, 3, 4, 6, 8, 12, and 24. Then the student will do something like make the product of all these divisors:
1 × 2 × 3 × 4 × 6 × 8 × 12 × 24
In the case of 24, you can find the prime factorization by taking 24 and dividing it by the smallest prime number that goes into 24: 24 ÷ 2 = 12.
Factoring Mathematical Expressions: Why It’s Important And How To Do It
The Indeed Editorial Team comprises a diverse and talented team of writers, researchers and subject matter experts equipped with Indeed’s data and insights to deliver useful tips to help guide your career journey.
Being able to reverse engineer an equation into parts can be an important skill in many business operations. Breaking certain kinds of mathematical equations down into their parts is called factoring. This process can be especially useful in jobs that involve data analysis and application because people in these roles often handle figures that other people have already processed. In this article, we define factoring, discuss some methods for factoring and how to use it and provide a few example equations.
Read Also: Geometry Circles Chapter Test Review
How To Use The Quadratic Equation
One particular kind of equation that uses trinomial expressions is a quadratic equation. This equation sets the polynomial ax2 + bx + c equal to zero, and it has a variety of practical applications that make factoring a practical skill to know. Quadratics are used to calculate area, profits, speed and other useful information. Try these steps for using the quadratic equation:
Types Of Factoring Polynomials
There are six different methods to factorising polynomials. The six methods are as follows:
- Greatest Common Factor
- Sum or difference in two cubes
- Difference in two squares method
- General trinomials
- Trinomial method
In this article, let us discuss the two basic methods which we are using frequently to factorise the polynomial. Those two methods are the greatest common factor method and the grouping method. Apart from these methods, we can factorise the polynomials by the use of general algebraic identities. Similarly, if the polynomial is of a quadratic expression, we can use the quadratic equation to find the roots/factor of a given expression. The formula to find the factors of the quadratic expression is given by:
Read Also: What Does Mks Stand For In Physics
How To Factor A Trinomial Example #1
For the first example, lets factor the trinomial: x^2 + 6x + 8
Again, note that a=1 in this example.
Now lets factor the trinomial:
Step 1: Identify the values for b and c.
In this example, b=6 and c=8.
Step 2: Find two numbers that ADD to b and MULTIPLY to c.
This step can take a little bit of trial-and-error.
For instance, you could pick 5 and 1 because 5+1=6. But 5 x 1 does not equal 8, so these numbers would not work.
However, if you chose 4 and 2, you can easily confirm that:
4 + 2 =6 and
4 x 2 = 8
Step 3: Use the numbers you picked to write out the factors and check
For this example, the factors would be and
Final Answer:
You can check your answer by multiplying the two factors together to see if the result is the original trinomial as follows:
Notice that 2x and 4x are like terms that can be combined.
Multiplying the factors results in the original trinomial.
Why Is Factoring So Useful
Factoring is used for solving quadratic equations. Some expressions can easily be factored so that the quadratic expression is rewritten as the product of two linear expressions, which we all know how to solve!
The most common factoring is using the square of the sum, the square of the difference, the difference of the squares, and factoring out the common factor.
Don’t Miss: What Is Direct Proportion In Physics
Using Relations Between Roots
It may occur that one knows some relationship between the roots of a polynomial and its coefficients. Using this knowledge may help factoring the polynomial and finding its roots. Galois theory is based on a systematic study of the relations between roots and coefficients, that include Vieta’s formulas.
Here, we consider the simpler case where two roots x
- -5x^-16x+80=.}
How To Factor A Number

wikiHow is a wiki, similar to Wikipedia, which means that many of our articles are co-written by multiple authors. To create this article, 68 people, some anonymous, worked to edit and improve it over time.There are 7 references cited in this article, which can be found at the bottom of the page. This article has been viewed 724,497 times.Learn more…
A number’s factors are numbers which multiply together to form it as a product. Another way of thinking of this is that every number is the product of multiple factors. Learning how to factor – that is, breaking up a number into its component factors – is an important mathematical skill that is used not only in basic arithmetic but also in algebra, calculus, and beyond. See Step 1 below to start learning how to factor!
You May Like: Common Core Algebra 2 Unit 12 Answer Key
What Is An Example Of Doing Prime Factorization
- Find the prime factorization of 1050.
I’ll do the upside-down division:
Reading around the outside, I find that my answer is:
1050 = 2 × 3 × 5 × 5 × 7
Some texts prefer that answers such as this be written using exponential notation, in which case the final answer would be written as 2×3×52×7.
You can do the repeated division “right-side up”, too, if you prefer. The process works the same way, but the division is reversed in orientation. The above problem would be worked out like this:
- Find the prime factorization of 1092.
I’ll do the repeated division:
Reading around the outside, I can see that the prime factorization is:
1092 = 2 × 2 × 3 × 7 × 13
This answer can also be written in exponential notation as 22 × 3 × 7 × 13.
Content Continues Below
What Are Some Divisibility Rules That Can Help With Prime Factorizations
There are many divisibility rules that can help you with prime factorizations, but the simplest to use are these:
- If the number is even, then it’s divisible by 2.
- If the number’s digits sum to a number that’s divisible by 3, then the number itself is divisible by 3.
- If the number ends with a 0 or a 5, then it’s divisible by 5.
Of course, if the number is divisible twice by 2, then it’s divisible by 4 if it’s divisible by 2 and by 3, then it’s divisible by 6 and if it’s divisible twice by 3 , then it’s divisible by 9. But since you’re finding the prime factorization, you don’t really care about these non-prime divisibility rules.
There are rules for divisibility by 7 , but you’ll probably find it to be easier to just do the division on your calculator and see if the result of the division comes out to be a whole number.
Affiliate
If you run out of small primes and you’re not done factoring, then keep trying bigger and bigger primes until you find something that works or until you reach primes whose squares are bigger than what you’re dividing into.
Don’t Miss: What Is The Electron Geometry Of Pf3
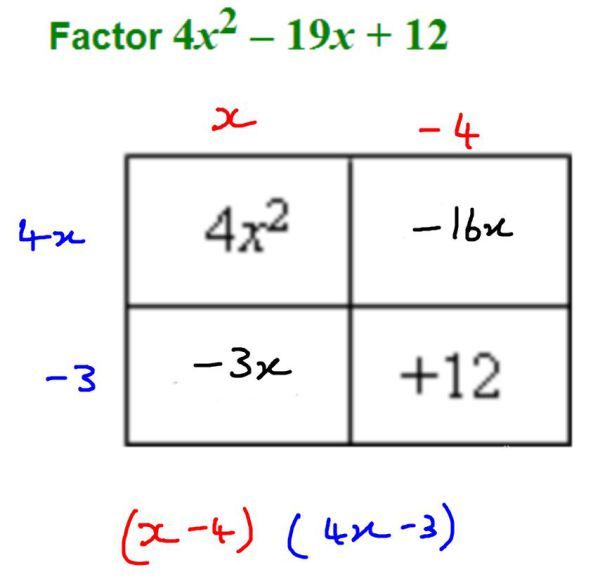
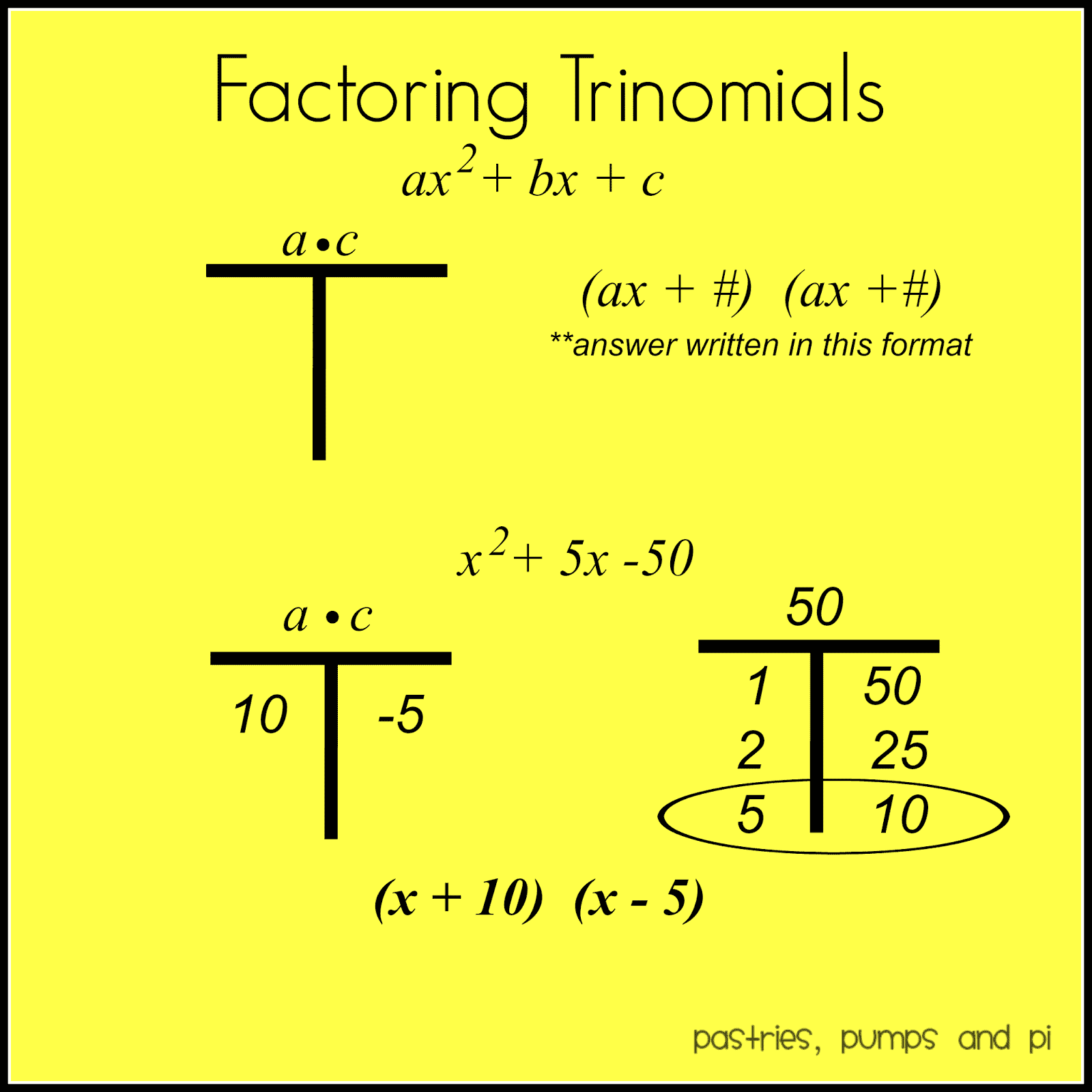
What Is Box Method
The box method is a technique used in factoring quadratic trinomials consisting of a box where you need to put the first and last terms of the quadratic polynomial.
Figure 1 shows what the box method looks like when you factor the polynomial $ax^2+bx+c$. You need to place the first and last terms in the diagonal, then you need to follow the indicated steps to solve the terms that need to be placed in the green cells. Using these cells, you will derive the terms $mx$, $px$, $n$, and $q$. Then the quadratic trinomial can be expressed as factors of $mx+n$ and $px+q$.
Factoring Polynomial With Four Terms
Let us learn how to factorize the polynomialhaving four terms. For example, x3 + x2 x 1 is the polynomial.
Break the given polynomial into two parts first.
Now find the highest common factor from both the parts and take that factor out of the bracket.
We can see, from the first part, x2 is the greatest common factor and from the second part we can take out the minus sign. Thus,
x2-1
Again, regrouping the terms as the factors.
Therefore, the factorisation of x3+ x2 x 1 gives
Don’t Miss: What Is The Difference Between Geography And Topography
History Of Factorization Of Expressions
The systematic use of algebraic manipulations for simplifying expressions ) may be dated to 9th century, with al-Khwarizmi‘s book The Compendious Book on Calculation by Completion and Balancing, which is titled with two such types of manipulation.
However, even for solving quadratic equations, the factoring method was not used before Harriot‘s work published in 1631, ten years after his death. In his book Artis Analyticae Praxis ad Aequationes Algebraicas Resolvendas, Harriot drew, tables for addition, subtraction, multiplication and division of monomials, binomials, and trinomials. Then, in a second section, he set up the equation aa ba + ca = + bc, and showed that this matches the form of multiplication he had previously provided, giving the factorization .
How To Factor Binomials
A binomial is a mathematical expression that includes two parts added together. For example, ax + b is a binomial because it has two componentsâax and bâadded together. In this example, a and b represent known numbers and x represents an unknown number, also called a variable. The first part of a binomial includes a variable, and the second part may or may not include a variable.
When you factor a binomial, you are trying to break it down into more simple expressions that you can multiply together to make the binomial expression. You can follow these steps when factoring a binomial:
Read Also: What Is The Relationship Between History And Geography
How To Factor Numbers
Factoring, in mathematics, refers to the simplification or reduction of a number, a polynomial, etc., to a result that can not be further reduced or simplified. In this post, you can learn how to factor numbers easily.
Factors are the numbers we multiply to get another number. Factoring, in mathematics, refers to the simplification or reduction of a number, a polynomial, etc., to a result that can not be further reduced or simplified. Known as factorization, this method is primarily used in polynomial simplification. In this post learn how to factor numbers easily.
Finding Factors Using Multiplication
Since multiplication of two numbers results in a product such that the two numbers become the factors of the product. Thus, to find the factors, we need to follow the below steps:
- If we need to find the factors of a number, say N, then write the multiplication of two numbers in different ways, such that the resulting value is equal to N
- All the individual numbers that results in the product equal to N are the factors
Let us see an example: What are the factors of 36?
We can find the factors of 36 using multiplication as given below:
Now we have got the repeated numbers in the multiplication. Hence, we should stop writing 36 as the product of other numbers.
Thus, the factors of 36 are 1, 2, 3, 4, 6, 9, 12, 18, and 36.
You May Like: What Is First Law Of Thermodynamics In Physics
Factoring Polynomials By Grouping
Sometimes you will get four or more terms, that look something like this:2x^2 + 6x^3 + 5x^7 + 15x^8There is no common coefficient, and factoring out x^2 doesn’t help much. This is where you would use grouping to factor.Grouping means factoring out the GCF of only two terms of the expression. You can see that 2x^2 + 6x^3 and 5x^7 + 15x^8 both can have a GCF taken out. Do so.2x^2 + 5x^7 Note that there is a common factor, 1+3x. This expression can be rephrased to . There’s your answer.Note that can be factored further by factoring out an x^2 from the first binomial: x^2 .
How To Factor A Trinomial Example #3
Factor: x^2 – 5x -24
Notice that this example includes subtraction signs, but the process of factoring is still the same.
Step 1: Identify the values for b and c.
In this example, b= -5 and c= -24.
Step 2: Find two numbers that ADD to b and MULTIPLY to c.
Again, you have to find two values to ADD to -5 and MULTIPLY to -24.
This step can get tricky when youre dealing with negative numbers. Remember that a negative times a negative is positive, so only one of the values can be negative if the product has to be -24.
After writing out all of the possible factors, you can conclude that:
-8 x 3 = -24
Step 3: Use the numbers you picked to write out the factors and check
The last step is to write out the factors:
Read Also: Which One Does Not Belong Geography