Difference Between Natural And Whole Numbers
One of the most important aspects of mathematics is numbers. We need numbers to count, measure, and label. These can be presented in different forms such as in digits, in words, and on a line. Numbers are those values that represent a particular quantity. In mathematics, numbers are of different types – Natural Numbers, Whole Numbers, Integers, Real Numbers, Rational Numbers, Irrational Numbers, and Complex Numbers.
The main difference between natural and whole numbers is the number 0 which is only a part of the set of whole numbers and not in natural numbers. In this article, we are going to learn the definition of natural numbers, the definition of whole numbers, and the difference between natural and whole numbers with examples.
| 1. |
| FAQs |
The Name Symmetric Difference
The name symmetric difference suggests a connection with the difference of two sets. This set difference is evident in both formulas above. In each of them, a difference of two sets was computed. What sets the symmetric difference apart from the difference is its symmetry. By construction, the roles of A and B can be changed. This is not true for the difference between two sets.
To stress this point, with just a little work we will see the symmetry of the symmetric difference since we see A B = = = B A.
Webster Dictionaryrate This Definition:
Differencenoun
the act of differing the state or measure of being different or unlike distinction dissimilarity unlikeness variation as, a difference of quality in paper a difference in degrees of heat, or of light what is the difference between the innocent and the guilty?
Differencenoun
disagreement in opinion dissension controversy quarrel hence, cause of dissension matter in controversy
Differencenoun
that by which one thing differs from another that which distinguishes or causes to differ mark of distinction characteristic quality specific attribute
Differencenoun
choice preference
Differencenoun
an addition to a coat of arms to distinguish the bearings of two persons, which would otherwise be the same. See Augmentation, and Marks of cadency, under Cadency
Differencenoun
the quality or attribute which is added to those of the genus to constitute a species a differentia
Differencenoun
the quantity by which one quantity differs from another, or the remainder left after subtracting the one from the other
Differenceverb
When they describe their youth and their childhood, these are young people who made mistakes that aren’t that different than the mistakes I made and the mistakes that a lot of you guys made, the difference is they did not have the kinds of support structures, the second chances, the resources that would allow them to survive those mistakes.
You May Like: Eoc Fsa Warm Ups Algebra 1 Answers
Examples Of Between In A Sentence
betweenbetweenbetweenbetweenbetweenbetweenBetweenbetweenbetweenbetweenbetween Fox Newsbetween CBS Newsbetween Los Angeles Timesbetween NBC Newsbetween Forbesbetween The New Yorkerbetween Quanta Magazinebetween Vulturebetweenchicagotribune.combetween albetweenNew York TimesbetweenThe New RepublicbetweenForbesbetweenVoguebetween ForbesbetweenNew York Times
These example sentences are selected automatically from various online news sources to reflect current usage of the word ‘between.’ Views expressed in the examples do not represent the opinion of Merriam-Webster or its editors. Send us feedback.
Between Vs Among: Usage Guide
Preposition
There is a persistent but unfounded notion that between can be used only of two items and that among must be used for more than two. Between has been used of more than two since Old English it is especially appropriate to denote a one-to-one relationship, regardless of the number of items. It can be used when the number is unspecified economic cooperation between nations , when more than two are enumerated between you and me and the lamppost partitioned between Austria, Prussia, and Russia Nathaniel Benchley , and even when only one item is mentioned . pausing between every sentence to rap the floor George EliotAmong is more appropriate where the emphasis is on distribution rather than individual relationships. discontent among the peasants When among is automatically chosen for more than two, English idiom may be strained. a worthy book that nevertheless falls among many stools John Simon the author alternates among mod slang, clichés and quotes from literary giants A. H. Johnston
Read Also: What Is The Molecular Geometry Of Ccl4
How To Use Math And Maths
The only difference between math and maths is where theyre used. Math is the preferred term in the United States and Canada. Maths is the preferred term in the United Kingdom, Ireland, Australia, and other English-speaking places.
Theres no real logical explanation as to why math became preferred in some places while maths was elsewhere. The usual argument goes that mathematics is plural because it ends in an -s, so maths should be its abbreviation. The problem is that, while it ends in an s, mathematics is a mass noun and usually takes a singular verb .
Both of these words date back to the turn of the 20th century. There are examples of math in writings from the 1840s, and of maths from the 1910s.
Why Is Term Life Better Than Whole Life Insurance
If that sounds familiar, term life insurance is your best option for affordable coverage. Because a term life insurance policy is only valid for a limited time, the premiums are significantly lower than with a life insurance policy. And if you’re young and in good health, you’ll get an even better price.
You May Like: Ccl4 Structural Formula
What Do You Do Can Make A Difference
Ten ways to improve your life in the volunteer community. Giving energy and skills to a local organization is a great way to do justice to your community. Donate blood. One donation can save many lives, but a patient may need multiple donors. Become a mentor. Organize a charity event. Buy locally. Adopt a neighbor. Attend community gatherings. Organize cleaning.
How To Solve Math
Definition of difference math Introduction:
In algebra basic arithmetic operation generally used in day to day life. Subtraction defined as the inverse of addition. . To subtract a one integer from another integer it is enough to add the additive inverse of the integer that is being subtracted, to the other integer.
Tutoring is a process of teaching by a person to another person. In the tutoring process the communication between the people is either by one to one, one to many and many to one by this way the tutoring process is handled. In this article, we are going to discuss about definition of difference math.Definition of Difference Math Subtraction Whole Number and Decimal Examples:
Definition of difference math Subtraction whole number examples:
Example 1:
Subtracting the given values,
62012 40112
Also Check: Is Ap Biology Easy
Other Differences Between British And American English
In some cases, British and American English use different words for the same concept. For example, American English speakers use the words truck, shopping cart, and sweater British English speakers say lorry, trolley, and jumper to mean the same things.
In other cases, the differences between British and American English words are much more subtle. For instance, American English uses the term racecar, while British English uses the word racing car.
In still other cases, British and American English words differ by just one letter, as in the case of math and maths. British English includes U in the spelling of French-derived words, such as colour or favourite, which American English omits.
This also happens with the words sport and sports. In American English, youd say, I enjoy playing sports, and I also like watching sports. In British English, this sentence would be I enjoy playing sport, and I also like watching sport. This time, its American English that likes the s!
What Is The Meaning Of Difference In This Question
I was given the following problem:
Let $X$ represent the difference between the number of heads and the number of tails obtained when a coin is tossed $n$ times. What are the possible values of $X$?
which already has an answer on this site. However, when I first approached this, I interpreted the word “difference” as the distance between two points lying on a horizontal/vertical line, and therefore always positive . Similar questions on the lexical use of the word in math, agree that the definition of difference is given by Big number – small number. On the other hand, Wikipedia states that difference is the result of subtraction, and therefore may be negative. At this point, I guess this is left to the interpretation of the reader, since to me the question is ambiguously phrased.
Not content, I wanted to see if there existed any probability distributions with discrete support on $\mathbb$. I found out on the Wikipedia list that only $2$ are mentioned, namely the Degenerate distribution and Rademacher distribution. Every other distribution mentioned is defined on $\mathbb$. So my question is, knowing this and what was mentioned in the paragraph above, wouldn’t the best answer only include positive values of $X$? As most problems of this type involve Binomial distributions, Geometric distributions, etc. which are defined on $\mathbb$.
You May Like: Does Mj Have Any Biological Kids
Difference Between Average And Mean
To understand the difference between average and mean, one must be aware of what separates one from the other. Average and mean are used interchangeably. In Statistics, instead of the term average, the term mean is used. Average can simply be defined as a quantity or a rate which usually fall under the centre of the data. The average is quite similar to mean but also has its key differences from mean as well. If one can understand the arithmetic mean and range, it can be incredibly helpful in understanding and solving math topics.
Definition Of Relation And Function In Maths

Relation- In maths, the relation is defined as the collection of ordered pairs, which contains an object from one set to the other set. For instance, X and Y are the two sets, and a is the object from set X and b is the object from set Y, then we can say that the objects are related to each other if the order pairs is to be in relation.
Functions- The relation that defines the set of inputs to the set of outputs is called the functions. In function, each input in the set X has exactly one output in the set Y.
Note: All functions are relations but all relations are not functions.
Consider for an example Set X & Set Y are related in a manner that all the elements of Set X are related to exactly one element of Set Y or many elements of set X are related to one element of Set y. Thus, this type of relation is said to be a function.
Note that a function cannot Possess One-to-Many relation between the set X and Y.
Recommended Reading: Lesson 4.5 Practice B Geometry Answers
Factorization Of Polynomials And Simplification Of Expressions
The formula for the difference of two squares can be used for factoring polynomials that contain the square of a first quantity minus the square of a second quantity. For example, the polynomial x can be factored as follows:
- x -1==}
As a second example, the first two terms of x -y^+x-y} can be factored as
- -y^+x-y=+x-y=}
Moreover, this formula can also be used for simplifying expressions:
- ^===4ab}
Solved Examples On Difference Between Natural And Whole Numbers
Example 1: Identify whole numbers from the given numbers 7/9, 0, 7, 69.6, 10, -21, -56, 90
Solution: From the given list, the whole numbers are 0, 7, 10, and 90
Example 2: “Every whole number is also a natural number.” Is this statement true? Justify your answer.
Solution: No, every whole number is not a natural number. By definition, natural numbers are a part of the number system that contains all positive integers starting from the number 1 to infinity. Whereas, a whole number includes all positive numbers starting from the number 0 to infinity. The number 0 is a whole number but not a natural number. But we can say that natural numbers contain all the whole numbers except the number 0. Therefore, the statement, every whole number is also a natural number is not true.
Example 3: Identify whole numbers and natural numbers from the given list 4.5, 121, 56/90, 4, -79, 0, 30
Solution: From the given list,
Read Also: What Does K Represent In Math
An Application: Radioactive Decay
That's one interpretation of f' it gives the slopes of the tangent lines to the graph of f. There are many other interpretations these may depend upon a physical interpretation of x and f. If, for example, x is time and f is the AMOUNT of radium remaining at time x , then f has the form f = C*e^,where C and k are constants depending upon the amount of radium at time 0 and upon the physical characteristics of radium. We may assume, for example, that f gives the amount of radium present at time x .
It turns out that this function form can be obtained from a differential equation, an equation that describes how the derivative is related to the function itself here we are taking it as a given.
When we know the function, we can differentiate it to find the rate of change:
f' then gives the rate at which the radium is decaying, in grams per year.You already know that the derivative of f at x is given by f' = limit as h-> 0 of the difference quotient /h. Some prefer to write this in the equivalent form f' = limit as x-> a of the difference quotient /.Graphically, the difference quotient / may be interpreted as the slope of the line joining points ) and ) on the graph of f.As x approaches a, the slope of this line approaches the slope of the tangent line to the graph of f at ), assuming that it exists.
The a here is the same as the \ in the first explanation. Observe how the two forms of the definition are related:
$$f’ = \lim_\frac$$
$$f’ = \lim_\frac$$
What Is The Difference Between Average And Mean
Average and mean are usually confused with one another as both the mathematical terms are used to explain the set of numbers. Mean can simply be calculated by adding the set of values and divided by the number of quantities. Thus, this is the core definition of what mean is. You can find the tabular column below to learn the difference between the average and mean.
| Difference between Average and Mean | |
|---|---|
|
Average |
Mean |
| Average can simply be defined as the sum of all the numbers divided by the total number of values. | A mean is defined as the mathematical average of the set of two or more data values. |
| Average is usually defined as mean or arithmetic mean. | Mean is simply a method of describing the average of the sample. |
| Average can be calculated for any discrete numbers where it assumes uniform distribution. | It is mainly used in Statistics, and it is applied for any distribution such as geometric, binomial, Poisson distribution, and so on. |
| The arithmetic mean is considered as a form of average. | There are various types of mean. |
| Average is usually used in conversations in general day to day English. | Mean is used in a more technical and mathematical sense. |
| The average is capable of giving us the median and the mode. | Mean, on the other hand, cannot give us the median or mode. |
Read Also: Geometry Segment Addition Postulate Worksheet
Creating New Definitions To Create New Problems
New definitions are an invitation to new problems. Students looking for new questions to pursue should try defining new objects, properties, or relationships. Give your class the definitions of “semicenter” and “semicentral”:
A point is a semicenter of a set if the segments connecting the point to every other point of the set lie entirely within the set. A figure with a semicenter is semicentral.
You can ask them to draw figures that are and are not semicentral. For semicentral shapes, have them identify their semicenters. If necessary, you can then use the figures below as additional test cases .
Some questions may arise in the course of seeking examples and coloring the set of semicenters. Ask sudents to write down as many new questions as they can about such figures. Here are some possibilities:
Do not expect an extensive list on the first try. If only a few questions arise at first, provide the class with some time to explore those questions. As they seek answers and further study the shapes, they are likely to pose new questions in response to their investigations. Have them share their questions and conjectures with one another as they arise, and, for settings that capture a classs imagination, the process will snowball.
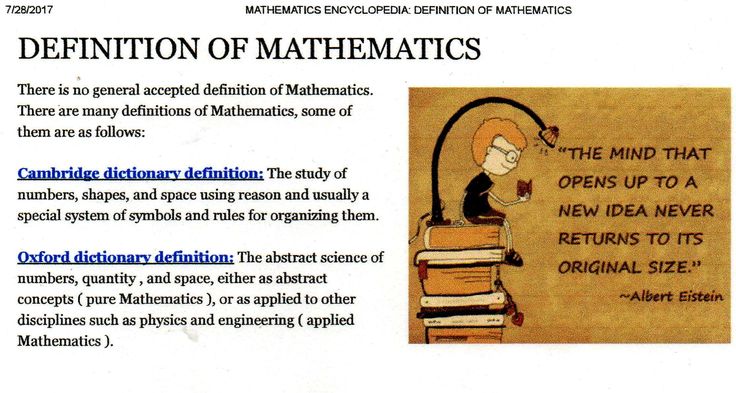
Difference Between Mathematics And Numeracy
The main difference between mathematics and numeracy is that mathematics is the broad study of numbers, quantities, geometry and forms while numeracy is ones knowledge and skills in mathematics and its use in real life.
Mathematics can be defined as the study of the measurement, properties, and relationships of quantities and sets, using numbers and symbols . Mathematics or math is not just a subject we learn at school we need a basic knowledge of numbers, quantities, shapes and forms when dealing with real-life situations. Our ability to understand simple mathematical concepts and use them in real life is called numeracy.
Recommended Reading: Founding Father Of Behaviorism
What Is The Difference Game
Spot the Differences Spot the Differences Just like in other logic puzzle games, you have to compare pictures and find differences in similar pictures. The game to find the difference in pictures is based on the premise of finding the difference, which is interesting and fun. Do you have everything to gain in this logic puzzle?