What Does Iqr Mean In Math

Example : Points Scored

The following box plot shows the distribution of points scored by basketball players in a certain league. What is the interquartile range of the distribution?

We can find the following values on the box plot to answer this:

• Q3 = 27
• Interquartile Range = 27 15 = 12

The interquartile range of the distribution is 12.

Box Plot Interquartile Range: How To Find It

Example question: Find the interquartile range for the above box plot.

• Step 1:Find Q1.Q1 is represented by the left hand edge of the box .

  In the above graph, Q1 is approximately at 2.6.

• Step 2:Find Q3.Q3 is represented on a boxplot by the right hand edge of the box.Q3 is approximately 12 in this graph.
• Step 3:Subtract the number you found in step 1 from the number you found in step 3.This will give you the interquartile range. 12 2.6 = 9.4.

Thats it!

Watch the video for step-by-step directions:

Outliers And Tukey Fences:

When there are no outliers in a sample, the mean and standard deviation are used to summarize a typical value and the variability in the sample, respectively. When there are outliers in a sample, the median and interquartile range are used to summarize a typical value and the variability in the sample, respectively.

Table 13 displays the means, standard deviations, medians, quartiles and interquartile ranges for each of the continuous variables in the subsample of n=10 participants who attended the seventh examination of the Framingham Offspring Study.

Table 13 – Summary Statistics on n=10 Participants

1 Determined byQ1-1.5

2 Determined by Q3+1.5

The Full Framingham Cohort

Table 15 – Summary Statistics on Sample of Participants

Read Also: Hbo Child Of Rage

S For Finding The Interquartile Range

Although theres only one formula, there are various different methods for identifying the quartiles. Youll get a different value for the interquartile range depending on the method you use.

Here, well discuss two of the most commonly used methods. These methods differ based on how they use the median.

Example : Comparing Plant Heights

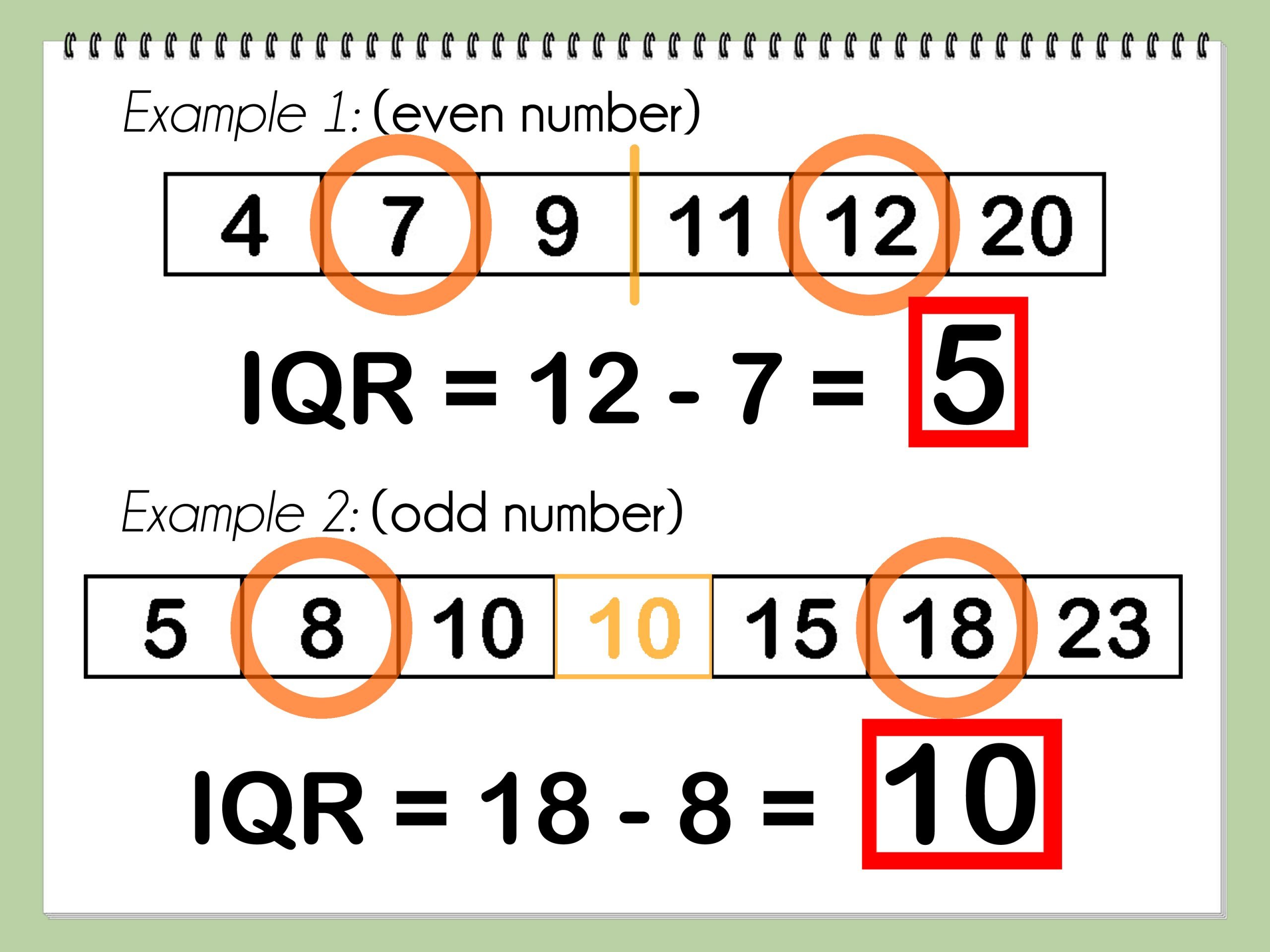
The following box plots show the distribution of heights for two different plant species: Red and Blue. Which distribution has a larger interquartile range?

First, lets find the interquartile range of the red box plot:

• Q3 = 30
• Interquartile Range = 30 20 = 10

Next, lets find the interquartile range of the blue box plot:

• Q3 = 27
• Interquartile Range = 27 15 = 12

The interquartile range for the Blue species is larger.

Also Check: How Many Biological Kids Does Steve Harvey Have

What Fields Use Iqr Calculations

You can use the interquartile range to determine how spread out data is from the mean of the values. When the IQR is a high value, the data spread further from the mean, while a lower IQR indicates data points that gather closer together around the mean. Therefore, measuring the IQR of a data set can be useful for a variety of applications in different industries, including:

What Is An Interquartile Range

The interquartile range is a measure of where the middle fifty is in a data set. Where a range is a measure of where the beginning and end are in a set, an interquartile range is a measure of where the bulk of the values lie. Thats why its preferred over many other measures of spread when reporting things like school performance or SAT scores.

The interquartile range formula is the first quartile subtracted from the third quartile:

IQR = Q3 Q1.

Watch the video for how to calculate the interquartile range by hand:

Don’t Miss: Pizzazz Bridge To Algebra

How To Find The Interquartile Range By Hand

The formula for finding the interquartile range takes the third quartile value and subtracts the first quartile value.

IQR = Q3 Q1

Equivalently, the interquartile range is the region between the 75th and 25th percentile .

Using the IQR formula, we need to find the values for Q3 and Q1. To do that, simply order your data from low to high and split the value into four equal portions.

Ive divided the dataset below into quartiles. The interquartile range extends from the Q1 value to the Q3 value. For this dataset, the interquartile range is 39 20 = 19.

Note that different methods and statistical software programs will find slightly different Q1 and Q3 values, which affects the interquartile range. These variations stem from alternate ways of finding percentiles. For details about that, read my post about Percentiles: Interpretations and Calculations.

Organizing The Data Set

Don’t Miss: Houghton Mifflin Geometry Worksheet Answers

Visualize The Interquartile Range In Boxplots

A boxplot, or a box-and-whisker plot, summarizes a data set visually using a five-number summary.

Every distribution can be organized using these five numbers:

• Lowest value
• Q3: 75th percentile
• Highest value

The vertical lines in the box show Q1, the median, and Q3, while the whiskers at the ends show the highest and lowest values.

In a boxplot, the width of the box shows you the interquartile range. A smaller width means you have less dispersion, while a larger width means you have more dispersion.

An inclusive interquartile range will have a smaller width than an exclusive interquartile range.

Boxplots are especially useful for showing the central tendency and dispersion of skewed distributions.

The placement of the box tells you the direction of the skew. A box thats much closer to the right side means you have a negatively skewed distribution, and a box closer to the left side tells you that you have a positively skewed distribution.

Calculate The Median In Even Data Sets

When calculating the interquartile range for a data set with an even number of values, the median can be less apparent. To find the median of your data in these cases, take the mean of the two middle values. Then, find the first and third quartiles and subtract to find the IQR. In the example data set , the two middle values are 46 and 58. Finding the mean of these two values gives you a median of 52.

Don’t Miss: Geometry: Homework Practice Workbook Answers

Using The Interquartile Range To Test Normality

You can even use the interquartile range as a simple test to determine whether your data are normally distributed. When data follow a normal distribution, the interquartile range will have specific properties. The image below highlights these properties. Specifically, in our calculations below, well use the standard deviations that correspond to the interquartile range, -0.67 and 0.67.

You can assess whether your IQR is consistent with a normal distribution. However, this test should not replace a formal normality hypothesis test.

To perform this test, youll need to know the sample standard deviation and sample mean . Input these values into the formulas for Q1 and Q3 below.

• Q1 = x
• Q3 = x +

Compare these calculated values to your datas actual Q1 and Q3 values. If they are notably different, your data might not follow the normal distribution.

Well return to our example dataset from before. Our actual Q1 and Q3 are 20 and 39, respectively.

The sample average is 31.3, and its standard deviation is 14.1. Ill input those values into the equations.

Q1 = 31.3 = 21.9

Q3 = 31.3 + = 40.7

The calculated values are pretty close to the actual data values, suggesting that our data follow the normal distribution. Ive included these calculations in the IQR example spreadsheet.

Interquartile Range Definition And Example

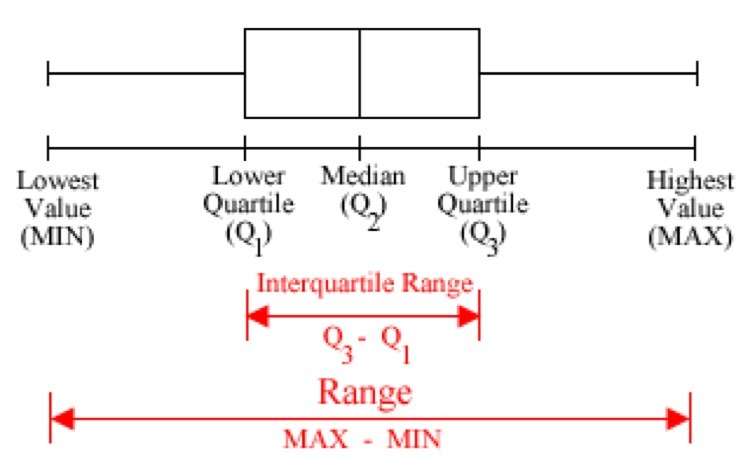
The interquartile definition states that the interquartile range is the difference between the third and the first quartiles. As we know quartiles are the divided values that divide the complete series into four equal parts. So, there are a total of 3 quartiles The first quartile also known as the lower quartile is represented by Q1, the second quartile is represented by Q2, and the last third quartile also known as the upper quartile is represented by Q3.

Let’s calculate the interquartile range of the below data

Read Also: Holt Geometry Worksheets

Measures Of Variability Based On Frequency

The calculation of interquartile ranges and standard deviations requires quantitative data. For categorical data, a different approach is needed. For such variables, there are measures of variability based on the frequency of occurrence of different attributes of a variable.

The Index of Diversity is one such measure. It is based on the proportions of the observations in each category of the qualitative variable. It is calculated as

where pk is the proportion of observations in category k, and K is the number of categories. If there is no variation, all the observations are in one category and D equals 0. With greater diversity, the measure approaches 1.

D. Rindskopf, M. Shiyko, in, 2010

Mean Of Angles And Cyclical Quantities

Angles, times of day, and other cyclical quantities require modular arithmetic to add and otherwise combine numbers. In all these situations, there will not be a unique mean. For example, the times an hour before and after midnight are equidistant to both midnight and noon. It is also possible that no mean exists. Consider a color wheelâthere is no mean to the set of all colors. In these situations, you must decide which mean is most useful. You can do this by adjusting the values before averaging, or by using a specialized approach for the mean of circular quantities.

Don’t Miss: Ccl4 Lewis Structure Polar Or Nonpolar

What Does The Iqr/median Mean

What does IQR/Med tell you about the data? What is the purpose of dividing the IQR by the Median?

Examples:

IQR = 3.0  Med = 8.1  IQR/Med = .37IQR = 2.1  Med = 7.1  IQR/Med = .29

I got the numbers from this site. I understand IQR and Median, and I was told that IQR/Med is an indicator of data quality . But I don’t understand why or how. I need to explain IQR/Med to a general audience, but I need to understand its utility first.

I can’t reach the site you link. It’s hard to know exactly what was meant by this number without more context. I can make an educated guess, though.

Many numbers will have a greater or lesser amount of variability depending on the level of their center. Starting with stats 101, we often think in terms of the normal distribution, in which case we can think of the standard deviation becoming larger with larger means. In such cases, it is often true that, although there is heteroscedasticity, there is a constant coefficient of variation . However, people may prefer to work outside of parameters like the mean and standard deviation, which have a strong connection to the normal distribution and are sensitive to outliers. The interquartile range can be considered a more robust measure of spread, and the median can likewise be considered a more robust measure of central tendency. Thus, the IQR/median can fill a similar role as the coefficient of variation while remaining more resistant to outliers.

Definition Of Interquartile Range

As seen above, the interquartile range is built upon the calculation of other statistics. Before determining the interquartile range, we first need to know the values of the first quartile and third quartile. .

Once we have determined the values of the first and third quartiles, the interquartile range is very easy to calculate. All that we have to do is to subtract the first quartile from the third quartile. This explains the use of the term interquartile range for this statistic.

Also Check: Imagine Math Parent Portal

What Is An Interquartile Range Used For

The IQR is used to measure how spread out the data points in a set are from the mean of the data set. The higher the IQR, the more spread out the data points in contrast, the smaller the IQR, the more bunched up the data points are around the mean. The IQR range is one of many measurements used to measure how spread out the data points in a data set are. It is best used with other measurements such as the median and total range to build a complete picture of a data sets tendency to cluster around its mean.Back to Top

Solved Examples Using Interquartile Range Formula

Example 1: Using the interquartile range formula, calculate the range of the following set of data:

Solution: Given: Number of terms = 12, Set =

Ordered set =

Dividing the set into quartiles, each quarter will have 3 terms as: , , ,

First Quartile,

\ = / 2 = 4

Third Quartile,

\ = /2 = 15

Using Interquartile Range Formula,\

= 15 – 4

Therefore, the Interquartile range of the given set = 11

Example 2: Determine the interquartile range value for the first ten odd numbers.

Solution:

To find IQR of the first 10 odd numbers:

The first ten odd numbers:1, 3, 5, 7, 9, 11, 13, 15, 17, 18

n = 10

Since 10 is even, using the median formula, we find the median as the mean of the 5th and 6th terms.

That is \ = /2 \ = 10.

Now \ part is

Here the number of data points = 5

\ = median of = 5

\ part is

Here the number of data points = 5

\ = median of = 15

Using Interquartile range formula, IQR = \

\ is 15 5 = 10

Answer: 10 is the interquartile range for the given set of first 10 odd numbers.

Example 3: Europe has an estimated population of 1,420,062,022 people. Brazil’s population is 132,328,035. India has a population of 1,368,737,513. The United States has a population of 329,093,110. What is the range of this set?

Solution: Let’s arrange the values in a set:

Using the interquartile range formula, IQR = \

\ is the highest number i.e. 1,420,062,022 and \ is the lowest number i.e. 132,328,038

IQR = 1,420,062,022 – 132,328,038

Also Check: Is Paris Jackson Michael’s Biological Kid

How To Interpret Interquartile Range

The interquartile range of a dataset, often abbreviated IQR, is the difference between the first quartile and the third quartile of the dataset.

In simple terms, it measures the spread of the middle 50% of values.

IQR = Q3 Q1

For example, suppose we have the following dataset that shows the height of 17 different plants in a lab:

Dataset: 1, 4, 8, 11, 13, 17, 19, 19, 20, 23, 24, 24, 25, 28, 29, 31, 32

According to the Interquartile Range Calculator, the interquartile range for this dataset is calculated as:

• Q1: 12
• Q3: 26.5
• IQR = Q3 Q1 = 14.5

This tells us that the middle 50% of values in the dataset have a spread of 14.5 inches.

Example 2 Range And Interquartile Range In Presence Of An Extreme Value

Find the range and interquartile range of the data set of example 1, to which a data point of value 75 was added.

The range would now be 69 . The median would be the mean of the values of the data point of rank 12 ÷ 2 = 6 and the data point of rank + 1 = 7. Because it falls between ranks 6 and 7, there are six data points on each side of the median. The lower quartile is the mean of the values of the data point of rank 6 ÷ 2 = 3 and the data points of rank + 1 = 4. The result is ÷ 2 = 25.5. The upper quartile is the mean of the values of data point of rank 6 + 3 = 9 and the data point of rank 6 + 4 = 10, which is ÷ 2 = 45. The interquartile range is 45 – 25.5 = 19.5.

In summary, the range went from 43 to 69, an increase of 26 compared to example 1, just because of a single extreme value. The more robust interquartile range went from 28 to 19.5, a decrease of only 8.5.

The second example demonstrated that the interquartile range is more robust than the range when the data set includes a value considered extreme. Its not a perfect measure, though. In this example, we might have expected that when adding an extreme value, the measure of dispersion would increase, but the opposite happened because there was a great difference between the values of data points of ranks 3 and 4.

Recommended Reading: Beth Thomas Documentary