How To Calculate The Force Of Friction
Surfaces exert a frictional force that resists sliding motions, and you need to calculate the size of this force as part of many physics problems. The amount of friction mainly depends on the normal force, which surfaces exert on the objects sitting on them, as well as the characteristics of the specific surface youre considering. For most purposes, you can use the formula:
to calculate friction, with N standing for the normal force and incorporating the characteristics of the surface.
Finding The Force Of Friction Experimentally
Use the object in question and a small section of the surface you can move freely to set up an inclined ramp. If you cant use the whole surface or the whole object, just use a piece of something made from the same material. For example, if you have a tiled floor as a surface, you could use a single tile to create the ramp. If you have a wooden cupboard as an object, use a different, smaller object made from wood . The closer you can get to the real situation, the more accurate your calculation will be.
Ensure that you can adjust the incline of the ramp, by stacking up a series of books or something similar, so you can make small adjustments to its maximum height.
The more inclined the surface, the more the force due to gravity will work to pull it down the ramp. The force of friction works against this, but at some point, the force due to gravity overcomes it. This tells you the maximum force of friction for these materials, and physicists describe this through the coefficient of static friction . The experiment allows you to find the value for this.
The math for the situation works out neatly, and it turns out that the tangent of the angle of the incline tells you the value of the coefficient. So:
Or, because tan = opposite / adjacent = length of base / height, you calculate:
Complete this calculation to find the value for the coefficient for your specific situation.
Tips
Here, m is the mass of the object and g is the acceleration due to gravity .
Normal Force With An External Upward Force
Don’t Miss: What Kind Of Math Is On The Ged Test
What Is Mu In Statistics
In statistics, Mu stands for the mean of a series of numbers. The mean can also be described as the average of the numbers.
To reach the solution to Mu, the mean or the average, the numbers should be added together and then divided by the amount of numbers there are in a series. For example, if the series of numbers is 12, 64, 13 and 83, the numbers would be added together to reach the total of 172. This number should then be divided by 4 since there are four numbers in the sequence. The mean of these numbers is equal to 43.
In mathematical applications, it is not unusual for a question to ask a student to find the mean, the median, the range and the mode of a series of numbers. In order to find the median, the range and the mode of the numbers, the mean needs to be used. Students should always find the mean first to ensure that they are able to properly identify the rest of the series. The median, range and mode can often be found by simply looking at the series of numbers and the order that they appear in.
Static Friction Vs Kinetic Friction

Static friction acts when the object remains stationary. Imagine you try to pull a heavy box. If we don’t take friction into account, even the smallest force should cause some acceleration of the box, according to Newton’s second law. In reality, you need to pull quite hard for the box to start moving because of the static friction force.
Kinetic friction acts on a moving object or, in other words, on an object with nonzero kinetic energy. If there were no kinetic friction, any object that you nudge would never stop moving, as, according to Newton’s first law, no force would act on it, so it would keep on going with a constant velocity.
Even though the formula is the same for static and kinetic friction, you need to remember that the coefficients of friction are different. The coefficient of kinetic friction is usually lower than the one of static friction.
Read Also: What Is Standardization In Chemistry
Inertial Forces And Noninertial Frames: The Coriolis Force
What do taking off in a jet airplane, turning a corner in a car, riding a merry-go-round, and the circular motion of a tropical cyclone have in common? Each exhibits inertial forcesforces that merely seem to arise from motion, because the observers frame of reference is accelerating or rotating. When taking off in a jet, most people would agree it feels as if you are being pushed back into the seat as the airplane accelerates down the runway. Yet a physicist would say that you tend to remain stationary while the seat pushes forward on you. An even more common experience occurs when you make a tight curve in your carsay, to the right ). You feel as if you are thrown toward the left relative to the car. Again, a physicist would say that you are going in a straight line but the car moves to the right, not that you are experiencing a force from the left.
A physicist will choose whatever reference frame is most convenient for the situation being analyzed. There is no problem to a physicist in including inertial forces and Newtons second law, as usual, if that is more convenient, for example, on a merry-go-round or on a rotating planet. Noninertial frames of reference are used when it is useful to do so. Different frames of reference must be considered in discussing the motion of an astronaut in a spacecraft traveling at speeds near the speed of light, as you will appreciate in the study of the special theory of relativity.
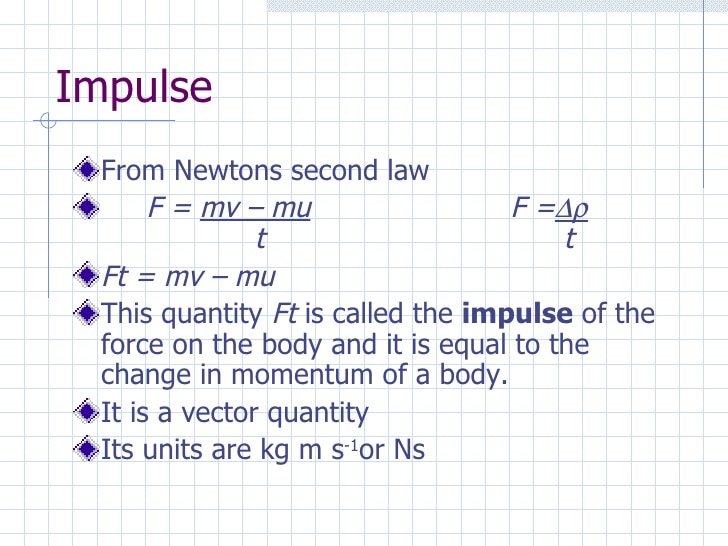
Calculating The Rate Of Change Of Momentum
You can combine two equations to show how to calculate the force involved when a change in momentum happens:
force = mass × acceleration
Acceleration appears in both equations, giving:
force = mass × \ = \
- force is measured in newtons
- change in momentum is measured in kilogram metres per second
- time taken is measured in seconds
The equation shows that the force involved is equal to the rate of change of momentum.
Don’t Miss: What Is An Experimental Study In Psychology
How Is Coefficient Of Friction Calculated
How is coefficient of static friction and coefficient of kinetic friction is calculated in real life without knowing the frictional force?
I can not give you an example for all surfaces, but for an inclined plane, the formula is pretty straightforward. Suppose you wanted to find the coefficient of friction between a block and an incline. Keep the block on the incline, and if the block is stationary, try to increase the angle of incline until the block just begins to slide. At this point, the force on the object is just equal to the maximum value of static friction, i.e. $\mu\ mg\ \text\ \theta$ where $\theta$ is the angle of incline. And what is the force on the object? Well, it’s the other component of weight i.e. $mg\ \text\ \theta$.For equilibrium condition, you equate these two-$$\mu\ mg\ \text\ \theta=mg\ \text\ \theta$$from which you simply get- $$\mu=\text\ \theta$$
For an increased accuracy, repeat the above experiment with heavier/lighter blocks, different inclines of the same material, and also take care to keep the surface smooth enough to allow a uniform incline. Take the mean and you will get a really close value. Note that in this answer I have simply shown a really general method which is far from rigorous, but I hope this still helps
Iii Using Autocorrect For Math:
When you work with many documents and often need to paste one specialsymbol, you may not want to insert an equation each time. Microsoft Word offers ahelpful feature named AutoCorrect. The AutoCorrect options in MicrosoftWord propose two different ways to quickly add any special character, such as amicro sign or mu letter from the Greek alphabet, or evenlarge pieces of text:
Also Check: What Are The Different Kinds Of Reasoning In Geometry
How To Find Force Of Friction
Example 2 Calculating The Number Of Electrons That Move Through A Calculator
If the 0.300-mA current through the calculator mentioned in;Example 1;is carried by electrons, how many electrons per second pass through it?
Strategy
The current calculated in the previous example was defined for the flow of positive charge. For electrons, the magnitude is the same, but the sign is opposite,;Ielectrons =;0.300;×;103C/s. Since each electron has a charge of;1.60×1019;C, we can convert the current in coulombs per second to electrons per second.
Solution
Starting with the definition of current, we have
_}=\frac_}}=\frac\times }^\text}}\\
We divide this by the charge per electron, so that
\begin\frac^}}}& =& \frac\times }^\text}}\times \frac^}}\times }^}\text}\\ & =& \text\times }^}\frac^}}}\end\\.
Discussion
There are so many charged particles moving, even in small currents, that individual charges are not noticed, just as individual water molecules are not noticed in water flow. Even more amazing is that they do not always keep moving forward like soldiers in a parade. Rather they are like a crowd of people with movement in different directions but a general trend to move forward. There are lots of collisions with atoms in the metal wire and, of course, with other electrons.
You May Like: When Was Geometry Dash Made
Values For Some Common Materials
The following table should be used with caution as the permeability of ferromagnetic materials varies greatly with field strength. For example, 4% Si steel has an initial relative permeability of 2,000 and a maximum of 35,000 and, indeed, the relative permeability of any material at a sufficiently high field strength trends toward 1 .
| Medium |
|---|
A good magnetic core material must have high permeability.
For passivemagnetic levitation a relative permeability below 1 is needed .
Permeability varies with a magnetic field. Values shown above are approximate and valid only at the magnetic fields shown. They are given for a zero frequency; in practice, the permeability is generally a function of the frequency. When the frequency is considered, the permeability can be complex, corresponding to the in-phase and out of phase response.
Example Question #2 : Normal Force And Weight
Consider the following system:
Correct answer:
There are two forces in play in this scenario: gravity and friction. We can use Newton’s second law to form an expression with these forces:
Substituting in expressions for each force, we get:
If you are unsure of whether to use cosine;or sine;for each force, think about the situation practically. The flatter the slope gets, the less the force of gravity will have an effect on moving the block down the plane, hence the use of the sine;function. Also, the flatter the slope gets, the greater the normal force will become, hence the use of the cosine;function.
Canceling out mass and rearranging for the coefficient of friction, we get:
Read Also: How To Find Biological Grandparents Uk
How To Find The Force Of Friction Without Knowing The Coefficient Of Friction
Most people understand friction in an intuitive way. When you try to push an object along a surface, the contact between the object and the surface resists your push up to a certain pushing strength. Calculating the frictional force mathematically usually involves the coefficient of friction, which describes how much the two specific materials stick together to resist motion, and something called the normal force that relates to the mass of the object. But if you dont know the coefficient of friction, how do you work out the force? You can achieve this either by looking up a standard result online or conducting a small experiment.
Why Does Solving $\mu Mg = M \frac$ Give The *max* Possible Velocity
So here is the standard problem setup of a car turning on an unbanked road:
A 1000 kg car is going around a curve with radius 30 meters. If the coefficient of friction between the car’s tires and the road is 0.5, what is the maximum speed at which the car can make the turn?
You setup $$f_K = \mu_k mg = m \frac$$
and solve for $v$ to get the maximum possible velocity with which you can still make the turn.
However, what about if you go lower than this velocity. Clearly you will still be able to make the turn, however the above equation no longer works, even though the equation should hold.
While your centripetal acceleration decreases, the force from kinetic friction remains at the same value it was before, and now both sides of the equation are not equal.
What changes in regards to the frictional force that compensates for the decrease in acceleration?
Essentially, my question is that while it is “clear by logic” that going lower than the maximum velocity allows you to make the turn, how would you show that using the above equations as per newton’s second law?
The coefficient of static friction and the normal force together allow you to calculate the maximum force that friction can apply, not the actual force.
A block sitting on a shelf with no external horizontal forces will have a friction force of zero. If you apply a force from the side that is less than the maximum friction force, then the actual friction force will rise so that it is equal to the external force.
You May Like: What Not To Do In The Chemistry Lab
Relative Permeability And Magnetic Susceptibility
Relative permeability, denoted by the symbol r }} , is the ratio of the permeability of a specific medium to the permeability of free space 0:
- 1. =\mu _-1.}
The number m is a dimensionless quantity, sometimes called volumetric or bulk susceptibility, to distinguish it from p and M .
Diamagnetism is the property of an object which causes it to create a magnetic field in opposition of an externally applied magnetic field, thus causing a repulsive effect. Specifically, an external magnetic field alters the orbital velocity of electrons around their nuclei, thus changing the magnetic dipole moment in the direction opposing the external field. Diamagnets are materials with a magnetic permeability less than 0 .
Consequently, diamagnetism is a form of magnetism that a substance exhibits only in the presence of an externally applied magnetic field. It is generally a quite weak effect in most materials, although superconductors exhibit a strong effect.
Paramagnetism is a form of magnetism which occurs only in the presence of an externally applied magnetic field. Paramagnetic materials are attracted to magnetic fields, hence have a relative magnetic permeability greater than one .
What Would Happen In A World Without Friction
If there were no friction, there would be no anything. The friction that holds atoms together would disappear, so nothing would be able to form. Life wouldn’t exist, as atoms wouldnt be near each other long enough to form simple molecules. The world would become a dangerous place to live, as cars in motion would lose the ability to stop. Youd probably die as well, as your blood would steadily move around quicker and quicker. Good thing this scenario is physically impossible!
Don’t Miss: Practice 2 4 Reasoning In Algebra Answer Key
The Attempt At A Solution
- 7,945
- 827
The answer must have F in it somewhere! So yeah, the book answer is wrong. I believe.My answer has tan in it, but it also has mg and F.
Is your answer equivalent to mine?
A lady pushes a box with mass with force at a horizontal angle of . There is a static friction
haruspex said:Is she pushing down at angle theta or up at that angle? I’ll guess it’s up.You haven’t said what the question asks. I’ll guess again: it asks for the angle which minimises F.
haruspex said:You’re still not saying what the book actually asks. Pls post the whole question verbatim.
University Physics
student34 said:I am not sure if I have to do this, but I am going to reference the question just in case I am not committing some kind of plagiarism. So just ignore the reference. “A large crate with mass m rests on a horizontal floor. The coefficients of friction between the crate and the floor are and . A woman pushes downward at an angle below the horizontal on the crate with force F. If is greater than some critical value, the woman cannot start the crate moving no matter how hard she pushes. Calculate this critical value of ” . Referenced from Sears and Zemansky’s University Physics
student34 said:no matter how hard she pushes.
That’s the piece of information that was missing. You obtained this equation correctly : = /With the understanding that F is not a specific value and is unlimited in magnitude, can you now derive the desired result from your equation?