How To Find Iqr In Math

Definition Of Interquartile Range

As seen above, the interquartile range is built upon the calculation of other statistics. Before determining the interquartile range, we first need to know the values of the first quartile and third quartile. .

Once we have determined the values of the first and third quartiles, the interquartile range is very easy to calculate. All that we have to do is to subtract the first quartile from the third quartile. This explains the use of the term interquartile range for this statistic.

How To Find The Interquartile Range Of A Box Plot

A box plot is a type of plot that displays the five number summary of a dataset, which includes:

• The minimum value
• The first quartile
• The median value
• The third quartile
• The maximum value

To make a box plot, we draw a box from the first to the third quartile. Then we draw a vertical line at the median. Lastly, we draw whiskers from the quartiles to the minimum and maximum value.

The interquartile range, often abbreviated IQR, is the difference between the third quartile and the first quartile.

• IQR = Q3 Q1

This tells us how spread out the middle 50% of values are in a given dataset.

The following examples show how to find the interquartile range of a box plot in practice.

How To Find An Interquartile Range In Spss

Like most technology, SPSS has several ways that you can calculate the IQR. However, if you click on the most intuitive way you would expect to find it , the surprise is that it wont list the IQR . You could take this route and then subtract the third quartile from the first to get the IQR. However, the easiest way to find the interquartile range in SPSS by using the Explore command. If you have already typed data into your worksheet, skip to Step 3.

Watch the video for the steps:

Cant see the steps? .

Also Check: How To Find The Half Life

What Is An Interquartile Range Used For

The IQR is used to measure how spread out the data points in a set are from the mean of the data set. The higher the IQR, the more spread out the data points in contrast, the smaller the IQR, the more bunched up the data points are around the mean. The IQR range is one of many measurements used to measure how spread out the data points in a data set are. It is best used with other measurements such as the median and total range to build a complete picture of a data sets tendency to cluster around its mean.Back to Top

Example : Comparing Plant Heights

The following box plots show the distribution of heights for two different plant species: Red and Blue. Which distribution has a larger interquartile range?

First, lets find the interquartile range of the red box plot:

• Q3 = 30
• Interquartile Range = 30 20 = 10

Next, lets find the interquartile range of the blue box plot:

• Q3 = 27
• Interquartile Range = 27 15 = 12

The interquartile range for the Blue species is larger.

Read Also: Is Paris Jackson Biological Daughter

Iqr As A Test For Normal Distribution

We can also use the IQR formula with the mean and standard deviation in order to test whether or not a population experiences a normal distribution. The formula to find out if or not a population is normally distributed is as mentioned below:

Q1 =

Q3 = +

Here, Q1 refers to the 1st quartile, Q3 is the 3rd quartile, represents the standard deviation and is the mean. For the purpose of stating whether a population is normally distributed, simplify for the values of Q1 and Q3 and then compare the outcomes. If the outcomes of the values of 1st or 3rd quartiles calculated this way and the previous way match exactly, then the population is normally distributed.

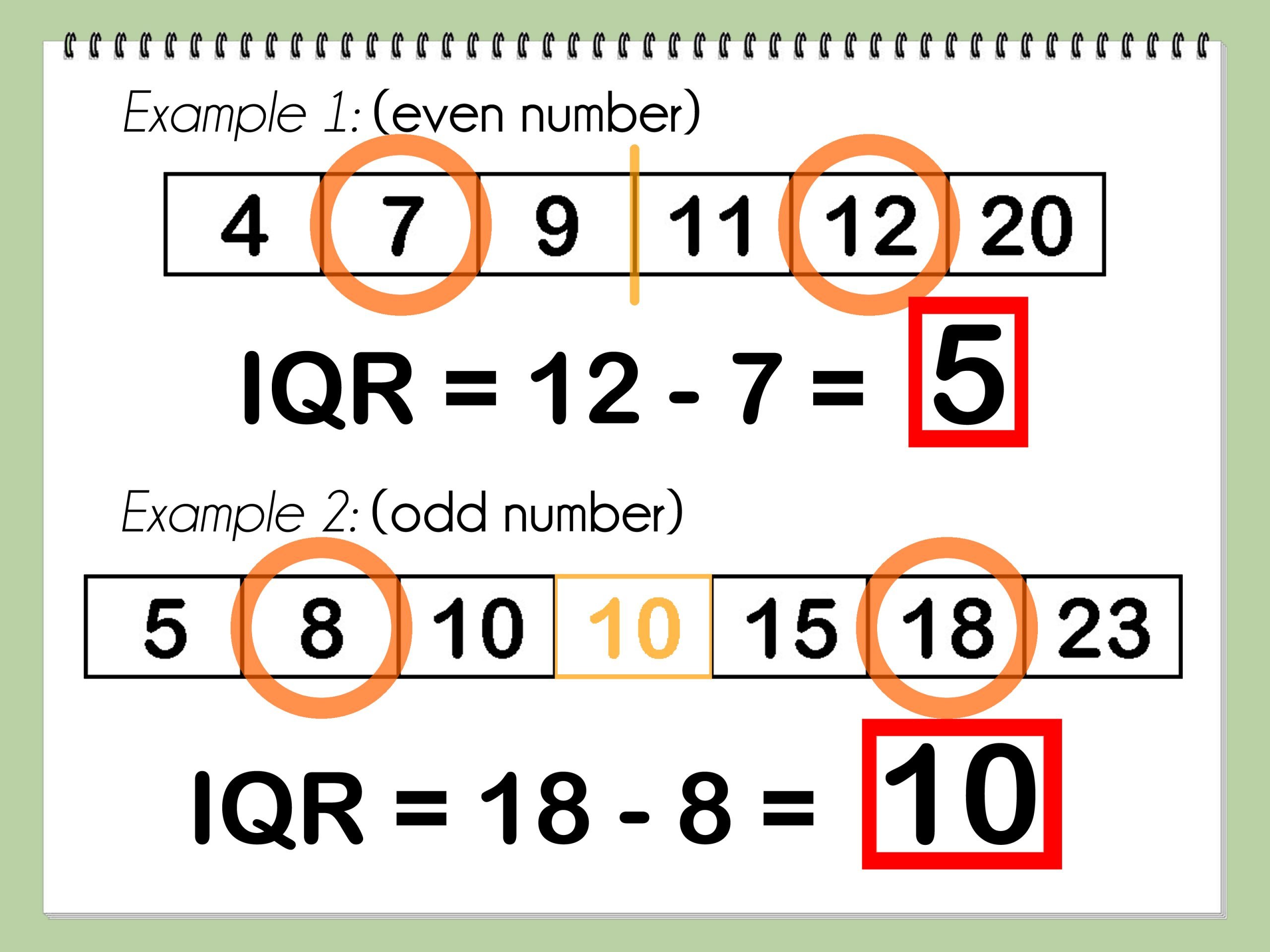
How To Find Interquartile Range For An Odd Set Of Numbers

Recommended Reading: What Is Elastic Force

How To Use The Quartile Calculator

To use this calculator, follow the steps given below:

• Enter the data set as a quartile range in the given input box. Separate each value using a comma.
• Press the Calculate button to see the results.

It will give you the calculated IQR, first quartile, second quartile, and third quartile.

In this post, we will explain the quartile definition, how to find the first quartile and the lower quartiles, and the interquartile range.

Upper And Lower Medians

Once we have found the median of the entire set, we can find the medians of the upper and lower portions of the data. If the data set has an odd number of values, we will omit the median or centermost value of the set. Afterwards, we will find the individual medians for the upper and lower portions of the data.

Omit the centermost value.

Find the median of the lower portion.

Calculate the average of the two values.

The median of the lower portion is

Find the median of the upper portion.

Calculate the average of the two values.

The median of the upper potion is

If the data set has an even number of values, we will use the two values used to calculate the original median to divide the data set. These values are not omitted and become the largest value of the lower data set and the lowest values of the upper data set, respectively. Afterwards, we will calculate the medians of both the upper and lower portions.

Find the median of the lower portion.

The median of the lower portion is two.

Find the median of the upper portion.

The median of the upper portion is eight.

Read Also: Similar Meaning In Math

Advantages And Disadvantages Of Iqr

The interquartile range carries an exceptional advantage of being able to determine and eradicate deviation on both ends of a data set. IQR is a more effective tool for data analysis than the mean or median of a data set. An interquartile range also makes for an outstanding measure of variation in situations of skewed data distribution. The method of calculating IQR can be functional for grouped data sets, so long as you use a cumulative frequency distribution to order your data points.

The IQR formula for grouped data is just the same as the non-grouped data, with the interquartile range being equal to the value of the 1st quartile subtracted from the value of the 3rd quartile.

On the other hand, it has some disadvantages in comparison to standard deviation. These comprise less susceptibility to several extreme scores and a sampling consistency that is not as powerful as standard deviation.

How To Find Q1 Q3 And The Interquartile Range Ti 89

Example problem: Find Q1, Q3, and the IQR for the following list of numbers: 1, 9, 2, 3, 7, 8, 9, 2.

Step 1:Press APPS. Scroll to Stats/List Editor . Press ENTER. If you dont have the stats/list editor you can download it here.

Step 2:Clear the list editor of data: press F1 8.

Step 3: Press ALPHA 9 ALPHA 1 ENTER. This names your list IQ.

Step 4:Enter your numbers, one at a time. Follow each entry by pressing the ENTER key. For our group of numbers, enter1,9,2,3,7,8,9,2

Step 5: Press F4, then ENTER .

Step 6:Tell the calculator you want stats for the list called IQ by entering ALPHA 9 ALPHA 1 into the List: box. The calculator should automatically put the cursor there for you. Press ENTER twice.

Step 7:Read the results. Q1 is listed as Q1X . Q3 is listed as Q3X . To find the IQR, subtract Q1 from Q3 on the Home screen. The IQR is 8.5-2=6.5.

Thats it!

Use the interquartile range formula with the mean and standard deviation to test whether or not a population has a normal distribution. The formula to determine whether or not a population is normally distributed are:Q1 + XQ3 + XWhere Q1 is the first quartile, Q3 is the third quartile, is the standard deviation, z is the standard score and X is the mean. In order to tell whether a population is normally distributed, solve both equations and then compare the results. If there is a significant difference between the results and the first or third quartiles, then the population is not normally distributed.

Don’t Miss: Paris Jackson’s Mother

How To Find An Interquartile Range Excel 2007

Watch the video or read the steps below to find an interquartile range in Excel 2007:

Steps:Step 1: Enter your data into a single Excel column on a worksheet. For example, type your data in cells A2 to A10. Dont leave any gaps in your data.

Step 2: Click a blank cell and then type =QUARTILE. Youll need to replace A2:A10 with the actual values from your data set. For example, if you typed your data into B2 to B50, the equation is =QUARTILE. The 1 in this Excel formula represents the first quartile .

Step 3: Click a second blank cell and then type =QUARTILE. Replace A2:A10 with the actual values from your data set. The 3 in this Excel formula represents the third quartile .

Step 4: Click a third blank cell and then type =B3-B2. If your quartile functions from Step 2 and 3 are in different locations, change the cell references.

Step 5: Press the Enter key. Excel will return the IQR in the cell you clicked in Step 4

Thats it!

How To Find The Iqr

Given a set of data ordered from smallest to largest,

the IQR can be found by subtracting Q1 from Q3, or:

IQR = Q3 – Q1

Refer to the quartile page for more information on how to find each quartile. Q2 is the median of the set of data, Q1 is the median of the data between the first element and Q2, and Q3 is the median of the data between Q3 and the final element of the set.

Thus, in the set above, Q1 is 7, Q2 is 16, and Q3 is 30. The interquartile range is therefore:

IQR = 30 – 7 = 23

where n is the number of terms in the set. Thus:

Q1 = 0.25 = 3.25

Q3 = 0.75 = 9.75

The decimal values indicate that the quartile lies between the elements closest to the value. Thus, Q1 lies between the 3rd and 4th element in the set, and Q3 lies between the 9th and 10th elements. Averaging the terms in those positions yields Q1 and Q3:

Thus, the interquartile range can be calculated as:

IQR = 37.5 – 7.5 = 30

Also Check: Geometry Segment And Angle Addition Worksheet Answers

Example 2 Range And Interquartile Range In Presence Of An Extreme Value

Find the range and interquartile range of the data set of example 1, to which a data point of value 75 was added.

The range would now be 69 . The median would be the mean of the values of the data point of rank 12 ÷ 2 = 6 and the data point of rank + 1 = 7. Because it falls between ranks 6 and 7, there are six data points on each side of the median. The lower quartile is the mean of the values of the data point of rank 6 ÷ 2 = 3 and the data points of rank + 1 = 4. The result is ÷ 2 = 25.5. The upper quartile is the mean of the values of data point of rank 6 + 3 = 9 and the data point of rank 6 + 4 = 10, which is ÷ 2 = 45. The interquartile range is 45 – 25.5 = 19.5.

In summary, the range went from 43 to 69, an increase of 26 compared to example 1, just because of a single extreme value. The more robust interquartile range went from 28 to 19.5, a decrease of only 8.5.

The second example demonstrated that the interquartile range is more robust than the range when the data set includes a value considered extreme. Its not a perfect measure, though. In this example, we might have expected that when adding an extreme value, the measure of dispersion would increase, but the opposite happened because there was a great difference between the values of data points of ranks 3 and 4.

Use Of The Interquartile Range

Besides being a less sensitive measure of the spread of a data set, the interquartile range has another important use. Due to its resistance to outliers, the interquartile range is useful in identifying when a value is an outlier.

The interquartile range rule is what informs us whether we have a mild or strong outlier. To look for an outlier, we must look below the first quartile or above the third quartile. How far we should go depends upon the value of the interquartile range.

Recommended Reading: Slader Geometry Workbook

How To Calculate The Interquartile Range

The procedure to calculate the interquartile range is given as follows:

• Arrange the given set of numbers into increasing or decreasing order.
• Then count the given values. If it is odd, then the center value is median otherwise obtain the mean value for two center values. This is known as Q2 value. If there are even number of values, the median will be the average of the middle two values.
• Median equally cuts the given values into two equal parts. They are described as Q1 and Q3 parts.
• The median of data values below the median represents Q1.
• The median of data values above the median value represents Q3.
• Finally, we can subtract the median values of Q1 and Q3.
• The resulting value is the interquartile range.

Interquartile Range In Minitab: Steps

Example question: Find an interquartile range in Minitab for the Grade Point Average in the following data set:Grade Point Average : 1, 1, 2, 2, 3, 3, 3, 4, 4, 5, 5, 5, 6, 6, 6, 7, 7, 8, 8.

Step 1: Type your data into a Minitab worksheet. Enter your data into one or two columns.

Step 2: then click Basic Statistics, then click Display Descriptive Statistics to open the Descriptive Statistics menu.

Step 3: and then click the Select button to transfer the variable name to the right-hand window.

Step 4:

Step 5: Check Interquartile Range..

Step 6: The IQR for the GPA in this particular data set is 1.8.

Thats it!

Tip: If you dont see descriptive statistics show in a window, click Window on the toolbar, then click Tile. Click the Session window and then scroll up to see your results.

Don’t Miss: Who Is The Biological Father Of Paris Jackson

Median And Interquartile Range

The median is the middle value of the distribution of the given data. The interquartile range is the range of values that resides in the middle of the scores. When a distribution is skewed, and the median is used instead of the mean to show a central tendency, the appropriate measure of variability is the Interquartile range.

Q1 Lower Quartile Part

Q2 Median

Q3 Upper Quartile Part

It is a measure of dispersion based on the lower and upper quartile. Quartile deviation is obtained from interquartile range on dividing by 2, hence also known as semi interquartile range.

Organizing The Data Set

You May Like: How To Find The Indicated Length

Find An Interquartile Range For An Odd Set Of Numbers: Alternate Method

As you may already know, nothing is set in stone in statistics: when some statisticians find an interquartile range for a set of odd numbers, they include the median in both both quartiles. For example, in the following set of numbers: 1, 2, 5, 6, 7, 9, 12, 15, 18, 19, 27 some statisticians would break it into two halves, including the median in both halves:, This leads to two halves with an even set of numbers, so you can follow the steps above to find the IQR.

Watch the video for the steps.