Are You Confused About What Is Taught In An Algebra 1 Curriculum
This page will give you an idea of the Algebra 1 concepts and skills that are taught and the order in which they are presented.
This is the order in which I like to teach the units however, not all curriculum is set up exactly the same. So, please use this as a guide, but remember that there are many different Algebra 1 textbooks and curricula and each is set up a little different.
- Solving Equations with the Distributive Property
- Solving Equations with Fractions
- Solving Equations With Variables on Both Sides
- Making Connections with Real World Problems
- Graphing Using a Table of Values
- Calculating Slope
- Rate of Change
- Standard Form Equations and X and Y Intercepts
- Writing Equations in Slope Intercept Form
- Writing Equations in Standard Form
- Writing Equations Given Slope and a Point
- Writing Equations Given Two Points
- Linear Combinations Method
- Making Connections with Real World Problems
- Inequalities in One Variable
- Graphing Linear Inequalities
- Introduction to Relations and Functions
- Identifying Functions
- Evaluating Functions
- Working with Negative and Zero Exponents
- Scientific Notation
- Factoring by Using the GCF
- Factoring by Grouping
- Factoring Trinomials with a Coefficient of 1
- Factoring Trinomials with a Coefficient Greater Than 1
- Solving Quadratic Equations by Graphing
- Solving by Factoring
Areas Of Mathematics With The Word Algebra In Their Name
Some subareas of algebra have the word algebra in their name linear algebra is one example. Others do not: group theory, ring theory, and field theory are examples. In this section, we list some areas of mathematics with the word “algebra” in the name.
- Elementary algebra, the part of algebra that is usually taught in elementary courses of mathematics.
xyc
Elementary algebra is the most basic form of algebra. It is taught to students who are presumed to have no knowledge of mathematics beyond the basic principles of arithmetic. In arithmetic, only numbers and their arithmetical operations occur. In algebra, numbers are often represented by symbols called variables . This is useful because:
- It allows the general formulation of arithmetical laws , and thus is the first step to a systematic exploration of the properties of the real number system.
- It allows the reference to “unknown” numbers, the formulation of equations and the study of how to solve these.
- It allows the formulation of functional relationships. = 3x 10, where f is the function, and x is the number to which the function is applied”.)
Linear Equations In Standard Form
The standard form or the general form of linear equations in one variable is written as, Ax + B = 0 where A and B are real numbers, and x is the single variable. The standard form of linear equations in two variables is expressed as, Ax + By = C where A, B and C are any real numbers, and x and y are the variables.
Don’t Miss: Who Is Paris Jackson’s Real Father
Preparing The Food Or Doubling Or Halving The Recipe
The kitchen is one of the places where Algebra is used in an interesting manner. Especially, when you are making items like cake, vegetable soups, etc, where the correct combination of the ingredients is very crucial, Algebra comes to your rescue and helps you find the correct quantity of ingredients to make the food sufficient for different sizes of servings.
All quantities of the ingredients and the number of servings make the variables. The cook uses Algebraic intelligence to ensure that not only the food is available in sufficient quantity, but also it is tasting great. Such is the extent of Algebra use in cooking!
What Is A Linear Equation
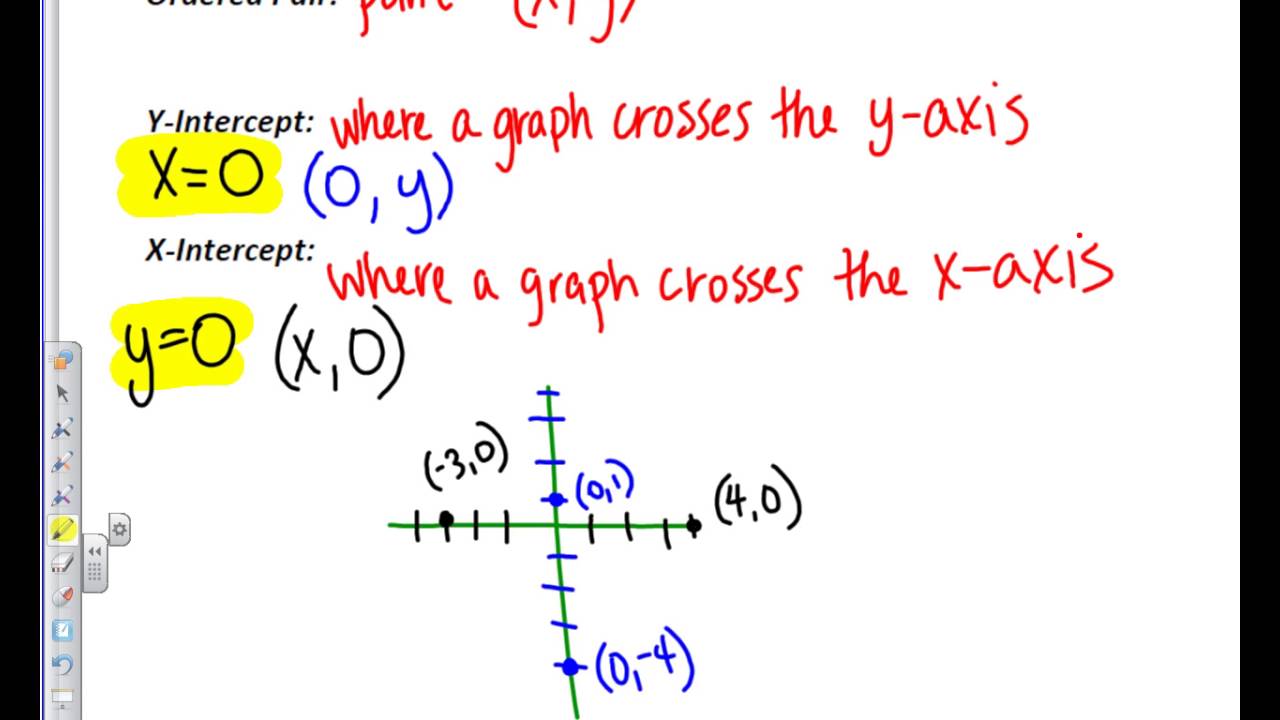
An equation that has the highest degree of 1 is known as a linear equation. This means that no variable in a linear equation has an exponent more than 1. The graph of a linear equation always forms a straight line.
Linear Equation Definition: A linear equation is an algebraic equation where each term has an exponent of 1 and when this equation is graphed, it always results in a straight line. This is the reason why it is named as a ‘linear equation’.
There are linear equations in one variable and linear equations in two variables. Let us learn how to identify linear equations and non-linear equations with the help of the following examples.
| Equations | |
|---|---|
| Non-Linear, the power of the variable x is 2 | |
| y + x = 6 | Non-Linear, the power of the variable y is 1/2 |
| y + 3x – 1 = 0 | |
| Non-Linear, the power of the variable x is 2 |
You May Like: Geometry Dash Hack No Jailbreak
How Are Algebra And Geometry Different
Algebra is a branch of mathematics in which arithmetic operations and other formal manipulations are applied to abstract symbols rather than specific numbers. Geometry is the branch of mathematics that deals with the shape of objects, their spatial relations, and the properties of the space the objects are in.
algebra, branch of mathematics in which arithmetical operations and formal manipulations are applied to abstract symbols rather than specific numbers. The notion that there exists such a distinct subdiscipline of mathematics, as well as the term algebra to denote it, resulted from a slow historical development. This article presents that history, tracing the evolution over time of the concept of the equation, number systems, symbols for conveying and manipulating mathematical statements, and the modern abstract structural view of algebra. For information on specific branches of algebra, seeelementary algebra, linear algebra, and modern algebra.
How Is Algebra Used In Business
Algebra can be used to predict sales and how much a customer will spend. Algebra is used to estimate the life time value of a customer, which calculates the percentage of annual spend.
The formula used is:
y = 12 + 8x
Algebra is used because x can change. y represents the percentage that a customer will spend in a year.
Imagine that a customer spends £50 in their first 2 months with us. We have to include their first month as x = 0, so their 2nd month will be x = 1.
Now we know x = 1, we can substitute it into y = 12 + 8x.
Therefore:
y = 12 + y = 20%
If 20% = £50. Then over a full year, 100% will equal £250 . This means we can predict a customer will spend £250 a year.
Also Check: 4 Main Areas Of Biological Contamination
What Is A Linear Equation Explain With An Example
A linear equation is an equation in which the highest power of the variable is always 1. It is also known as a one-degree equation. When this equation is graphed, it always results in a straight line. This is the reason why it is termed as a ‘linear equation’. There are linear equations in one variable, in two variables, in three variables, and so on. The standard form of a linear equation in one variable is of the form Ax + B = 0. An equation of the form Ax + By = C is called a linear equation in two variables. A few examples of linear equations are 5x + 6 = 1, 42x + 32y = 60, 7x = 84, etc.
Examples Of Algebra In A Sentence
algebra Quanta MagazinealgebraThe New York Review of Booksalgebra alalgebra Milwaukee Journal SentinelalgebraForbesalgebra Washington Postalgebra Anchorage Daily Newsalgebra Vulture
These example sentences are selected automatically from various online news sources to reflect current usage of the word ‘algebra.’ Views expressed in the examples do not represent the opinion of Merriam-Webster or its editors. Send us feedback.
Recommended Reading: Ruler Postulate Calculator
Algebra 1 Topics And Concepts
- The Albert Team
Looking for all the topics in Algebra 1?
Algebra 1 is a math course exploring how to use variables, numbers, and mathematical operations to solve problems. Explore below for a list of all Algebra 1 topics, a summary of the typical Algebra 1 course, and a discussion of what makes Algebra 1 unique.
What We Review
How To Simplify Algebraic Expressions
To simplify an algebraic expression, we just combine the like terms. Hence, the like variables will be combined together. Now, out of the like variables, the same powers will be combined together. For example, let us take an algebraic expression and try to reduce it to its lowest form in order to understand the concept better. Let our expression be:
x3 + 3×2 2×3 + 2x x2 + 3 x
= + + + 3
= x3 + 2×2 + x + 3
Hence, the algebraic expression x3 + 3×2 2×3 + 2x x2 + 3 x simplifies to x3 + 2×2 + x + 3.
Also Check: What Happened To Beth Thomas Brother Jonathan
Basic Algebra Explanation & Examples
Algebra? The mere mention of the term makes most of the students break out in a cold sweat. There is this notion that algebra is the hardest course in mathematics.
This is just a mere fallacy, and in fact, algebra is one of the easiest topics in mathematics. This article is meant to alleviate this fear and misconception from students and make algebra an enjoyable lesson for beginners.
Making A Schedule Of Activities
When you are making a schedule of activities, you actually are dividing the whole day and summing up the times taken to complete the intended activities. So, the simple process of finding whether you will reach the office on time or not if you have chosen to set the alarm at 6 am, is an algebraic calculation that you do mentally. Hours, times taken in various activities, and some new unforeseen tasks are Algebraic variables in real life, that you have to work upon to be on time at the office or any meeting.
Scheduling is common to all. And, Algebra knowledge makes you the true master of your day because of which you know how to use variables and put them in an equation. Thus, you get all things done right and on time due to Algebra playing its role.
Also Check: I Ready Answers Level E
Doing Interiors And Landscape Designing
Not only interiors planning or landscape designing, in fact, but the whole of Architecture stream employs Algebra. When the designers are trying to find the correct elevation or try to arrive upon the collection of things required to do a space, they make use of data like measurements of the space and that of the items to fit in.
This is the Algebra use in real life that helps to understand how things are going to amplify the looks of the space and will contribute to the overall appearance of the space.
Similarly, in an office environment, the decisions like moving to larger spaces to accommodate people or designing cabins are some of the premises where Algebraic calculations come into play.
Types Of Algebraic Expressions
The types of algebraic expressions are based on the variables found in that particular expression, the number of the terms of that expression, and the values of the exponents of the variables in each expression. Given below is a table that divides the algebraic expressions into five different categories. Let us have a look at the table.
| Types |
|---|
Don’t Miss: Cpm Algebra 1 Answers
A Kid Developing Spatial Intelligence
When kids have just started to learn to walk or stand, they need to find the safe height or distance of any object that they can choose to rely upon for standing or walking. Even while using a walker, they are actually using Algebra mentally to find how far the walker will take them before hitting any object, by propelling it at a particular speed. Similarly, Algebra comes into action when the kid is trying to find whether the shape of an object is wieldable or not.
Also, Algebra is there in action when the kids are trying to play spatial reasoning games. They visualize the shapes and patterns and solve puzzles only because of certain Algebraic equations that they may come to know about in later stages of their education. So, though without cognition, kids gather spatial intelligence by applying Algebra.
Linear Equations In One Variable
A linear equation in one variable is an equation in which there is only one variable present. It is of the form Ax + B = 0, where A and B are any two real numbers and x is an unknown variable that has only one solution. It is the easiest way to represent a mathematical statement. This equation has a degree that is always equal to 1. A linear equation in one variable can be solved very easily. The variables are separated and brought to one side of the equation and the constants are combined and brought to the other side of the equation, to get the value of the unknown variable.
Example: Solve the linear equation in one variable: 3x + 6 = 18.
In order to solve the given equation, we bring the numbers on the right-hand side of the equation and we keep the variable on the left-hand side. This means, 3x = 18 – 6. Then, as we solve for x, we get, 3x = 12. Finally, the value of x = 12/3 = 4.
Recommended Reading: Movement In Geography Definition
What Are Algebra Concepts
4.2/5AlgebraAlgebraconcept
Algebra basics
- Quadratics and polynomials.
- Equations and geometry.
One may also ask, what are pre algebra concepts? Pre–algebra includes several broad subjects: Review of natural number arithmetic. New types of numbers such as integers, fractions, decimals and negative numbers. Simple roots and powers. Rules of evaluation of expressions, such as operator precedence and use of parentheses.
Also, what are the basic principles of algebra?
The Basic Laws of Algebra are the associative, commutative and distributive laws. They help explain the relationship between number operations and lend towards simplifying equations or solving them. The arrangement of addends does not affect the sum. The arrangement of factors does not affect the product.
What is algebra and examples?
Examples of algebra on a chalkboard. Licensed from iStockPhoto. noun. The definition of algebra is a type of math that focuses on demonstrating the properties and relationships of abstract things in symbolic form. Graphing, absolute value equations and scientific notation are each an example of a topic in algebra.
Problem Solving In Egypt And Babylon
The earliest extant mathematical text from Egypt is the Rhind papyrus . It and other texts attest to the ability of the ancient Egyptians to solve linear equations in one unknown. A linear equation is a first-degree equation, or one in which all the variables are only to the first power. Evidence from about 300 bc indicates that the Egyptians also knew how to solve problems involving a system of two equations in two unknown quantities, including quadratic equations. For example, given that the perimeter of a rectangular plot of land is 100 units and its area is 600 square units, the ancient Egyptians could solve for the fields length l and width w. However, throughout this period there was no use of symbolsproblems were stated and solved verbally. The following problem is typical:
- Method of calculating a quantity,
- multiplied by 1 1/2 added 4 it has come to 10.
- What is the quantity that says it?
- First you calculate the difference of this 10 to this 4. Then 6 results.
- Then you divide 1 by 1 1/2. Then 2/3 results.
- Then you calculate 2/3 of this 6. Then 4 results.
- Behold, it is 4, the quantity that said it.
- What has been found by you is correct.
Note that except for 2/3, for which a special symbol existed, the Egyptians expressed all fractional quantities using only unit fractions, that is, fractions bearing the numerator 1. For example, 3/4 would be written as 1/2 + 1/4.
You May Like: Imaginary Numbers Worksheet Kuta
Algebra 2 Or Advanced Algebra
This is the intermediate level of Algebra. This algebra has a high level of equations to solve as compared to pre-algebra. Advanced algebra will help you to go through the other parts of algebra such as:
- Equations with inequalities
- Solving system of linear equations
- Graphing of functions and linear equations
- Conic sections
- Polynomials and expressions with radicals
- Sequences and series
- Discrete mathematics and probability
Algebra 1 Or Elementary Algebra
Elementary Algebra covers the traditional topics studied in a modern elementary algebra course. Arithmetic includes numbers along with mathematical operations like +, -, x, ÷. But in algebra, the numbers are often represented by the symbols and are called variables such as x, a, n, y. It also allows the common formulation of the laws of arithmetic such as, a + b = b + a and it is the first step that shows the systematic exploration of all the properties of a system of real numbers.
The concepts coming under elementary algebra include variables, evaluating expressions and equations, properties of equalities and inequalities, solving the algebraic equations and linear equations having one or two variables, etc.
You May Like: Cyte Root Word
Tips And Tricks On Algebra 1
- To understand Algebra 1, we need to be familiar with the pre-algebra topics like integers, one-step equations, inequalities and equations, graphs and functions, percent, probabilities, an introduction to geometry, and, right triangles. Once we go through a refresher, then we can proceed to algebra 1.
- When multiplying two rational expressions in algebra, there is always a risk of getting false solutions or extraneous solutions so be careful with your calculations part.
- We can add polynomials by just adding the like terms to combine the two polynomials into one.
Important Notes on Algebra 1
- The addition property of inequality: Adding the same number to each side of the inequality produces an equivalent inequality.
- Negative exponents: The reciprocals of the positive exponents in exponential functions.
- The quotient of powers property: It tells us that when we divide the powers with the same base we just have to subtract the exponents.
- The constants have a monomial degree of 0.