What Are Even And Odd Numbers
- An even number is a whole numberA counting number: 0, 1, 2, 3 etc. It does not have a decimal or fraction part. in the two times table.
- An even number can be halved to leave another whole number.
- An odd number is not in the two times table and cannot be divided equally into two whole numbers.
- 6 can be divided into two lots of three, so it is an even number.
- 7 cannot be divided into two equal whole numbers, so it is an odd number.
- Even numbers end in 0, 2, 4, 6 or 8.
- Odd numbers end in 1, 3, 5, 7 or 9.
An even number is in the two times table, ending in 0, 2, 4, 6 or 8.
An even number is a whole number that can be halved to get another whole number.
- Even numbers are in the two times table and can be arranged in pairs of whole numbersA counting number: 0, 1, 2, 3 etc. It does not have a decimal or fraction part..
- Odd numbers cannot be arranged in a pair and will always have a spare unit.
- We can see that the first odd number is one because it does not form a pair.
- We alternate between odd and even every time we add one.
- The first odd numbers are: 1,3, 5, 7 and 9.
- The first even numbers are: 0, 2, 4, 6, and 8.
What Do The Letters R Q N And Z Mean In Math
In math, the letters R, Q, N, and Z refer, respectively, to real numbers, rational numbers, natural numbers, and integers.
Who are the experts?Our certified Educators are real professors, teachers, and scholars who use their academic expertise to tackle your toughest questions. Educators go through a rigorous application process, and every answer they submit is reviewed by our in-house editorial team.
The letters R, Q, N, and Z refers to a set of numbers such that:
R = real numbers includes all real number
Q= rational numbers
N = Natural numbers
z = integers ( all integers…
What Are Odd And Even Numbers
Children in Key Stage 1 need to learn about odd and even numbers.
An even number is a number that can be divided into two equal groups.
An odd number is a number that cannot be divided into two equal groups.
Even numbers end in 2, 4, 6, 8 and 0 regardless of how many digits they have .
Odd numbers end in 1, 3, 5, 7, 9.
You May Like: Algebra 2 Common Core June 2016
Odd Numbers And Even Numbers
The numbers that are not odd, are even numbers, which are divisible by 2. Odd numbers cannot be divided into two parts equally but even numbers can be divided equally. For example, 4 can be divided and paired into two parts as 2-2, but 3 cannot be divided and paired.
|
A few more key points to remember:
|
What Are The Heads Of 100 Composite Numbers
List composite numbers They are from 1 to 100: 4, 6, 8, 9, 10, 12, 14, 15, 16, 18, 20, 21, 22, 24, 25, 26, 27, 28, 30, 32, 33, 34 35, 36, 38, 39, 40, 42, 44, 45, 46, 48, 49, 50, 51, 52, 54, 55, 56, 57, 58, 60, 62, 63, 64, 6, 65 6 68, 69, 70, 72, 74, 75, 76, 77, 78, 80, 81, 82, 84, 85, 86, 87, 88, 90, 91, 92, 93, 94, 95, 8, 96 9 99, 100 .
Read Also: Why Is Ap Bio So Hard
What Is Equal Set
Equal set definition math states that when two sets have the same and equal elements, they are called Equal Sets. The arrangement or the order of the elements does not matter, only the same elements in each set matter. … An equal set is an equivalent set, but an equivalent set necessarily cannot be an equal set.
The Meaning Of Root Of Unity
The root of unity refers to the state in which for any integer n, the nth root of a number k is a number that, when multiplied by itself n times, yields the number k. A root of unity in, most simply put, any number which when multiplied by itself any number of times always equals 1. Therefore, an nth root of unity is any number k that satisfies the following equation:
k^n = 1 , where n is a positive integer.
Roots of unity are also sometimes called de Moivre numbers, after the French mathematician Abraham de Moivre. Roots of unity are traditionally used in branches of mathematics like number theory.
When considering real numbers, the only two that fit this definition of roots of unity are the numbers one and negative one . But the concept of the root of unity does not generally appear within such a simple context. Instead, the root of unity becomes a topic for mathematical discussion when dealing with complex numbers, which are those numbers that can be expressed in the form a + bi, where a and b are real numbers and i is the square root of negative one or an imaginary number. In fact, the number i is itself also a root of unity.
Recommended Reading: Practice And Homework Lesson 1.7 Answers
Vedantu Online Maths Class
Maths is an essential subject in school. In secondary classes, you learn the fundamentals of mathematics that help you in the higher secondary, to solve complex problems. Vedantu offers you a chance to learn from the best maths teachers that present you with clear and detailed explanations of the topics. Also, the teacher uses 3-D visuals of different topics to explain things more clearly.
How Do Even And Odd Numbers Work
This is the division of the rule. Numbers Those which are completely divisible by 2 or can be evenly divided are called even number and numbers The remainder is when a divisor cannot be divided by 2 or into equal parts odd numbers .So inter odd numbers is In a way even number and between even numbers is In a way odd the number.
Recommended Reading: How To Find Displacement In Physics
Adding Two Odd Numbers
Any odd number added to another odd number always gives an even number. This statement is also proved below.
| Odd + Odd = Even |
Let two odd numbers be a and b.
These numbers can be written in the form where
a = 2k1 + 1
and b = 2k2 + 1 where k1, k2 Z
Adding a + b we have,
+ = 2k1 + 2k2 + 2 = 2 which is surely divisible by 2.
What Is A Basic Definition Of Odd
Odd refers to a number that will have a remainder of 1 when divided by 2. Odd also describes something that is unusual or weird. Odd has many other senses as an adjective and a few as a noun.
An odd number is any number that has a remainder of 1 when its divided by 2. For example, 7 divided by 2 is 3 with a remainder of 1. 7 is an odd number. On the other hand, 8 divided by 2 is 4 with no remainder. A number, such as 8, that has no remainder when divided by 2 is called an even number.
Real-life examples: 5, 11, 37, and 649 are examples of odd numbers.
Used in a sentence:I always like to cut my pizzas into an odd number of slices.
Odd also describes something that is unusual or not what a person would expect.
Real-life examples: People often make odd choices or odd decisions that catch other people by surprise. For example, it might be considered odd to go swimming in regular clothing because people usually wear a bathing suit. A firefighter showing up to a fire in an ice cream truck would be odd because they are expected to drive fire trucks, which have firefighting tools.
Used in a sentence:They were caught off guard by the mans odd choice of words.
Odd can also refer to something that is very strange, weird, or bizarre.
Real-life examples: Works of fantasy like the Harry Potter series often include very odd creatures, such as plant monsters or giant, flying jellyfish. In everyday life, people may display odd behavior, such as walking on their hands down the street.
Don’t Miss: Chapter 7 Test Form A Geometry Answers
Division Of Two Odd Numbers
Division of two odd numbers always results in an odd number if and only if the denominator is a factor of the numerator, or else the number result in decimal point number.
| Odd Odd = Odd |
If we summarise the properties of odd numbers , we have:
| Operation |
|---|
Even for negative odd numbers, consecutive ones will be:
- -5 and -3
- -13 and -11, etc.
How To Quickly Determine The Number In The Middle
The data sets in the examples above only had a few numbers, so we could determine the middle values just by looking at them. For longer sets of data, if we know how many numbers there are in total , we can perform basic division to quickly determine which number is in the middle:
- If there is an odd number of digits in step 1, the result of step 3 indicates the position of the median in the data set
- If there is an even number of digits in step 1, round the result of step 3 up and down to the nearest whole number these two numbers indicate the position of the two middle numbers in the data set. Find the arithmetic mean of these two numbers to find the median
Examples
Case 1 : The data set has 131 digits
We add 131 + 1 = 132, then divide by 2 to get 66, so the 66th digit in the data set is the median.
Case 2 : The data set has 256 digits
We add 256 + 1 = 257, then divide by 2 to get 128.5, so the 128th and 129th digits in the data set are the two middle numbers. We find the arithmetic mean of the values in the 128th and 129th position of the data set. The result is the median.
Don’t Miss: Michael Jackson Biological Children
Properties Of Odd Numbers
If you try to carry out a few BODMAS operations on the odd numbers, can you come to a common conclusion of all of the numbers? Well yes, there does exist a set of properties that applies not only for the odd numbers given in the list of 1 to 200 but are applicable to any odd number that you may come across. Given below is a list of the properties that will always apply for an odd number. Each of these properties can be explained in a detailed way as given below,
- Addition of Two Odd Numbers: The addition of two odd numbers will always give an even number, i.e., the sum of two odd numbers is always an even number. For example, 3 + 5 = 8 .
- Subtraction of Two Odd Numbers: Subtraction of two odd numbers will always give an even number. For example, 7 + 1 = 6 .
- Multiplication of Two Odd numbers: Multiplication of two odd numbers will always give an odd number. For example, 3 × 7 = 21 .
- Division of Two Odd Numbers: Division of two odd numbers will always give an odd number. For example, 33 ÷ 11 = 3 .
Let’s summarize our learning of properties using the table and simulation given below:
| Arithmetic Operation |
| Odd number |
Benefits Of Vedantu Maths Class
At Vedantu, you learn from the expert in that particular subject, but also you get unlimited access to the rated resources like previous papers, sample papers, NCERT textbooks etc. Vedantu provides you with solutions to the textbook exercise according to the CBSE guidelines.
Join Vedantu to learn the reasons behind each step you see in mathematics learning the depth of the concepts help you understand the complexity of the reasoning problems. Also, you get a chance to clear your doubts from the subject expert in one-on-one sessions.
1. What are the things Vedantu offers on its website?
Vedantu is not just an online tuition provider you will find indefinite resources that will assist you in your preparation. After signing up on the Vedantu website or installing an app on your device, you will find solutions to the question papers, important topics to learn, books as per the CBSE syllabus, revision notes and many more. Learning is not limited to the Vednatu, and thats why we consider providing you with more questions to enhance your skills and knowledge.
2. Why is zero not an odd number?
3. Do I need to learn the different properties of the odd numbers?
Yes, it is necessary to learn the different properties of odd numbers. There are only four properties to remember that you will use intensively. Below you read the properties of odd numbers:
-
When you add an odd number to another odd number, a result is always an even number.
E.g., 3 + 3 = 6
E.g., 9 – 5 = 4
Don’t Miss: Introduction To Exponential Functions Common Core Algebra 1 Homework Answers
Unity As An Identity Element
Unity, or the number one, also represents an identity element, which is to say that when combined with another number in a certain mathematic operation, the number combined with the identity remains unchanged. For example, in the addition of real numbers, zero is an identity element as any number added to zero remains unchanged . Unity, or one, is also an identity element when applied to numerical multiplication equations as any real number multiplied by unity remains unchanged . It is because of this unique characteristic of unity that is called the multiplicative identity.
Identity elements are always their own factorial, which is to say that the product of all positive integers less than or equal to unity is unity . Identity elements like unity are also always their own square, cube, and so on. That is to the say that unity squared or cubed is equal to unity .
Unlock This Answer Now
Start your 48-hour free trial to unlock this answer and thousands more. Enjoy eNotes ad-free and cancel anytime.
Already a member? Log in here.
Median is a measure of the center of data. Median is the best measure of center when extreme outliers are involved, because it is less affected by outliers than mean.
To find the median of a data set, we need to arrange the data set in ascending order. After arranging the data, we need to count data points in the data set.
If a data set has an odd number of data points, then its median would be the value of the middlemost data point. For example, the median of a data set with 7 data points would be the value of the 4th data point.
If a data set has an even number of data points, then its median would be the value of the average of the two middlemost data points. For example, the median of a data set with 8 data points would be the average of the 4th and 5th data points.
Don’t Miss: Holt Mcdougal Geometry Book Answers
Examples For Small Values
First we will look at a few examples of the factorial with small values of n:
- 1! = 1
- 2! = 2 x 1 = 2
- 3! = 3 x 2 x 1 = 6
- 4! = 4 x 3 x 2 x 1 = 24
- 5! = 5 x 4 x 3 x 2 x 1 = 120
- 6! = 6 x 5 x 4 x 3 x 2 x 1 = 720
- 7! = 7 x 6 x 5 x 4 x 3 x 2 x 1 = 5040
- 8! = 8 x 7 x 6 x 5 x 4 x 3 x 2 x 1 = 40320
- 9! = 9 x 8 x 7 x 6 x 5 x 4 x 3 x 2 x 1 = 362880
- 10! = 10 x 9 x 8 x 7 x 6 x 5 x 4 x 3 x 2 x 1 = 3628800
As we can see the factorial gets very large very quickly. Something that may seem small, such as 20! actually has 19 digits.
Factorials are easy to compute, but they can be somewhat tedious to calculate. Fortunately, many calculators have a factorial key . This function of the calculator will automate the multiplications.
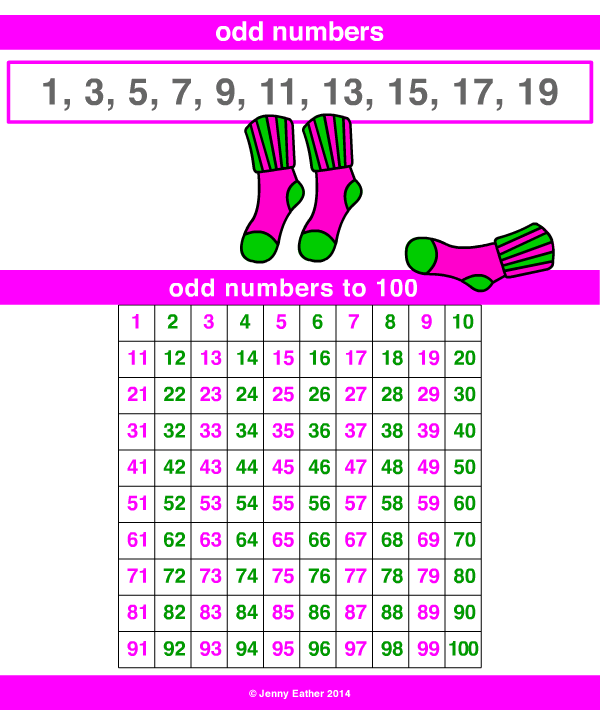
What Are Odd Numbers
Odd numbers definition is given as those numbers which cannot be divided into two parts equally.
Odd numbers are basically integer numbers that cannot be categorized into groups of two each. For example: 1, 3, 5, 7, etc. Let’s visualize it using an example of footwear and cherries. Let us assume that we have footwear in counts of 1, 3, 5, and 7. On the other hand, we have cherries in the counts of 2, 4, 6, and 8. Look at the image given below in order to understand how the pairing of these numbers will work.
It is to note here that the footwear, when odd in number, does not form a pair entirely. One among all remains unpaired. On the contrary, even numbers are those numbers that can be divided into two parts equally. For example: 2, 4, 6, 8, etc.
Don’t Miss: Accuracy And Precision Worksheet Answer Key