Venn Diagram Of Empty Set
Venn Diagrams are considered the most effective tool to represent the relationships between sets, especially finite sets. We can represent an empty set through a Venn diagram. The below-given image illustrates the relation as follows:
Consider a set X = and a set Y =
We can see from the above-given Venn diagram that there are no common elements that are intersecting elements between the two sets X and Y, hence we can conclude that the intersection between these two sets is empty. Thus, X Y = .
Containing Something And Nothing
This is really just more discussion about the concept of subsets. Once again, is a subset of does not mean is a member of.
Consider a set S described as .
It “possesses something,” in your words, because it “possesses” A, B, and C. Now, does it make sense to say that it “possesses nothing”? Well, maybe, but that’s incredibly vague terminology, so let’s go back to what’s actually being claimed: “the null set is a subset of all sets, and therefore the null set is a subset of S.”
Does this mean that the null set is a member of the set S? No, because S’s members are A, B, and C, and as far as we know, none of those are the null set.
But it does mean that S has within it every member of the null set. In other words, there are no members of the null set that are not members of S. How do we know this? Because there are no members of the null set… period.
There are some issues with the intuitive definition of a set which you have taken as the basis for your understanding.
It is possible to say “a set is a well-defined collection of objects”. What do we mean by “well-defined”? – Well that is the whole question of the foundations of set theory. In studying the question, mathematicians have found it necessary to have some control over the nature of the “objects” in the “collection” – as always with mathematical definitions, we have to be absolutely precise. So mathematics proceeds by building standard sets as a model.
It’s also very convenient to have an empty set. For example.
Property : Subset Of Any Set
An empty set can be considered as the subset of any given set. For any finite or infinite set X, if we exclude all the possible subsets of set X, then we can always include an empty set in this.
For example,
- Consider a finite set X = .
- All the possible subsets of this set X can be written as: X = , X = , X = , X = , X = , X = , X = .
- As you can see, the empty set has also been included along with the other subsets because of this property.
You May Like: What Is Human Biology Class Like
Null Sets $\\ \subset\\$
Regarding null sets, I’m wondering if anyone can explain this $\\} \subset \\}$ I don’t understand how the left set is a proper set of the right set.
In particular, I’m wondering what the extra brace on the left means and how it is different from say just plain $\$. It seems if I disregard the outer brace in the left hand set, my answer matches that of the answer key .
I’m posting this again because it was in the wrong stackexchange.
Thanks!
- 3$\begingroup$Think of the curly braces used in set notation as specifying a box. An empty box isn’t the same thing as a box that contains an empty box, right? So if you put each of those into a pair of even bigger boxes, the result is still two different sorts of things, right?$\endgroup$
The only element of $\\}$ is $\$ which is in $\\}$.
Thus it is a subset…
a general rule:$$a \in S \Rightarrow \ \subset S$$since, in your example$$\ \in \\}$$the result follows
When you see curly braces around something, that means you have a set with that something as an element. For example, $\$ is a set, and its only element is $S$. Similary, $\$ is a set, and its only element is $1$. $\$ is a set, and it has two elements: Hi and .
Similarly, when you have $\ \}$, this is a set, and its only element is $\$. It just so happens that the element of our set is a set itself, but there is nothing wrong with that.
So, every element of $\ \}$ is an element of $\ \}$.
So, this explains why $\ \} \subset \ \}$.
Insert Null Set Symbol In Word/excel/powerpoint
For Microsoft Office users like Word, Excel, or PowerPoint, you can use this simple mouse navigation to quickly insert the Empty Set sign.
Below steps will show you how to insert the Symbol for Empty Set in Word or Excel or PowerPoint.
- Open your Word or Excel or PowerPoint document.
- Go to the Insert tab.
- At the far-right section, youll see the Symbols group. Select Symbol> More Symbols.
The Symbol window will appear as below.
- From this window, you can find the symbol . To find it easily, type 00D8 in the Character code: box. As soon as you type the character code, the Empty Set sign will appear selected.
- Click the Insert button to insert it into your Word document.
- Close the Symbol dialog.
These are the steps you may use to insert the this and any other symbol in Microsoft Word or other Office apps in both Windows and Mac OS.
You May Like: Algebra Lineal Ejercicios Resueltos Numeros Complejos
Properties Of Empty Set
The most important properties of an empty set are listed below:
Cardinality: The number of elements of the empty set or the cardinality of empty set is zero, || = 0
Empty set is a subset of every set: For any set A, the empty set is a subset of A, i.e. A A
Empty set subset: The only subset of an empty set is the empty set itself, i.e. A A =
Cartesian product with empty set: The Cartesian product of A and the empty set is the empty set, i.e. A × = A
Power set of empty set: The power set of the empty set is the set containing only the empty set, i.e. 2 =
Union with Empty set: The union of A with the empty set is the set A itself, i.e. A = A A
Intersection with Empty: The intersection of A with the empty set is again the empty set, i.e. A = A
Equal Vs Unequal Sets
Now, if two sets contain precisely the same elements, regardless of the order or possible repetition, then they are considered equal.
Suppose set A = and set B = . Sets A and B are equal because they contain the exact same elements, namely 1, 3, and 5. Remember, order and multiplicity of elements dont matter.
Lets look at another example. Determine if the sets A and B are equal
Equal Sets Example
Unequal Sets Example
Don’t Miss: How To Solve Acceleration In Physics
Cardinality Of Empty Set:
The cardinality of the empty set is always zero.
The cardinality is defined as the set size or the total number of elements in the set. Since empty sets contain no elements, hence they have a zero cardinality. This is shown below:
|$\phi$| = 0
Therefore, according to the above relation, the cardinality of the empty set will always be zero.
Lets consider an example based on this property.
Example 8
Find set Xs cardinality where set X = .
Solution
For solving this example, we will first simplify the set.
As there are no odd multiples of 10 in existence, hence the set is empty.
The cardinality can be found as:
|$\phi$| = |x : x is an odd multiple of 10|
|$\phi$ | = 0
Properties Of The Empty Set
Since there is only one empty set, it is worthwhile to see what happens when the set operations of intersection, union, and complement are used with the empty set and a general set that we will denote by X. It is also interesting to consider subset of the empty set and when is the empty set a subset. These facts are collected below:
- The intersection of any set with the empty set is the empty set. This is because there are no elements in the empty set, and so the two sets have no elements in common. In symbols, we write X = .
- The union of any set with the empty set is the set we started with. This is because there are no elements in the empty set, and so we are not adding any elements to the other set when we form the union. In symbols, we write X U = X.
- The complement of the empty set is the universal set for the setting that we are working in. This is because the set of all elements that are not in the empty set is just the set of all elements.
- The empty set is a subset of any set. This is because we form subsets of a set X by selecting elements from X. One option for a subset is to use no elements at all from X. This gives us the empty set.
Also Check: Is Paris Jackson Michael’s Biological Daughter
What Is The Empty Set In Set Theory
- M.S., Mathematics, Purdue University
- B.A., Mathematics, Physics, and Chemistry, Anderson University
When can nothing be something? It seems like a silly question, and quite paradoxical. In the mathematical field of set theory, it is routine for nothing to be something other than nothing. How can this be?
When we form a set with no elements, we no longer have nothing. We have a set with nothing in it. There is a special name for the set which contains no elements. This is called the empty or null set.
How To Get The Empty Set Sign
Even though theres no dedicated key for the Empty Set symbol on the keyboard, you can still get this symbol using the keyboard.
If you are also using any of the Microsoft Office or Google apps, there are quick navigations you can perform to insert this symbol in Word, Excel, PowerPoint, or Google Docs.
Without any further ado, below are the various ways to get this symbol whether with your keyboard or using the navigation in MS Office or Google Docs.
Don’t Miss: What I Learned In Math
How To Represent An Empty Set
In set theory, empty sets are represented by using the empty curly brackets that are generally used to denote sets. However, since empty sets are unique kinds of sets, these sets can also be represented by using the special character . Let us consider an example of an empty set A containing multiples of 5 between 6 and 8. Since there are no multiples of 5 between 6 and 8, so the given set is an empty set. Set A =
- The notation of this empty set is: A = .
- The same empty set A can also be represented using the following notations: Empty set = or, X = .
- We can use the special symbol to represent an empty set.
Why Are Sets Important

Sets are the fundamental property of mathematics. Now as a word of warning, sets, by themselves, seem pretty pointless. But it’s only when we apply sets in different situations do they become the powerful building block of mathematics that they are.
Math can get amazingly complicated quite fast. Graph Theory, Abstract Algebra, Real Analysis, Complex Analysis, Linear Algebra, Number Theory, and the list goes on. But there is one thing that all of these share in common: Sets.
Recommended Reading: What Does Integrated Math Mean
What Are The Properties Of Sets In Set
Different properties associated with sets in math are,
- Commutative Property: A U B = B U A and A B = B A
- Associative Property: C = A and U C = A U
- Distributive Property: A U = and A = U
- Identity Property: A U = A and A U = A
- Complement Property: A U A’ = U
- Idempotent Property: A A = A and A U A = A
What Are The Properties Of An Empty Set
Here are some of the important properties of empty sets:
- An empty set can be considered as the subset of any given set.
- The union operation between any set and an empty set will always result in the set itself.
- The intersection operation between any set and an empty set will always result in the set itself.
Also Check: What Is Chain Migration In Human Geography
Empty Set As Element Of A Set
Since the empty set is a set, it can be an elementof another set
Example
Consider this: although “$\varnothing$” and “$\$” both denote the empty set, $\left\$ is notthe empty set it is the set whose only element is the empty set. The set $\left\$ is in fact a singleton set.
Example
The set of subsets of the two-element set $\$ is the set \
More about “only one empty set”
Because there is only one empty set, $\left\}\,|\,^}\lt 0 \right\}$, $$ and $$ are allexactly the same set. The two statements below say this in two different ways. I recommend that you think about them until you understand that they are really saying the same thing.
- $\left\}\,|\,^}\lt 0 \right\}==$.
- “$\left\}\,|\,^}\lt 0 \right\}$”, $$ and$$ are three differentrepresentations of the same set, namely the empty set.
Representation Of Empty Set Through Venn Diagram
Venn Diagrams are the most effective medium to represent sets, especially finite sets. These diagrams are also used to depict the relations of union and intersection between two sets.
An empty set can be represented through a Venn diagram and the relation of the intersection. The relation and presentation are as follows:
Consider a set A = and a set B = .
As it is clear from the Venn diagram that there are no common or intersecting elements between the two sets, hence the intersection between the two sets is empty.
AB = $\phi$
Lets consider an example relating to this concept.
Example 4
Let set A = and set B = . Find the intersection between the 2 sets.
Solution
We can solve this example with the help of a Venn diagram.
The two sets are indicated below. It is evident from the Venn diagram that there are no common or intersecting elements between the two sets. Hence, the intersection of the two sets is an empty set.
AB = $\phi$
Don’t Miss: Geometry Dash 1.9 Sneak Peek
Video Tutorial W/ Full Lesson & Detailed Examples
1 hr 28 min
- Introduction to Video:Sets and Subsets
- 00:00:41
- Determine if the sets are equal
- 00:24:22 Write each set using either roster method or set builder notation
- 00:33:56
- Are the pair of sets equal?
- 00:52:06 Determine which are subsets
- 00:56:27
- Intro to Cardinality and Power Sets
- 01:08:59 What is the cardinal number of each set?
- 01:13:00 Find the power set and determine its cardinality
- 01:17:40 Explanation of n-tuple and Cartesian Product
- 01:19:15 Discover the cartesian product
- Practice Problems with Step-by-Step Solutions
- Chapter Tests with Video Solutions
Get access to all the courses and over 450 HD videos with your subscription
Monthly and Yearly Plans Available
What Are The Types Of Sets
Sets differ from each depending upon elements present in them. Based on this, we have the following types of sets. They are singleton sets, finite and infinite sets, empty or null sets, equal sets, unequal sets, equivalent sets, overlapping sets, disjoint sets, subsets, supersets, power sets, and universal sets.
Also Check: The Algebra Of Happiness Scott Galloway
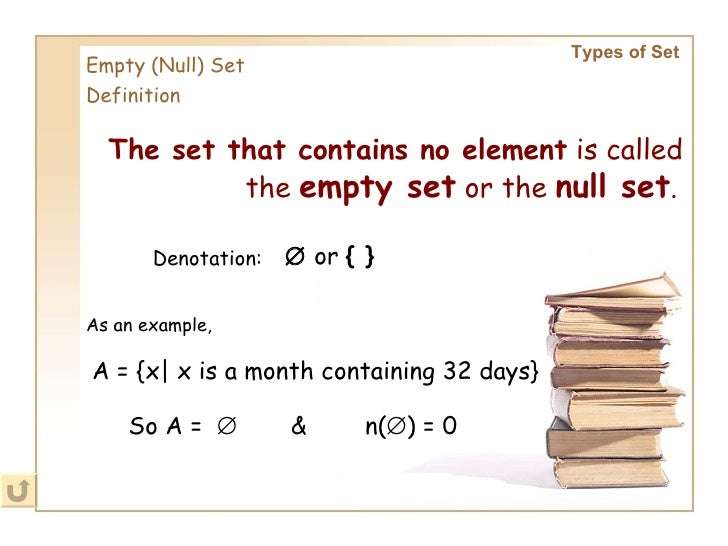
Empty Set Or Null Set
In set theory the concept of an empty set or null set is very important and interesting. Its definition is as follows: a set which contains no elements is called as empty set or null set, and it is sometimes known as void set or vacuous set. It is usually denoted by $$\emptyset $$ inspired by the letter Ø in the Norwegian and Danish alphabets, and not related to the Greek letter .
This definition of empty seems to be opposing the definition of a set, but this apparent contradiction makes sense when we understand the meanings of well defined objects. By well defined objects we mean objects which can be considered as the members of the set under consideration.
For example, a bottle is not a well defined object for a jewelry set. Similarly, a chair is not a well defined object for a tea set. But if we consider the set of necessary things of a house, then both of the above mentioned things are well defined objects for such a set.
Thus, if an object does not exist then it is a well defined object for an empty set.
For example,
Empty Set And Subsets

So let’s go back to our definition of subsets. We have a set A. We won’t define it any more than that, it could be any set. Is the empty set a subset of A?
Going back to our definition of subsets, if every element in the empty set is also in A, then the empty set is a subset of A. But what if we have no elements?
It takes an introduction to logic to understand this, but this statement is one that is “vacuously” or “trivially” true.
A good way to think about it is: we can’t find any elements in the empty set that aren’t in A, so it must be that all elements in the empty set are in A.
So the answer to the posed question is a resounding yes.
The empty set is a subset of every set, including the empty set itself.
Read Also: What Does G Represent In Physics