Solving Exponential Equations Algebra 2

How To Solve Exponential Equations Using Logarithms

In our previous lesson, you learned how to solve exponential equations without logarithms. This time around, we want to solve exponential equations requiring the use of logarithms. Why? The reason is that we cant manipulate the exponential equation to have the same or common base on both sides of the equation. If you encounter such type of problem, the following are the suggested steps:

However We Will Also Use In The Calculation The Common Base Of 10 And The Natural Base Of Colorrede Denoted By Colorblueln Just To Show That In The End They All Have The Same Answers

Solving exponential equations same base worksheet answers. The square root of a number x is the same as x raised to the 05 th power sqrtxsqrt2xxfrac12. Mathematically proficient students check their answers to problems using a different method and they continually ask themselves Does this make sense They can understand the approaches of others to solving complex problems and identify correspondences between different approaches.

With the same base then the problem can be solved by simply dropping the logarithms. If you like this Page please click that 1 button too. These worksheets explain how to multiply and divide exponents as well as how to consolidate exponents that have the same base.

Solving Equations with Radicals and Exponents. Personal Bankruptcy algebra 2 test answers solving numerical equations software. Teen Quote Coloring Pages Printable.

Addition and subtraction of fractions. Glyn and his staff helped him focus on the math problems break them down and really understand the concepts needed to get the answer correct. Kindergarten math worksheets addition.

Thank you for your support. What this ends up looking like is something that appears to be. We have a whole lot of quality reference tutorials on subjects starting from intermediate algebra syllabus to equation.

If you are not logged into your Google account ex gMail Docs a login window opens when you click on 1. Log Base of 5. How can i find worksheet answers.

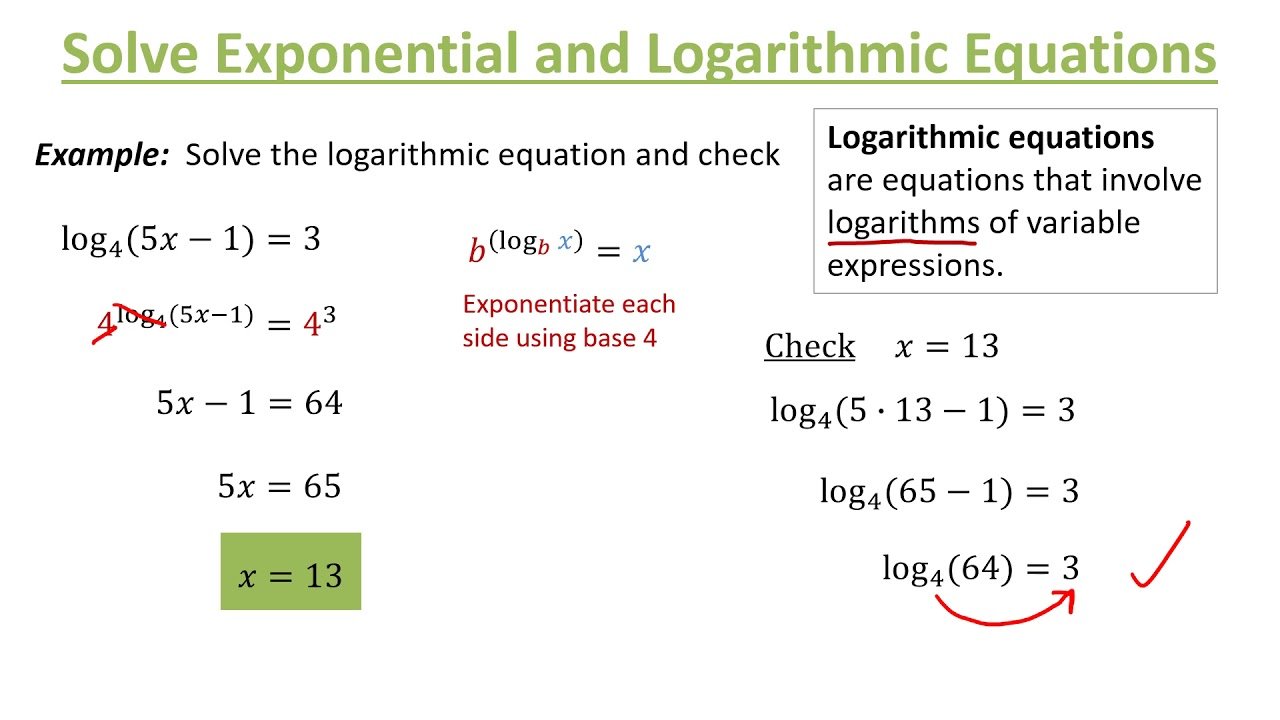
Example: Solving Exponential Functions In Quadratic Form

Solve ^-^=56.

\begin^-^=56\hfill \\ ^-^-56=0\hfill & \text.\hfill \\ \left\left=0\hfill & \text.\hfill \\ ^+7=0\text^-8=0 & \text.\hfill \\ ^=-7}^=8\hfill & \text.\hfill \\ ^=8\hfill & \text.\hfill \\ x=\mathrm8\hfill & \text.\hfill \end

Analysis of the Solution

When we plan to use factoring to solve a problem, we always get zero on one side of the equation because zero has the unique property that when a product is zero, one or both of the factors must be zero. We reject the equation ^=-7 because a positive number never equals a negative number. The solution x=\mathrm\left is not a real number and in the real number system, this solution is rejected as an extraneous solution.

Read Also: Eoc Fsa Warm Ups Algebra 1 Answers

Example: Solving An Equation Containing Powers Of Different Bases

Solve ^=^.

\begin\text^=^\hfill & \text.\hfill \\ \text\mathrm^=\mathrm^\hfill & \text.\hfill \\ \text\left\mathrm5=x\mathrm4\hfill & \text.\hfill \\ \textx\mathrm5+2\mathrm5=x\mathrm4\hfill & \text.\hfill \\ \textx\mathrm5-x\mathrm4=-2\mathrm5\hfill & \textx\textx\text.\hfill \\ x\left=-2\mathrm5\hfill & \textx.\hfill \\ \textx\mathrm\left=\mathrm\left\hfill & \text.\hfill \\ \textx=\frac\left}\left}\hfill & \textx.\hfill \end

What Are Exponential Equations

An exponential equation is an equation with exponents where the exponent a part of the exponent is a variable. For example, 3x = 81, 5x – 3 = 625, 62y – 7 = 121, etc are some examples of exponential equations. We may come across the use of exponential equations when we are solving the problems of algebra, compound interest, exponential growth, exponential decay, etc.

Don’t Miss: Figure Ground Psychology

Exponential Equations To Logarithmic Form

We know that logarithms are nothing but exponents and vice versa. Hence an exponential equation can be converted into a logarithmic equation. This helps in the process of solving an exponential equation with different bases. Here is the formula to convert exponential equations into logarithmic equations.

bx = a log\ a = x

Solving Exponential Equations With Different Bases

Sometimes, the bases on both sides of an exponential equation may not be the same cannot be made the same. We solve the exponential equations using logarithms when the bases are not the same on both sides of the equation. For example, 5x = 3 neither has the same bases on both sides nor the bases can be made the same. In such cases, we can do one of the following things.

• Convert the exponential equation into the logarithmic form using the formula bx = a log\ a = x and solve for the variable.
• Apply logarithm on both sides of the equation and solve for the variable. In this case, we will have to use a property of logarithm, log am = m log a.

We will solve the equation 5x = 3 in each of these methods.

Method 1:

We will convert 5x = 3 into logarithmic form. Then we get,

log\ 3 = x

x = /

Method 2:

We will apply log on both sides of 5x = 3. Then we get

log 5x = log 3

Using the property log am = m log a on the left side of the equation, we get

x log 5 = log 3

Dividing both sides by log 5,

x = /

Important Notes:

Here are some important notes with respect to the exponential equations.

Related Topics:

Also Check: Geometry Segment Addition Postulate Worksheet

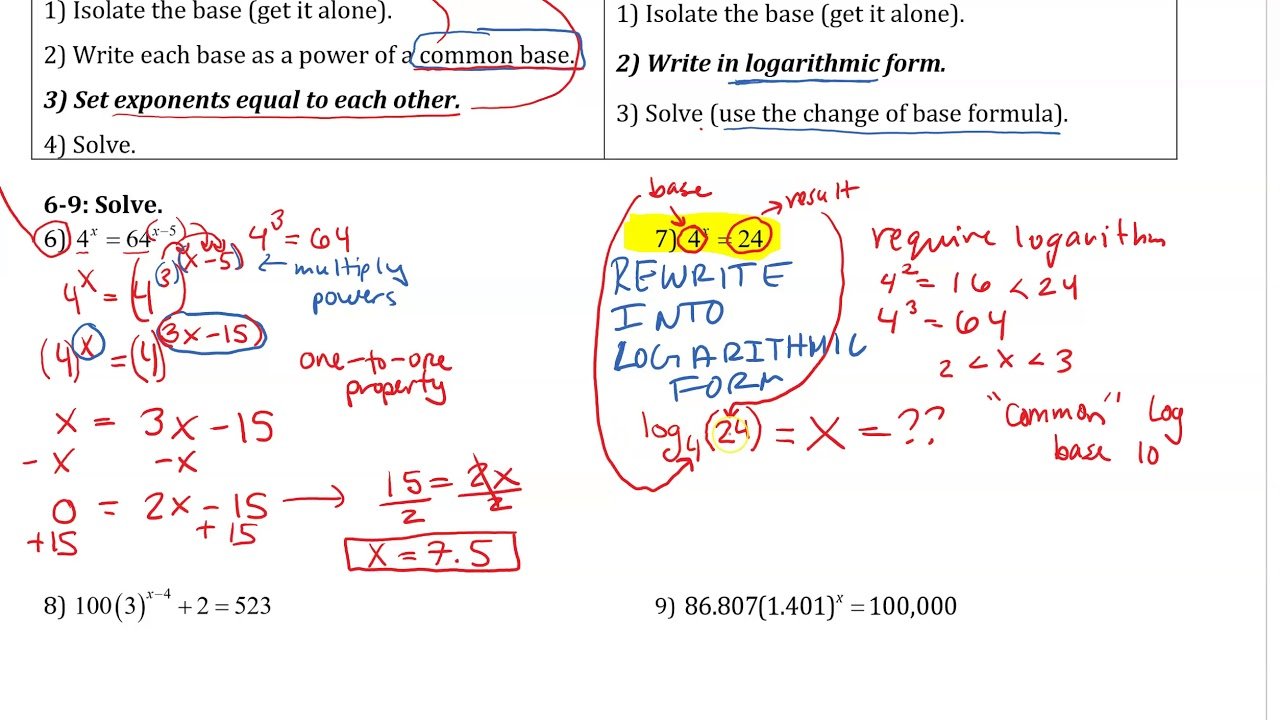
Rewriting Equations So All Powers Have The Same Base

Sometimes the common base for an exponential equation is not explicitly shown. In these cases we simply rewrite the terms in the equation as powers with a common base and solve using the one-to-one property.

For example, consider the equation 256=^. We can rewrite both sides of this equation as a power of 2. Then we apply the rules of exponents along with the one-to-one property to solve for x:

\begin256=^\hfill & \hfill \\ ^=^\right)}^\hfill & \text.\hfill \\ ^=^\hfill & \text.\hfill \\ 8=2x – 10\hfill & \text.\hfill \\ 18=2x\hfill & \text.\hfill \\ x=9\hfill & \text.\hfill \end

S To Solve Exponential Equations Using Logarithms

1) Keep the exponential expression by itself on one side of the equation.

2) Get the logarithms of both sides of the equation. You can use any bases for logs.

3) Solve for the variable. Keep the answer exact or give decimal approximations. In addition to the steps above, make sure that you review the Basic Logarithm Rules because you will use them in one way or another.

Lets go over some examples!

Recommended Reading: Eoc Fsa Practice Test Algebra 1 Calculator Portion

Solving Logarithmic Equations Worksheet Worksheets Solving Exponential Equations Openlay Studying Math Teaching Algebra Solving Linear Equations

Solving logarithmic and exponential equations worksheet. Log 5 x1 log 5 x 2 4. 001t 0 to answer the. 2 1 log 49 x 2.

Log 2×1 log x 1 Solve each equation. Graph each of the following functions. To solve an exponential equation first isolate the exponential expression then take the logarithm of both sides of the equation and solve for the variable.

Algebra 2 Worksheets Exponential And Logarithmic Functions Worksheets Algebra 2 Worksheets Simplifying Algebraic Expressions Logarithmic Functions. How to solve a logarithmic equation using properties of logarithms. Solving exponential equations with logarithms.

Give an exact solution. Solving Exponential Logarithmic Equations Properties of Exponential and Logarithmic Equations Let be a positive real number such that and let and be real numbers. Find the value of y.

This circuit worksheet consists of 20 questions of solving exponential equations including using the same base and taking the log or ln. Exponential and logarithmic equations worksheet. Precalculus Solving Exponential And Logarithmic Equations Worksheet.

This is the easier sort of exponential equation. It is possible to write both sides of the equation with the same base. ARTS VISA 7 5.

Some of the worksheets below are exponential and logarithmic. Log 4 3×2 2 6. 1 log 5 x log 2x 9 2 log 10 4x log 10 3x 3 log 4p 2 log 5p 5 4 log 4k 5 log 2k 1 5 log 2a 9 log 7 4a 6 2log 7 2r 0 7 10 log 3 n 3 10 8 2log 5.

Solving Exponential Equations With Same Bases

Sometimes, an exponential equation may have the same bases on both sides of the equation. For example, 5x = 53 has the same base 5 on both sides. Sometimes, though the exponents on both sides are not same, they can be made the same. For example, 5x = 125, though doesn’t have the same bases on both sides of the equation, they can be made the same by writing it as 5x = 53 . To solve the exponential equations in each of these cases, we just apply the property of equality of exponential equations, using which, we set the exponents to be the same and solve for the variable.

Here is another example where the bases are not same, but can be made the same.

Example: Solve the exponential equation 7y + 1 = 343y.

Solution:

We know that 343 = 73. Using this, the given equation can be written as,

7y + 1 = y

7y + 1 = 73y

Now the bases on both sides are the same. So we can set the exponents to be the same.

y + 1 = 3y

Subtracting y from both sides,

2y = 1

Dividing both sides by 2,

y = 1 /2

You May Like: Ap Human Geography Self Study

How To Find Exponential Functions

Finding the equation of exponential functions is often a multi-step process, and every problem is different based upon the information and type of graph we are given. Given the graph of exponential functions, we need to be able to take some information from the graph itself, and then solve for the stuff we are unable to take directly from the graph. Below is a list of all of the variables we may have to look for, and how to usually find them:

a solve for it using algebra, or it will be given

b solve for it using algebra, or it will be given

c let x = 0 and imagine “c” is not there, the value of y will equal the y-intercept now count how many units the y value for the y-intercept is from the y-axis, and this will equal “c”

d solve for it using algebra

k equal to the value of the horizontal asymptote

Of course, these are just the general steps you need to take in order to find the exponential function equation. The best way to learn how to do this is to try some practice problems!

Exponential Functions Examples:

Now let’s try a couple examples in order to put all of the theory we’ve covered into practice. With practice, you’ll be able to find exponential functions with ease!

Example 1:

Determine the exponential function in the form y y=abx of the given graph.

Step 1: Solve for “a”

Step 2: Solve for “b”

Step 3: Write the Final Equation

Now we that we have found all of the necessary variables, all that’s left is to write out our final equation in the form y

Use The Graph To Solve The Exponential Equation

Use the graph to solve the equation 3=50.85x.

The graph shows all x-y points that satisfy the function rule y=50.85x. Let’s compare the function rule and the equation.Function rule:y=50.85xEquation:3=50.85xThe only difference between these two equalities is that the independent variable, y, is replaced by a 3 in the equation. Thus, we solve the equation by finding the x-coordinate of any point on the graph that has the y-coordinate 3.

We can identify one such point in the graph. Let’s now find the x-coordinate of this point graphically.

This x-coordinate is not easily read from the graph, so we’ll have to make an approximation. It’s just a bit bigger than 3, so we’ll use 3.1. This means that an approximate solution to the equation is x3.1. We can verify this by substituting it into equation to see if a true statement is made.

Recommended Reading: What Is The Geography Of Paraguay

Example: 24 = 2 2 2 2 = 16

• In words: 24 could be called “2 to the fourth power” or “2 to the power 4” or simply “2 to the 4th”

Exponents make it easier to write and use many multiplications

Example: 96 is easier to write and read than 9 × 9 × 9 × 9 × 9 × 9

You can multiply any number by itself as many times as you want using exponents.

Try here:

Examples Of How To Solve Exponential Equations Using Logarithms

Example 1: Solve the exponential equation } = 21.

The good thing about this equation is that the exponential expression is already isolated on the left side. We can now take the logarithms of both sides of the equation. It doesnt matter what base of the logarithm to use. The final answer should come out the same. The best choice for the base of log operation is 5 since it is the base of the exponential expression itself. However, we will also use in the calculation the common base of 10, and the natural base of \colore just to show that in the end, they all have the same answers.

• Log Base of 5

• Log Base of e

Example 2: Solve the exponential equation 2\left = 12 .

As you can see, the exponential expression on the left is not by itself. We must eliminate the number 2 that is multiplying the exponential expression. To do that, divide both sides by 2. That would leave us just the exponential expression on the left, and 6 on the right after simplification.

Its time to take the log of both sides. Since the exponential expression has base 3, thats the convenient base to use for log operation. In addition, we will also solve this using the natural base e just to compare if our final results agree.

• Log Base of 3

• Log Base of e

Example 3: Solve the exponential equation 2\left – 7 = 13 .

Now isolate the exponential expression by adding both sides by 7, followed by dividing the entire equation by 2.

Example 4: Solve the exponential equation ^}} \right)^x} + 3 = 53 .

Recommended Reading: Who Are Paris Jackson’s Biological Parents

Example: Solve An Equation Of The Form Y=a^

Solve 100=20^.

\begin100\hfill & =20^\hfill & \hfill \\ 5\hfill & =^\hfill & \text\text\hfill \\ \mathrm5\hfill & =\mathrm^}\hfill & \text\hfill \\ \mathrm5\hfill & =2t\hfill & \text\mathrm\left\text^\text\text\hfill \\ t\hfill & =\frac5}\hfill & \textt\text\hfill \end

Analysis of the Solution

Using laws of logs, we can also write this answer in the form t=\mathrm\sqrt. If we want a decimal approximation of the answer, then we use a calculator.

Solving Exponential Equations With The Different Bases

University of MichiganRuns his own tutoring company

Carl taught upper-level math in several schools and currently runs his own tutoring company. He bets that no one can beat his love for intensive outdoor activities!

• Transcript

Sometimes we are given exponential equations with different bases on the terms. In order to solve these equations we must know logarithms and how to use them with exponentiation. We can access variables within an exponent in exponential equations with different bases by using logarithms and the power rule of logarithms to get rid of the base and have just the exponent.

Also Check: How To Avoid Parallax Error In Physics