How To Solve Acceleration Problems In Physics

College Acceptance Essay Formats

literature review on diabetic eye diseasemla research paper topic proposal outlinepoor children essay writingdoing your homework that start with zsample business plan for school projectinteresting topic for term papersrandom assignment stataart comparison essaysolving problems with linear equations in 1 variable calculatorresearch process and terminology paper design

Example 5 Calculating Displacement: How Far Does A Car Go When Coming To A Halt

On dry concrete, a car can decelerate at a rate of 7.00 m/s2, whereas on wet concrete it can decelerate at only 5.00 m/s2. Find the distances necessary to stop a car moving at 30.0 m/s on dry concrete and on wet concrete. Repeat both calculations, finding the displacement from the point where the driver sees a traffic light turn red, taking into account his reaction time of 0.500 s to get his foot on the brake.

Strategy

Draw a sketch.

Figure 9.

In order to determine which equations are best to use, we need to list all of the known values and identify exactly what we need to solve for. We shall do this explicitly in the next several examples, using tables to set them off.

Solution for

1. Identify the knowns and what we want to solve for. We know that v0 = 30.0 m/s v = 0 a = -7.00 m/s2 . We take x0 to be 0. We are looking for displacement x, or xx0.

2. Identify the equation that will help up solve the problem. The best equation to use is

This equation is best because it includes only one unknown, x. We know the values of all the other variables in this equation.

3. Rearrange the equation to solve for x.

4. Enter known values.

Thus,

x

Solution for

This part can be solved in exactly the same manner as Part A. The only difference is that the deceleration is 5.00 m/s2. The result is

x

Solution for

1. Identify the knowns and what we want to solve for. We know that \bar=30.0 \text treaction = 0.500 s areaction = 0. We take x0-reaction = to be 0. We are looking for xreaction.

Cylinder Equations To Solve Pulley Tension Problems

Only one equation and that is along Y-axis.W2 T = maNow combining equation 2 and 3, we getmg Ma = maor, a = / Here in equation 4, we get the expression of the acceleration of the cylinder and the cart.Now again from equation 2 and 4 we get a complete expression of the tension T in the rope. T = Ma = M . /or, T = / .

So the acceleration of the cart is = /And the tension in the rope is /

Also Check: What Does Floor Mean In Math

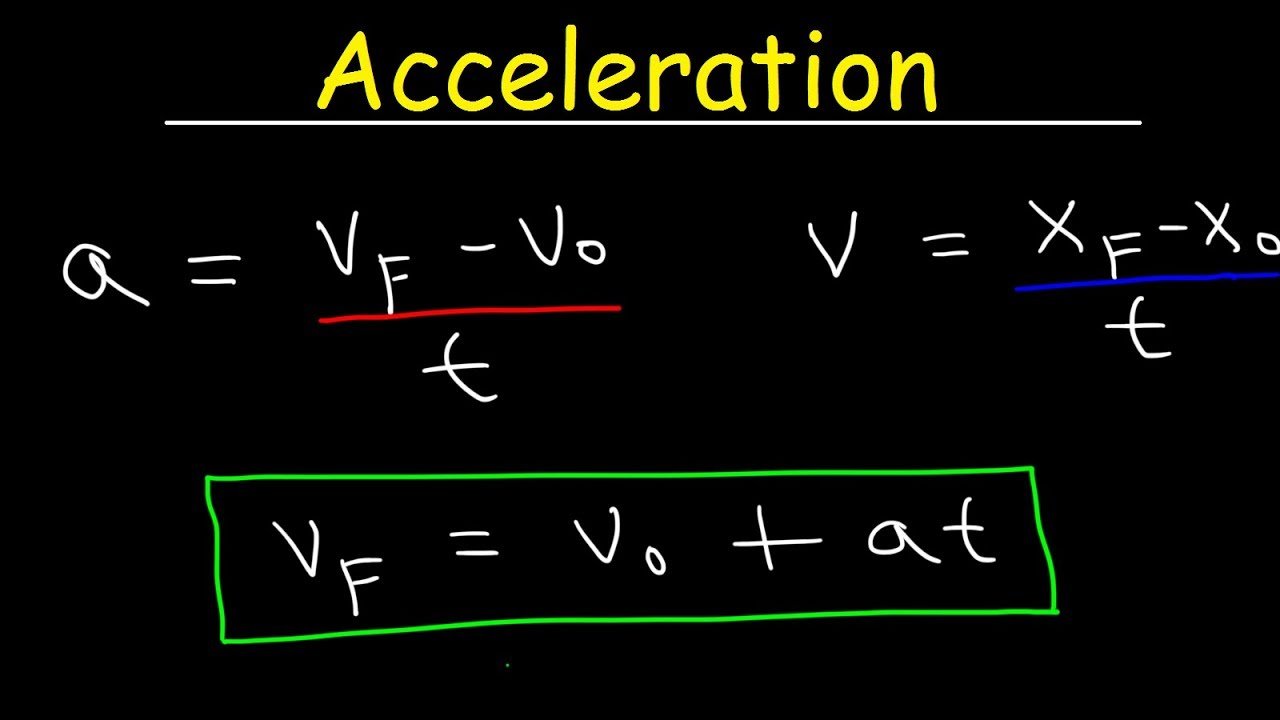
Solving For Final Velocity From Distance And Acceleration

A fourth useful equation can be obtained from another algebraic manipulation of previous equations. If we solve v

Strategy

Solution

vxxa

Second, we substitute the knowns into the equation v

Significance

An examination of the equation v ) can produce additional insights into the general relationships among physical quantities:

• The final velocity depends on how large the acceleration is and the distance over which it acts.
• For a fixed acceleration, a car that is going twice as fast doesnât simply stop in twice the distance. It takes much farther to stop.

Example 2 Calculating Final Velocity: An Airplane Slowing Down After Landing

An airplane lands with an initial velocity of 70.0 m/s and then decelerates at 1.50 m/s2 for 40.0 s. What is its final velocity?

Strategy

Draw a sketch. We draw the acceleration vector in the direction opposite the velocity vector because the plane is decelerating.

Figure 4.

1. Identify the knowns. v0 = 70.0 m/s, a = 1.50 m/s2, t = 40.0 s.

2. Identify the unknown. In this case, it is final velocity, vf.

3. Determine which equation to use. We can calculate the final velocity using the equation v=_+.

4. Plug in the known values and solve.

Discussion

The final velocity is much less than the initial velocity, as desired when slowing down, but still positive. With jet engines, reverse thrust could be maintained long enough to stop the plane and start moving it backward. That would be indicated by a negative final velocity, which is not the case here.

Figure 5. The airplane lands with an initial velocity of 70.0 m/s and slows to a final velocity of 10.0 m/s before heading for the terminal. Note that the acceleration is negative because its direction is opposite to its velocity, which is positive.

In addition to being useful in problem solving, the equation v=_+\text gives us insight into the relationships among velocity, acceleration, and time. From it we can see, for example, that

Recommended Reading: Unit 1 Homework 2 Segment Addition Postulate Answer Key

Summary Of Kinematic Equations

Before we get into the examples, letâs look at some of the equations more closely to see the behavior of acceleration at extreme values. Rearranging Equation 3.12, we have

From this we see that, for a finite time, if the difference between the initial and final velocities is small, the acceleration is small, approaching zero in the limit that the initial and final velocities are equal. On the contrary, in the limit t for a finite difference between the initial and final velocities, acceleration becomes infinite.

Similarly, rearranging Equation 3.14, we can express acceleration in terms of velocities and displacement:

Thus, for a finite difference between the initial and final velocities acceleration becomes infinite in the limit the displacement approaches zero. Acceleration approaches zero in the limit the difference in initial and final velocities approaches zero for a finite displacement.

Case Study 4 Elevator Problems In Physics

The elevator is now moving downwards with an acceleration a.

So again in this case there will be a non zero net force working on the system.

As the elevator is moving downwards, i.e. in the direction of Weight, therefore the net force would be W R downwards.

Following the equation obtained from Newtons second law of motion , we can write here:

W R = m aor, mg R = maor, R = m

So its evident that the Reaction force is not equal to the Weight in this case as well. Now its less than the Weight of the person.

Therefore in this case the person will feel lighter than his normal weight.

Recommended Reading: What Is Span Linear Algebra

Case Study With Pseudo Force

Now as said above the above listed case studies are analyzed considering the observer is on aninertial frame of reference.We have discussed one more intersting elevator case studywith respect to an observer who is in a non-inertial frame of reference. We have applied the concepts of Pseudo force to apply Newtons laws in that case.

**Read the complete case study here:

**One set of numerical elevator problemsis listed in the following paragraph.

You may solve these using the above case studies.Watch for the next version for some harder numerical problems.Happy reading and problem solving.Please note: who is searching for Lift problems this tutorial will help you.

Case Study 3 Elevator Problems In Physics

The elevator is now moving upwards with an acceleration say a.

So obviously in this case there will be a non zero net force working on the system.

As the elevator is moving upwards, i.e. in the direction of Reaction force, therefore the net force would be R W upwards. Following the equation obtained from Newtons second law , we can write here:

R W = m aor, R = m

So its very clear that unlike the first two cases, the Reaction force is no more equal to the Weight only. Now its more than the Weight of the person.

Therefore in this case the person will feel heavier than his normal weight.

Recommended Reading: What Is Relationship In Math

How To Solve Physics Problems Involving Multiple Pulleys Using The Conservation Of String Trick

Well show you how to solve problems involving systems of pulleys.

In problems with systems of pulleys, you actually need to learn to use a trick called the conservation of string, it is a means of relating accelerations between different pulleys.

Depending on how string is wrapped around a pulley will make its acceleration different compared to other pulleys!

Well work out a generic example involving 2 pulleys, and well plug some numbers in afterwards to show you how to solve these kinds of problems.

Lets say we have the system of two pulleys as shown below. Weights are referred to as weight 1 and weight 2 on pulleys 1 and 2, respectively.

Notice that the string wrapped around pulley 1 is wrapped around the pulley twice, once on each side, whereas pulley 2 is only wrapped on the right.

We first need to make equations, which are force balances around each individual weight. Remember that ma = sum of forces. In general the sum of forces includes the force of tension, and the force of gravity on the weight. We say T represents force of tension, and g is gravity acceleration.

Notice that we use subscripts, masses 1 and 2 can be different, and the accelerations are certainly different.

Weight 1 has two tensions because each side of the rope will count! How can you solve this?

You actually need to relate the two acceleration values somehow, this is what is known as the conservation of string!

The conservation of string means that the length of string is conserved.

Motion In A Two Dimensional Plane

• Select a coordinate system and resolve the initial velocity vector into x and ycomponents.
• Find out acceleration in each direction and solve in each direction according to one rectilinear motion equation.
• if the acceleration is in vertical direction only.Follow the techniques for solving constant-velocity problems to analyze the horizontal motion. Follow the techniques for solving constant-acceleration problems to analyze the vertical motion. The x and y motions share the same time of flight t.
• There might be question about trajortory in the Problem ,find out the motion in x and y direction with respect to time from previous point.And then find the value of t from one equation and then put that value in another equation to find out the equation of trajactory

You May Like: Algebra And Trigonometry 4th Edition Stewart Pdf Free

Speed Velocity And Acceleration Problems For Free

Problem : A stone is thrown vertically upward from a building of $15\,$ high with an initial velocity of $10\,$. What is the stone’s velocity just before hitting the ground?

Solution: In the free-fall problems, the important note is choosing the origin. Usually, the throwing point is the best choice. In this case, the hitting point is below the origin so its vertical displacement is negative.

Applying the time-independent free fall kinematic equation, we have \beginv_f^-v_i^& =2\,\Delta y\\v_f^-^& =2\,\\\Rightarrow v_f& =\pm 20\,\end Since velocity is a vector quantity and just before striking to the ground its direction is vertically downward so the negative value must be chosen i.e. $v_f=-20\,$.

Practice Problem : A bullet is released without initial velocity from a height of $h$ and in the last second of falling, it drops a distance of $35\,$. Determine the height of $h$?

Solution: This is left up to you as a practice problem.

Problem : A bullet is fired with an initial velocity of $15\,$ from the top of a tower of $20\,$ high. What is its velocity at the instant of striking at the ground?

Solution: Let the origin be the firing point. Using the below kinematic equation we have \beginv_f^-v_i^& =2\Delta y\\v_f^-0& =2\\\Rightarrow v_f& =-20\,\end where we chose the minus sign as the direction of velocity is downward.

Problem : A stone is dropped vertically from the top of a building with a height of $h$. What is its speed at the height of $\frac h2$?

More Acceleration Word Problems

Problem #1:

What is the acceleration of a motorcycle moving on a straight path when the speed goes from 0 to 70 miles per hour in 5 seconds ?

The motorcycle goes from 0 to 70 m/h, so the change in speed is

70 – 0 = 70

It took the motorcycle 5 seconds to reach this velocity so the time interval is 5.

Plug these numbers into the formula.

Do you remember how to read the answer?

We read 14 m/h.s as 14 miles per hour-second.

In order words, each second, the speed increases by 14 miles per hour.

Read Also: Fsa Practice Test Algebra 1

Setting Equations For The Pulley

For cart M, the equation would be, T Fr = Ma or, T Mg = Ma

For the cylinder m the FBD and equation remain same as the previous case.mg T = ma .. Adding equation 1 and 2, mg Mg =a or, a = g/ from eqn 2, T = m = m = mg = mg or, T =/

So the acceleration of the cart isg/And the tension in the rope is /

We will add other pulley systems soon and study those as well. If you like this, please share as much as possible.

Challenging And Interesting Acceleration Word Problems

Problem #2:

What is the acceleration of a Honda Accord with a constant velocity of 50 km/h for 20 seconds? Does the car have a constant acceleration?

If the Honda has a constant velocity, this means that the speed is constant and the car is going on a straight path.

Because the speed is constant, the car is not accelerating.

The driver probably put the car on cruise control with a speed of 50 km/h for 20 seconds.

So a = 0.

There is a world of difference between constant acceleration and constant velocity.

Constant acceleration means that the speed is changing. However, it is changing by the same amount.

For example from 10 km/h to 20 km/h, then from 20 km/h to 30 km/h, etc.

The change in speed is always 10 km/h, so this is a constant acceleration.

Constant velocity on the other hand means that the speed is not changing!

Since the speed is not changing at all, the car does not have constant acceleration.

Problem #3:

You can speed up from 0 to 25 feet/s in 25 seconds and your brother can speed up from 0 to 6 feet/s in 6 seconds.

Who is faster?

Each second, your speed can increase by 1 foot/second.

If it is hard to see, you can always use the acceleration formula

You May Like: How To Find Ksp In Chemistry

Case Study 5 Elevator Free Fall

This is a special case. Say due to some reason the rope of the elevator is torn. Naturally, without any other support available, the elevator is now moving downwards.

And its going downwards with an acceleration g due to the effect of gravity.

The elevator is on a FREE FALL with an acceleration equal to the acceleration due to gravity . So again in this case there will be a non zero net force working on the system.

As the elevator is moving downwards, i.e. in the direction of Weight, therefore the net force would be W R downwards. Following the equation obtained from Newtons second law of motion , we can write here:

W R = m g => mg R = mgor, R = m =0

So its evident that the Reaction force is 0 this time and the person inside will feel weightless.

Two Forces On The Cylinder

Gravity or the weight W2 which is equal to mg. . This weight mg works vertically downwards. The tension force T also works on the cylinder through the string tied with it. Its direction is upwards. Here the cylinder is with acceleration. Therefore these two forces are not balanced and there is a net force acting on the cylinder which causes an acceleration of it downwards.

You May Like: Elton John Children Biological

Unit Relationships For M=f/a

Solves for mass: Problem 3

Here we will show that in the equation F=mathe acceleration vector, a, has the same direction as thenet force vector, F.

First, recall that when we multiply a scalar times a vector, the resultis a vector that has the same direction as the original. So if we multiplyscalar 2 times vector P we get a vector as the result whichhas the same direction as vector P. We could give thisresult vector a name, say Q. This is all shown in thefollowing animation:

Now let’s see how all this works out with the F and thea vector in the equation F=ma.Note that the right side of the equation is mass times acceleration. Mass isa scalar, and acceleration is a vector. So the right side of this equationis a scalar times a vector. This multiplication yields a vector that iscalled the force vector, or F, which is on the left side ofthe equation.

As above with P and Q, vector ais in the same direction as vector F. Therefore, theacceleration of an object is in the same direction of the applied net force.Here is another animation showing all of this for the a andF vectors:

And so we have shown that the formula F=macontains the information that an object’s acceleration vector is aimed inthe same direction as its applied net force vector.

6 m/s2 =

6 m/s2 = 6 m/s2

There is really nothing special about our choice offactor changes here. Try changing the mass by a factor of 5 and calculate tosee the acceleration change by a factor of 1/5.

Example 6 Calculating Time: A Car Merges Into Traffic

Suppose a car merges into freeway traffic on a 200-m-long ramp. If its initial velocity is 10.0 m/s and it accelerates at 2.00 m/s2, how long does it take to travel the 200 m up the ramp?

Strategy

Draw a sketch.

Figure 11.

We are asked to solve for the time t. As before, we identify the known quantities in order to choose a convenient physical relationship .

Solution

1. Identify the knowns and what we want to solve for. We know that v0 = 10 m/s a = 2.00 m/s2 and x = 200 m.

2. We need to solve for t. Choose the best equation. x=_+_t+\frac}^ works best because the only unknown in the equation is the variable t for which we need to solve.

3. We will need to rearrange the equation to solve for t. In this case, it will be easier to plug in the knowns first.

\text=\text+\leftt+\frac\left^

4. Simplify the equation. The units of meters cancel because they are in each term. We can get the units of seconds to cancel by taking t = ts, where t is the magnitude of time and s is the unit. Doing so leaves

t t

5. Use the quadratic formula to solve for t.

Rearrange the equation to get 0 on one side of the equation.

tt

This is a quadratic equation of the form

}^+\text+c=0

where the constants are a = 1.00, b = 10.0 and c = -200.

Its solutions are given by the quadratic formula:

This yields two solutions for t, which are

t = 10.0 and -20.0.

In this case, then, the time is t = t in seconds, or

t = 10.0 s and -20.0 s.

t = 10.0 s.

Discussion

You May Like: Abiotic Def