Stock Turnover And Sales
These ratios help businesses measure how quickly they are selling products. They are able to determine where they have excess stock and if they need to change production processes. A stock turnover ratio measures how often a business sells and replaces its goods. Usually, the higher this number is, the higher the ROI. A material to sales ratio measures how a company’s cost of direct materials, like raw materials or wholesale products, compares to its total sales. The higher the material to sales ratio, the higher the ROI and profits will be.
Act Math: Dealing With Ratios
You might see ACT ratios written in fraction form, colon form, or in plain English. Whatever the case may be, you can treat them all the same way. In the case of the fraction form, do not get it confused with a regular fraction! The denominator of a ratio is not necessarily equivalent to the denominator of a ratio.
For example, the ratio 12/8, 12:8, and 12 to 8 are all the same. Like fractions, you should reduce ratios down to simplest terms in this case, it is 3/2. Keep your numbers manageable, especially when you need to look for the lowest common multiple later on in the multi-step ratio section.
On the test, ratios will be clearly spelled out for you. If you are looking at a ratio problem, youll know it because the test makers will make it obvious.
The important part lies in knowing how to manipulate ratios to get to your answer. The two main things you need to know are proportions and multi-step ratios.
How To Calculate Ratios And Proportions In Math
The concept of proportion is probably familiar to you, but you might not be able to write a strict mathematical definition for it. For example, you might recognize that a 10-year-old is smaller than a normal-sized adult in the same “way” that same adult is smaller than a professional basketball player, even though the three sizes are different.
Similarly, you’re probably no stranger to the notion of a ratio. For example, if you are at a sports contest and know that the ratio of opposing fans to friendly fans is high, you might be inclined to be less demonstrative when your favored club scores a goal than you would if this ratio were reversed.
In math and statistics, proportion, percentage and ratio questions abound. Fortunately, a brief explanation of the underlying concepts and a few examples should be enough to make you a proportionally better math student.
Read Also: Houghton Mifflin Harcourt Geometry Workbook Answers
How To Solve Ratio Problems For A Test
To solve the majority ratio problems on the test, we set two equivalent fractions equal. This is an equation of the form, fraction = fraction. An equation of this form is known a proportion. And if youre not familiar with the mathematics of proportion, what you are allowed to do and what youre not allowed to do with proportions I highly recommend you watch the video, Operations with Proportions.
Rates In The Real World
Rate and unit rate are used to solve many real-world problems. Look at the following problem. Tonya works 60 hours every 3 weeks. At that rate, how many hours will she work in 12 weeks? The problem tells you that Tonya works at the rate of 60 hours every 3 weeks. To find the number of hours she will work in 12 weeks, write a ratio equal to 60/3 that has a second term of 12.
60/3 = ?/1260/3 = 240/12
Removing the units makes the calculation easier to see. However, it is important to remember the units when interpreting the new ratio.
Tonya will work 240 hours in 12 weeks.
You could also solve this problem by first finding the unit rate and multiplying it by 12.
60/3 = 20/120 × 12 = 240
When you find equal ratios, it is important to remember that if you multiply or divide one term of a ratio by a number, then you need to multiply or divide the other term by that same number.
Let’s take a look at a problem that involves unit price. A sign in a store says 3 Pens for $2.70. How much would 10 pens cost? To solve the problem, find the unit price of the pens, then multiply by 10.
$2.70 ÷ 3 pens = $0.90 per pen$0.90 × 10 pens = $9.00
Finding the cost of one unit enables you to find the cost of any number of units.
Recommended Reading: Beth Thomas Cohen
What Is A Ratio
Ratios describe how to share out a given amount and are written with numbers separated by colons. The amount of numbers in the ratio tells us how many groups the quantity is being shared between. The size of each number tells us the proportion of the total amount each group gets.
For example, in the ratio 1:3 there are two different numbers: 1 and 3.
Because there are two numbers, we are sharing an amount between two people.
This ratio means that for every 1 part that the person on the left gets, the person on the right gets 3. The person on the right will have three times as much as the person on the left.
The person on the left will get $5 and the person on the right will get $15. Both numbers add to make the total of $20 but $15 is three times larger than $5. This is why the ratio is 1 to 3.
What Is A Proportionality Constant
A proportionality constant represents the constant difference between proportional ratios. If a is proportional to b, then in the expression a = kb, k is the constant of proportionality. Two variables a and b are said to be inversely proportional when their product ab is a constant for all a and b, that is, when a = C/b and b = C/a.
Example: The number of archery fans is proportional to the number of baseball fans in a given coffee shop. At first, there are 6 archery fans and 9 baseball fans. If the number of baseball fans increases to 24, how many archery fans must there be?Solve for k, where a = kb, a = 6 and b = 9:k = 6/9 = 2/3 = 0.667Now, solve the equation a = to get 16 archery fans in the now-more-crowded cafe.
Related Articles
Recommended Reading: Grade 6 Fsa Warm-ups Answer Key
How To Write A Ratio
There are several different ways to express a ratio. One of the most common is to write a ratio using a colon as a this-to-that comparison such as the children-to-adults example above. Because ratios are simple division problems, they can also be written as a fraction. Some people prefer to express ratios using only words, as in the cookies example.
In the context of mathematics, the colon and fraction format are preferred. When comparing more than two quantities, opt for the colon format. For example, if you are preparing a mixture that calls for 1 part oil, 1 part vinegar, and 10 parts water, you could express the ratio of oil to vinegar to water as 1:1:10. Consider the context of the comparison when deciding how best to write your ratio.
How To Use Online Ratio Calculator:
Our calculator is an accurate tool to simplify, and for finding the unknown value in the ratio. You just have to stick to the following points for calculating ratios:
Swipe on!
Inputs:
- First of all, hit the tab to choose on how many ratios you want to do the calculations. It is either be A:B or A:B:C
- Very next, select the method of calculation from the dropdown of this calculator
- Then, enter in the fields according to the selected input parameters
- Once you done, hit the calculate button
Outputs:
- Visual representation of the ratio
Note:
This ratio calculator will not give you the values you dont need it will give you the output according to the input parameters.
Don’t Miss: Geometry Holt Mcdougal Answers
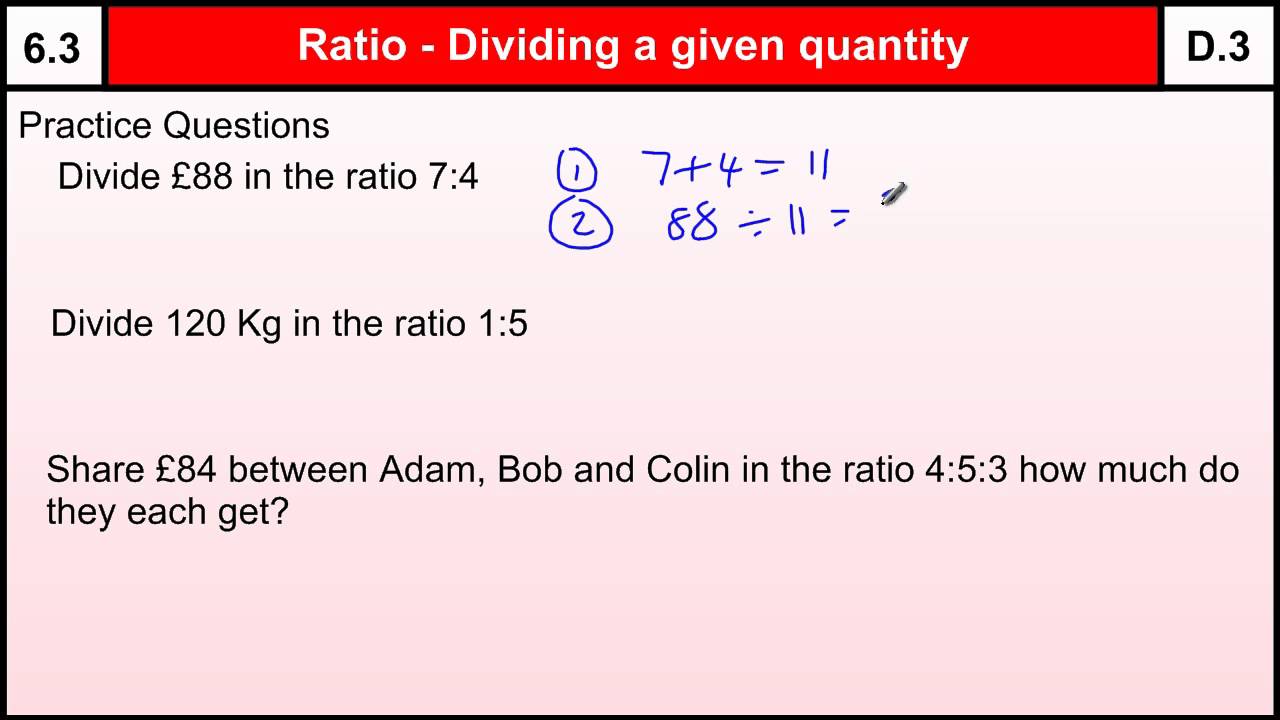
How To Use Ratios To Find An Unknown Number
Question:
The sweet company likes to put uneven numbers of sweets in their bags. They are currently creating a bag of blue and pink sweets in the ratio 4:6.
If you get a bag with 12 blue sweets in it, how many will there be in total?
Explanation:
To work this one out, it is necessary to divide the number of blue sweets by the ratio, to find the common denominator.
12 divided by 4 = 3
We can then multiply the consequent number by 3 to find the unknown quantity:
6 x 3 = 18
Therefore, if there are 12 blue sweets, there will be 18 pink ones
To find the total number of sweets, we need to add both sides of the scaled-up ratio 12:18
12 + 18 = 30
Ratios Do Not Give Exact Numbers
In other words, we have no idea simply from the ratio what the absolute size of the group could be. The absolute size of the group could be anything. For some positive integer n, we definitely have 3n boys and 4n girls. So we can definitely say that if were given a 3 to 4 ratio. Here, n is sometimes called the scale factor.
Notice, once again, if we are given a ratio, 3 to 4, we have no idea about the absolute size of either group. Thats a big idea to which we will return. There are many different ways of presenting ratio information. The first, Ill call it p to q form. The ratio of boys to girls is 3 to 4.
Don’t Miss: Holt Geometry Workbook Answer Key
By Step Tutorial On How To Solve Ratios In Mathematics
When we have to compare two or more than two numbers in mathematics then we can use ratios for the same as ratios can compare two or more quantitative numbers or amount or you can compare the portions of numbers of the larger numbers. Ratio is one of the tools of data analysts. This is why many people face difficulty in solving ratios so they are always in search of methods on how to solve ratios. If you are also stuck with solving ratios then this article will help you to understand the concept of ratios and methods on how to solve ratios.
Question : Finding Unknown Quantities From Existing Equivalent Ratios
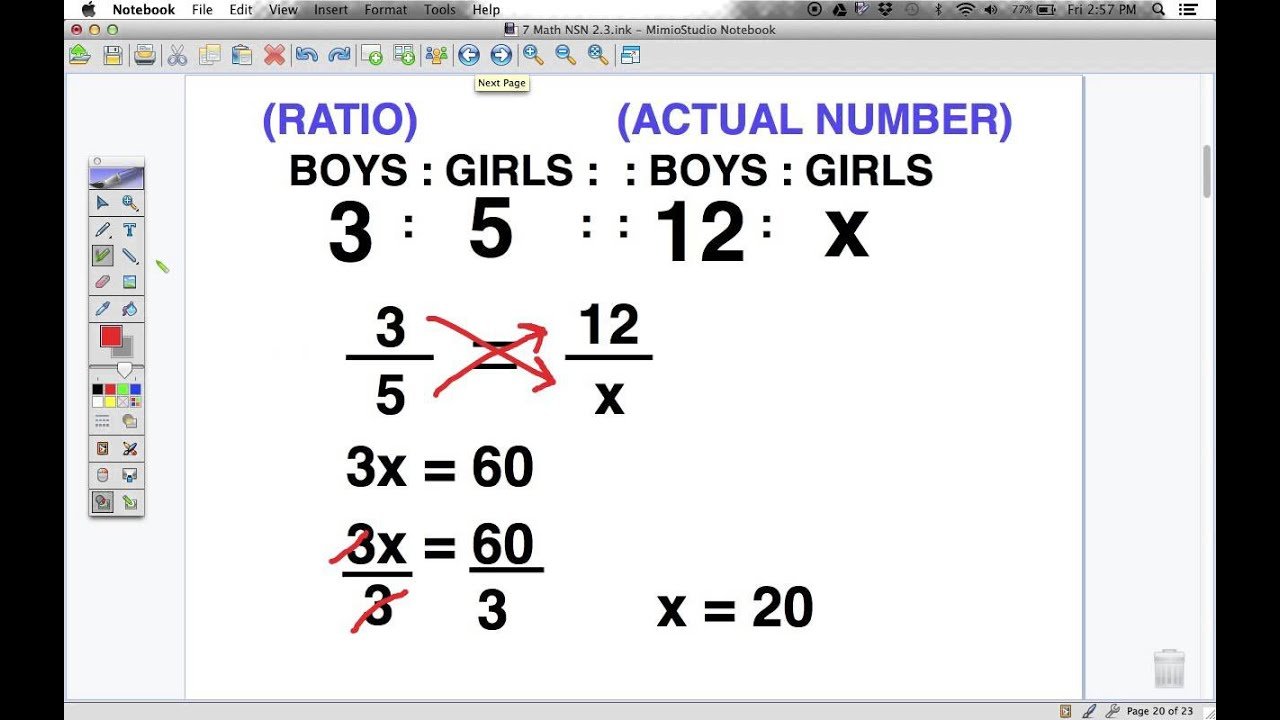
Gabriel and Mandeep are getting married. They have calculated that they will need 35 bottles of wine for their 70 guests. Suddenly they find out that another 20 guests are planning to attend. How much wine do they need in total?
First, you need to work out the ratio of wine to guests they used = 35 wine:70 guests.
Then simplify this so 1 wine:2 guests .
They now have 90 guests attending so you need to multiply 90 by 0.5 = 45 bottles of wine in total.
Watch out for the wording in this sort of question, which can sometimes ask for thetotal required, and sometimes the extra required.
Don’t Miss: What Was The Geography And Climate Of New England
Example: How Tall Is The Tree
Sam tried using a ladder, tape measure, ropes and various other things, but still couldn’t work out how tall the tree was.
But then Sam has a clever idea … similar triangles!
Sam measures a stick and its shadow , and also the shadow of the tree, and this is what he gets:
Now Sam makes a sketch of the triangles, and writes down the “Height to Length” ratio for both triangles:
Height:Shadow Length: h2.9 m = 2.4 m1.3 m
Multiply across the known corners, then divide by the third number:
h = / 1.3 = 5.4 m
Answer: the tree is 5.4 m tall.
And he didn’t even need a ladder!
The “Height” could have been at the bottom, so long as it was on the bottom for BOTH ratios, like this:
Let us try the ratio of “Shadow Length to Height”:
Shadow Length:Height: 2.9 mh = 1.3 m2.4 m
Multiply across the known corners, then divide by the third number:
h = / 1.3 = 5.4 m
It is the same calculation as before.
How To Solve A Ratio Problem
When looking at a ratio problem, the key pieces of information that you need are what the ratio is, whether you have been given the whole amount or a part of the whole and what you are trying to work out.
If you have been given the whole amount you can follow these steps to answer the question:
If you have been been given a part of the whole you can follow these steps:
Don’t Miss: Is Paris Jackson Michael’s Biological Kid
How To Use Ratios In Math
- B.B.A., Finance and Economics, University of Oklahoma
Ratios are a helpful tool for comparing things to each other in mathematics and real life, so it is important to know what they mean and how to use them. These descriptions and examples will not only help you to understand ratios and how they function but will also make calculating them manageable no matter what the application.
Difference Between Ratio And Proportion
Let’s recall ratio and proportion through some points of difference between them.
| Ratio | |
|---|---|
| It is used to compare the size of two quantities with the same unit. | It is used to express the relation of two ratios. |
| Symbols used are – a colon , slash | Symbols used are – the double colon |
| It is referred to as an expression. | It is referred to as an equation. |
| In a problem, the ratio can be identified with the keyword to every | In a problem, the proportion can be identified with the keyword out of |
Tips and Tricks
- The two quantities with the same units only can be compared.
- The two ratios are said to be in proportion only if they are equal.
- To check whether two ratios are equal and form a proportion, we can also use the cross-product method.
Important Notes
- If we multiply and divide each term of a ratio by the same number, it doesnt affect the ratio.
- For any three quantities, if the ratio between the first and the second is equal to the ratio between the second and the third, then these are said to be in a continued proportion
- Similarly, in the case of any four quantities in a continued proportion, the ratio between the first and the second equal to the ratio between the third and the fourth.
- For ratio and proportion, we can write in two ways, either by using equal to sign as a/b = c/d or using a colon, a:b = c:d.
Related Articles on Ratio and Proportion
Don’t Miss: Introduction To Exponential Functions Common Core Algebra 1 Homework
Ratios In Fraction Form
We just spell it out literally like that. Second is fraction form. The ratio of boys to girls is 3/4, weve write it as a fraction. Third is colon form. This is very common on the test. The ratio of boys to girls.
We read this as three to four but its written with a colon. And then, finally, a tricky one. Ill call this idiom form. For every 3 boys, there are 4 girls. So thats an idiomatic way in English to say exactly the same thing. All four of these contain exactly the same information.
Now, of course, of these 4 forms, the most useful by far is fraction form because when we rewrite the ratio in fraction form, then we can do math with it. Notice in all of these, order is important. If we talked about the ratio of girls to boys, all the numbers would have to switch. So we add girls to boys that would be 4 to 3 or four-thirds, something along those lines.
Business Uses For Ratios
Businesses use ratios to calculate their financial success, allocate resources and determine if they are meeting their goals. Ratios allow businesses to compare data over time and understand how different types of information relate to each other. Here are some ways that businesses use ratios:
-
Profitability
-
Product returns
You May Like: The Four Goals Of Psychology Are
Sample Questions And Their Solutions
Understanding how to work out ratios is an important skill and can be particularly useful when applying for jobs where a good understanding of mathematics is required.
It is a good idea to revise skills like this before taking numerical reasoning or other math-based aptitude tests.
Here are the key ratio skills that you need to master:
How To Calculate A Ratio Of A Number In Steps
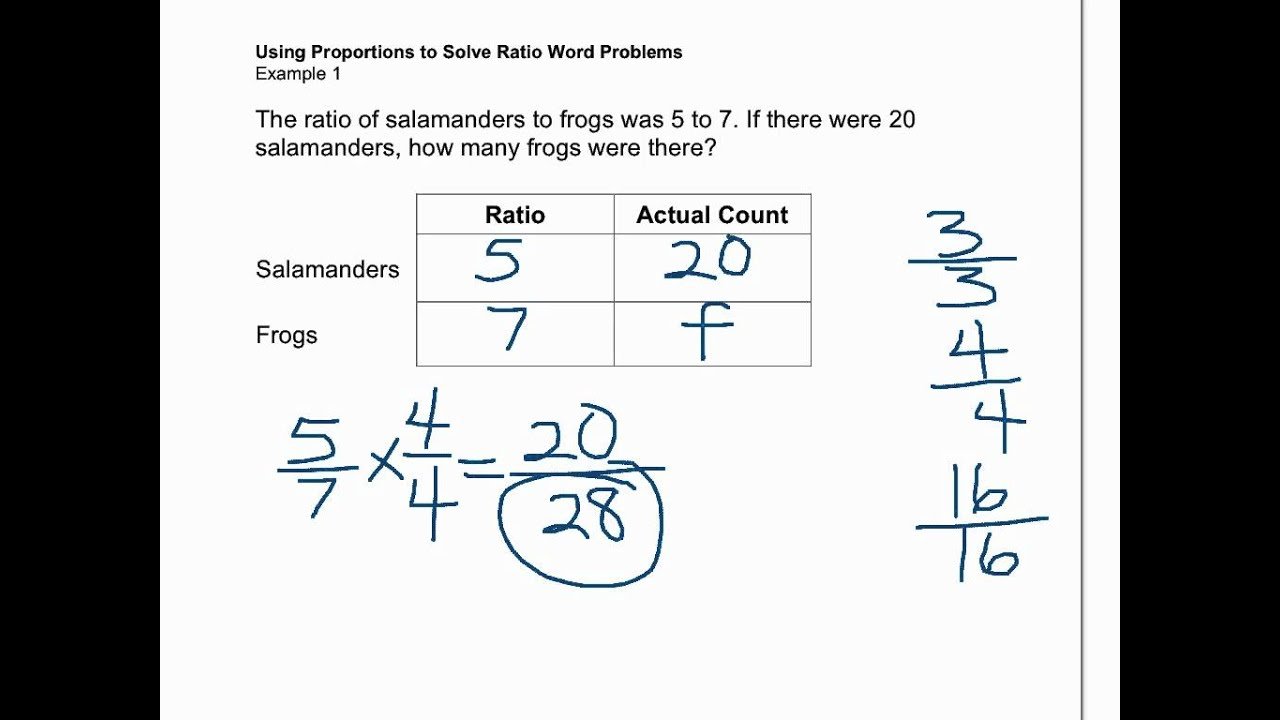
To calculate a ratio of a number, follow these 3 steps:
For example, share $20 in the ratio 1:3.
This means that in this ratio problem, we will be sharing $20 between two people with one person getting three times as much as the other.
We follow the 3 steps above to work out the ratio.
- Step 1: Find the total number of parts
Looking at the ratio 1:3, we have:
1 + 3 = 4
So, we have four parts in total in our ratio.
- Step 2: Divide the amount by the total number of parts
The amount is $20 and the total number of parts is 4.
20 ÷ 4 = 5
Each of the four parts is worth $5.
- Step 3: Multiply each number in the ratio by the value of one part
We have four parts in total and each of these parts are worth $5.
We will multiply both numbers in the ratio 1:3 .
1 x $5 = $5
3 x $5 = $15
The money is shared in the ratio $5:$15.
We have solved our ratio problem to find that one person gets $5 and the other person gets $15.
To check a ratio calculation, add the final values to see if the total is equal to the original amount. If the amounts shared can also be simplified to make the ratio given, the calculation is correct.
To confirm our ratio calculation, we check that $5:$15 simplifies to give us our original ratio.
Don’t Miss: Chapter 7 Test Form 2b Answer Key Geometry