How To Calculate Percentage Error In Physics

How To Calculate Percentage Error: The Formula

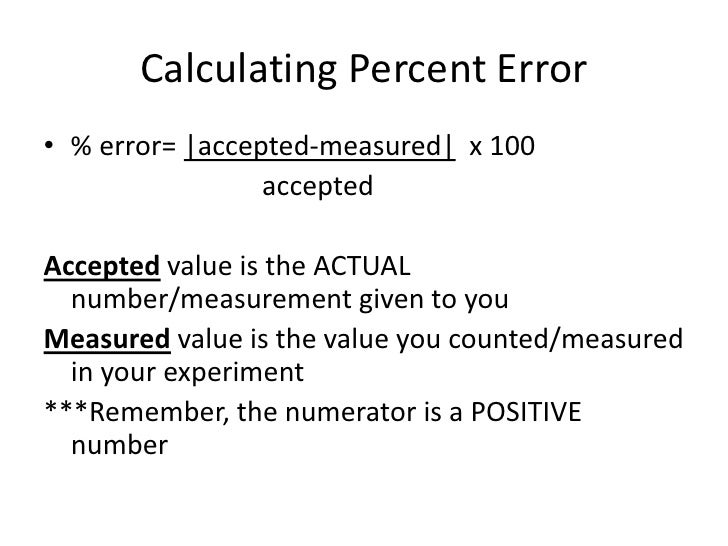
The percent error is the difference between a measured or experimental value and an accepted or known value, divided by the known value, multiplied by 100%.

In many applications, the percentage error is always expressed as a positive value. The absolute value of the error is divided by an accepted value and given as a percentage. Also read how to find percentage increase with our step-by-step guide.

accepted value experimental value \ accepted value x 100%

It is common for chemistry and other sciences to keep a negative value in case one occurs. Whether the mistake is positive or negative is important. For example, you wouldnt expect a positive percentage error when comparing actual versus theoretical yield from a chemical reaction. If a positive value were calculated, this would indicate possible problems with the procedure or reactions that were not taken into account.

If the sign for an error is retained, the calculation is the experimental or measured value minus the known or theoretical value, divided by the theoretical value and multiplied by 100%.

percentage error = / theoretical value x 100%

What Is Percent Error

Percent errors tells you how big your errors are when you measure something in an experiment. Smaller values mean that you are close to the accepted or real value. For example, a 1% error means that you got very close to the accepted value, while 45% means that you were quite a long way off from the true value. Measurement errors are mostly unavoidable: equipment can be imprecise, hands can shake, or your instruments just might not have the capability to measure accurately. Percent error will let you know how badly these unavoidable errors affected your results.

How To Calculate Percent Error

The error calculation formula consists of the difference between the measured and exact value divided by the exact value, further multiplied by 100 to obtain the percentage value. Many times, during the application, the percentage error is positive. Now let us see how to calculate percent error.

The Percent Error formula is given by:

Absolute difference = Exact value – Experimental value

Percent error= *100

Once you know how to find a percent error, you can calculate it easily and incorporate the error percentage in your experiments to get the correct values. This allows you to get your experiments done correctly.

Also Check: Movement Geography Example

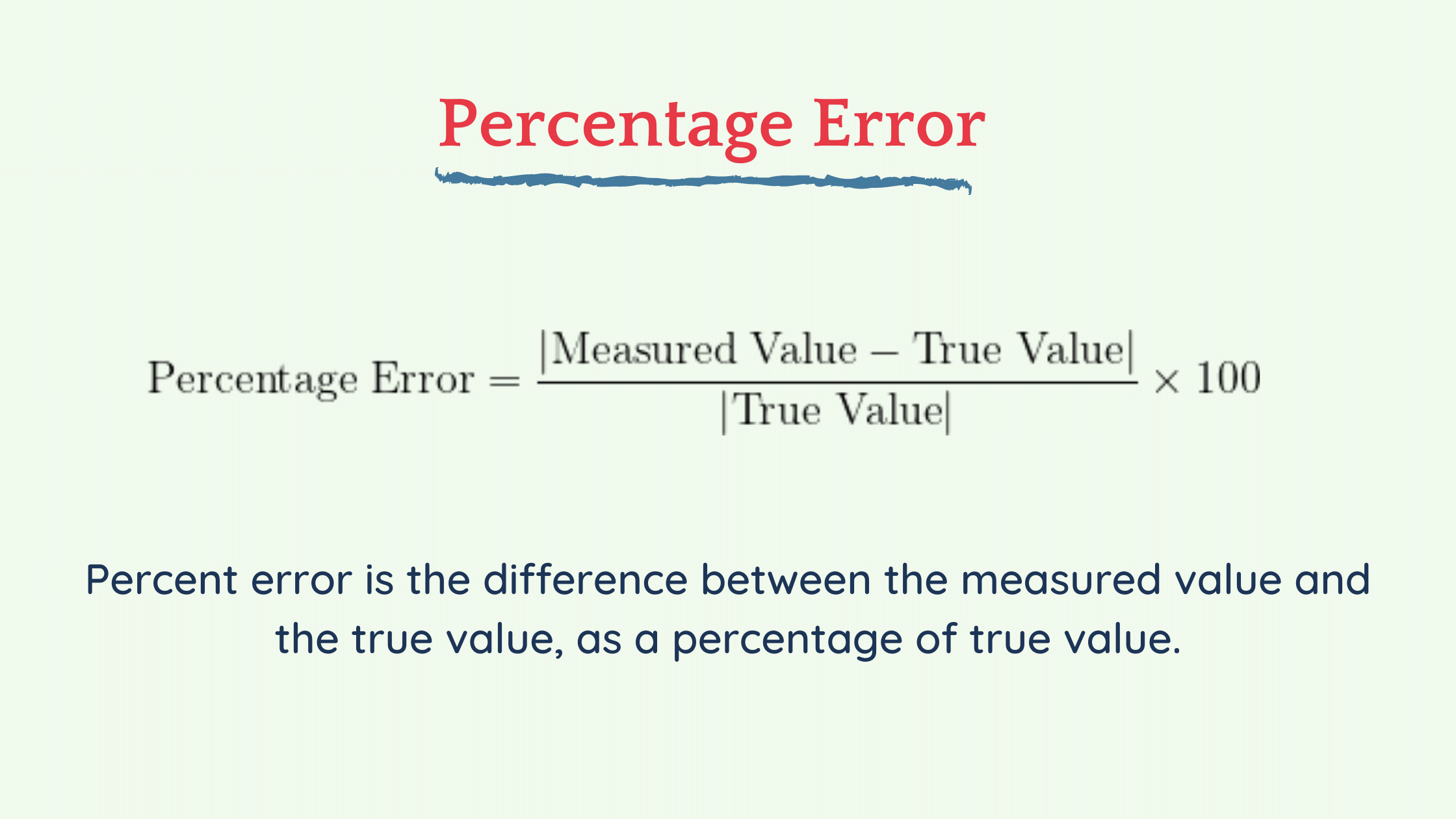
Percentage Error Versus Absolute And Relative Error

The percentage error refers to the absolute error and the relative error . The difference between an experimental and a known value is the absolute error. If you divide this number by the known value, you will get a relative error . The percentage error is the relative error multiplied by 100%. In all cases, give values with the appropriate number of significant digits.

Why Do We Calculate Percentage Error

There are many reasons for calculating percentage errors. Engineers use it to determine the precision of a measuring instrument. Within the finance sector, statisticians and data analysts rely on it to verify if a data set is progressing in the right direction. Outside the workspace, we use percentage errors for lots of everyday tasks. For instance, if youre baking a cake and the recipe calls for four and a half teaspoons of sugar, you could simply round it off. You could indulge your sweet tooth by adding five teaspoons or opt for four spoons. Either option would be about a 10% error marginand still lead to tasty results.

Note: If the measured value is the same as the actual value, then the percentage error is zero.

Read Also: Does Elton John Have Children

What Is The Percent Error

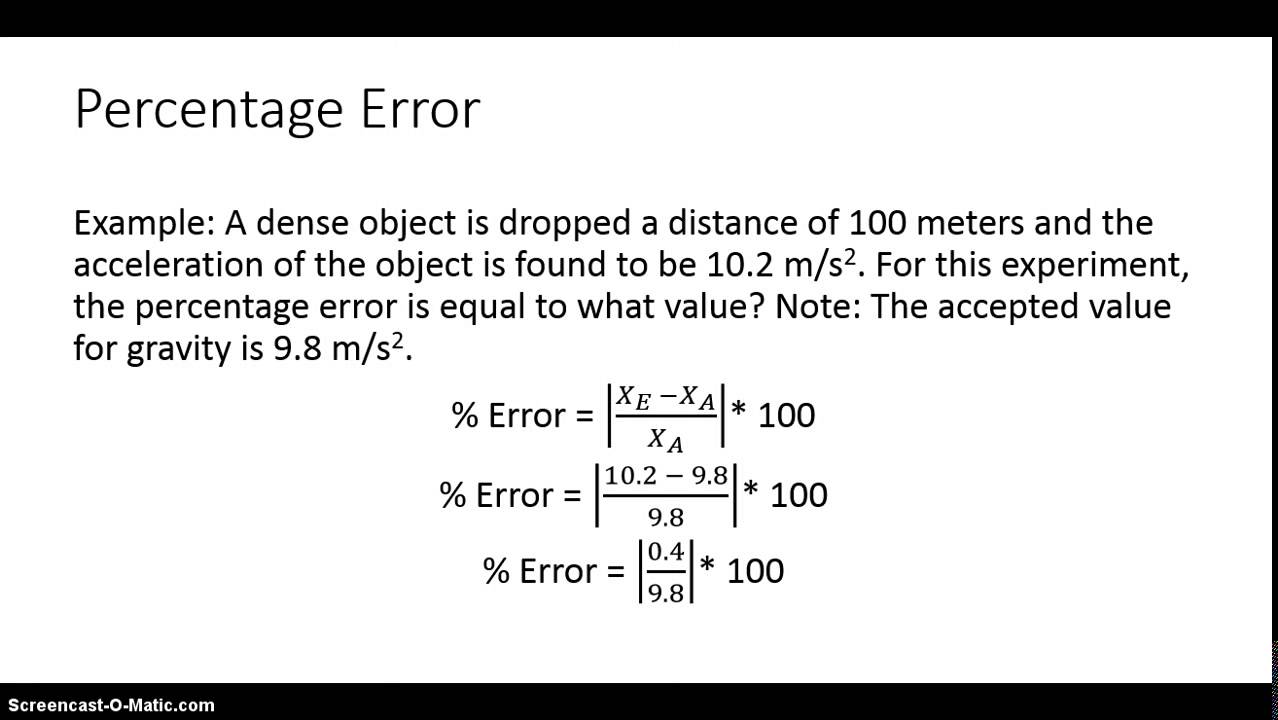
Sometimes, we try to estimate the value of a particular quantity by making repeated measurements. For example, we can try to find out what is the gravitational acceleration by measuring the time of free fall from a specific height.

The measured acceleration will probably deviate a bit from the actual acceleration of 9.80665 m/s². To check what is the relative – or percent – error, you need to know two values: the real value and observed one.

How To Calculate The Percentage Error Of $e^x$ If Percentage Error In Measuring $x$ Is Given

How to calculate the error of $e^x$ if percentage error in measuring $x$ is given?

My attempt: calculate the ln or natural log of given function which we usually do in case of exponential function. Then we differentiate that. Then we get equation for plugging in to get the error. But in this case ln of function is x. By differentiating we get 1. So my question is that right. I mean the error would be 1 always and doesn’t matter for errors made in x. Is that right. Or I’m wrong anywhere ? Suppose we have a error of n% in calculation of x. Then what should be percentage error in calculation of $e^x$?

• $\begingroup$Suppose we have a error of n% in calculation of x. Then what should be percentage error in calculation of $e^x$? Note that the relative or percent error in $e^x$ is more directly related to the absolute error in x in this case.$\endgroup$ user4552

The error propagates via the derivative of the function of the variable in which there is uncertainty. This means that if $x$ has uncertainty $\Delta x$, the uncertainty of a function $f$ is given by$$ \Delta f \approx \frac \Delta x. $$This can be seen by a first-order Taylor expansion. For the case of $f = e^x$, we have $\Delta = e^x \Delta x$.

• Nobody recognizeableJun 15 2018 at 8:47
• $\begingroup$This is one approach. There is also the functional approach. I would recommend pointing out this method is not the only one.$\endgroup$ JamalSJun 15 2018 at 13:37

In this case $f=\mathrm^x$ and $f’=\mathrm^x$.

Don’t Miss: Child Of Rage Documentary Beth Thomas

How To Calculate Uncertainty

Quantifying the level of uncertainty in your measurements is a crucial part of science. No measurement can be perfect, and understanding the limitations on the precision in your measurements helps to ensure that you dont draw unwarranted conclusions on the basis of them. The basics of determining uncertainty are quite simple, but combining two uncertain numbers gets more complicated. The good news is that there are many simple rules you can follow to adjust your uncertainties regardless of what calculations you do with the original numbers.

TL DR

If youre adding or subtracting quantities with uncertainties, you add the absolute uncertainties. If youre multiplying or dividing, you add the relative uncertainties. If youre multiplying by a constant factor, you multiply absolute uncertainties by the same factor, or do nothing to relative uncertainties. If youre taking the power of a number with an uncertainty, you multiply the relative uncertainty by the number in the power.

How To Calculate Percent Error: An Example

To make it easier for you to understand what percent error is, we’ll analyze a simple example. Let’s assume that we want to find out what is the relative error between the measured and true values of the speed of sound.

343 – 329 = 14 m/s

percent error = 14 / 343 *100% = 4.082%

You May Like: 4 Goals Of Psychology Example

Solved Examples For Percent Error

A few solved examples are given here that can help students to understand the type of questions asked in the exam related to the per cent error and they can also understand the method for finding the per cent error.

1. A man installed a stall and thought that daily 100 people would visit the stall but only 80 turned up every day. Calculate the per cent error.

Solutions: Students have to apply the formula:

Estimated value: 100

How To Define Higher Order Components In React

In order to be able to carry out measurements that are as accurate as possible or to be able to assess the accuracy of measurements that have already been carried out, one must know the causes of measurement errors, the size of such errors and their effects on the accuracy of the result. We outline step by step how to calculate percentage error in chemistry, physics or business.

Recommended Reading: What Does Percent Error Tell You

What Percentage Error Is Acceptable

How acceptable the percentage error depends on the application. Sometimes, the measurement might be too difficult to take that you may consider an error of 10% or more acceptable. But in other cases, an error as small as 1% is already too high. In most schools, instructors may accept a percentage error of 5%.

Some say that the percentage error can never be too high as long as you perform the calculation correctly. The utilization of any value with a high percent error in measurement depends on your own judgment. To make sense of this measurement, you have to take other factors into consideration too.

Along with the percent error, the other factors are precision and accuracy. Statisticians and scientists both say that theres no upper limit when it comes to percent error. Theres only the individuals judgment on whether or not he can make use of the data he acquired. But this doesnt apply to both precision and accuracy.

In measurement designs, both precision and accuracy are inherent. They are what they are and you can only improve them when you improve the device or equipment. Several measurements may help improve the statistic accuracy of a measurement. However, these measurements cannot change the measurement error which is inherent.

Percent Error Example Calculation

In a lab, you are given a block of aluminum. You measure the dimensions of the block and its displacement in a container of a known volume of water. You calculate the density of the block of aluminum to be 2.68 g/cm3. You look up the density of a block of aluminum at room temperature and find it to be 2.70 g/cm3. Calculate the percent error of your measurement.

Read Also: Paris Jackson Father

Can Your Percent Error Be Over 100

The short answer to this is yes, it can be. A value of a given quantity x you acquired from your experiment is the approximation of an average x of a specific number of n measurements. The relativity of this value is what you take as the standard error.

The percent error can become over 100 if the fraction on the right is more than 1 and this is a possibility. Generally, this occurs when you take the measurement of a quantity thats small on average but has a distribution thats wide and has a small number of measurements.

In other words, a percent error of more than 100 is entirely possible. Another way you can acquire this value when your observed value is twice as large as the true value. When you perform experiments, theres always the possibility of getting a value which is either lesser or greater than the true value because of experimental or human errors.

Percent Error Solved Question With Example

Here we have given some solved examples of percent error, the students must practice the questions from percent error instead of mugging up the concept. To ease students, we have given the example, the students can refer to these examples. Many students struggled in percent error because they just try to mug up the concepts and ignore the question-solving part, application is more important than mugging concept. Refer to the questions and practice the similar patterns of questions to consolidate the percent error topic.

Q. I estimated that 60 people would attend the show. However, just 70 people attended the performance on the day of the event. Find out how much of my calculation was off by a percentage.

Solution: Well start by calculating the absolute difference of values . which is

Absolute Difference = Accepted Value Experimental Value

Don’t Miss: Find The Length Indicated

How To Calculate Percent

The following steps need to be taken in order to calculate per cent error in any experiment or observation:

You get the error value by subtracting one value from another. If you are not keeping the sign, then the order does not matter, but if you are keeping a negative sign, then you get the error value by subtracting the exact value from the measured value.

You then divide this error value by the known or exact value .

This division will give you a decimal number. Multiply this decimal value with 100 to convert it into a percentage value.

Finally, you would add a % notation in front of the calculated value to report your per cent error.

Solved Examples on Percent Error

We have here a few diverse examples on calculating per cent errors to delve into the concept and get more clarity:

1. In a concert, it was estimated by the organizers that 90 people would show up but in fact, 120 people came to the concert. Calculate the per cent error in the guess value of organizers.

The formula for percent error =

\ 100

Putting the above values we get % error = \ = 25%

% error = \ 100 = 26.62%

S For Calculating Percentage Error

The following are the steps you can follow for calculating percentage error when you have information about the measured value and true/accepted value of a physical or chemical quantity.

Also Check: 4 Main Areas Of Biological Contamination

What Does A Percent Error Tell You

Percent error tells us how much extent few unavoidable errors affect our experimental results. It is measured by taking the difference between the actual value and the observed value. Small percent errors indicate that you are close to the accepted or real value.

Percent errors tell how big your errors are when you measure something in an experiment.

What Is Meant By Percent Error

Whenever an experiment is done, we get results that will match with the actual value or sometimes vary with the actual value. Error is the difference between the estimated value and the actual value. Measurement errors arise because of unavoidable faults in the measuring instrument and limitations of the human eye. Errors come in all sizes, and sometimes we need to decide if the error in our measurement is so big that it makes the measurement useless. The smaller the error indicates that we are close to the actual value. Therefore, scientists have devised a method to calculate the extent of error in estimation.

Also Check: Cyte Suffix Meaning

Examples Of Percent Error Formula

Lets take an example to understand the calculation of Percent Error in a better manner.

Example #1

The density of the aluminum block is 2.68 g/cm cube, while the density of the same aluminum block at room temperature is to be 2.70 g/cm cube. Next, calculate the Percent Error of the measurement.

Solution:

Percent Error is calculated using the formula given below

Percent Error = || / |Theoretical Value| * 100

• Percent Error = ||/ |2.68| * 100
• Percent Error = 0.75%

Example #2

The user needs to find the volume and mass of a paper cube in the experimental lab. When the user is calculating the value of the measurements, it gets the value as 8.78 g/cm cube. Whereas the accepted density of the cube is to be 8.96 g/cm cube. Calculate the percent error.

Solution:

Percent Error is calculated using the formula given below

Percent Error = || / |Theoretical Value| * 100

• Percent Error = || / |8.78| * 100
• Percent Error = 2.05%

Example #3

A scale measures wrongly a value of 10 cm due to some marginal error in the computation. The user needs to calculate the percent error of the measurement when the actual value of the scale is to be 12 cm.

Solution:

Percent Error is calculated using the formula given below

Percent Error = || / |Theoretical Value| * 100

• Percent Error = || / |10| * 100
• Percent Error = 20%

How To Calculate Percentage Error: Step By Step Guide

• Subtract one value from another. The order doesnt matter if you drop the sign (take the absolute value. Subtract the theoretical value from the experimental value if you keep negative signs. This value is your error.
• Divide the error by the exact or ideal value . This results in a decimal number.
• Convert the decimal number to a percentage by multiplying it by 100.
• Add a percent or percent symbol to report your percentage error value.

Sample calculation for percentage error

You can get an aluminum block in a laboratory . You measure the dimensions of the block and its displacement in a container of known volume of water. You calculate the density of the aluminum block to be 2.68 g / cm 3 . To determine the density of an aluminum block at room temperature and find that they 2.70 g / cm 3 is . Calculate the percentage error of your measurement.

• Subtract one value from the other:
• 2.68 2.70 = -0.02
• Depending on what you need, you can discard any negative sign : 0.02
• This is the error.
• Divide the error by the true value: 0.02 / 2.70 = 0.0074074
• Multiply this value by 100% to get the percentage error:
• 0.0074074 x 100% = 0.74% .

Significant numbers are important in science. Reporting an answer with too many or too few answers can be considered incorrect even if you have properly set up the problem.

Read Also: Geometry Chapter 7 Quiz 1 Answer Key