Videos To Help Introduce Regrouping
Secondly, using technology to reinforce this concept is effective and fun for the students. These BrainPop Jr. videos break down the steps of regrouping in several ways. In turn, this ensures that each student connects with the regrouping process. Students may take notes while watching the videos. Upon completion of the videos, students can practice with example problems to further their understanding.
What Is Math Regrouping
Regrouping in math has had several names over the years, including carrying and borrowing. The concept of regrouping involves rearranging, or renaming, groups in place value. The position of the numeral is the place value, and it tells how many groups of one, 10, 100 and so on are found in the number. For example, in 8,364, there are eight groups of 1,000, three groups of 100, six groups of 10 and four groups of one.
Using Regrouping In Addition
When the sum of a place value column is greater than nine, sets that match the next column must be regrouped into the next place. For example, if the ones place totals 13, three is recorded in the ones place and 10 is renamed as one in the tens place. If the tens column totals 38, eight is recorded in the tens place and three is regrouped to the hundreds place. When you add 734 + 69, the ones column totals 13. Regroup 10 of the 13 into the tens column and write the remaining three in the ones column. Add the 1 you carried over to the 3, place the 6 in the tens column and repeat the process, for a final sum of 803.
Read Also: 100 Day Countdown To The 5th Grade Math Fsa 1-50 Answer Key
Mini Math Teaching Course
This is a little “virtual” 2-week course, where you will receive emails on important topics on teaching math, including:
– How to help a student who is behind- Troubles with word problems- Why fractions are so difficult- The value of mistakes- Should you use timed tests- And more!
You will also receive:
GIFT400Note
Regrouping With The Expanded Notation

The next regrouping strategy is expanded notation. Your students have learned about expanded notation in previous lessons. Now is a great time to revisit that skill and practice writing numbers in expanded form. After reviewing, the students are ready to learn how to apply expanded notation to addition and subtraction. This skill helps students quickly combine the tens and ones places to add or subtract. If they are subtracting and regrouping is needed, this expanded form makes it easier for students to understand. The concept of borrowing from the tens place is visualized with this model.
Don’t Miss: The Branch Of Chemistry That Involves The Study Of Substances
How To Solve An Addition Problem With Regrouping
- Category:
- Addition and Subtraction
Do you know how to solve an addition problem with regrouping? Do you know all the steps and why theyre important? Sometimes you learn how to solve math problems, but you dont understand why you solve them in a particular way.
This is why we are going to review how to solve an addition problem with regrouping today, and well explain why we solve them the way that we do with visuals.
Lets do two examples using some math cubes to visualize the problem. Were going to solve the following addition problems:
How To Do Regrouping In Subtraction
Regrouping in subtraction is taking value from one number and giving it to another number. Just as youcarry digits to another number in long addition, youborrow digits from another number in long subtraction.
Also Check: Algebra 2 Chapter 7 Test Answers
How To Regroup Ones Into Tens
In the last lesson, we learned how to add two-digit numbers using column form.
What’s 11 + 18?
Start by writing the addition in column form, like this:
Now, add the Ones digits.
Then, add the Tens place digits:
So, 11 + 18 = 29.
Can you do that in your head? Try it.
Now something cool happens when we add even bigger numbers. Let’s take a look.
Solve 13 + 18
Start by writing the equation in column form:
What’s 8 + 3? 11. But you can’t write two digits in the Ones place.
Each column can only have one digit!
That’s when you regroup.
When one column adds up to more than 10, you regroup 10 Ones into 1 Tens.
This is also called “carrying the 1 over”:
After we carry the 1, we add all the Tens place digits, including the carry.
So, 13 + 18 = 31
Congratulations, you learned how to regroup, or “carry the 1“.
Now, try the practice to become a regrouping master. You’ll use this skill a lot.
Believe in yourself! You can learn anything.
What Does Regroup Mean
In math, regrouping can be defined as the process of making groups of tens when carrying out operations like addition and subtraction with two-digit numbers or larger. To regroup means to rearrange groups in place value to carry out an operation. We use regrouping in subtraction, when digits in the minuend are smaller than the digits in the same place in the subtrahend.
Heres how we regroup hundred and tens to subtract 182 from 427.
We use regrouping in addition when the sum of two digits in the place value column is greater than nine.
Heres how we regroup ones and tens to add 248 and 75.
| Fun FactsRegrouping is referred to as the carrying forward in addition and borrowing in subtraction problems. |
Don’t Miss: Geography Vs Topography
Using The Standard Algorithm For Regrouping
The next regrouping strategy is using the standard algorithm. Anchor charts are an effective, kid-friendly way to introduce the steps of regrouping. They show the students when regrouping is needed. Hang these anchor charts around the classroom for student reference. It is a good idea for the students to solve example problems using the steps shown on the anchor chart.
One of my favorite regrouping strategies is the Sticky Note strategy. This simple, yet effective strategy will help students understand when they need to regroup during addition problems. In addition, it gives them more practice with place value. First, they will add the ones column and write that number on the sticky note. If it is 10 or greater, they will cut the sticky note, leaving the ones half under the ones column and place the tens half above the tens column. Finally, they will add up all of the numbers in the tens column and arrive at their answer.
After the students have become more comfortable with the standard algorithm, it is important to give them problems presented both horizontally and vertically. This ensures that they understand how to apply the standard algorithm regrouping strategy to both problem formats. T-charts are helpful for students too. These charts allow the students to split up the different place values and differentiate the tens and ones. T-charts are especially helpful when solving horizontal problems.
To Regroup Or Not To Regroup
Sometimes when we subtract one number from another number we “regroup,” and sometimes we don’t. For example, if we subtract 38 from 375, we can “regroup” by converting a ten to 10 ones:
Find a 3-digit number to subtract from 375 so that:
In each case, explain how you chose your numbers and complete the problem.
Read Also: Who Is Paris Jackson’s Mom
Addition With Regrouping Strategies
Some people teach regrouping without teaching the underlying concept, but this ultimately hinders the childs learning. Start by using manipulatives like linking cubes or place value disks to make groups of tens with leftover ones.
The next step is using a place value chart . Represent a two-digit number with place value disks and put the disks in the correct column of the place value chart.
For example, to represent the number 28, put 8 ones disks in the ones column, and two 10s disks in the tens column.
Then add a second number .
For example, add 5 ones to your ones column. You are now representing the problem 28+5.
Starting with the one column, regroup the disks to make a ten and any remaining ones. Move the new ten disk to the tens column. Count the resulting number.
For example, gather 10 ones from the ones column and exchange them for a 10 disk. Move this disk to the tens column. Now you have 3 tens disks and 3 ones disks. That is the answer: 33.
When To Teach Addition With Regrouping

Before you even consider teaching addition with regrouping, the child needs to have a firm understanding of place value.
When we write numbers, each digit represents a value. The number 319 has three digits, but the 3 is in the hundreds place, which means its value is 300.
Another foundational concept is knowing the addition math facts. If a child cannot quickly recall 5+4=9 or 9+6=15, he is not ready for addition with regrouping.
Yes, children can reason their way through basic addition with strategies such as counting on, but addition with regrouping is a big leap for most students.
Let him have his math facts firmly under his belt before you ask him to tackle this concept.
Recommended Reading: Eoc Fsa Practice Test Algebra 1 No Calculator Portion
How To Teach Regrouping In 2nd Grade
This blog post is full of tips for teaching your 2nd grade students about regrouping strategies. These strategies will help students fluently add and subtract using place value and properties of operations. In 2nd grade, students will be introduced to base-ten blocks, expanded notation, and the standard algorithm as strategies for regrouping.
Regrouping One Hundred As 10 Tens In Subtraction
In this lesson, we deal with 3-digit subtraction, and there is only ONE regrouping: a hundred is regrouped with the tens.
We first look at a visual model to understand how regrouping works, and then go on to the regular subtraction algorithm .
The second part of the lesson deals with some word problems and puzzles. 🙂
If you cannot see the video above, for an alternative video player.
Recommended Reading: Why Was The Pail Pale Answers
Teaching Addition With Regrouping
Addition with regrouping is a strategy that allows us to add large numbers using only our basic addition facts. Its a very useful tool! Start with a firm foundation in math facts and place value. Then ease your way into the concept with hands-on manipulatives. Follow it up with a fun review, and youve got it!
You May Also Like:
What Is Addition With Regrouping
When you first learn to add, you work with single-digit numbers, like 2+2= 4. Then you kick it up a notch by adding numbers whose sums are larger than 10, like 5+7=12. Even at this second stage, you can use regrouping. Regrouping involves making groups of 10s to solve an addition problem.
Lets look at the simple math problem of 5+7. The number 7 can be regrouped as 5+2. So you can think of the problem 5+7 as 5+5+2 instead. Quickly you see that 5+5=10. So what is left? 10+2. That is easy to add: 12.
I know what youre thinking, Thats awfully labor-intensive for a simple math problem like 5+7. But these small numbers are just the beginning. When you add multi-digit numbers, you can try to count on in your head , or you can use regrouping to make it simpler to solve.
You stack the numbers one on top of the other, line them up by place value, and add each column. If one column makes a sum greater than or equal to 10, you regroup the number and place the extra ten in the column to the left.
Also Check: How Do Noise Canceling Headphones Work Physics
Lessons Are Tied To These Standards:
Second Grade CCSS.MATH.CONTENT.2.NBT.B.5
Fluently add and subtract within 100 using strategies based on place value, properties of operations, and/or the relationship between addition and subtraction.
Second Grade CCSS.MATH.CONTENT.2.NBT.B.7
Add and subtract within 1000 . . . Understand that in adding or subtracting three-digit numbers, one adds or subtracts hundreds and hundreds, tens and tens, ones and ones and sometimes it is necessary to compose or decompose tens or hundreds.
Third Grade CCSS.MATH.CONTENT.3.NBT.A.2
Fluently add and subtract within 1000 using strategies and algorithms based on place value, properties of operations, and/or the relationship between addition and subtraction.
Fourth Grade CCSS.MATH.CONTENT.4.NBT.B.4
Fluently add and subtract multi-digit whole numbers using the standard algorithm
Second Grade CCSS.MATH.CONTENT.2.OA.A.1
Use addition and subtraction within 100 to solve one- and two-step word problems involving situations of adding to, taking from, putting together, taking apart, and comparing, with unknowns in all positions, e.g., by using drawings and equations with a symbol for the unknown number to represent the problem.
How To Add Without Regrouping
- Place the addends one on top of the other so that the place values fall in the same columns.
- Add each column together separately, starting with the 1s place.
- The sums go below each column, underneath the line.
Lets take a look at an example adding 83 + 14.
We start by writing the number 83 and below it, the number 14, making sure to line up the 1s columns, or in other words, the 3 and 4.
We then add 3 + 4 = 7 and write it underneath the 1s place column. Then, we add 8 + 1 = 9 and we write it under the 10s place column.
And we get the answer 97.
Recommended Reading: Algebra And Trigonometry 4th Edition Stewart Pdf Free
Regrouping In Addition And Subtraction
Regrouping is needed in both addition and subtraction. It is important to teach regrouping because it is necessary for understanding written methods of addition and subtraction. It is particularly the case for adding or subtracting numbers that contain more than 1 digit.
When performing addition, regrouping is often called carrying. Carrying is regrouping up through the place value columns, moving from units to tens to hundreds.
When performing addition, regrouping is used when the addition produces a digit larger than 9 in a place value column.
Here is an example of carrying / regrouping in addition.
When adding 38 + 16, we start by adding the ones column.
8 + 6 = 14, which has two digits.
We write down the 4 in the units but carry the 1 over to the tens column using regrouping.
We add this carried ten as part of the tens column addition.
3 + 1 + 1 = 5
And so, 38 + 16 = 54.
In subtraction, regrouping is called borrowing. Borrowing is regrouping down through the place value columns, moving from hundreds to tens to units.
When performing subtraction, borrowing is used when the digit being subtracted is larger than the digit being subtracted from.
Here is an example of borrowing / regrouping in subtraction.
We have 36 18. The 8 is larger than the 6 and so we need to use regrouping.
1 of the tens is worth 10 ones. We can borrow a ten from the 3 and it becomes a 2. We can now write this ten in the ones column. Adding 10 to 6, we get 16.
16 8 = 8
Using Regrouping In Subtraction
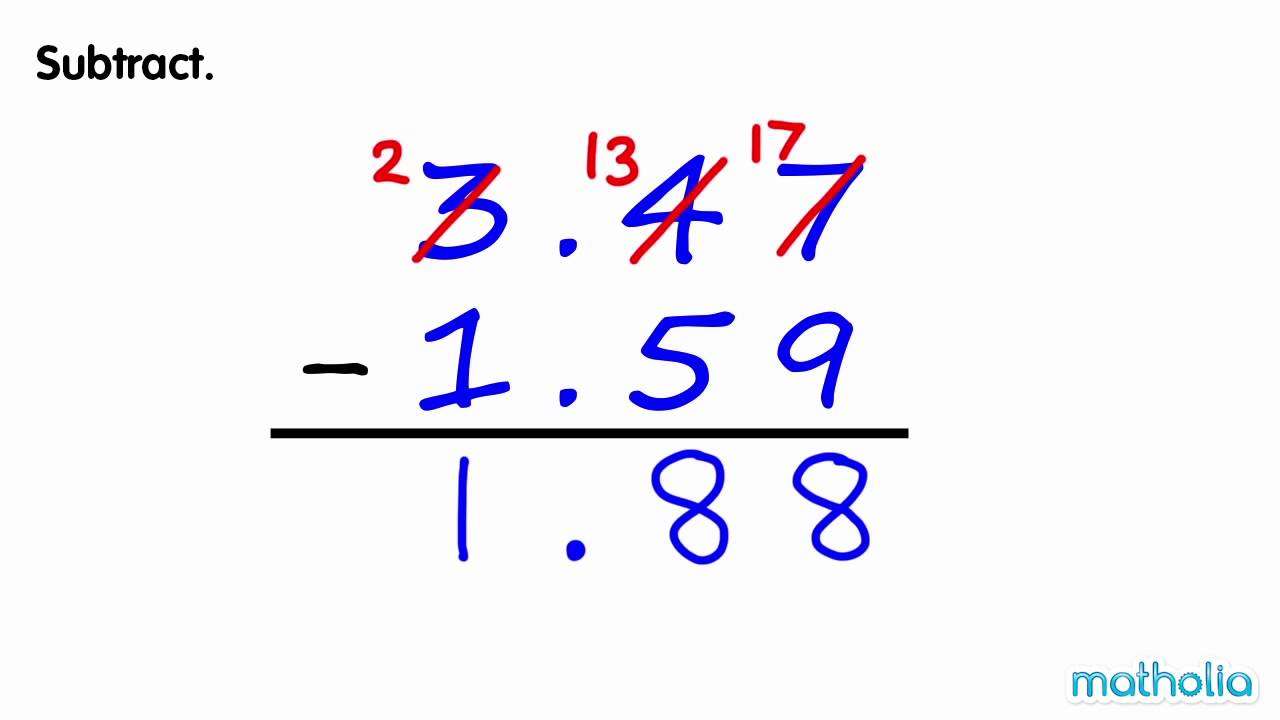
Use regrouping in subtraction when a place-value digit in the minuend, or number from which you are subtracting, is less than the digit in that same place in the subtrahend, or number being subtracted. If the equation is 41-17, for example, you will need to regroup to subtract the ones column. Rewrite the numbers as – , and then subtract 10-7 for the ones column to yield an answer of 24.
Related Articles
Recommended Reading: Shormann Math Placement Test
Students Will Be Able To:
Subtraction with Regrouping
- Two-digit subtraction, regroup from tens to ones
- Two-digit subtraction, rewrite vertically to solve one-step word problems
- Three-digit subtraction, regroup from tens to ones
- Three-digit subtraction, regroup from hundreds to tens
- Three-digit subtraction, regroup from both hundreds and tens
- Three-digit subtraction, regroup with a zero in the tens place of the minuend
- Three-digit subtraction, rewrite vertically to solve one-step word problems
- Four-digit subtraction, regroup from thousands to hundreds
- Four-digit subtraction, regroup with a zero in either the tens or hundreds place
- Four-digit subtraction, regroup with zeros in both the tens and hundreds place
- Four-digit subtraction, from 1000 and 1100
- Four-digit subtraction, rewrite vertically to solve one-step word problems
Addition & Subtraction Word Problems
S For Long Subtraction With Regrouping
Long subtraction lets you find the difference between two numbers.
Don’t Miss: How To Find Halflife