How Do You Find Acceleration In Physics

Displacement And Position From Velocity

To get our first two equations, we start with the definition of average velocity:

Substituting the simplified notation for Î

Solving for x gives us

where the average velocity is

reflects the fact that when acceleration is constant, v v â is just the simple average of the initial and final velocities. Figure 3.18 illustrates this concept graphically. In part of the figure, acceleration is constant, with velocity increasing at a constant rate. The average velocity during the 1-h interval from 40 km/h to 80 km/h is 60 km/h:

In part , acceleration is not constant. During the 1-h interval, velocity is closer to 80 km/h than 40 km/h. Thus, the average velocity is greater than in part .

Acceleration As A Vector

Acceleration is a vector in the same direction as the change in velocity, v. Since velocity is a vector, it can change either in magnitude or in direction. Acceleration is therefore a change in either speed or direction, or both.

Keep in mind that although acceleration is in the direction of the change in velocity, it is not always in the direction of motion. When an object slows down, its acceleration is opposite to the direction of its motion. This is known as .

Figure 2. A subway train in Sao Paulo, Brazil, decelerates as it comes into a station. It is accelerating in a direction opposite to its direction of motion.

Solved Examples On Acccerelation Formula

Question- A woman is traveling by her sports car at a constant velocity v = 5.00 m/s. When she steps on the gas, it makes the car to accelerate forward. Further, past 10.0 seconds, she stops the acceleration and continues a constant velocity v = 25.0 m/s. Calculate the acceleration of the car.

Answer- In the forward direction, initial velocity is \ = 5.00 m/s. Further, the final velocity in the forward direction is \ = 25.0 m/s. The time in which the change took place is 10.0 s. Therefore, the acceleration is in the forward direction, with a value:

a = \

a = \

a = \

a = 2.00 m/s2

Therefore, we see that the acceleration of the car is 2.00 m/s2 forward.

Question- A man takes a rock and drops it off from a cliff. It falls for 15.0 s before it hits the ground. The acceleration due to gravity g = 9.80 m/s2. Calculate the velocity of the rock the moment before it had hit the ground.

Answer-;The man released the rock from rest, therefore, we get the initial velocity as \ = 0.00 m/s. The time for the change to take place is 15.0 s. The acceleration for this is 9.80 m/s2. Therefore, to find the velocity we will rearrange the equation like:

a = \ + at

\ + at

\ = 0.00 m/s +

\ = 147 m/s

Therefore, as the rock is falling, the direction of the velocity is down.

You May Like: Holt Geometry Lesson 4.5 Practice B Answers

Example 1 Calculating Acceleration: A Racehorse Leaves The Gate

Figure 4.

A racehorse coming out of the gate accelerates from rest to a velocity of 15.0 m/s due west in 1.80 s. What is its average acceleration?

Strategy

First we draw a sketch and assign a coordinate system to the problem. This is a simple problem, but it always helps to visualize it. Notice that we assign east as positive and west as negative. Thus, in this case, we have negative velocity.

Figure 5.

We can solve this problem by identifying v and t from the given information and then calculating the average acceleration directly from the equation;\bar=\frac=\frac_-_}_-_}.

Solution

1. Identify the knowns. v0;= 0, vf;= 15.0 m/s , t;= 1.80 s.

2. Find the change in velocity. Since the horse is going from zero to 15.0 m/s, its change in velocity equals its final velocity: v;=;vf;= 15.0 m/s.

3. Plug in the known values and solve for the unknown \bar.

Discussion

The negative sign for acceleration indicates that acceleration is toward the west. An acceleration of 8.33 m/s2 due west means that the horse increases its velocity by 8.33 m/s due west each second, that is, 8.33 meters per second per second, which we write as 8.33 m/s2. This is truly an average acceleration, because the ride is not smooth. We shall see later that an acceleration of this magnitude would require the rider to hang on with a force nearly equal to his weight.

Calculating Average Acceleration From Two Velocities

Don’t Miss: What Does Kw Mean In Chemistry

Charged Particle In An Electromagnetic Field

One of the fundamental forces of nature, the electromagnetic force, consists of the electric and the magnetic field. On one hand, the electric field always acts on the charged particle, and on the other hand, the magnetic field acts only when this particle is moving.

The magnitude of force F which is exerted on the charged particle in the electric field E can be described with the Coulomb’s law. It states that F = q * E, where q is the charge of the particle. You can see that particles with the higher charge will always be attracted stronger.

Solving For Final Position With Constant Acceleration

We can combine the previous equations to find a third equation that allows us to calculate the final position of an object experiencing constant acceleration. We start with

to each side of this equation and dividing by 2 gives

for constant acceleration, we have

Now we substitute this expression for v into the equation for displacement, x

Recommended Reading: What Math Class Do 11th Graders Take

Example 3 Comparing Distance Traveled With Displacement: A Subway Train

What are the distances traveled for the motions shown in parts and of the subway train in Figure 7?

Strategy

To answer this question, think about the definitions of distance and distance traveled, and how they are related to displacement. Distance between two positions is defined to be the magnitude of displacement, which was found in Example 1. Distance traveled is the total length of the path traveled between the two positions. In the case of the subway train shown in Figure 7, the distance traveled is the same as the distance between the initial and final positions of the train.

Solution

1. The displacement for part was +2.00 km. Therefore, the distance between the initial and final positions was 2.00 km, and the distance traveled was 2.00 km.

2. The displacement for part was 1.5 km. Therefore, the distance between the initial and final positions was 1.50 km, and the distance traveled was 1.50 km.

Discussion

An airplane lands on a runway traveling east. Describe its acceleration.

Solution

If we take east to be positive, then the airplane has negative acceleration, as it is accelerating toward the west. It is also decelerating: its acceleration is opposite in direction to its velocity.

Speed Velocity And Acceleration

Average speed is distance divided by time. Velocity is speed in a given direction. Acceleration is change in velocity divided by time. Movement can be shown in distance-time and velocity-time graphs.

You can calculate the acceleration of an object from its change in velocity and the time taken.

Velocity is not exactly the same as speed. Velocity has a direction as well as a speed. For example, 15 m/s is a speed, but 15 m/s North is a velocity .

Commonly velocities are + or – .

For example, -15 m/s means moving backwards at 15 metres every second.

Don’t Miss: How To Prepare Geography For Upsc Quora

Examples Of Acceleration Gravitational

Gravitational acceleration refers to the force between two object masses. As you now know from Newtons Third Law of Motion, the earth uses the same amount of force on you as you use on it, but as the planet is bigger than you, you dont feel the gravity. How this fits into an acceleration calculator: Standard gravity = 31.17405 ft/s²Average human weight = 137 poundsTo get the gravitational force = 4270.84 pdl

How To Find The Acceleration From The Velocity Difference

First things first – both acceleration and velocity are vectors. From the previous section, we know that the acceleration results from subtracting the final and the initial velocity divided by the time difference.

Imagine a sphere in the Cartesian coordinates system. The initial velocity is v0 = m/s, and the final velocity equals v1 = m/s. The velocity changed in time interval t = 5 s. We can ask two questions: What is the acceleration? and How to calculate the magnitude of acceleration? Let’s find out:

• Evaluate the velocities’difference. For vectors, subtract each of the coordinates separately:

  v1 – v0 = – = = = m/s;

• Divide both components by time difference: = ;

• The result is our acceleration: a = m/s².

So how to find the magnitude of the acceleration? Let’s use the formula with acceleration coordinates:

• Square each of the components: ² = 1.44, ² = 0.16;

• Add these numbers: 1.44 + 0.16 = 1.6;

• Estimate the square root of this value: = 1.265. We will stick with four significant figures; and

• That’s all! The magnitude of the acceleration is 1.265 m/s².

Read Also: What Is Three Dimensional Geometry

How To Calculate Acceleration: Step

Now well breakdown the acceleration formula step-by-step using a real example.

A race car accelerates from 15 m/s to 35 m/s in 3 seconds. What is its average acceleration?

First, write the acceleration equation.

$$a = /$$

Next, define your variables.

$a$ = what we are solving for

$$V = 35 m/s$$

Now, plug your variables into the equation and solve:

$$A =/s}/$$

$$A = / m/s^2$$

$$A = -23.2/1.4 m/s^2$$

$$A = -16.57 m/$$

Formulas And Equations For Acceleration

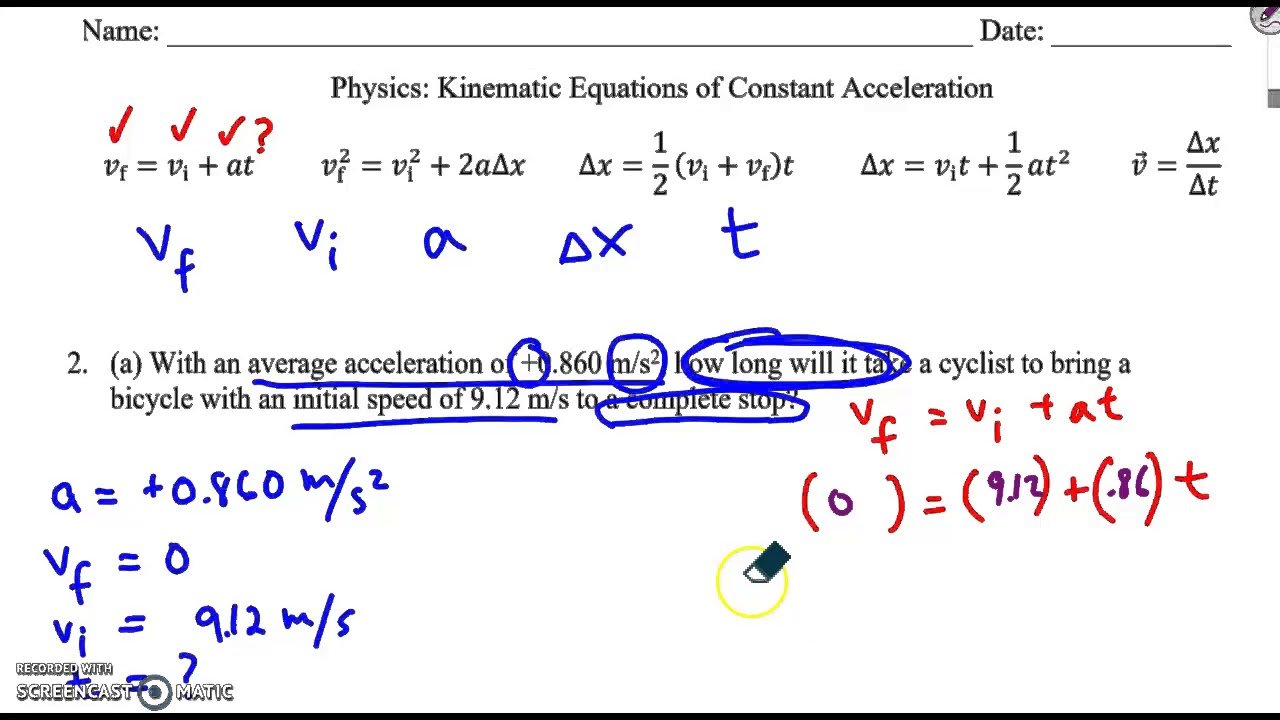
Most people know that Sir Isaac Newton was one of the most influential scientists of the 17th century  and he still is today. He discovered that when two mass objects attract each other, the force depends on the distance between the objects. The weight of the objects also matters. Gravitational force increases based on how large they are. Many people also know Isaac Newton for his laws of motion which appear prominently in the world of physics. 1.a =  / t2.a = 2 *  / t²3.a = F / ma = accelerationv_i = initial velocity v_f = final velocityt = acceleration time d = distance traveled during acceleration F = net force M = mass

Also Check: What Does Abiotic Mean In Biology

What Is The Acceleration Formula

You can use the acceleration equation to calculate acceleration. Here is the most common acceleration formula:

$$a = /$$

where $v$ is the change in velocity and $t$ is the change in time.

You can also write the acceleration equation like this:

$$a = /$$

In this acceleration equation, $v$ is the final velocity while is the $v$ initial velocity. $T$ is the final time and $t$ is the initial time.

Some other things to keep in mind when using the acceleration equation:

• You need to subtract the initial velocity from the final velocity. If you reverse them, you will get the direction of your acceleration wrong.

• If you dont have a starting time, you can use 0.

• If the final velocity is less than the initial velocity, the acceleration will be negative, meaning that the object slowed down.

Now lets breakdown the acceleration equation step-by-step in a real example.

Newton’s Second Law In Action

Rockets traveling through space encompass all three of Newton’s laws of motion.

If the rocket needs to slow down, speed up, or change direction, a force is used to give it a push, typically coming from the engine. The amount of the force and the location where it is providing the push can change either or both the speed and direction.

Now that we know how a massive body in an inertial reference frame behaves when it subjected to an outside force, such as how the engines creating the push maneuver the rocket, what happens to the body that is exerting that force? That situation is described by Newtons Third Law of Motion.;

Additional reporting by Rachel Ross, Live Science contributor.

See also:

Don’t Miss: What Is Biomass In Biology

Tangential And Centripetal Acceleration

aaLocal coordinates

The velocity of a particle moving on a curved path as a function of time can be written as:

v

; , \mathbf &= }}\\&=}\mathbf _ }+v _ }}}\\&=}\mathbf _ }+}}\mathbf _ }\ ,\end}}

where un is the unit normal vector to the particle’s trajectory , and r is its instantaneous radius of curvature based upon the osculating circle at time t. These components are called the tangential acceleration and the normal or radial acceleration .

Geometrical analysis of three-dimensional space curves, which explains tangent, normal and binormal, is described by the FrenetSerret formulas.

• } is the uniform rate of acceleration.

In particular, the motion can be resolved into two orthogonal parts, one of constant velocity and the other according to the above equations. As Galileo showed, the net result is parabolic motion, which describes, e.;g., the trajectory of a projectile in a vacuum near the surface of Earth.

rva

• For a given speed , the magnitude of this geometrically caused acceleration is inversely proportional to the radius r

. } =-\omega ^\mathbf \;.}

. =r\alpha .}

The sign of the tangential component of the acceleration is determined by the sign of the angular acceleration , and the tangent is always directed at right angles to the radius vector.

Finding Velocity And Displacement From Acceleration

• Derive the kinematic equations for constant acceleration using integral calculus.
• Use the integral formulation of the kinematic equations in analyzing motion.
• Find the functional form of velocity versus time given the acceleration function.
• Find the functional form of position versus time given the velocity function.

This section assumes you have enough background in calculus to be familiar with integration. In Instantaneous Velocity and Speed and Average and Instantaneous Acceleration we introduced the kinematic functions of velocity and acceleration using the derivative. By taking the derivative of the position function we found the velocity function, and likewise by taking the derivative of the velocity function we found the acceleration function. Using integral calculus, we can work backward and calculate the velocity function from the acceleration function, and the position function from the velocity function.

Recommended Reading: What Does Algebra 2 Look Like

How Do You Calculate Acceleration For An Object Moveing In A Straight Line

How do you calculate acceleration for an object moveing in a straight line

Answer

The average acceleration over a given time interval is calculated by dividing the change in the object’s velocity by the time that it takes the change to occur.So average acceleration = /time

Virtual Teaching Assistant: John B.

Question Level: Basic

Calculating Acceleration From A Force

Also Check: What Is Work Done In Physics

Summary Of Kinematic Equations

Before we get into the examples, letâs look at some of the equations more closely to see the behavior of acceleration at extreme values. Rearranging Equation 3.12, we have

From this we see that, for a finite time, if the difference between the initial and final velocities is small, the acceleration is small, approaching zero in the limit that the initial and final velocities are equal. On the contrary, in the limit t for a finite difference between the initial and final velocities, acceleration becomes infinite.

Similarly, rearranging Equation 3.14, we can express acceleration in terms of velocities and displacement:

Thus, for a finite difference between the initial and final velocities acceleration becomes infinite in the limit the displacement approaches zero. Acceleration approaches zero in the limit the difference in initial and final velocities approaches zero for a finite displacement.

Examples Of Acceleration Centripetal And Tangential

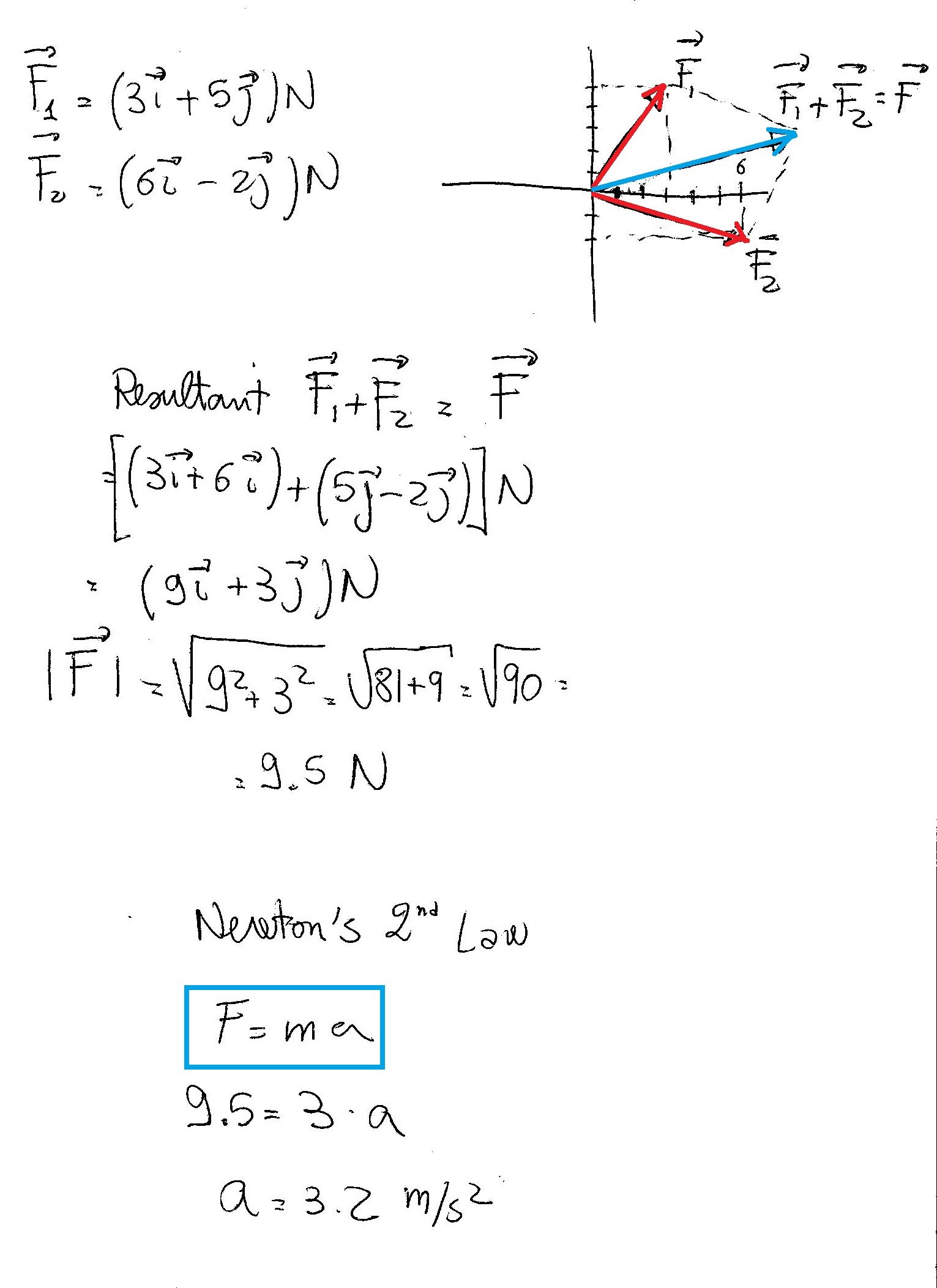
Acceleration is something you can deconstruct so you can understand it better. Two types of acceleration are known as centripetal and tangential. Centripetal acceleration is responsible for changing the velocitys direction. However, it doesnt alter the velocitys value. Tangential acceleration sits perpendicular to the motion trajectory and changes the value of the velocity but not its direction. Therefore, the two have different jobs to do. Imagine a circle with an object moving around the outside of it. The direction of it is the centripetal acceleration. The objects speed remains consistent and constant. When you bring tangential acceleration into the picture, it changes the velocity value but not its direction.

Don’t Miss: What Was The Geography And Climate Of New England