Examples Of Conjugate In A Sentence
conjugateconjugatingconjugate SELFconjugate CNNWashington Postconjugatedkansascity.comconjugatingThe New YorkerconjugatingThe HiveconjugateForbesconjugate BostonGlobe.comconjugate The Salt Lake Tribuneconjugate oregonliveconjugate Forbesconjugate Science | AAASconjugate San Diego Union-Tribuneconjugate Science | AAAS
These example sentences are selected automatically from various online news sources to reflect current usage of the word ‘conjugate.’ Views expressed in the examples do not represent the opinion of Merriam-Webster or its editors. Send us feedback.
Multiply Both Top And Bottom By The Conjugate
There is another special way to move a square root from the bottom of a fraction to the top … we multiply both top and bottom by theconjugate of the denominator.
The conjugate is where we change the sign in the middle of two terms:
| Example Expression |
|---|
It works because when we multiply something by its conjugate we get squares like this:
= a2 b2
Here is how to do it:
Dividing By Square Roots
Just as we can swap between the multiplication of radicals and a radical containing a multiplication, so also we can swap between the division of roots and one root containing a division.
-
Simplify:
I can simplify this by working inside, and then taking the square root:
…or else by splitting the division into two radicals, simplifying, and cancelling:
Either way, my final answer is the same.
-
Simplify:
I can see that the denominator contains a perfect square, but the numerator contains a prime number. So simplification will be easier if I split the radical containing a fraction into a fraction containing radicals:
URL: https://www.purplemath.com/modules/radicals4.htm
Don’t Miss: What Does The Denominator Tell You
Properties Of Complex Conjugates
Below are some properties of complex conjugates given two complex numbers, z and w. Conjugation is distributive for the operations of addition, subtraction, multiplication, and division.
If a complex number only has a real component:
The complex conjugate of the complex conjugate of a complex number is the complex number:
Below are a few other properties.
If And Only If Z Is Real For Any Integer N
, involution
if z is non-zero The latter formula is the method of choice to compute the inverse of a complex number if it is given in rectangular coordinates.
if z is non-zero In general, if and is a holomorphic function whose restriction to the real numbers is real-valued,
is defined, then
Consequently, if is a polynomial with real coefficients, and , then as well. Thus, non-real roots of real polynomials occur in complex conjugate pairs . The map from to is a homeomorphism and antilinear, if one considers as a complex vector space over itself. Even though it appears to be a well-behaved function, it is not holomorphic it reverses orientation whereas holomorphic functions locally preserve orientation. It is bijective and compatible with the arithmetical operations, and hence is a field automorphism. As it keeps the real numbers fixed, it is an element of the Galois group of the field extension . This Galois group has only two elements: and the identity on . Thus the only two field automorphisms of that leave the real numbers fixed are the identity map and complex conjugation.
Use as a variableOnce a complex number or reproduce the parts of the z-variable: is given, its conjugate is sufficient to
You May Like: How To Find Ksp Chemistry
Example: Move The Square Root Of 2 To The Top: 132
We can multiply both top and bottom by 3+2 , which won’t change the value of the fraction:
132×3+23+2 = 3+2322 = 3+27
= a2 b2 which simplifies to 92=7)
Use your calculator to work out the value before and after … is it the same?
So try to remember this little trick, it may help you solve an equation one day!
Real Part: Imaginary Part: Modulus/absolute Value: Argument: So
Thus the pair of variables and also serve up the plane as do x,y and and . Furthermore, the variable is useful in specifying lines in the plane:
is a line through the origin and perpendicular to since the real part of is zero only when the cosine of the angle between and is zero. Similarly, for a fixed complex unit u = exp, the equation:
Also Check: Unit 1 Test Geometry Basics Answers Key
What Is The Conjugate
What is the conjugate?$\sqrt x +2 \sqrt b$I’m not sure how to find the conjugate of any term. Help, please?
You have a number $\sqrt+2\sqrt$ and you’re asking for the conjugate.
Most times this refers to the complex conjugate, which involves replacing the imaginary part of the complex number with its negative.
Your number is a purely real number, so its complex conjugate is itself.
However, you may be wanting to rationalize a denominator of a fraction, in which case perhaps what you’re looking for is the same expression with the second term having opposite sign, also called the binomial conjugate, so you can multiply top and bottom by it to get rid of the square roots in the bottom?
$$\frac + 2 \sqrt} \times \frac – 2 \sqrt} – 2 \sqrt}= \frac + 2 \sqrt}.$$
In this case, the conjugate involves switching the sign of the second term: $\sqrt-2\sqrt$.
Using the conjugate we switch the sign in between the two terms $\sqrt+2\sqrt$. We do this to create a difference of squares. The difference of squares can be seen in this example: $$=a^2-b^2$$ Notice how we don’t have a middle term. This is intentional and the result of using the difference of squares.
$$=\left\cdot\left $$
$$=\dfrac-2\sqrt\right)}+2\sqrt\right)\left}$$
$$=\boxed-2\sqrt}}$$
Get Access To The World’s Best Math Tutors
Loading…
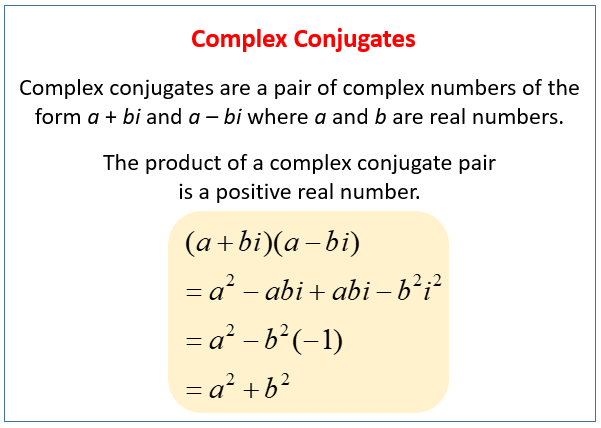
Demonstrating that multiplication of two complex conjugate numbers produces a real result, consider an example, y=7+2i and Ó¯=7â2i. Multiplying these two gives yÓ¯=49+14iâ14iâ4i2=49+4=53. Such a real result from complex conjugate multiplication is important, particularly in considering systems at the atomic and sub-atomic levels. Frequently, mathematical expressions for tiny physical systems include an imaginary component. The discipline in which this is especially important is quantum mechanics, the non-classical physics of the very small.
In quantum mechanics, the characteristics of a physical system consisting of a particle are described by a wave equation. All that is to be learned about the particle in its system can be revealed by these equations. Frequently, wave equations feature an imaginary component. Multiplying the equation by its complex conjugate results in a physically interpretable âprobability density.â The characteristics of the particle may be determined by mathematically manipulating this probability density.
Looking for private one-on-one math tutoring? Speak with our academic advisor to get custom matched with your online math tutor today!
You May Like: 6 Major Branches Of Chemistry
Simplifying Radical Expressions With Conjugates Worksheet
Problem 1 :
Simplifying the above radical expression is nothing but rationalizing the denominator.
So, rationalize the denominator.
Here, the denominator is 2 + 5.
In the given fraction, multiply both numerator and denominator by the conjugate of 2 + 5. That is 2 – 5.
Problem 2 :
Simplifying the above radical expression is nothing but rationalizing the denominator.
So, rationalize the denominator.
Here, the denominator is 6 – 5.
In the given fraction, multiply both numerator and denominator by the conjugate of 6 – 5. That is 6 + 5.
/ = /
/ = /
/ = 2 /
/ = /
/ = / 31
/ = / 31
Problem 3 :
Simplifying the above radical expression is nothing but rationalizing the denominator.
So, rationalize the denominator.
Here, the denominator is 8 – 25.
In the given fraction, multiply both numerator and denominator by the conjugate of 8 – 25. That is 8 + 25.
1 / = 1 / [
1 / = /
1 / = /
1 / = /
1 / = / 44
1 / = 2 / 44
1 / = / 22
Problem 4 :
Simplifying the above radical expression is nothing but rationalizing the denominator.
So, rationalize the denominator.
Here, the denominator is 3.
In the given fraction, multiply both numerator and denominator by 3.
2 / 3 = /
2 / 3 = 23 / 3
Problem 5 :
To add the above two fractions, make the denominators same.
Least common multiple of 2 and 5 is
= 2 5
1/2 + 1/5 = 5/10 + 2/10
1/2 + 1/5 = / 10
To rationalize the denominator on the right side, multiply both numerator and denominator by 10.
1/2 + 1/5 = /
1/2 + 1/5 = / 10
What Is Conjugation The Conjugate Definition
Let’s start with the conjugate definition because it’s so simple.
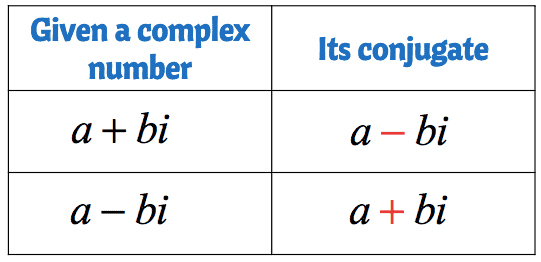
The conjugate of a complex numberz = a + bi is the number a – bi. We denote this operation by putting a horizontal line over the value, like this:
It may seem like the conjugate in math is a simple, useless operation that doesn’t change much. In fact, the conjugate of a number has a deeper meaning that we’ll try to explain in a second.
Do you know how we mark real numbers on a line? With complex numbers, it’s not that easy because we don’t know if i is smaller or larger than 0 . Therefore, we need two axes to describe them, which together form the complex plane.
Above, we see an example of a complex number represented on the complex plane – in this case, it’s z = 2 + i. Just as it is on the image, the horizontal axis marks the real part , and the vertical axis is the imaginary part .
So what is conjugation? It is the symmetric reflection of the point with respect to the real axis. To put it in simpler terms, imagine that you have a mirror along the horizontal line. Where would the reflection of z be? Check the picture below:
Note that:
|z| = |a + bi| = .
Shall we?
Also Check: Eoc Fsa Warm Ups Algebra 1 Answers
How To Conjugate Binomials
The conjugate of binomials can be found out by flipping the sign between two terms.
For example the conjugate of \ is \. In other words, it can be also said as \ is conjugate of \.
The conjugate of \ is \
Look at the table given below of conjugate in math which shows a binomial and its conjugate.
| Binomial | |
| \ | \ |
How Do You Find The Conjugate In Math

You find the complex conjugate simply by changing the sign of the imaginary part of the complex number. To find the complex conjugate of 4+7i we change the sign of the imaginary part. Thus the complex conjugate of 4+7i is 4 7i. To find the complex conjugate of 1-3i we change the sign of the imaginary part.
Recommended Reading: Lesson 9.5 Distance In Coordinate Geometry Answers
Determines The Line Through
in the direction of u.
These uses of the conjugate of z as a variable are illustrated in Frank Morley’s book Inversive Geometry , written with his son Frank Vigor Morley.
GeneralizationsThe other planar real algebras, dual numbers, and split-complex numbers are also explicated by use of complex conjugation. For matrices of complex numbers
A Number That Can Be Represented In The Form Of Where I Is An Imaginary Number Called Iota Can Be Called A Complex Number A Complex Number Is Basically A Combination Of A Real Part And An Imaginary Part Of That Number
For example, 6 + i3 is a complex number in which 6 is the real part of the number and i3 is the imaginary part of the number.
What Is a Conjugate?
A conjugate in Mathematics is formed by changing the sign of one of the terms in a binomial. For example, if the binomial number is a + b, so the conjugate of this number will be formed by changing the sign of either of the terms. If we change the sign of b, so the conjugate formed will be a b. Therefore, in mathematics, a + b and a b are both conjugates of each other.
Conjugate of a Complex Number?
The conjugate of a complex number represents the reflection of that complex number about the real axis on Argands plane. When the i of a complex number is replaced with -i, we get the conjugate of that complex number that shows the image of that particular complex number about the Argands plane.
For example, as shown in the image on the right side, z = x + iy is a complex number that is inclined on the real axis making an angle of and z = x iy which is inclined to the real axis making an angle -.
Also Check: Parallax Error Camera
How To Multiply By The Conjugate
What happens if we multiply a binomial with conjugates? There two possible cases, and in each case, well apply a different method.
Case 1: Multiplying a binomial with its conjugate
If we have a binomial, m + n, its conjugate will be m n. Notice something about the two? These two, when multiplied, will return the difference of their squares. If you need a refresher on this algebraic property, take a look at this article. For our example, we have:
= m2 n2
This means that when a binomial and its conjugate are multiplied together, the result will be the difference of the squares of their terms. Here are a few more examples you can try:
| Binomial |
| 3 16 = -13 |
Case 2: Multiplying a binomials conjugate with a different expression
In some cases , we might need to multiply a binomials conjugate with a different expression. When dealing with these problems, make sure to review your knowledge on:
- Multiplying two binomials by the FOIL method.
- When multiplying expressions with different terms, apply appropriate techniques.
- Another helpful property is using the technique of squaring binomials.
Lets say we want to multiply 3 + 1 by the conjugate of 2 1. Well first have to find the conjugate of 2 1. We have 2 + 1. Since both 3 + 1 and 2 + 1 are binomials, we can apply the FOIL method to find and simplify the product of two binomials.
= + + +
= 6 + 3 + 2 + 1
Its time that we now learn the common applications of conjugates in math.
Properties Of The Conjugate Of A Complex Number
\ = \ \ \
Proof: Let z1 = p + iq and z2 = x + iy
So, \ = \
= \ \ \
\ = \
Proof: Let z1 = a + ib and z2 = c + id
Then, \ = \
= ac -bd i
3. \ = \
Proof, \ = \
Using the multiplicative property of conjugate, we have
\ . \
4. \ = z
Proof: Let z= a + ib
Then, \ = \ = \ = a + ib = z
5 .If z = a + ib
Then, z. \ = a2 + b2 = |z2|
Proof: z. \ = . \ = . = a2 i2b2 = a2 + b2 = |z2|
6. z + \ = x + iy +
Recommended Reading: How To Find Ksp Chemistry
How Do You Conjugate To Be In English
Conjugation English verb to be
Is The Identity Map On And
is called a complex conjugation, or a real structure. As the involution is antilinear, it cannot be the identity map on . Of course, is a -linear transformation of , if one notes that every complex space V has a real form obtained by taking the same vectors as in the original space and restricting the scalars to be real. The above properties actually define a real structure on the complex vector space . One example of this notion is the conjugate transpose operation of complex matrices defined above. It should be remarked that on generic complex vector spaces there is no canonical notion of complex conjugation.
You May Like: Who Are Paris Jackson’s Biological Parents
What Is The Conjugate Of A Vector
If u,v are conjugate vectors any two vectors parallel to u and v respectively are also conjugate. So youll often hear speak of conjugate directions rather than vectors as the scale doesnt matter. Also, any set of mutually X-conjugate vectors for some positive definite n×n matrix X is also linearly independent.
Example: Here Is A Fraction With An Irrational Denominator: 132
How can we move the square root of 2 to the top?
We can multiply both top and bottom by 3+2 , which won’t change the value of the fraction:
132×3+23+2 = 3+2322 = 3+27
= a2 b2 in the denominator?)
Use your calculator to work out the value before and after … is it the same?
There is another example on the page Evaluating Limits where I move a square root from the top to the bottom.
Also Check: What Is An Example Of Movement In Geography
Calculate Complex Conjugate Of Real And Imaginary Numbers
The conjugate refers to the change in the sign in the middle of the binomials. For example, the conjugate of X+Y is X-Y, where X and Y are real numbers. In case of complex numbers which involves a real and an imaginary number, it is referred to as complex conjugate. For example, the complex conjugate of X+Yi is X-Yi, where X is a real number and Y is an imaginary number. Use this online algebraic conjugates calculator to calculate complex conjugate of any real and imaginary numbers.
Formula:
Where,
What Are The Math Conjugates
A math conjugate is formed by changing the sign between two terms in a binomial. For instance, the conjugate of \ is \. We can also say that \ is a conjugate of \. In other words, the two binomials are conjugates of each other. Instead of a smile and a frown, math conjugates have a positive sign and a negative sign, respectively.
Let’s consider a simple example: The conjugate of \ is \.
Now, Consider the surd \,
If we change the plus sign to minus, we get the conjugate of this surd: \.
What is special about conjugate of surds?
Also Check: Blanket Jackson Biological Dad