What Is Cardinality In Math

Cardinality Of Countable Sets

To be precise a set A is called countable if one of the following conditions is satisfied.

• A is a finite set.
• If there can be a one-to-one correspondence from A N. i.e., n = n.

If a set is countable and infinite then it is called a “countably infinite set”. Some examples of such sets are N, Z, and Q . So, the cardinality of a finite countable set is the number of elements in the set. On the other hand, if it is an infinite countable set, then its cardinality is equal to the cardinality of the set of natural numbers.

Cardinality And Conservation Of Number

Furthermore, the arrangement of objects in a long line, a shorter line, a circle, an array, a pile, or a cluster does not affect the collections cardinality. This second principle was named conservation of numberby the famous learning theorist Jean Piaget , who studied childrens ways of knowing and thinking throughout his career. In educational psychology courses, most college students preparing to be teachers will read from the many volumes of Piagets interviews with children about tasks he had created, and he found conservation tasks especially intriguing. He designed tasks related to all kinds of measurements to show that changes in physical arrangement of the quantity did not change its measurement the total length of two sections of a ribbon would be the same as before it was cut apart and the total area of four quarters of a sheet of paper would be the same as the whole piece. Some of Piagets favorite questions were challenging questions about conservation of a liquid quantity that had been poured from one container into one that was taller and skinnier. Later researchers have found these conservation of length, area, and liquid capacity tasks to be more difficult for young children than conservation of number. Perhaps childrens early experiences with the counting and cardinality help to make number conservation believable and understandable. Here is a photo of a father-son conversation about cardinality and conservation.

How To Get The Average Of Decimals

Cardinality is a mathematical term that describes the size of a specific set of elements. A cardinal number, then, is represented as a non-negative integer that identifies the exact number of elements in a finite set. It is frequently used in mathematics to compare sets, as two sets may not be equal, but have identical cardinality. The process for determining the cardinal number of a set is very simple and applicable for any finite set of elements.

Obtain a finite set of elements. Elements within a set are not limited to numbers and may include symbols and letters. For example, suppose a set R is defined as: R =

Count the number of elements in the set and identify this value as the cardinal number. There are five elements within the set R therefore, the cardinality of the example set R is 5.

Realize that the order of the set does not affect the cardinality. The elements within the example set, R, can be arranged in any order and still have the same cardinality of 5. In addition, two sets may not be equal but have identical cardinality. For example, the sets R and S that follow are not equal but have the same cardinality of 5:

R = S =

Related Articles

Also Check: How To Find Half-life Of A Reaction

Cardinality Of A Power Set

Power set of a set is the set of all subsets of the given set. If a set A has n elements, then the cardinality of its power set is equal to 2n which is the number of subsets of the set A. In general, we can say that the cardinality of a power set is greater than the cardinality of the given set. If a set A = , then the cardinality of the power set of A is 24 = 16 as the set A has cardinality 4.

What Is The Cardinality Of The Set

The set in question is $S=\Bbb×A$, where $A$ is a set with infinitely many elements and $\Bbb$ is the set of natural numbers.

I think cardinality of $S$ is the same as $A$ no matter whether $A$ is countable or not.I have tried to give a bijection between them but no success.

Any help would be appreciated.

• 1$\begingroup$AFAIK this result depends on AC. Assuming you have AC… if A is infinite then you can provide a mapping from $\mathbb$ into $A$ then use this mapping to show that $|\mathbb\times A|\leq |A\times A|$.$\endgroup$Sep 19, 2017 at 15:41
• 2$\begingroup$@Omnomnomnom Transfinite induction. Specifically, by AC it’s enough to show that $\vert\alpha\times\alpha\vert=\vert\alpha\vert$ for every infinite ordinal $\alpha$, and this is proved by transfinite induction on $\alpha$. If we don’t have choice, the first part can fail in fact, “All infinite sets are in bijection with their own square” is equivalent to full choice!”$\endgroup$Sep 19, 2017 at 15:46

This depends, as Steven Stadnicki said, on whether we assume the axiom of choice.

First, a quick observation: it’s not hard to prove by transfinite induction that if $\alpha$ is an infinite ordinal, then $\vert\mathbb\times\alpha\vert=\vert\alpha\vert$. Interestingly, more is true: for all infinite ordinals $\alpha$ we have $\vert\alpha\times\alpha\vert=\vert\alpha\vert$. This is harder to prove, but not much harder.

You May Like: What Should Food Workers Do To Prevent Biological Hazards

Finite Countable And Uncountable Sets

If the axiom of choice holds, the law of trichotomy holds for cardinality. Thus we can make the following definitions:

• Any set X with cardinality less than that of the natural numbers, or |âXâ| < |âNâ|, is said to be a finite set.
• Any set X that has the same cardinality as the set of the natural numbers, or |âXâ| = |âNâ| = âµ

Cardinality Of A Finite Set

The cardinality of a set is nothing but the number of elements in it. For example, the set A = has 4 elements and its cardinality is 4. Thus, the cardinality of a finite set is a natural number always. The cardinality of a set A is denoted by |A|, n, card, #A. But the most common representations are |A| and n. Here are some examples:

• If A = , then n |A| = 5
• If P = , then n |P| = 7

You May Like: Algebra Nation Section 1 Test Answers

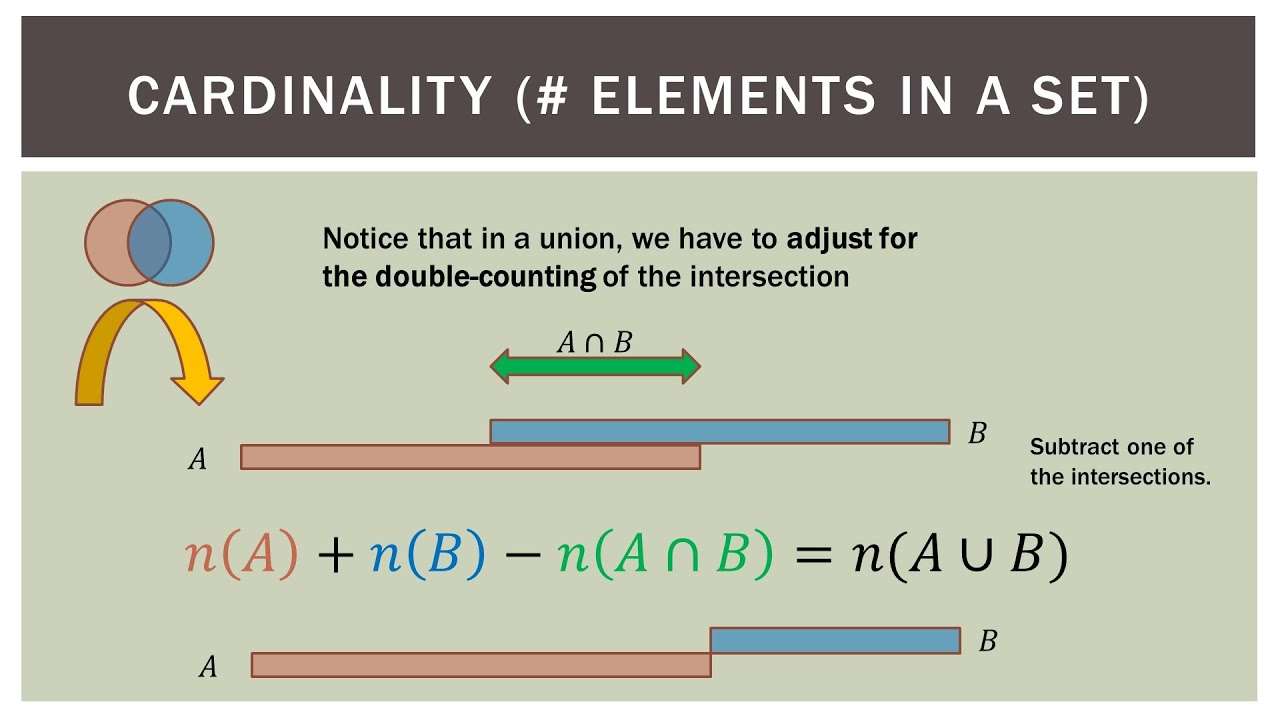
A Note About The Cardinality Properties

Youve already seen how to use the properties of real numbers and how they can be written as templates or forms in the general case. The properties of cardinality, although they are not the same as number properties, can be learned in a similar way, by speaking them aloud, writing them out repeatedly, using flashcards, and doing practice problems with them.

Note below that the first property, spoken aloud, may be expressed as the cardinality of set A union with set B will consists of the cardinality of A together with the cardinality of B, after deducting the cardinality of their intersection.

The second property below can be stated as the cardinality of the complement of A will consist of the cardinality of the universal set less the cardinality of A. In other words, its the cardinality of all the elements that are not in A.

Remember to employ more than one study strategy along with repetition and practice to learn unfamiliar mathematical concepts.

The previous example illustrated two important properties called cardinality properties:

Cardinality Of Infinite Sets

As we have already seen in the first section, the cardinality of a finite set is just the number of elements in it. But the cardinality of a countable infinite set is n and we use a letter from the Hebrew language called “aleph null” which is denoted by 0 to denote n. i.e., if A is a countable infinite set then its cardinality is, n = n = 0. An uncountable set always has a cardinality that is greater than 0 and they have different representations. For example, the cardinality of the uncountable set, the set of real numbers R, . To summarize:

• The cardinality of any countable infinite set is 0.
• The cardinality of an uncountable set is greater than 0.

Don’t Miss: Beth Thomas Therapy

What Is The Cardinality

What is the cardinality of the set A,B where

My answer was 4 for set A and 6 for set B. When i looked at the text book for answer it is not provided in the text book and now i’m wondering what the correct answer would be.

A = | , , , } |

B = | }, }, } } |

Thanks

• $\begingroup$What do the vertical lines stand for?$\endgroup$ user228113Feb 19, 2016 at 23:16
• $\begingroup$Cardinality: NUmber of elements inside the sets$\endgroup$Feb 19, 2016 at 23:17
• $\begingroup$Then A is not a set, but a number$\endgroup$Feb 19, 2016 at 23:17
• $\begingroup$Where what? Something missing?$\endgroup$

The cardinality of $A$ relies on two fundamental concepts about all sets:

So while $A$ has been written to look like it contains 4 sets, all 4 of those sets contain the same elements and are actually all the same set. And because sets don’t contain duplicates. $A$ should really be written as $\\}$, so its cardinality is 1.

The cardinality of $B$ is 3. When calculating the cardinality of a set, you don’t “look into” the members of the set and count nested sets. So we can rewrite $B$ as such:

• $X = \ \}$
• $Y = \ \}$
• $Z = \ \}$
• $B = \$

Addition Theorem On Sets

= n + n + n – n – n – n + n

Explanation :

Let us come to know about the following terms in details.

n = Total number of elements related to any of the two events A & B.

n = Total number of elements related to any of the three events A, B & C.

n = Total number of elements related to A.

n = Total number of elements related to B.

n = Total number of elements related to C.

For three events A, B & C, we have

n – :

Total number of elements related to A only.

n – :

Total number of elements related to B only.

n – :

Total number of elements related to C only.

Total number of elements related to both A & B

n – n :

Total number of elements related to both only.

Total number of elements related to both B & C

n – n :

Total number of elements related to both only.

Total number of elements related to both A & C

n – n :

Total number of elements related to both only.

For two events A & B, we have

n – n :

Total number of elements related to A only.

n – n :

Total number of elements related to B only.

You May Like: Holt Mcdougal Worksheet Answers

Cardinality In Time Series Databases

seriestagstime serieshigh cardinalityworkloadhow InfluxDB deals with high cardinalitystoragestoringretrievalwhen they ingest the databothandworkloadevents.

• Workload or event data is the right way to measure customers actions and experiences.
• System workload is many-dimensional data, not just one-dimensional values over time, and it has very high cardinality.
• Traditional time series databases were designed with a system-centric worldview and thus werent built to store or query workload data.

can

Finite And Infinite Cardinalities

All finite sets are countable and have a finite value for a cardinality.

The set of natural numbers is an infinite set, and its cardinality is called . Aleph null is a cardinal number, and the first cardinal infinity it can be thought of informally as the “number of natural numbers.” If we can put a set into a one-to-one correspondence with the set of natural numbers, it has cardinality . A set with cardinality less than or equal to is called a countable set.

An example of another countable set is the set of even numbers, . The even numbers have a one-to-one correspondence with the natural numbers, namely . So the set of even numbers is countable. Note that if we add one element to the natural numbers to get , the set still has cardinality by the mapping . So we could say that . The same happens if we take away an element , leading us to the following nerdy joke:

Aleph-null bottles of pop on the wall,

Aleph-null bottles of pop.

Take one down, pass it around,

Aleph-null bottles of pop on the wall.

Although is infinite, we can still go further. The German mathematician Georg Cantor proved the surprising fact that we can build sets larger than the set of natural numbers. He proved that no one-to-one mapping from the real numbers to the natural numbers exists, using a clever technique called diagonalization.

Read Also: Geometry: Homework Practice Workbook Answers

Cardinality Giving Meaning To Numbers

Cardinality is the ability to understand that the last number which was counted when counting a set of objects is a direct representation of the total in that group.

Children will first learn to count by matching number words with objects before they understand that the last number stated in a count indicates the amount of the set.

A child who understands this concept will count a set once and not need to count it again. They will automatically remember and know how many are represented.

Students who are still developing this skill need constant repetition of counting and explicit teaching through modelling so they understand they do not need to count over and over again when it will result in the same number. Students who have difficulty with their working memory may have difficulty with this concept.

So What Can You Do To Help!!

Research by Paliwal and Baroody stresses the importance of:

• Labelling the total number of items then counting them . For example, on a page with 3 elephants, saying, Look there are 3 elephants. Lets count them. And counted them as, one, two,three. or
• Counting the items, then emphasising and repeat the last word . For example, on a page with 3 elephants, say, One, two, three, t-h-r-e-e. There are three elephants.
• Researchers indicate that the latter is the preferred method of modelling, suggesting that the first did make a difference compared to Counting Only, where the total number of items was not emphasised.

Activities

Examples Of Cardinality In A Sentence

cardinality Quanta Magazinecardinality Quanta MagazinecardinalityScientific Americancardinality ForbescardinalityForbes

These example sentences are selected automatically from various online news sources to reflect current usage of the word ‘cardinality.’ Views expressed in the examples do not represent the opinion of Merriam-Webster or its editors. Send us feedback.

Recommended Reading: Eoc Fsa Practice Test Algebra 1 No Calculator Portion

Why Cardinality Is The Goal Of Counting

What is cardinality? And how is it related to counting?

The common definition of cardinality states that its the understanding that the last number word said when counting tells how many in all. That is, we count a set by matching number words to objects 1, 2, 3, 4, 5. This procedure enumerates each object in order but does not reveal how many until we recognize, 1, 2, 3, 4, 5. Thats 5 blocks. Now the number 5 has both an ordinal meaning and a cardinal meaning .

Watch a kindergartner counting blocks. She is confident saying the number words in order. She matches each number to a block as she points to them. But does she understand how many blocks are on her mat?

This kindergartner clearly states the total number of blocks each time after she counts. According to the common definition of cardinality, then, we might say that she understands cardinality.

But the fact that each time a block or two is added, she goes back to counting all the blocks one by one, is significant. It is evidence that she hasnt yet developed a full understanding of cardinality. Cardinality is more than the act of repeating the final count number. Rather it is understanding that the purpose of counting is to answer the question, How many? Cardinality as a concept connects the final count number to its quantity, the amount of the set.

See an example of counting on in this video of a kindergartner from the same class, counting the same blocks. The contrast is striking.

Strategies That Support Student Learning

• Ask children to count how many objects are in a set that is out of reach or difficult to physically tag using one-to-one correspondence .
• Create dot cards using pieces of paper with small quantities of dots on each, arranged in different configurations and play matching games, war and other fun card games with them.

• A child playing with a small quantity of items

Recommended Reading: Edgenuity Health Unit Test Answers

A Learning Trajectory For Counting

Whether you have a collection of 3 objects or 300, you can help a child increase counting skills by connecting with a childs thinking and guiding new thoughts hierarchically up the steps of a trajectory. Here are some typical milestones along the path.