Why Are We Learning This How Teaching Geometry Shapes Student Worldview
In this installment of our series on why learning matters, we explore the importance of geometry. From assisting with creative career paths to laying a foundation for viewing the world, geometry is used in ways many of us dont even realize. Heres why geometry is so important both for life skills and future careers.
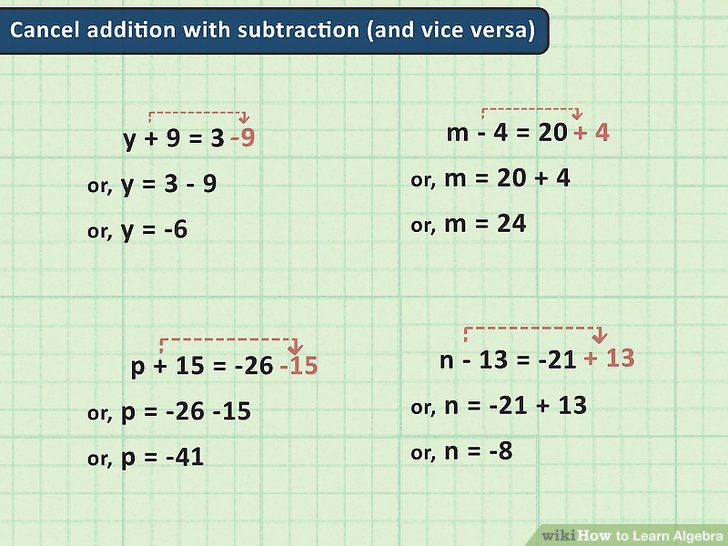
How To Study Basic Mathematics Algebra And Geometry
The following tips are just my advice They are not absolute. Choose those that will best fit you.
- Plan a regular time to study
- Choose a quiet place where you will not be distracted.
- When you study, try to study an entire lesson, or an entire chapter.
- When you finish a whole lesson or a whole chapter, it may leave you with a great feeling of accomplishment
- When you feel sleepy, take a short break you will not learn much, if anything if you are not alert.
- You are now ready to experience the most rewarding endeavor right here in this website.
This lesson will show you how to construct parallel lines with easy to follow steps
Unexpected Career Applications Of Geometry
Students who arent strong in math especially those who prefer more creative subjects may be particularly disinterested in geometry. However, relating geometric principles to their strengths can make mathematics more engaging. Meena Mehta at learning app Toppr explains how mathematics and art are actually related in many ways.
The theory of perspective showed that there is more to geometry than just the metric properties of figures: and this perspective is the basis of the origin of projective geometry, she writes.
Mehta also points out that geometry is an essential component of computer aided design software, which is used in a variety of creative professions, including architecture.
Laurie Brenner at Sciencing agrees that geometry is essential in the use of CAD software, which most architecture, engineering and contracting jobs require. Before a contractor builds a structure, someone must design the buildings shape and create blueprints. A computer outfitted with computer-aided design software contains the math to render the visual images on the screen, she explains.
While young students may not understand the concept of CAD, they can understand the relationship between a physical structure and concepts of geometry.
Recommended Reading: Ccl4 Vsepr Model
Signs Symbols And Terminology
The shape illustrated here is an irregular pentagon, a five-sided polygon with different internal angles and line lengths .
Degrees ° are a measure of rotation, and define the size of the angle between two sides.
Angles are commonly marked in geometry using a segment of a circle , unless they are a right angle when they are squared off. Angle marks are indicated in green in the example here. See our page on Angles for more information.
Tick marks indicate sides of a shape that have equal length . The single lines show that the two vertical lines are the same length while the double lines show that the two diagonal lines are the same length. The bottom, horizontal, line in this example is a different length to the other 4 lines and therefore not marked. Tick marks can also be called hatch marks.
A vertex is the point where lines meet . The plural of vertex is vertices. In the example there are five vertices labelled A, B, C, D and E. Naming vertices with letters is common in geometry.
In a closed shape, such as in our example, mathematical convention states that the letters must always be in order in a clockwise or counter-clockwise direction. Our shape can be described ABCDE, but it would be incorrect to label the vertices so that the shape was ADBEC for example. This may seem unimportant, but it is crucial in some complex situations to avoid confusion.
If you want to write the measured angle at point B in shorthand then you would use:
mABC = 128°
mEAB=90°
Points: A Special Case: No Dimensions
A point is a single location in space. It is often represented by a dot on the page, but actually has no real size or shape.
You cannot describe a point in terms of length, width or height, so it is therefore non-dimensional. However, a point may be described by co-ordinates. Co-ordinates do not define anything about the point other than its position in space, in relation to a reference point of known co-ordinates. You will come across point co-ordinates in many applications, such as when you are drawing graphs, or reading maps.
Almost everything in geometry starts with a point, whether its a line, or a complicated three-dimensional shape.
Also Check: Fsa Warm Ups Grade 5 Answer Key
The Time4learning Curriculum Structure
Time4Learning has been refined through years of feedback from educators, parents, and students. Subjects are organized into chapters composed of interactive lessons, , quizzes and tests. Students are guided through the activities at their own pace by an automated system.
When students log in, they choose a subject, select a chapter, pick a lesson and complete the activities. A check-mark tells them where they left off, and completed work is clearly labeled with a check-mark or a gold star. Visual and auditory prompts guide students through the lessons, making it easy for even young learners to follow, and an online playground rewards and motivates them to finish their lessons.
Parents get access to printable lesson plans, teaching tools, detailed reporting and parental support through our onlineParent Forum.
Does your child have different achievement levels for math and language arts? No problem! Time4Learning lets you set each individual subject at the appropriate graded level, making this curriculum great for special needs and gifted students.
What Kids Learn In Kindergarten
As parents its hard to keep up with our kids learning schedules. When are they supposed to start learning addition? By what age should they be able to read? Are they on track with their grade-level learning or do we need to help them catch up?
These are all questions we thought wed cover in our next series on what kids learn in each grade. Today, well start with kindergarten.
Recommended Reading: Kuta Software Operations With Complex Numbers
When Should Kids Learn To Read Write And Do Math
Your child starting to read is just one of many educational milestones to watch for as a parent
At one time or another, most parents wonder how their child is stacking up in school. Part of answering that is knowing when kids should learn to read, write, and do different kinds of math?
Ross A. Thompson, PhD, professor of psychology at the University of California at Davis tells WebMD there is a wide range of normal variation in many areas for young children. This can make it difficult, he says, to tell if a delay is really a problem. Thompson also says that measuring children against defined age benchmarks sometimes raises undue anxiety in parents.
Still, general milestones can be helpful to parents as a guide. Missing a milestone doesn’t always mean a child has a learning deficit or disability. It may simply mean that you need to make some changes in the classroom or at home to help your child learn and reach their full potential.
Fundamental Concepts Of Geometry
This video explains and demonstrates the fundamental concepts of geometry: points,lines, ray, collinear, planes, and coplanar. The basic ideas in geometry and how we represent themwith symbols.
A point is an exact location in space. They are shown as dots on aplane in 2 dimensions or a dot in space in 3 dimensions. It is labeled with capital letters. It doesnot take up any space.
A line is a geometric figure that consists of an infinite number ofpoints lined up straight that extend in both directions for ever .A line is identified by a lower case letter or by two points that the line passes through. There isexactly 1 line through two points. All points on the same line are called collinear. Points not onthe same line are noncollinear.
Two lines are either parallel or they will meet at a point of intersection.
A line segment is a part of a line with two endpoints. A line segmentstarts and stops at two endpoints.
A ray is part of a line with one endpoint and extends in one directionforever.
A plane is a flat 2-dimensional surface. A plane can be identifiedby 3 points in the plane or by a capital letter. There is exactly 1 plane through three points. Theintersection of two planes is a line.
Coplanar points are points in one plane.
Don’t Miss: What Is The Difference Between Electron Geometry And Molecular Geometry
Geometry And Spatial Thinking
Geometry is essential for helping children understand spatial relationships. This is detailed in the report Mathematics Learning in Early Childhood: Paths Toward Excellence and Equity, co-edited by Taniesha A. Woods, Ph.D..
Spatial relationships are important even for young children, because it helps them understand their place in the world. It teaches them to determine how large a room is, how far away a desk is or which way to move. Geometry allows students to connect mapping objects in the classroom to real-world contexts regarding direction and place.
Understanding of spatial relationships is also considered important in the role of problem solving and higher-order thinking skills. Kindergarten specialist Edward Schroeter emphasizes the importance of stocking a classroom with objects and ideas that can reinforce spatial learning. Since young children learn best by working with concrete objects and through stories, educators should stock their classrooms with picture books that model building and design, spatial vocabulary, spatial gestures, and spatial and geometric concepts, he writes.
Puzzles, blocks, shape sorters and building toys are fun and engaging elements that inspire young students to learn more about shapes. Paper folding tasks, like origami and airplane making, help students with the tactile aspect of learning geometry.
Principle : Let It Make Sense
Let us strive to teach for understanding of mathematical concepts and procedures, the “why” something works, and not only the “how”.
This understanding, as I’m sure you realize, doesn’t always come immediately. It may take even several years to grasp a concept. For example, place value is something children understand partially at first, and then that deepens over a few years.
This is why many math curricula use spiraling: they come back to a concept the next year, the next year, and the next. This can be very good if not done excessively .
However, spiraling has pitfalls also: if your child doesn’t get a concept, don’t blindly “trust” the spiraling and think, “Well, she gets it the next year when the book comes back around to it.”
The next year’s schoolbook won’t necessarily present the concept at the same level – the presentation might be too difficult. If a child doesn’t “get it”, they might need very basic instruction for the concept again.
The “how” something works is often called procedural understanding: the child knows how to work long division or knows the procedure for fraction addition. It is often possible to learn the “how” mechanically without understanding why something works. Procedures learned this way are often forgotten very easily.
As a rule of thumb, don’t totally leave a topic until the student both knows “how” and understands the “why”.
Read Also: Kuta Software Infinite Algebra 2 Systems Of Inequalities Answer Key
Principle : Remember The Goals
What are the goals of your math teaching? Are they…
- to finish the book by the end of school year
- make sure the kids pass the test …?
Or do you have goals such as:
- My student can add, simplify, and multiply fractions
- My student can divide by 10, 100, and 1000.
These are all just “subgoals”. But what is the ultimate goal of learning school mathematics?
Consider these goals:
The more you can keep these big real goals in mind, the better you can connect your subgoals to them. And the more you can keep the goals and the subgoals in mind, the better teacher you will be.
For example, adding, simplifying, and multiplying fractions all connect with the broader goal of understanding part-and-whole relationships. It will soon lead to ratios, proportions, and percent. Also, all fraction operations are a necessary basis for solving rational equations and for the operations with rational expressions .
Tying in with the goals, remember that the BOOK or CURRICULUM is just a tool to achieve the goals not a goal in itself. Don’t ever be a slave to any math book.
Why Cant I Do Geometry

Students are often faced with many doubts like Why is geometry so hard?Is geometry hard?Why can’t I do geometry?Why learn geometry?How hard is geometry and Geometry is hard. And thus, discussing this is integral in clearing these conceptions to the point they thinking Geometry is easy!.
Children often have this fear in their minds that geometry is hard and ask why I can’t do geometry. Removing this fear of geometry is hard is the first step that should be taken by you as a parent. It’s possible that children are not vocal about it, and you may have to identify this difficulty for them. Observe if they are:
Also Check: Who Is Prince Jackson’s Biological Father
How We Developed The New Curriculum
This new curriculum was informed by the results of Ontarios 2018 public consultation with parents, educators and stakeholders about the areas of focus that would help improve student achievement.
The curriculum has also been informed by extensive research led by Dr. Christine Suurtamm, Vice-Dean of Research and Professional Development and Full Professor of Mathematics Education at the Faculty of Education, University of Ottawa, with input from academics and education experts in the area of math learning.
To understand current approaches to teaching math, we researched trends in high achieving regions and reviewed best practices in math education.
We continue to work with leaders, researchers and teachers in math education to ensure the new curriculum is relevant and meets the needs of Ontario.
Find Out What Students Learn Grade By Grade
Key concepts and skills like addition, subtraction, division and multiplication help set the stage for more advanced skills like working with decimals, data and integers.
New areas like coding, mathematical modelling and financial literacy will help provide the foundation students need to be competent and succeed in the world today. Prioritizing their well-being will help give students the tools and confidence to approach math positively, enjoy and appreciate mathematics as well as overcome any anxiety they may experience.
Here are some of the knowledge and skills that students are expected to learn.
Number
Students work with numbers up to 50 and begin to develop an understanding of the ways we use numbers. They are also introduced to the idea of fractions, through the context of sharing things equally.
Algebra
Students begin to look at how patterns can be used to make predictions. They also begin to work on the idea that in a number sentence both sides must be equal to each other. These ideas are foundational to algebra work in later grades. Students will begin to write code to order a sequence of steps. They will also be introduced to mathematical modelling to analyze and create solutions for real-life situations, such as creating a seating arrangement for a class event.
Data
Spatial sense
Financial literacy
Students learn to recognize Canadian coins and bills and compare their values.
Social emotional learning skills and math processes
Don’t Miss: Is Paris Jackson Michael Jacksons Biological Daughter
Principle : Know Your Tools
A math teacher’s tools are quite numerous nowadays.
First of all of course comes a black or white board or paper something to write on, then we have pencils, compass, protractor, ruler, eraser…. And the book you’re using.
Then we have computer software, interactive activities, animated lessons and such.There are workbooks, fun books, worktexts, books, and online tutorials.Then we have manipulatives, abacus, measuring cups, scales, algebra tiles, and so on. And then there are games, games, games.
The choices are so numerous it’s daunting. What’s a teacher to do?
Well, you just have to start somewhere, probably with the basics, and then add to your “toolbox” little by little as you have opportunity.
There is no need to try ‘hog’ it all at once. It’s important to learn how to use any tool you might acquire. Quantity won’t equal quality. Knowing a few “math tools” inside out is more beneficial than a mindless dashing to find the newest activity to spice up your math lessons.
Science And Social Studies
In fifth grade, science includes lessons on the human body and its systems, basic biology and chemistry, and timely topics like climate change and humans’ impact on the environment. Students continue learning about the planet, weather, land, and oceans.
In social studies, students learn the different branches of government. They study the United States Constitution and the system of checks and balances in place to protect it. Important events in our nation’s history are explored along with important historical figures.
Also Check: Geometry Chapter 4 Practice Workbook Answers
Do You Need Algebra For Geometry
Again, the answer to this question can depend on each schools policy and each students personal ability. However, in regard to common curriculum for many schools, students will need to know algebra in order to succeed in their geometry classes. Many geometric questions will include at least one step of algebra, as they often fuse geometry and algebra concepts together. For example, students could encounter a question about similar triangles in geometry class with the sides labeled with expressions like x-2. Therefore, in order to solve this problem, students would have to apply their previous knowledge of algebraic expressions.
Specifically for the SAT, algebra and geometry play very close roles within the exam. Its important to build up a foundation of algebra knowledge, as many geometry questions within the exam do involve algebra, similar to the hypothetical question from the previous paragraph. For this reason, algebra is necessary in most curriculums for geometry. Students should build a good base understanding of algebra in order to succeed in higher level math classes, which include geometry.
Students should do some research about their schools policy and curriculum if they are curious about what order to take certain math classes. If possible, speaking to the math teachers at the school can give helpful and detailed information to guide any decisions.