The Difference Between Frequency And Relative Frequency
To see the difference between frequency and relative frequency we will consider the following example. Suppose we are looking at the history grades of students in 10th grade and have the classes corresponding to letter grades: A, B, C, D, F. The number of each of these grades gives us a frequency for each class:
- 7 students with an F
- 9 students with a D
- 18 students with a C
- 12 students with a B
- 4 students with an A
To determine the relative frequency for each class we first add the total number of data points: 7 + 9 + 18 + 12 + 4 = 50. Next we, divide each frequency by this sum 50.
- 0.14 = 14% students with an F
- 0.18 = 18% students with a D
- 0.36 = 36% students with a C
- 0.24 = 24% students with a B
- 0.08 = 8% students with an A
The initial data set above with the number of students who fall into each class would be indicative of the frequency while the percentage in the second data set represents the relative frequency of these grades.
An easy way to define the difference between frequency and relative frequency is that frequency relies on the actual values of each class in a statistical data set while relative frequency compares these individual values to the overall totals of all classes concerned in a data set.
How Do You Find The Relative Value
To use the relative value formula, SmartAsset indicates that one method is to divide the price of one security by that of the other and multiply the result by 100 for each day in your range. If the relative value is far lower than its historic average, the stock in the numerator is cheap by historic standards.
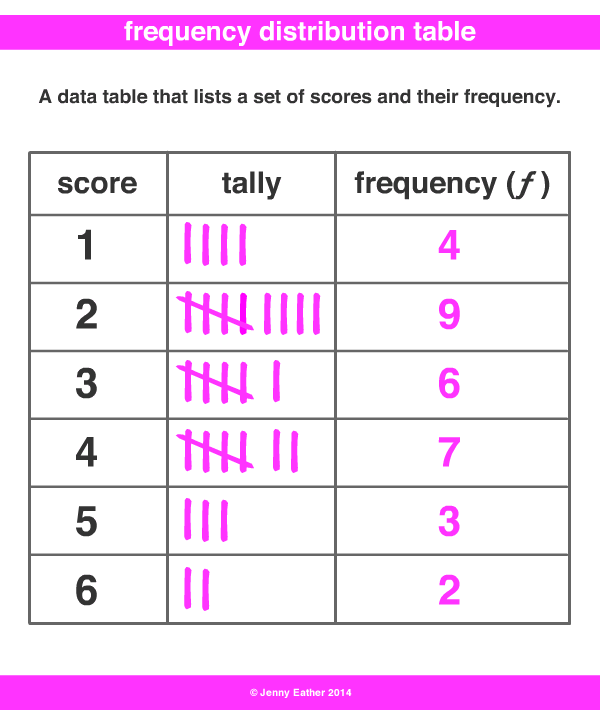
What Is Frequency Distribution
Frequency distribution is used to organize the collected data in table form. The data could be marks scored by students, temperatures of different towns, points scored in a volleyball match, etc. After data collection, we have to show data in a meaningful manner for better understanding. Organize the data in such a way that all its features are summarized in a table. This is known as frequency distribution.
Let’s consider an example to understand this better. The following are the scores of 10 students in the G.K. quiz released by Mr. Chris 15, 17, 20, 15, 20, 17, 17, 14, 14, 20. Let’s represent this data in frequency distribution and find out the number of students who got the same marks.
| Quiz Marks | |
|---|---|
| 14 | 2 |
We can see that all the collected data is organized under the column quiz marks and the number of students. This makes it easier to understand the given information and we can see that the number of students who obtained the same marks. Thus, frequency distribution in statistics helps us to organize the data in an easy way to understand its features at a glance.
Recommended Reading: Common Core Algebra 1 Homework Answers
Key Facts And Summary
What Is Relative Frequency
In mathematics, the relative frequency of events is defined as the ratio of the number of successful tests to the total number of tests performed. Relative frequency is simply the number of times something happened divided by the number of all attempts. The relative frequency distribution must be in the percentage.
Since this is experimental, different relative frequencies can be obtained by repeating the experiment. To calculate the frequency, we need to calculate:
- Calculate the frequency of the entire population
- Calculate the frequency of a subgroup of the population
Recommended Reading: Physics Is The Most Basic Science Because
Relative Frequency Distributions: Tables And Graphs
A relative frequency distribution describes the relative frequencies for all possible outcomes in a study. While a single value is for one type of event, the distribution displays percentages for all possible results. Analysts typically present these distributions using tables and bar charts.
Lets bring them to life by working through an example!
Types Of Frequency Distribution
There are four types of frequency distribution under statistics which are explained below:
- Ungrouped frequency distribution: It shows the frequency of an item in each separate data value rather than groups of data values.
- Grouped frequency distribution: In this type, the data is arranged and separated into groups called class intervals. The frequency of data belonging to each class interval is noted in a frequency distribution table. The grouped frequency table shows the distribution of frequencies in class intervals.
- Relative frequencydistribution: It tells the proportion of the total number of observations associated with each category.
- Cumulative frequencydistribution: It is the sum of the first frequency and all frequencies below it in a frequency distribution. You have to add a value with the next value then add the sum with the next value again and so on till the last. The last cumulative frequency will be the total sum of all frequencies.
You May Like: Steve Harvey How Many Kids
How Do You To Find Relative Frequency In Probability
Relative frequency or experimental probability is calculated from the collection of instances an match occurs, divided via the whole selection of trials in an actual experiment. The theoretical probability of getting a head whilst you turn a fair coin is , but if a coin was once actually flipped One hundred times you would possibly not get precisely 50
Difference Between Frequency And Relative Frequency
Science has evolved so much, and a lot of things have changed as a result. One thing that has remained the same is the fact that nothing is constant. Like the good book will put it there is nothing permanent under the sun, everything is under probability.
Probability expresses the belief that an experiment will turn out in a number of ways. To better describe how this works, were going to review the difference between relative frequency and frequency.
This will throw more light on the different number of results that can be gotten from virtually any event at all. But before we go into the difference between frequency and relative frequency, lets take some time to learn what they really mean.
You May Like: Paris Jackson Biological Parents
Cumulative Relative Frequency Distributions
A cumulative relative frequency distribution sums the progression of relative frequencies through all the possible outcomes. Creating this type of distribution entails adding one more column to the table and summing the values as you move down the rows to create a running, cumulative total.
For this example, well return to school students. The cumulative relative frequency table below adds the final column.
| School Grade | |
| 88 | 100% |
To find the cumulative value for each row, sum the relative frequencies as you work your way down the rows. The first value in the cumulative row equals that rows relative frequency. For the 2nd row, add that rows value to the previous row. In the table, we add 26.1 + 22.7 = 48.8%. In the third row, add 17% to the previous cumulative value, 17 + 48.8 = 65.8%. And so on through all the rows.
The final cumulative value must equal 1 or 100%, excepting rounding error.
You can also display cumulative relative frequency distributions on graphs. In the chart below, I added the orange cumulative line. Use these cumulative distributions to determine where most of the events/observations occur. In the example data, the first and second graders comprise about half the school.
To learn about functions that describe distributions, read my post, Understanding Probability Distributions.
Frequencies Vs Relative Frequencies
A frequency is a count of a particular event. For example, Jim read ten statistics books this year. The football team won 12 games. For more information, read my post about frequency tables.
In contrast, relative frequencies do not use raw counts. Instead, they relate the count for a particular type of event to the total number of events using percentages, proportions, or fractions. Thats where the term relative comes ina specific tally relative to the total number. For instance, 25% of the books Jim read were about statistics. The football team won 85% of its games.
If you see a count, its a frequency. If you see a percentage, proportion, ratio, or fraction, its a relative frequency.
Relative frequencies help you place a type of event into a larger context. For example, a survey indicates that 20 students like their statistics course the most. From this raw count, you dont know if thats a large or small proportion. However, if you knew that 30 out of 40 respondents indicated that statistics was their favorite, youd consider it a high number!
Additionally, they allow you to compare values between studies. Imagine that different sized schools surveyed their students and obtained different numbers of respondents. If 30 students indicate that statistics is their favorite, that could be a high percentage in one school but a low percentage in another, depending on the total number of responses.
Relative frequencies facilitate apples-to-apples comparisons.
You May Like: Algebraic Proof Worksheet Answer Key
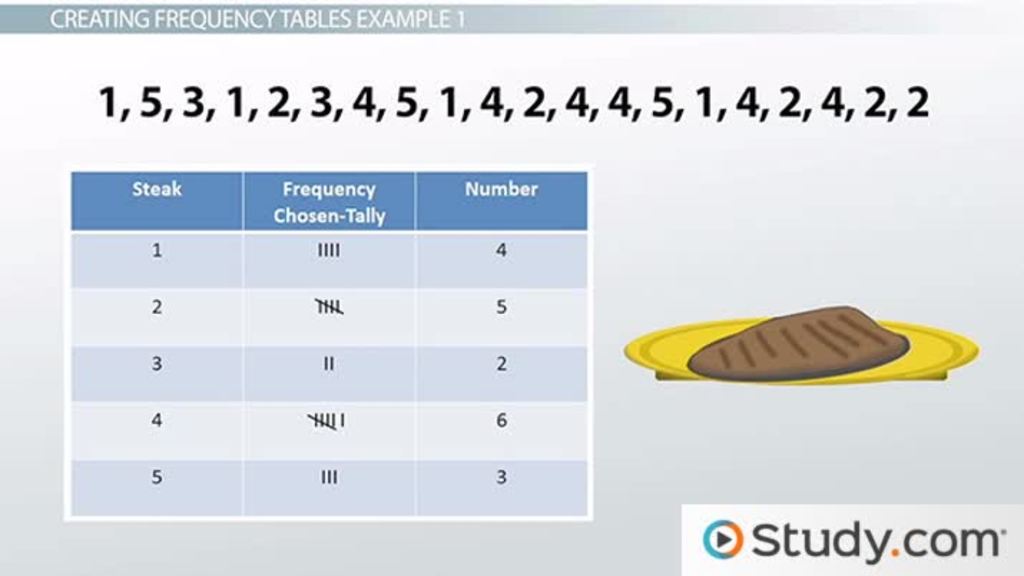
What Does Frequency Mean In Mathematics
In math, the frequency is the number of times a specific value appears in a data set or list. To find the frequency of these values, one constructs a frequency table and inputs all the different values from the set.
For example, for the data set 2, 5, 4, 7, 2, 8, 2, 6, 7, 2, one makes a table with columns, where the first column contains these given values in ascending order as 2, 4, 5, 6, 7 and 8. The table also has a tally column and frequency column. One records the tally in the second column and the frequency in the third column.
After constructing a table for the given values of the data set, one sees that for the value 2, the frequency is 4, for 7 the frequency is 2, and the other values 4, 5, 6, 8 have a frequency of 1 each.
How Do You Provide An Explanation For Frequency To A Child
Frequency is the collection of instances a worth occurs in a suite of knowledge. For instance, Victor attempted nine times to get a purple gumball. The frequency in this case will be the collection of each colour of gumballs that got here out. Lets look at our numbers of each and every color on a frequency desk, which presentations how incessantly an match took place.
Recommended Reading: Beth Thomas Age
How To Calculate Relative Frequency
The ratio of the number of times a value of the data occurs in the set of all outcomes to the number of all outcomes gives the value of relative frequency.
Lets understand the Relative Frequency formula with the help of an example
Lets look at the table below to see how the weights of the people are distributed.
Step 1: To convert the frequencies into relative frequencies, we need to do the following steps.
Step 2: Divide the given frequency bt the total N i.e 40 in the above case.
Step 3 : Divide the frequency by total number Lets see how : 1/ 40 = 0.25.
Example: Let us solve a few more examples to understand the concepts better.
This is a frequency table to see how many students have got marks between given intervals in Maths.
| 7 | 1 / 40 x 100 = 0.025 |
It is necessary to know the disparity between the theoretical probability of an event and the observed relative frequency of the event in test trials. The theoretical probability is a number which is calculated when we have sufficient information about the test. If each probable outcome in the sample space is equally likely, then we can consider the number of outcomes of a happening and the number of outcomes in the sample space to calculate the theoretical probability.
Is Relative Frequency Equivalent To Probability
Another means of expressing the connection is to explain the relative frequency of each outcome. The relative frequency is the fraction of occasions each and every consequence is accomplished. Based in this assumption, we will be able to state that the predicted relative frequency of an outcome is equal to the probability of that result.
You May Like: My Hrw Com Algebra 2
What Is A Relative Frequency Distribution
A relative frequency distribution is a type of frequency distribution.
The first image here is a frequency distribution table. A frequency distribution table shows how often something happens. In this particular table, the counts are how many people use certain types of contraception.
A frequency distribution table.
relative frequency distribution, percentages
This relative frequency distribution table shows how peoples heights are distributed.
Image: SHU.edu
This information can also be turned into a frequency distribution chart. This chart shows the relative frequency distribution table and the frequency distribution chart for the information. How to we know its a frequency chart and not a relative frequency chart? Look at the vertical axis: it lists frequency and has the counts:
Image: SHU.edu
Chart showing how book sales compare to each other as percentages of a whole.
How Do You Find Frequency Distribution
Follow the steps to find frequency distribution:
- Step 1: To make a frequency chart, first, write the categories in the first column.
- Step 2: In the next step, tally the score in the second column.
- Step 3: And finally, count the tally to write the frequency of each category in the third column.
Thus, in this way, we can find the frequency distribution of an event.
Also Check: Does Kamala Harris Have Any Biological Children
Solved Examples Using Relative Frequency Formula
Example 1: A cubical die is tossed 30 times and lands 5 times on the number 6. What is the relative frequency of observing the die land on the number 6?
Solution: Given, number of times a die is tossed = 30Number of the successful trials of getting number 6 = 5By the formula, we know,Relative frequency = Number of positive trial / Total number of trialsf = 5/ 30 = 16.66%
Answer: The relative frequency of observing the die land on the number 6 is 16.66%
Example 2: Anna has a packet containing 20 candies. Her favorites are the yellow ones and the red ones. The table below shows the frequency of each different candy selected as she picked all 20 sweets one by one and finished them all.
| Candy color |
|---|
A) What is the relative frequency of the picked candy being one of her favorites?
B) What is the relative frequency for the brown candy
Solution: Relative frequency = number of times an event has occurred / number of trials
A) Relative frequency of the picked candy to be one of her favorites:
/ 20 = 12/ 20 = 60%
B) Relative frequency of the brown candy
Frequency of brown candy/ 20 = 5/ 20 = 25%
Answer: 60% and 25%
Example 3: A coin is flipped 100 times, the coin lands on heads 48 times. What is the relative frequency of the coin landing on tails?
Solution: Relative frequency = number of times an event has occurred / number of trials
The event in consideration is the coin landing on tails = 100 – 48 = 52 times
Relative frequency of the coin landing on tails = 52/100 = 0.52 = 52%
Probability Frequency Vs Relative Frequency
Can we say that the probability and the relative frequency are the same or do they differ from each other? Probability and the relative frequency are certainly not the same. Let us understand how probability is different from the relative frequency. We know that Probability is the measure of an expected event or an event that might occur. This means that probability is useful in the cases when each outcome is equally likely. On the other hand, Relative frequency on the contrary measures an actual event that has already occurred. In other words, while relative frequency is a practical approach, the probability is a theoretical concept.
| Probability | Relative Frequency |
| Probability is the measure of an expected event or an event that might occur. | Relative frequency is the ratio of the number of times a value of the data occurs in the set of all outcomes to the number of all outcomes. |
| It is useful in the cases when each outcome is equally likely. | It measures an actual event that has already occurred. |
| It is a theoretical concept | It is a practical approach |
Let us understand this through an example.
We know that in a deck of 52 cards, 26 of the cards are white while the other 26 cards are black. Suppose, we wish to draw a white car from the deck. What would be the probability of this draw?
We know that Probability = $\frac$
Now, in this case, we will have
Favourable number of outcomes = 26
Total number of outcomes = 52
Probability = $\frac$ = $\frac$ = 0.5
Hence,
Read Also: Percent Difference Formula Chemistry
Types Of Frequency Distribution Table
There are two types of frequency distribution tables: Grouped and ungrouped frequency distribution tables.
Grouped Frequency Distribution Table: To arrange a large number of observations or data, we use grouped frequency distribution table. In this, we form class intervals to tally the frequency for the data that belongs to that particular class interval.
For example, Marks obtained by 20 students in the test are as follows. 5, 10, 20, 15, 5, 20, 20, 15, 15, 15, 10, 10, 10, 20, 15, 5, 18, 18, 18, 18. To arrange the data in grouped table we have to make class intervals. Thus, we will make class intervals of marks like 0 â 5, 6 â 10, and so on. Given below table shows two columns one is of class intervals and the second is of frequency . In this, we have not used tally marks as we counted the marks directly.
| No. of Students |
|---|