What Does Finite Mean In Math

Is Empty Set A Finite Set

Finite Math: Introduction to Markov Chains

An empty set is a set which has no elements in it. It is represented as , which shows that there is no element in the given set. The cardinality of an empty set is 0 as the number of elements is zero.

A= or n=0.

The finite set is a set with countable elements. As the empty set has zero elements in it, so it has a definite number of elements.

Therefore, an empty set is a finite set with cardinality zero.

Graphical Representation Of Finite And Infinite Sets

Here in the above picture,

A = B = A U B = AB =

Both A and B are finite sets as they have a limited number of elements.

n = 5 and n = 5

AUB and AB are also finite.

So, a Venn diagram can represent the finite set but it is difficult to do the same for an infinite set as the number of elements cant be counted and bounced in a circle.

Algebraic Geometry And Commutative Algebra

In algebraic geometry, a singularity of an algebraic variety is a point of the variety where the tangent space may not be regularly defined. The simplest example of singularities are curves that cross themselves. But there are other types of singularities, like cusps. For example, the equation y2 x3 = 0 defines a curve that has a cusp at the origin x = y = 0. One could define the x-axis as a tangent at this point, but this definition can not be the same as the definition at other points. In fact, in this case, the x-axis is a “double tangent.”

For affine and projective varieties, the singularities are the points where the Jacobian matrix has a rank which is lower than at other points of the variety.

Recommended Reading: What Is The Molecular Geometry Of Ccl4

From The American Heritage Dictionary Of The English Language 5th Edition

adjective Having bounds; limited.
adjective Existing, persisting, or enduring for a limited time only; impermanent.
adjective Being neither infinite nor infinitesimal.
adjective Having a positive or negative numerical value; not zero.
adjective Possible to reach or exceed by counting. Used of a number.
adjective Having a limited number of elements. Used of a set.
adjectiveGrammar Of or relating to any of the forms of a verb that can occur on their own in a main clause and that can formally express distinctions in person, number, tense, mood, and voice, often by means of conjugation, as the verb sees in She sees the sign.
noun A finite thing.

Are Two Sets A And B Equal

Definition 2: Two sets A and B are said to be equivalent if they have the same cardinality i.e. n = n. In general, we can say, two sets are equivalent to each other if the number of elements in both the sets is equal. And it is not necessary that they have same elements, or they are a subset of each other.

Also Check: Exponential Growth And Decay Common Core Algebra 1 Homework Answers

What Is The Difference Between The Finite And Infinite Set

Finite sets are sets that have a fixed number of elements and are countable and can be written in roster form. An infinite set is a set that is not finite and the elements of the set are endless or uncountable and cannot be written in roster form. This is the basic difference between finite and infinite sets.

How To Know If A Set Is Finite Or Infinite

As we know that if a set has a starting point and an ending point both, it is a finite set, but it is infinite if it has no end from any side or both sides.

Points to identify a set is whether a finite or infinite are:

An infinite set is endless from the start or end, but both the side could have continuity unlike in Finite set where both start and end elements are there.
If a set has the unlimited number of elements, then it is infinite and if the elements are countable then it is finite.

Properties Of Bernoulli Trials

To be a Bernoulli Trial, these conditions must be true for the trial.

·;;;;;; Only two possible outcomes

·;;;;;; Each outcome has a fixed probability of occurring; a success has the probability of p, and a failure has the probability of 1 p.

·;;;;;; Each trial is completely independent of all others.

Note: Consider the experiment of selecting a card from a deck and a face card is success. We know on the first trial p=3/13, but what if we do not replace the card before the second trial? This would cause the two trials to be dependent and thus would not be Bernoulli trials.

Are Exclamation Points Flirty

[Discrete Mathematics] Finite State Machines

1 | Punctuation: Exclamation point! Because when you start overusing it, you look like an overeager, un-confident amateur. However, when used properly, an exclamation point can set a light, flirtatious tone can convey excitement and can even demonstrate interest in the person.

Also Check: Eoc Fsa Warm Ups Algebra 1 Answers

Cardinality Of Empty Finite Set

An empty set is a set that has no elements and can be represented as or;;. For example P = Or . Set P is an empty set. As we discussed above the finite set has a countable number of elements. The empty set has zero elements so, it is having a finite number of elements with no continuity. So, an empty set is a finite set with a cardinality of zero.

The Goal Of Finite Math

In finite math classes, the goal is to give students enough information to use mathematical analysis in the real world, at jobs or at home. Topics covered include matrix algebra, probability, statistics, logic and discrete mathematics. You learn simple, immediately useful ways to count, calculate, add, subtract, multiply and divide. While success in finite math can be immensely helpful in the real world, it does not necessarily prepare you for a full calculus class.

Finite And Infinite Sets Venn Diagram

A Venn diagram is formed by overlapping closed curves, mostly;circles, each representing a set, or in other words, it is a;figure used to show the relationships among sets, or groups of objects. The;given below image of Venn diagram shows the relation between finite set and infinite set.

In the above image, Set containing elements is a finite set, and a set of natural numbers and a set of whole numbers are infinite sets. There are multiple finite sets that can be created from an infinite set. The image given above is showing one example of it where a finite set is lying inside infinite sets.

Difference Between Finite And Infinite Sets

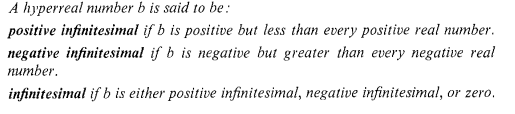
Finite and infinite sets are opposite to each other. The listed below points shows the difference between them.

The elements are countable in a finite set whereas a number of elements are uncountable in the infinite set.
The start and end elements are present in the finite sets therefore there is no continuity whereas infinite sets are endless from the start or end or both sides could also have continuity.
The union of two finite sets is finite whereas the union of two infinite sets is infinite.
The power set of a finite set is finite whereas the power set of an infinite set is infinite.
Finite sets can be easily represented in roster form whereas infinite sets cannot be represented in roster form.

Also Check: Eoc Fsa Warm Ups Algebra 1 Answers

Necessary And Sufficient Conditions For Finiteness

In ZermeloFraenkel set theory without the axiom of choice , the following conditions are all equivalent:

S is a finite set. That is, S can be placed into a one-to-one correspondence with the set of those natural numbers less than some specific natural number.

S has all properties which can be proved by mathematical induction beginning with the empty set and adding one new element at a time.

S can be given a total ordering which is well-ordered both forwards and backwards. That is, every non-empty subset of S has both a least and a greatest element in the subset.

Every one-to-one function from P) into itself is onto. That is, the powerset of the powerset of S is Dedekind-finite .

Every surjective function from P) onto itself is one-to-one.

Every non-empty family of subsets of S has a minimal element with respect to inclusion.

S can be well-ordered and any two well-orderings on it are order isomorphic. In other words, the well-orderings on S have exactly one order type.

If the axiom of choice is also assumed , then the following conditions are all equivalent:

S is a finite set.

Every one-to-one function from S into itself is onto.

Every surjective function from S onto itself is one-to-one.

S is empty or every partial ordering of S contains a maximal element.

Comparison Of Finite And Infinite Sets:

Lets compare the differences between Finite and Infinite set:

The sets could be equal only if their elements are the same, so a set could be equal only if it is a finite set, whereas if the elements are not comparable, the set is infinite.

Factors
The power set of a finite set is also finite	The power set of an infinite set is infinite
Roster form	Can be easily represented in roster form	As the set in infinite set cant be represented in Roster form, so we use three dots to represent the infinity

Don’t Miss: Segment Addition Postulate And Midpoint Worksheet Answer Key

What Does If And Only If Mean In Mathematics

To understand if and only if, we must first know what is meant by a conditional statement. A conditional statement is one that is formed from two other statements, which we will denote by P and Q. To form a conditional statement, we could say if P then Q.

The following are examples of this kind of statement:

If it is raining outside, then I take my umbrella with me on my walk.
If you study hard, then you will earn an A.
If n is divisible by 4, then n is divisible by 2.

Other Concepts Of Finiteness

Finite Sets and Infinite Sets | Don’t Memorise

In ZF set theory without the axiom of choice, the following concepts of finiteness for a set S are distinct. They are arranged in strictly decreasing order of strength, i.e. if a set S meets a criterion in the list then it meets all of the following criteria. In the absence of the axiom of choice the reverse implications are all unprovable, but if the axiom of choice is assumed then all of these concepts are equivalent.

I-finite. Every non-empty set of subsets of S has a -maximal element.
Ia-finite. For every partition of S into two sets, at least one of the two sets is I-finite.
II-finite. Every non-empty -monotone set of subsets of S has a -maximal element.
III-finite. The power set P is Dedekind finite.
IV-finite. S is Dedekind finite.
V-finite. S = 0 or 2S > S|.
VI-finite. S = 0 or S = 1 or S2> S.
VII-finite. S is I-finite or not well-orderable.

The forward implications are theorems within ZF. Counter-examples to the reverse implications in ZF with urelements are found using model theory.

Most of these finiteness definitions and their names are attributed to Tarski 1954 by Howard & Rubin 1998, p.;278. However, definitions I, II, III, IV and V were presented in Tarski 1924, pp.;49, 93, together with proofs for the forward implications. At that time, model theory was not sufficiently advanced to find the counter-examples.

Recommended Reading: What Is Figure Ground Perception Psychology

What Does It Mean When A Function Is Finite

When someone says a real valued function $f$ on $\mathbb$ is finite, does it mean that $|f| \leq M$ for all $x \in \mathbb$ with some $M$ independent of $x$?

15$\begingroup$What you are describing is what is usually called a bounded function. I have not seen the English word finite used in this context. Perhaps you could mention the context in which the term was used.$\endgroup$May 17 ’12 at 4:03
2$\begingroup$As we can see from the contradictory answers , the OP must provide some context to get something useful.$\endgroup$;GEdgarFeb 10 ’13 at 17:42
$\begingroup$Just pointing out that “finite” sometimes also means nonzero . In the sense that $dx$ can be understood as infinitesimal, but still finite interval. Most likely not in this case, but if we are discussing semantics, we should include all the cases.$\endgroup$;orionAug 7 ’15 at 7:12
$\begingroup$For example $x\mapsto \frac$ is finite valued on $]0,\infty 0,\infty [$, $-\infty <f<\infty $, but it’s not valued on $[0,\infty [$ since $f=+\infty $.$\endgroup$

Properties Of Finite Sets

The following finite set conditions are always finite.

A subset of Finite set
The union of two finite sets
The power set of a finite set

Few Examples:

R = {2, 3)

Here, all the P, Q, R are the finite sets because the elements are finite and countable.
R \ P, i.e R is a Subset of P because all the elements of set R are present in P. So, the subset of a finite set is always finite.
P U Q is , so the union of two sets is also finite.

The number of elements of a power set = 2n.

The number of elements of the power set of set P is 24;= 16, as the number of elements of set P is 4. So it shows that the power set of a finite set is finite.

Developing The Binomial Formula

Lets use the last example to look at how we can get a formula that will give us the probability of success on exactly x out of n trials in a binomial experiment.

Example: Planting three tulip bulbs that advertise a 90% maturity rate. Let success be that a tulip matures.

Success: Tulip Matures. p=0.9;;;;;;;;;;;;;;;;;;;;;; ;Failure: Tulip does not mature. q=0.1

Solution: Let X be the random variable that represents the number of bulbs that mature. Then X could be 0,1,2, or 3 successes. X=. We would like to find the probability of each value, P,P,P and P.

Sample Space: We know each tulip has 2 possible outcomes. Mature or not mature . So that means there are =8 possible outcomes.

x=0. This one is easy. There is only one way that can happen . Each tulip has a 0.1 chance of dying. Let m be mature and d be die.

P=1

=103

x=1. For this to occur, one must mature and two must die. But there are 3 ways this can happen. ,,

P=3

=312

x=2. For this to occur, two must mature and one must die. But there are 3 ways this can happen. ,,

P=3

=321

x=3. For this to occur, all three must mature and none die. One way this can happen. ;

P=1

=130

Notice a couple of things about the formulas on the right. The coefficients are the coefficients of the binomial 3. They are also the same numbers you would get if you wrote combinations for n=3 and r=0,1,2 or 3.

;3C0=1

What Is A Finite Set

In the set theory of mathematics, a finite set is defined as a set that has a finite number of elements. In other words, a finite set is a set which you could in principle count and finish counting. For example, is a finite set with four elements. The element in the finite set is a natural number, i.e. non-negative integer. A set S is called finite if there exists a bijection f:S = for natural number n. The empty set is also considered finite. So, S is a finite set, if S admits a bijection to some set of natural numbers of the form .

Don’t Miss: What Is The Molecular Geometry Of Ccl4

What Are The Examples Of Finite And Infinite Sets

Some common examples of finite and infinite sets are given below:

Let W be the set of the days of the week. Then W is a finite set.
Let Q be the set of points on a line. Then Q is an infinite set.
Let R be a set of rats in America, then R is an infinite set.
Let M be a set of months in a year, then M is a finite set.

What Is Infinite Set

If a set is not finite, it is called an infinite set because the number of elements in that set is not countable and also we cannot represent it in Roster form. Thus, infinite sets are also known as uncountable sets.

So, the elements of an Infinite set are represented by 3 dots thus, it represents the infinity of that set.

Cardinality Of Finite And Infinite Sets

The cardinality of a set is basically the size of the set. In the case of finite sets, suppose if set G has only a finite number of elements, its cardinality will be the number of elements in set G. For example,;a set containing the months in a year is a finite set as the elements are countable that is 12, therefore cardinality of this set is 12.

In the case of infinite sets, the cardinality is;”” because the infinite sets can be written in this form N = but the elements cannot be listed or counted.

Examples Of Binomial Experiments

:PARENTHESIS: FINITE-MEAN.

Experiment 1: Flipping two coins; a total of three times with success getting a head.

Success: The coins are the same. p=1/2 ;;;;;;;;; ;;;;;;;;; Failure: The coins are not the same. q=1/2

Experiment 2: Rolling a die ten times with success rolling a three.

Success: Rolling a three. p=1/6;;;;;;;;;;;;; ;;;;;;;;;;; ;;;;;;;;;Failure: Not rolling a three. q=5/6

Experiment 3: Shooting 5 free throws when you have a 72% average with success be making the shot.

Success: Making the shot;; p=0.72;;;;;;;;;;;;;;;;;;;;;;;;;;;; Failure: Not making the shot. q=0.28

Experiment 4:; Guessing on five 4-part multiple choice questions with success being a correct answer.

Success: Answering correctly. p=1/4;;;;;;;;;;;;;;;;;;;;;;;;; Failure: Answering incorrectly. q=3/4

Experiment 5: Selecting an item from a group that contains 7 good items and 3 defective. Performing this experiment four times constitutes a Binomial experiment.

Success: Selecting a defect. p=3/10;;;;;;;;;;;;;;;; ;;;;;;;;;; Failure: Not selecting a defect. q=7/10

Experiment 6: Trying a new medicine out on twenty people with success being the symptom eliminated. Assume the company says they get an 80% success rate.

Success: Symptom Eliminated. p=0.8;;;;;;;;;;;;;;;;;;;;;;;;; Failure: Symptom Not Eliminated. q=0.2

Experiment 7: Planting three tulip bulbs that advertise a 90% maturity rate. Let success be that a tulip matures.

Success: Tulip Matures. p=0.9;;;;;;;;;;;;;;;;;;;;;;;;;;;;;;;; Failure: Tulip does not mature. q=0.1

Also Check: Angle Addition Postulate Practice