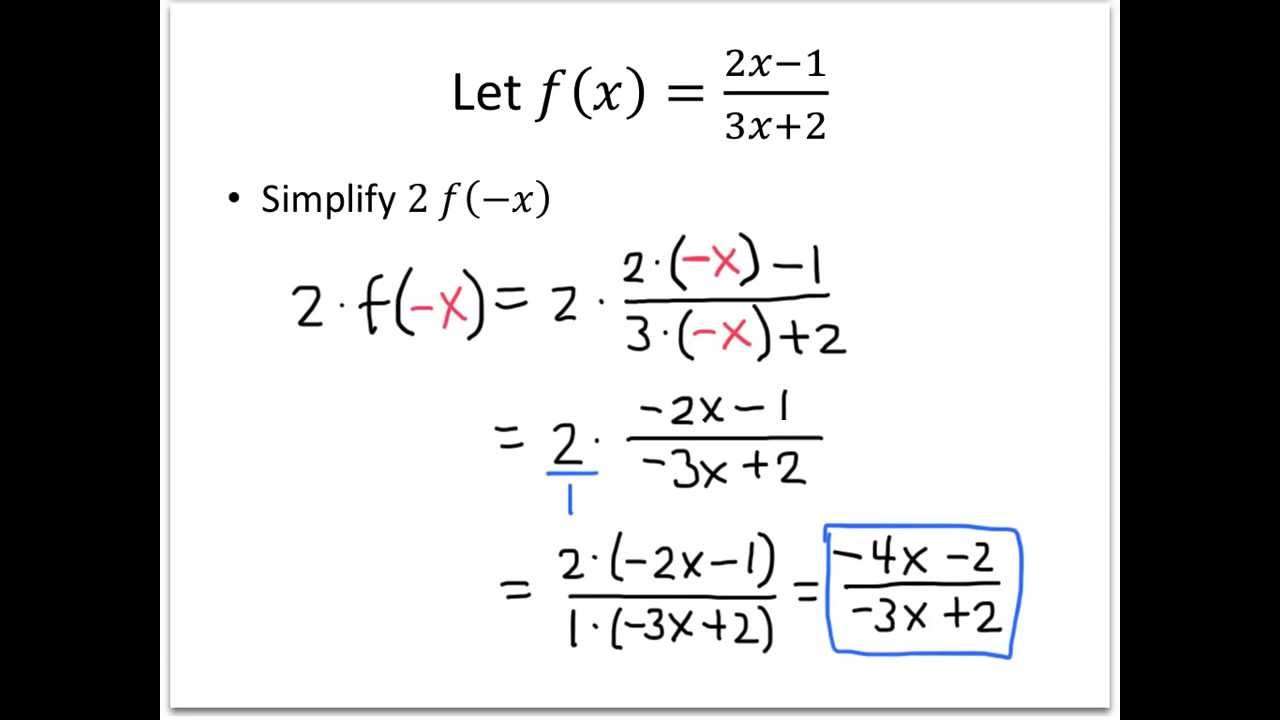
Example : Evaluating Functions For Algebraic Expressions
Find f when f = 5x 7
Write out the function for x using function notation, replacing the x with an empty set of brackets.
Replace the x in the function with the number or algebraic term in the brackets next to the name of the function.
Apply the correct operations to the number or term as appropriate and simplify.
Summary Of Polynomial Functions
A polynomial function is a function such as a quadratic, cubic, quartic, among others, that only has non-negative integer powers of x. A polynomial of degree n is a function that has the general form:
$$f=a_^n}+a_^}++a_^2}+a_x+a_$$
where the coefficients a are all real numbers. Although the general form looks very complicated, the particular examples are simpler. For example,
$latex f=2^3}+4^2}+2x$
is a polynomial function of degree 3 since 3 is the largest power of the function. And the function:
$$f=-4^5}+2^4}+3^2}+6$$
is a polynomial function of degree 5 since 5 is the greatest power of the function.
Example: Finding An Equation Of A Function
Express the relationship 2n+6p=12 as a function p=f\left, if possible.
To express the relationship in this form, we need to be able to write the relationship where p is a function of n, which means writing it as p= expression involving n.
\begin& 2n+6p=12\\ & 6p=12 – 2n & & \text2n\text. \\ & p=\frac & & \text. \\ & p=\frac-\frac \\ & p=2-\fracn \end
Therefore, p as a function of n is written as
p=f\left=2-\fracn
Analysis of the Solution
It is important to note that not every relationship expressed by an equation can also be expressed as a function with a formula.
Watch this video to see another example of how to express an equation as a function.
You May Like: 5 Areas Of Chemistry
Representing Functions Using Tables
A common method of representing functions is in the form of a table. The table rows or columns display the corresponding input and output values. In some cases, these values represent all we know about the relationship other times, the table provides a few select examples from a more complete relationship.
Table \ lists the input number of each month , \, and so on) and the output value of the number of days in that month. This information represents all we know about the months and days for a given year . Note that, in this table, we define a days-in-a-month function \ where \\) identifies months by an integer rather than by name.
| Month number, \ |
Evaluating A Function Given In Tabular Form
As we saw above, we can represent functions in tables. Conversely, we can use information in tables to write functions, and we can evaluate functions using the tables. For example, how well do our pets recall the fond memories we share with them? There is an urban legend that a goldfish has a memory of 3 seconds, but this is just a myth. Goldfish can remember up to 3 months, while the beta fish has a memory of up to 5 months. And while a puppys memory span is no longer than 30 seconds, the adult dog can remember for 5 minutes. This is meager compared to a cat, whose memory span lasts for 16 hours.
The function that relates the type of pet to the duration of its memory span is more easily visualized with the use of a table. See the table below.
| Pet | |
|---|---|
| Beta fish | 3600 |
At times, evaluating a function in table form may be more useful than using equations. Here let us call the function P.
The domain of the function is the type of pet and the range is a real number representing the number of hours the pets memory span lasts. We can evaluate the function P at the input value of goldfish. We would write P\left=2160. Notice that, to evaluate the function in table form, we identify the input value and the corresponding output value from the pertinent row of the table. The tabular form for function P seems ideally suited to this function, more so than writing it in paragraph or function form.
Also Check: Beth Thomas Child Of Rage Today
Composition Of Functions Practice Problems
Put into practice what you have learned about the composition of functions by solving the following problems. Choose an answer and check it to see if you got the correct answer. Check out the solved examples above in case you need help.
Find the composition $latex f)$ if we have the functions $latex f=4x+2$ and $latex g=x+2$.
Choose an answer
Determining Whether A Relation Represents A Function
A relation is a set of ordered pairs. The set of the first components of each ordered pair is called the domain and the set of the second components of each ordered pair is called the range. Consider the following set of ordered pairs. The first numbers in each pair are the first five natural numbers. The second number in each pair is twice that of the first.
The domain is \. The range is \.
Note that each value in the domain is also known as an input value, or independent variable, and is often labeled with the lowercase letter \. Each value in the range is also known as an output value, or dependent variable, and is often labeled lowercase letter \.
A function \ is a relation that assigns a single value in the range to each value in the domain. In other words, no \-values are repeated. For our example that relates the first five natural numbers to numbers double their values, this relation is a function because each element in the domain, , is paired with exactly one element in the range, \.
Now lets consider the set of ordered pairs that relates the terms even and odd to the first five natural numbers. It would appear as
Notice that each element in the domain, is not paired with exactly one element in the range, \. For example, the term odd corresponds to three values from the range, \ and the term even corresponds to two values from the range, \. This violates the definition of a function, so this relation is not a function.
Function
Solution
Solution
Read Also: How To Figure Out Displacement In Physics
Algebra Functions On Act Math: Lesson And Practice Questions
Functions. Just hearing the word is enough to send some students running for the hills. But never fear! Though function problems are considered some of the more challenging questions on the ACT, this is only due to the fact that most of you will be far more used to dealing with other math topics than you are functions.
On the ACT, question difficulty is categorized by how familiar you are likely to be with any given question, and the only way to combat this challenge is to practice and get used to dealing with questions that are a little less familiar to you. You will generally see 3-4 function questions on any given ACT, so for those of you who are not yet comfortable with functions , this guide is for you.
This will be your complete guide to ACT functions. We’ll walk you through exactly what functions mean, how to use, manipulate, and identify them, and exactly what kind of function problems you’ll see on the ACT.
Solutions To The Above Questions
Don’t Miss: How To Find The Half Life
Function Notation Explanation & Examples
The concept of functions was developed in the seventeenth century when Rene Descartes used the idea to model mathematical relationships in his book Geometry. The term function was then introduced by Gottfried Wilhelm Leibniz fifty years later after publication of Geometry.
Later, Leonhard Euler formalized the usage of functions when he introduced the concept of function notation y = f . It was until 1837 when Peter Dirichlet a German mathematician gave the modern definition of a function.
What Is A Function
In mathematics, a function is a set of inputs with a single output in each case. Every function has a domain and range. The domain is the set of independent values of the variable x for a relation or a function is defined. In simple words, the domain is a set of x-values that generate the real values of y when substituted in the function.
On the other hand, the range is a set of all possible values that a function can produce. The range of a function can be expressed in interval notation or inform of inequalities.
You May Like: How To Find Half Life Chemistry
Function Terms And Definitions
Now that we’ve seen what functions do, let’s talk about the pieces of a function.
Functions will be presented to you either by their equations, their tables, or by their graph . Let’s look at a sample function equation and break it down into its components.
An example of a function:
$f = x^2 + 12$
$f$ is the name of the function
$, $g$, $r$, or anything else.)
$$ is the input
$ or $f$ are both functions with the inputs of $q$ and $\bananas$, respectively.)
$x^2 + 5$ is the equation that gives us the output once we plug in the input value of $x$
An ordered pair is the coupling of a particular input with its output for any given function. So for the function $f = x – 6$, with an input of 2, we can have an ordered pair of:
$f = x – 6$
$f = -4$
So our ordered pair is $$.
Ordered pairs also act as coordinates, so we can use them to graph our function graph.
Now that we have all of our function pieces and definitions, let’s look at how they work together.
For Function Equations And Nested Equations
#1: Always work inside out
Nested functions can look beastly and difficult, but take them piece by piece. Work out the equation in the center and then build outwards slowly, so as not to get any of your variables or equations mixed up.
#2: Remember to FOIL
It is quite common for ACT to make you square an equation. This is because many students get these types of questions wrong and distribute their exponents instead of squaring the entire expression.
If you don’t properly FOIL, then you will get these questions wrong. Whenever possible, try not to let yourself lose points due to these kinds of careless errors.
Ready to test your function knowledge?
Now let’s put our function knowledge to the test, using real ACT math problems.
1. A function $f$ is defined as $f=-8x^2$. What is $f$?
F. -72
Our final answer is A, -70.
Congrats! You’ve mastered ACT functions!
Also Check: Did Michael Jackson Have Biological Kids
Roots Of Polynomial Functions
When we have $latex =0$, we know that a and b are the roots of the function $latex f=$. Therefore, we can find the polynomial roots by forming an equation by setting the polynomial part of the function equal to zero and factoring or solving for x.
It is also possible to use the opposite of this. For example, if we have that a and b are roots, we know that the polynomial function with these roots must be $latex f = $, or a multiple of this.
If $latex x=3$ and $latex x=-4$ are the roots of the polynomial function, then this function must be $latex f=$, or a constant multiple of this.
Finding Input And Output Values Of A Function
When we know an input value and want to determine the corresponding output value for a function, we evaluate the function. Evaluating will always produce one result because each input value of a function corresponds to exactly one output value.
When we know an output value and want to determine the input values that would produce that output value, we set the output equal to the functions formula and solve for the input. Solving can produce more than one solution because different input values can produce the same output value.
You May Like: Paris Jackson’s Biological Father
What Is A Function Notation
Notation can be defined as a system of symbols or signs that denote elements such as phrases, numbers, words etc.
Therefore, function notation is a way in which a function can be represented using symbols and signs. Function notation is a simpler method of describing a function without a lengthy written explanation.
The most frequently used function notation is f which is read as f of x. In this case, the letter x, placed within the parentheses and the entire symbol f, stand for the domain set and range set respectively.
Although f is the most popular letter used when writing function notation, any other letter of the alphabet can also be used either in upper or lower case.
How To Solve A Function Problem
Now that you’ve seen all the different kinds of function problems in action, let’s look at some tips and strategies for solving function problems.
For clarity, we’ve split these strategies into multiple sectionstips for all function problems and tips for function problems by type. So let’s look at each strategy.
Read Also: Valence Electrons In Ccl4
Evaluation Of Functions In Algebraic Forms
When we have a function in formula form, it is usually a simple matter to evaluate the function. For example, the function \=53x^2\) can be evaluated by squaring the input value, multiplying by 3, and then subtracting the product from 5.
How To: Given the formula for a function, evaluate.
Given the formula for a function, evaluate.
Identifying Basic Toolkit Functions
In this text, we will be exploring functionsthe shapes of their graphs, their unique characteristics, their algebraic formulas, and how to solve problems with them. When learning to read, we start with the alphabet. When learning to do arithmetic, we start with numbers. When working with functions, it is similarly helpful to have a base set of building-block elements. We call these our toolkit functions, which form a set of basic named functions for which we know the graph, formula, and special properties. Some of these functions are programmed to individual buttons on many calculators. For these definitions we will use x as the input variable and \\) as the output variable.
We will see these toolkit functions, combinations of toolkit functions, their graphs, and their transformations frequently throughout this book. It will be very helpful if we can recognize these toolkit functions and their features quickly by name, formula, graph, and basic table properties. The graphs and sample table values are included with each function shown in Table \.
| Name |
|---|
- Absolute value function \=|x|\)
- Quadratic function \=x^2\)
- Cubic function \=x^3\)
- Reciprocal function \=\dfrac\)
- Reciprocal squared function \=\frac\)
- Square root function \=\sqrt\)
- Cube root function \=3\sqrt\)
Don’t Miss: Fsa Warm Ups Grade 5
Summary Of Composition Of Functions
The composition of functions is an operation where two functions like $latex f$ and $latex g$ generate a new function like $latex h$ in such a way that we have $latex h=g)$.
This means that the function $latex g$ is applied to the function $latex f$. This means that basically a function is applied to the result of another function.
Symbol: A composition of functions is also denoted as $latex $, where the small circle, $latex \circ$, is the symbol of the composition of functions. We cannot replace the circle with a point since this indicates the product of two functions.
Domain: The composition $latex f)$ is read as f of g of x. In this composition, the domain of the function f becomes $latex g$ since the domain is the set of all input values of the function.
To apply the composition $latex f\circ g$, we carry out the following two steps:
Step 1: We apply the function g to the input x and obtain the result $latex g$ as the output.
Step 2: We apply the function f using $latex g$ as the input and we get the result $latex f)$ as the output.